Chapter 2, Problem 2.52P

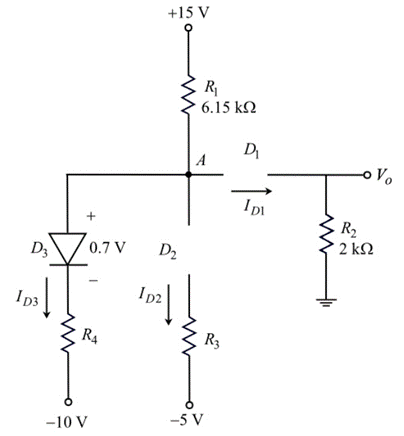
The cut−in voltage of each diode in the circuit shown in Figure P2.52 is $V_{\gamma }=0.7\text{V}$ . Determine $I_{D1},I_{D2},I_{D3}$ , and $V_A$ for (a) $R_3=14\text{k}\Omega ,R_4=24\text{k}\Omega$ ; (b) $R_3=3.3\text{k}\Omega ,R_4=5.2\text{k}\Omega$ ; and (c) $R_3=3.3\text{k}\Omega ,R_4=1.32\text{k}\Omega$ .

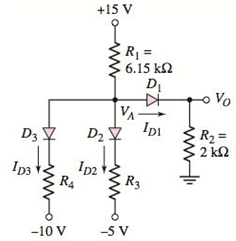
Figure P2.52

To determine

The values of $I_{D1},I_{D2},I_{D3},\text{ and }{V}_A$

Answer to Problem 2.52P

The current flowing through diodes is

  $I_{D3}=0.5\text{ mA}$

  $I_{D2}=0.5\text{ mA}$ , $I_{D1}=1\text{ mA}$ and $V_A=2.7\text{ V}$

Explanation of Solution

Given:

  

  $\begin{array}{l}{R}_3=14k\Omega \\ R_4=24k\Omega \end{array}$

Calculation:

The cut-in voltage for each diode is, $V_{\gamma }=0.7\text{V}$

Assume all diodes are ON.

Draw the circuit diagram with node voltages and cut-in voltages.

  

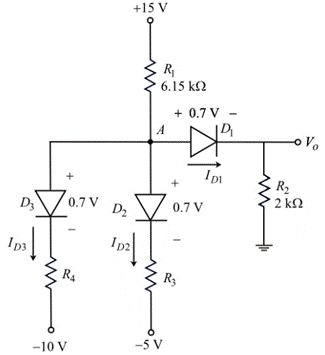
Apply Kirchhoff’s current law node A.

  $\begin{array}{l}\frac{V_A-15}{6.15k}+\frac{V_A-0.7}{2k}+\frac{V_A-0.7-\left(-5\right)}{R_3}+\frac{V_A-0.7-\left(-10\right)}{R_4}=0\\ \frac{V_A-15}{6.15k}+\frac{V_A-0.7}{2k}+\frac{V_A+4.3}{R_3}+\frac{V_A+9.3}{R_4}=0\end{array}$

Substitute $14\text{k}\Omega$ for $R_3$ and $24\text{k}\Omega$ for $R_4$ .

  $\begin{array}{l}\frac{V_A-15}{6.15k}+\frac{V_A-0.7}{2k}+\frac{V_A+4.3}{14k}+\frac{V_A+9.3}{24k}=0\\ \frac{V_A-15}{6.15}+\frac{V_A-0.7}{2}+\frac{V_A+4.3}{14}+\frac{V_A+9.3}{24}=0\\ \left(\frac{1}{6.15}+\frac{1}{2}+\frac{1}{14}+\frac{1}{24}\right)V_A-\frac{15}{6.15}-\frac{0.7}{2}+\frac{4.3}{14}+\frac{9.3}{24}=0\\ 0.7756V_A-2.094=0\\ V_A=2.7\text{ V}\end{array}$

Therefore, the voltage at node $\text{A, }{V}_A$ is $2.7\text{ V}$

Calculate the current flowing through diode, $D_1$ .

  $I_{D1}=\frac{V_A-0.7}{2k}$

Substitute $2.7\text{V}$ for $V_A$ .

  $\begin{array}{l}{I}_{D1}=\frac{2.7-0.7}{2k}\\ =\frac{2}{2k}\\ =1\text{ mA}\end{array}$

Therefore, the current flowing through diode $D_1,$ is $I_{D1}=1\text{ mA}$ .

Calculate the current flowing through diode, $D_2$ .

  $I_{D2}=\frac{V_A-0.7-\left(-5\right)}{R_3}$

Substitute $2.7\text{V}$ for $V_A$ and $14\text{ k}\Omega$ for $R_3$ .

  $\begin{array}{l}{I}_{D2}=\frac{2.7-0.7+5}{14k}\\ =\frac{7}{14k}\\ =0.5\text{ mA}\end{array}$

Therefore, the current flowing through diode $D_2,$ is $I_{D2}=0.5\text{ mA}$ .

Calculate the current flowing through diode, $D_3$ .

  $I_{D3}=\frac{V_A-0.7-\left(-10\right)}{R_4}$

Substitute $2.7\text{V}$ for $V_A$ and $24\text{ k}\Omega$ for $R_4$ .

  $\begin{array}{l}{I}_{D3}=\frac{2.7-0.7+10}{24k}\\ =\frac{12}{24k}\\ =0.5\text{ mA}\end{array}$

Therefore, the current flowing through diodes is $I_{D3}=0.5\text{ mA}$

Conclusion:

Therefore, the current flowing through diodes is

  $I_{D3}=0.5\text{ mA}$

  $I_{D2}=0.5\text{ mA}$ , $I_{D1}=1\text{ mA}$ and $V_A=2.7\text{ V}$

To determine

The values of $I_{D1},I_{D2},I_{D3},\text{ and }{V}_A$

Answer to Problem 2.52P

The current flowing through diodes is $V_A=-0.991\text{V}$ , $I_{D1}\text{= }0\text{ A}$ , $I_{D2}=1.003\text{ mA}$ and $I_{D3}=1.6\text{ mA}$

Explanation of Solution

Given:

  $\begin{array}{l}{R}_3=3.3k\Omega \\ R_4=5.2k\Omega \end{array}$

Calculation:

Assume diode $D_1$ is OFF and remaining diodes are ON.

Draw the circuit diagram with node voltages and cut-in voltages.

  

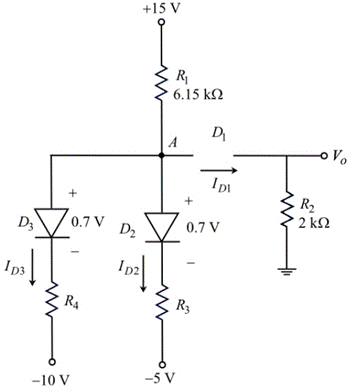
Apply Kirchhoff’s current law node A.

  $\begin{array}{l}\frac{V_A-15}{6.15k}+\frac{V_A-0.7-\left(-5\right)}{R_3}+\frac{V_A-0.7-\left(-10\right)}{R_4}=0\\ \frac{V_A-15}{6.15k}+\frac{V_A+4.3}{R_3}+\frac{V_A+9.3}{R_4}=0\end{array}$

Substitute $3.3\text{k}\Omega$ for $R_3$ and $5.2\text{k}\Omega$ for $R_4$ .

  $\begin{array}{l}\frac{V_A-15}{6.15k}+\frac{V_A+4.3}{3.3k}+\frac{V_A+9.3}{5.2k}=0\\ \frac{V_A-15}{6.15}+\frac{V_A+4.3}{3.3}+\frac{V_A+9.3}{5.2}=0\\ \left(\frac{1}{6.15}+\frac{1}{3.3}+\frac{1}{5.2}\right)V_A-\frac{15}{6.15}+\frac{4.3}{3.3}+\frac{9.3}{5.2}=0\\ 0.6579V_A+0.6525=0\\ V_A=-\frac{0.6525}{0.6579}\\ =-0.991\text{V}\end{array}$

Therefore, the voltage at node $\text{A, }{V}_A$ is $-0.991\text{V}$

The diode $D_1$ is reverse biased. Therefore, the current flowing through diode $D_1$ is zero.

That is, $I_{D1}=0\text{ A}$ .

Therefore, the current flowing through diode $D_1,$

  $I_{D1}\text{= }0\text{ A}$

Calculate the current flowing through diode, $D_2$ .

  $I_{D2}=\frac{V_A-0.7-\left(-5\right)}{R_3}$

Substitute $-0.991\text{V}$ for $V_A$ and $\text{3}\text{.3 k}\Omega$ for $R_3$ .

  $\begin{array}{l}{I}_{D2}=\frac{-0.991-0.7+5}{3.3k}\\ =\frac{3.309}{3.3k}\\ =1.003\text{ mA}\end{array}$

Therefore, the current flowing through diode $D_2,$ is $I_{D2}=1.003\text{ mA}$ .

Calculate the current flowing through diode, $D_3$ .

  $I_{D3}=\frac{V_A-0.7-\left(-10\right)}{R_4}$

Substitute $-0.991\text{V}$ for $V_A$ and $\text{5}\text{.2 k}\Omega$ for $R_4$ .

  $\begin{array}{l}{I}_{D3}=\frac{-0.991-0.7+10}{5.2k}\\ =\frac{8.309}{5.2k}\\ =1.6\text{ mA}\end{array}$

Therefore, the current flowing through diode $D_3,$ is $I_{D3}=1.6\text{ mA}$ .

Conclusion:

Therefore, the current flowing through diodes is $V_A=-0.991\text{V}$ , $I_{D1}\text{= }0\text{ A}$ , $I_{D2}=1.003\text{ mA}$ and $I_{D3}=1.6\text{ mA}$

To determine

The values of $I_{D1},I_{D2},I_{D3},\text{ and }{V}_A$

Answer to Problem 2.52P

The current flowing through diodes is $V_A=-5\text{V}$ , $I_{D1}=0\text{ A}$ , $I_{D2}=0\text{ A}$

  $I_{D3}=3.25\text{ mA}$

Explanation of Solution

Given:

  $\begin{array}{l}{R}_3=3.3k\Omega \\ R_4=1.32k\Omega \end{array}$

Calculation:

Assume diode $D_1$ and $D_2$ are OFF and diode $D_3$ is ON.

Draw the circuit diagram with node voltages and cut-in voltages.

  

Apply Kirchhoff’s current law node A.

  $\begin{array}{l}\frac{V_A-15}{6.15k}+\frac{V_A-0.7-\left(-10\right)}{R_4}=0\\ \frac{V_A-15}{6.15k}+\frac{V_A+9.3}{R_4}=0\end{array}$

Substitute $1.32\text{k}\Omega$ for $R_4$ .

  $\begin{array}{l}\frac{V_A-15}{6.15k}+\frac{V_A+9.3}{1.32k}=0\\ \frac{V_A-15}{6.15}+\frac{V_A+9.3}{1.32}=0\\ \left(\frac{1}{6.15}+\frac{1}{1.32}\right)V_A-\frac{15}{6.15}+\frac{9.3}{1.32}=0\\ 0.92V_A+4.606=0\\ V_A=-5\text{V}\end{array}$

Therefore, the voltage at node $\text{A, }{V}_A$ is $-5\text{V}$ .

The Diode $D_1$ and $D_2$ are reverse biased. Therefore, the current flowing through diode $D_1$ is zero.

That is, $I_{D1}=0\text{ A}$ .

Therefore, the current flowing through diode $D_1,I_{D1}\text{ is }0\text{ A}\text{.}$

The current flowing through the diode, $D_2$ is zero.

That is,

  $I_{D2}=0\text{ A}\text{.}$

Therefore, the current flowing through diode $D_2,I_{D2}\text{ is }0\text{ A}\text{.}$

Calculate the current flowing through diode, $D_3$ .

  $I_{D3}=\frac{V_A-0.7-\left(-10\right)}{R_4}$

Substitute $-5\text{V}$ for $V_A$ and $\text{1}\text{.32 k}\Omega$ for $R_4$ .

  $\begin{array}{l}{I}_{D3}=\frac{-5-0.7+10}{1.32k}\\ =\frac{4.3}{1.32k}\\ =3.25\text{ mA}\end{array}$

Therefore, the current flowing through diode $D_3,$ is $I_{D3}=3.25\text{ mA}$ .

Conclusion:

Therefore, the current flowing through diodes is $V_A=-5V$ , $I_{D1}=0\text{ A}$ , $I_{D2}=0\text{ A}$

  $I_{D3}=3.25\text{ mA}$