Chapter 9, Problem 69A

(a)

To determine

To Identify: The before and after situations, and to draw a diagram of both.

Explanation of Solution

Given:

Mass of the fullback is $m_{FB}=95\text{ kg}$

Initial velocity of the fullback is $v_{FBi}=8.2\text{ m/s}$

Mass of the defensive tackle is $m_{DT}=128\text{ kg}$

Defensive tackle moving in the direction opposite to that of fullback.

Final speed of both players is zero. That is $v_{FBf}=v_{DTf}=0\text{ m/s}$

Before collision:

  $m_{FB}=95\text{ kg}$

  $v_{FBi}=8.2\text{ m/s}$

  $m_{DT}=128\text{ kg}$

  $v_{DTi}=\text{?}$

After collision:

  $m=m_{FB}+m_{DT}=\left(95\text{ kg}\right)+\left(128\text{ kg}\right)=223\text{ kg}$

  $v_{FBf}=v_{DTf}=0\text{ m/s}$

The diagrams showing the situation before and after the collision between the players are as shown in figure $\text{1}$ and figure $\text{2}$ respectively.

  

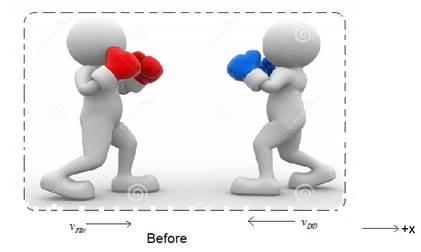
Figure 1

  

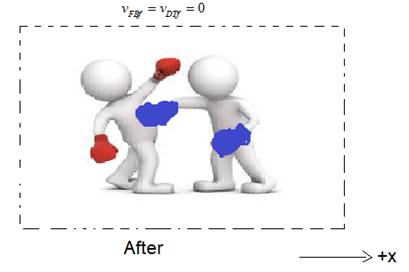
Figure 2

(b)

To determine

The fullback’s momentum before collision.

Answer to Problem 69A

  $p_{FBi}=7.8\times {10}^2\text{ kg}\text{.m/s}$

Explanation of Solution

Given:

Mass of the fullback is $m_{FB}=95\text{ kg}$

Initial velocity of the fullback is $v_{FBi}=8.2\text{ m/s}$

Formula used:

Momentum $\left(p\right)$ is the product of mass $\left(m\right)$ and velocity $\left(v\right)$ of a moving body. That is,

  $p=mv$

Initial momentum of fullback can be calculated using the equation,

  $p_{FBi}=m_{FB}{v}_{FBi}$$\left(1\right)$

Where,

  $m_{FB}$ is the mass of a fullback

  $v_{FBi}$ is initial velocity of a fullback

Calculation:

Substituting the numerical values in equation $\left(1\right)$ ,

  $p_{FBi}=\left(95\text{ kg}\right)\left(8.2\text{ m/s}\right)$

  $=7.8\times {10}^2\text{ kg}\cdot \text{m/s}$

Conclusion:

The fullback’s momentum before collision is $7.8\times {10}^2\text{ kg}\cdot \text{m/s}$ .

(c)

To determine

The change in fullback’s momentum.

Answer to Problem 69A

  $\Delta {\text{p}}_{FB}=-7.8\times {10}^2\text{ kg}\cdot \text{m/s}$

Explanation of Solution

Given:

Mass of the fullback is $m_{FB}=95\text{ kg}$

Initial velocity of the fullback is $v_{FBi}=8.2\text{ m/s}$

Final velocity of the fullback is $v_{FBf}=0\text{ m/s}$

Formula used:

Change in momentum = final momentum − initial momentum

That is,

  $\Delta {\text{p = p}}_f-p_i$

Momentum $\left(p\right)$ is the product of mass $\left(m\right)$ and velocity $\left(v\right)$ of a moving body. That is,

  $p=mv$

Hence the initial momentum of the fullback can be written as,

  $p_{FBi}=m_{FB}{v}_{FBi}$

Where,

  $m_{FB}$ is the mass of the fullback

  $v_{FBi}$ is initial velocity of the fullback

And, the final momentum of the fullback can be written as,

  $p_{FBf}=m_{FB}{v}_{FBf}$

Where $v_{FBf}$ is the final velocity of the fullback.

Therefore, change in momentum of the fullback is,

  $\Delta {\text{p}}_{FB}\text{ = }{p}_{FBf}\text{- }{p}_{FBi}$$\left(2\right)$

Substituting for $p_{FBf}$ and $p_{FBi}$ in equation $\left(2\right)$ ,

  $\Delta {\text{p}}_{FB}\text{ = }{m}_{FB}{v}_{FBf}\text{- }{m}_{FB}{v}_{FBi}$$\left(3\right)$

Calculation:

Substituting the numerical values in equation $\left(3\right)$ ,

  $\Delta {\text{p}}_{FB}\text{ =}\left(95\text{ kg}\right)\left(0\text{ m/s}\right)-\left(95\text{ kg}\right)\left(8.2\text{ m/s}\right)$

  $=0-7.8\times {10}^2\text{ kg}\cdot \text{m/s}$

  $=-7.8\times {10}^2\text{ kg}\cdot \text{m/s}$

Conclusion:

The change in fullback’s momentum is $-7.8\times {10}^2\text{ kg}\cdot \text{m/s}$ .

(d)

To determine

The change in defensive tackle’s momentum.

Answer to Problem 69A

  $\Delta p_{DT}=7.8\times {10}^2\text{ kg}\cdot \text{m/s }$

Explanation of Solution

Since defensive tackle running in the direction opposite to the direction of motion of fullback, the change in defensive tackle’s momentum is $7.8\times {10}^2\text{ kg}\cdot \text{m/s }$ .

(e)

To determine

The defensive tackle’s original momentum.

Answer to Problem 69A

  $p_{DTi}=-7.8\times {10}^2\text{ kg}\cdot \text{m/s }$

Explanation of Solution

Since the defensive tackle running in the direction opposite to the direction of motion of fullback, the initial momentum of defensive tackle is $-7.8\times {10}^2\text{ kg}\cdot \text{m/s }$ .

(f)

To determine

The Defensive tackles initial velocity.

Answer to Problem 69A

  $v_{DTi}=-6.1\text{ m/s}$

Explanation of Solution

Given:

Mass of the defensive tackle is $m_{DT}=128\text{ kg}$

Formula used:

Momentum $\left(p\right)$ is the product of mass $\left(m\right)$ and velocity $\left(v\right)$ of a moving body. That is,

  $p=mv$

Hence the initial momentum of the defensive tackle can be written as,

  $p_{DTi}=m_{DT}{v}_{DTi}$

  $\Rightarrow v_{DTi}=\frac{p_{DTi}}{m_{DT}}$$\left(4\right)$

Where,

  $m_{DT}$ is the mass of the defensive tackle

  $v_{DTi}$ is initial velocity of the defensive tackle

Calculation:

From the part (e), the initial momentum of defensive tackle is $-7.8\times {10}^2\text{ kg}\text{.m/s }$

Initial velocity of the defensive tackle can be calculated by substituting the numerical values in equation $\left(4\right)$ ,

  $v_{DTi}=\frac{-7.8\times {10}^2\text{ kg}\cdot \text{m/s }}{128\text{ kg}}$

  $=-6.1\text{ m/s}$

Conclusion:

The Defensive tackle’s initial velocity is $-6.1\text{ m/s}$ .