Steel Design (Activate Learning with these NEW titles from Engineering!)
6th Edition
ISBN: 9781337094740
Author: Segui, William T.
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 4, Problem 4.7.8P
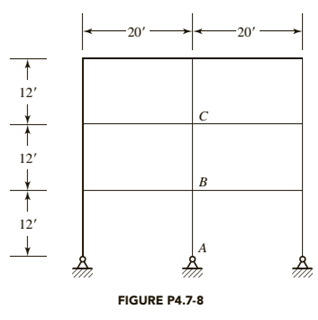
The frame shown in Figure P4.7-8 is unbraced, and bending is about the x-axis of the members. All beams are
a. Determine the effective length factor
b. Determine the effective length factor
c. If
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
- Find reactions and draw Shear and Bending Moment Diagram.
30 N
15 N/m
D
B
A
2 m
1 m
2 mm
: A 5ms- long current pulse is desired for two linear lamps connected in
series and pumped at a total energy input of (1KJ). Each of lamps has
an arc-length of (10cm) and a bore of (1cm). If we assume a peak
current of (i, -650A). Design a multiple mesh network including
number of LC sections, inductance and capacitance per section and
capacitor voltage.
Laser design
What would be the best way to handle when a contractor misses a concrete pour deadline which causes delays for other contractors?
Chapter 4 Solutions
Steel Design (Activate Learning with these NEW titles from Engineering!)
Ch. 4 - Prob. 4.3.1PCh. 4 - Prob. 4.3.2PCh. 4 - Prob. 4.3.3PCh. 4 - Prob. 4.3.4PCh. 4 - Prob. 4.3.5PCh. 4 - Prob. 4.3.6PCh. 4 - Prob. 4.3.7PCh. 4 - Prob. 4.3.8PCh. 4 - Prob. 4.4.1PCh. 4 - Prob. 4.4.2P
Ch. 4 - Prob. 4.6.1PCh. 4 - Prob. 4.6.2PCh. 4 - Prob. 4.6.3PCh. 4 - Prob. 4.6.4PCh. 4 - Prob. 4.6.5PCh. 4 - Prob. 4.6.6PCh. 4 - Prob. 4.6.7PCh. 4 - Prob. 4.6.8PCh. 4 - Prob. 4.6.9PCh. 4 - Prob. 4.7.1PCh. 4 - Prob. 4.7.2PCh. 4 - Prob. 4.7.3PCh. 4 - Use A992 steel and select a W14 shape for an...Ch. 4 - Prob. 4.7.5PCh. 4 - Prob. 4.7.6PCh. 4 - Prob. 4.7.7PCh. 4 - The frame shown in Figure P4.7-8 is unbraced, and...Ch. 4 - Prob. 4.7.9PCh. 4 - Prob. 4.7.10PCh. 4 - Prob. 4.7.11PCh. 4 - Prob. 4.7.12PCh. 4 - Prob. 4.7.13PCh. 4 - Prob. 4.7.14PCh. 4 - Prob. 4.8.1PCh. 4 - Prob. 4.8.2PCh. 4 - Prob. 4.8.3PCh. 4 - Prob. 4.8.4PCh. 4 - Prob. 4.9.1PCh. 4 - Prob. 4.9.2PCh. 4 - Prob. 4.9.3PCh. 4 - Prob. 4.9.4PCh. 4 - Prob. 4.9.5PCh. 4 - Prob. 4.9.6PCh. 4 - Prob. 4.9.7PCh. 4 - Prob. 4.9.8PCh. 4 - Prob. 4.9.9PCh. 4 - Prob. 4.9.10PCh. 4 - Prob. 4.9.11PCh. 4 - Prob. 4.9.12P
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, civil-engineering and related others by exploring similar questions and additional content below.Similar questions
- Please solve manuallyarrow_forward. The free fall distance was 1753 mm. The times for the release and catch recorded on the fall experiments were in millisecond: 222.22 800.00 61.11 641.67 0.00 588.89 11.11 588.89 8.33 588.89 11.11 588.89 5.56 586.11 2.78 583.33 Calculate the time taken for the fall for each experiment. Calculate for each fall the acceleration based on time and distance. Calculate the mean of the accelerations. Give in the answer window the calculated mean of accelerations in m/s2.arrow_forwardneed help. explain plzarrow_forward
- -Design the traffic signal intersection using all red 2 second, for all phase the truck percent 5% for all movement, and PHF -0.95 Check for capacity only Approach Through volume Right volume Left volume Lane width Number of lane Veh/hr Veh/hr Veh/hr m North 700 100 150 3.0 3 south 600 75 160 3.0 3 East 300 80 50 4.0 R west 400 50 55 4.0 2arrow_forwardneed helparrow_forwardFor the beam show below, draw A.F.D, S.F.D, B.M.D A 2 N M 10 kN.m B 2 M Carrow_forward
- B: Find the numerical solution for the 2D equation below and calculate the temperature values for each grid point shown in Fig. 2 (show all steps). (Do only one trail using following initial values and show the final matrix) T₂ 0 T3 0 I need a real solution, not artificial intelligence locarrow_forward: +0 العنوان use only Two rods fins) having same dimensions, one made orass (k = 85 Wm K) and the mer of copper (k = 375 W/m K), having of their ends inserted into a furna. At a section 10.5 cm a way from furnace, the temperature of brass rod 120 Find the distance at which the ame temperature would be reached in the per rod ? both ends are ex osed to the same environment. ns 2.05 ۲/۱ ostrararrow_forwardI need a real solution, not artificial intelligencearrow_forward
- I need detailed help solving this exercise from homework of Applied Mechanics. I do not really understand how to do, please do it step by step, not that long but clear. Thank you!arrow_forwardI need detailed help solving this exercise from homework of Applied Mechanics. I do not really understand how to do, please do it step by step, not that long but clear. Thank you!arrow_forwardI need detailed help solving this exercise from homework of Applied Mechanics. I do not really understand how to do, please do it step by step, not that long but clear. Thank you!arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Structural Analysis (10th Edition)Civil EngineeringISBN:9780134610672Author:Russell C. HibbelerPublisher:PEARSON
Principles of Foundation Engineering (MindTap Cou...Civil EngineeringISBN:9781337705028Author:Braja M. Das, Nagaratnam SivakuganPublisher:Cengage Learning
Fundamentals of Structural AnalysisCivil EngineeringISBN:9780073398006Author:Kenneth M. Leet Emeritus, Chia-Ming Uang, Joel LanningPublisher:McGraw-Hill Education
Traffic and Highway EngineeringCivil EngineeringISBN:9781305156241Author:Garber, Nicholas J.Publisher:Cengage Learning
Structural Analysis (10th Edition)
Civil Engineering
ISBN:9780134610672
Author:Russell C. Hibbeler
Publisher:PEARSON
Principles of Foundation Engineering (MindTap Cou...
Civil Engineering
ISBN:9781337705028
Author:Braja M. Das, Nagaratnam Sivakugan
Publisher:Cengage Learning
Fundamentals of Structural Analysis
Civil Engineering
ISBN:9780073398006
Author:Kenneth M. Leet Emeritus, Chia-Ming Uang, Joel Lanning
Publisher:McGraw-Hill Education
Traffic and Highway Engineering
Civil Engineering
ISBN:9781305156241
Author:Garber, Nicholas J.
Publisher:Cengage Learning
Stress Distribution in Soils GATE 2019 Civil | Boussinesq, Westergaard Theory; Author: Gradeup- GATE, ESE, PSUs Exam Preparation;https://www.youtube.com/watch?v=6e7yIx2VxI0;License: Standard YouTube License, CC-BY