Introduction to Heat Transfer
6th Edition
ISBN: 9780470501962
Author: Frank P. Incropera, David P. DeWitt, Theodore L. Bergman, Adrienne S. Lavine
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 3, Problem 3.68P
Referring to the semiconductor processing tool of Problem 5.13, it is desired at some point in the manufacturing cycle to cool the chuck, which is made of aluminum alloy 2024. The proposed cooling scheme passes air at 20°C between the air-supply head and the chuck surface.
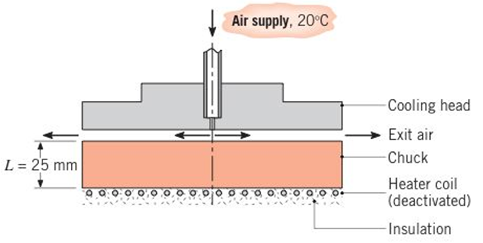
(a) If the chuck is initially at a uniform temperature of l00°C, calculate the time required for its lower surface to reach 25°C, assuming a uniform convection coefficient of 50 W/m2⋅K at the head—chuck interface.
(b) Generate a plot of the time-to-cool as a function of the convection coefficient for the range 10≤h≤2000 W/m2⋅K. If the lower limit represents a free convection condition without any head present, comment on the effectiveness of the head design as a method for cooling the chuck.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
The cantilevered bar in the figure is made from a ductile material and is statically loaded
with F,, = 200 lbf and Fx = F₂ = 0. Analyze the stress situation in rod AB by obtaining
the following information. Note that the stress concentration factors are neglected in the
following questions (Kt and Kts=1).
(a) Determine the precise location of the critical stress element.
(b) Sketch the critical stress element and determine magnitudes and direction for all
stresses acting on it. (Transverse shear may only be neglected if you can justify this
decision.)
(c) For the critical stress element, determine the principal stresses and maximum shear
stress.
6 in
1-in dia.
B
+1-
in
in
2 in
5 in
A laminated thick-walled hydraulic cylinder was fabricated by shrink-fitting
jacket having an outside diameter of 300mm onto a SS 304 steel tube having an inside
diameter of 100mm and an outside diameter of 200mm as shown in the figure. The
interference (8) was 0.15mm. When the Young's modulus for both SS304 and 1020 steel
is the same as 200GPa, and the Poisson's ratio is also the same as 0.3 for both materials,
find the followings.
Initially 100 mm
Initially 200 mm
Initially 300 mm
SS 304
1020 steel
(a) P; (interfacial contact stress)
(b) The maximum stresses (σ, and σ+) in the laminated steel cylinder resulting from the
shrink fit.
Auto Controls
Design a proportional derivitivecontroller for a plant orsystemthat satisfies the following specifications :
1. is steady-state error is less than 2 % for a ramp input.
2.) Damping ratio (zeta) is greater than 0.7have determined the
3. Once youvalue of kp and kd, then plotthe response of the compensated(with controller) and uncompensated( without the controller, only the plantsystem using MATLAB.
Chapter 3 Solutions
Introduction to Heat Transfer
Ch. 3 - Consider the plane wall of Figure 3.1, separating...Ch. 3 - A new building to be located in a cold climate is...Ch. 3 - The rear window of an automobile is defogged by...Ch. 3 - The rear window of an automobile is defogged by...Ch. 3 - A dormitory at a large university, built 50 years...Ch. 3 - In a manufacturing process, a transparent film is...Ch. 3 - Prob. 3.7PCh. 3 - A t=10-mm-thick horizontal layer of water has a...Ch. 3 - Prob. 3.9PCh. 3 - The wind chill, which is experienced on a cold,...
Ch. 3 - Prob. 3.11PCh. 3 - A thermopane window consists of two pieces of...Ch. 3 - A house has a composite wall of wood, fiberglass...Ch. 3 - Prob. 3.14PCh. 3 - Prob. 3.15PCh. 3 - Work Problem 3.15 assuming surfaces parallel to...Ch. 3 - Consider the oven of Problem 1.54. The walls of...Ch. 3 - The composite wall of an oven consists of three...Ch. 3 - The wall of a drying oven is constructed by...Ch. 3 - The t=4-mm-thick glass windows of an...Ch. 3 - Prob. 3.21PCh. 3 - In the design of buildings, energy conservation...Ch. 3 - Prob. 3.23PCh. 3 - Prob. 3.24PCh. 3 - Prob. 3.25PCh. 3 - A composite wall separates combustion gases at...Ch. 3 - Prob. 3.27PCh. 3 - Prob. 3.28PCh. 3 - Prob. 3.29PCh. 3 - The performance of gas turbine engines may...Ch. 3 - A commercial grade cubical freezer, 3 m on a...Ch. 3 - Prob. 3.32PCh. 3 - Prob. 3.33PCh. 3 - Prob. 3.34PCh. 3 - A batt of glass fiber insulation is of density...Ch. 3 - Air usually constitutes up to half of the volume...Ch. 3 - Prob. 3.37PCh. 3 - Prob. 3.38PCh. 3 - The diagram shows a conical section fabricatedfrom...Ch. 3 - Prob. 3.40PCh. 3 - From Figure 2.5 it is evident that, over a wide...Ch. 3 - Consider a tube wall of inner and outer radii ri...Ch. 3 - Prob. 3.43PCh. 3 - Prob. 3.44PCh. 3 - Prob. 3.45PCh. 3 - Prob. 3.46PCh. 3 - To maximize production and minimize pumping...Ch. 3 - A thin electrical heater is wrapped around the...Ch. 3 - Prob. 3.50PCh. 3 - Prob. 3.51PCh. 3 - Prob. 3.52PCh. 3 - A wire of diameter D=2mm and uniform temperatureT...Ch. 3 - Prob. 3.54PCh. 3 - Electric current flows through a long rod...Ch. 3 - Prob. 3.56PCh. 3 - A long, highly polished aluminum rod of diameter...Ch. 3 - Prob. 3.58PCh. 3 - Prob. 3.59PCh. 3 - Prob. 3.60PCh. 3 - Prob. 3.61PCh. 3 - Prob. 3.62PCh. 3 - Consider the series solution, Equation 5.42, for...Ch. 3 - Prob. 3.64PCh. 3 - Copper-coated, epoxy-filled fiberglass circuit...Ch. 3 - Prob. 3.66PCh. 3 - A constant-property, one-dimensional Plane slab of...Ch. 3 - Referring to the semiconductor processing tool of...Ch. 3 - Prob. 3.69PCh. 3 - Prob. 3.70PCh. 3 - Prob. 3.71PCh. 3 - The 150-mm-thick wall of a gas-fired furnace is...Ch. 3 - Steel is sequentially heated and cooled (annealed)...Ch. 3 - Prob. 3.74PCh. 3 - Prob. 3.75PCh. 3 - Prob. 3.76PCh. 3 - Prob. 3.77PCh. 3 - Prob. 3.78PCh. 3 - The strength and stability of tires may be...Ch. 3 - Prob. 3.80PCh. 3 - Prob. 3.81PCh. 3 - A long rod of 60-mm diameter and thermophysical...Ch. 3 - A long cylinder of 30-min diameter, initially at a...Ch. 3 - Work Problem 5.47 for a cylinder of radius r0 and...Ch. 3 - Prob. 3.85PCh. 3 - Prob. 3.86PCh. 3 - Prob. 3.87PCh. 3 - Prob. 3.88PCh. 3 - Prob. 3.89PCh. 3 - Prob. 3.90PCh. 3 - Prob. 3.91PCh. 3 - Prob. 3.92PCh. 3 - In Section 5.2 we noted that the value of the Biot...Ch. 3 - Prob. 3.94PCh. 3 - Prob. 3.95PCh. 3 - Prob. 3.96PCh. 3 - Prob. 3.97PCh. 3 - Prob. 3.98PCh. 3 - Work Problem 5.47 for the case of a sphere of...Ch. 3 - Prob. 3.100PCh. 3 - Prob. 3.101PCh. 3 - Prob. 3.102PCh. 3 - Prob. 3.103PCh. 3 - Consider the plane wall of thickness 2L, the...Ch. 3 - Problem 4.9 addressed radioactive wastes stored...Ch. 3 - Prob. 3.106PCh. 3 - Prob. 3.107PCh. 3 - Prob. 3.108PCh. 3 - Prob. 3.109PCh. 3 - Prob. 3.110PCh. 3 - A one-dimensional slab of thickness 2L is...Ch. 3 - Prob. 3.112PCh. 3 - Prob. 3.113PCh. 3 - Prob. 3.114PCh. 3 - Prob. 3.115PCh. 3 - Derive the transient, two-dimensional...Ch. 3 - Prob. 3.117PCh. 3 - Prob. 3.118PCh. 3 - Prob. 3.119PCh. 3 - Prob. 3.120PCh. 3 - Prob. 3.121PCh. 3 - Prob. 3.122PCh. 3 - Consider two plates, A and B, that are each...Ch. 3 - Consider the fuel element of Example 5.11, which...Ch. 3 - Prob. 3.125PCh. 3 - Prob. 3.126PCh. 3 - Prob. 3.127PCh. 3 - Prob. 3.128PCh. 3 - Prob. 3.129PCh. 3 - Consider the thick slab of copper in Example 5.12,...Ch. 3 - In Section 5.5, the one-term approximation to the...Ch. 3 - Thermal energy storage systems commonly involve a...Ch. 3 - Prob. 3.133PCh. 3 - Prob. 3.134PCh. 3 - Prob. 3.135PCh. 3 - A tantalum rod of diameter 3 mm and length 120 mm...Ch. 3 - A support rod k=15W/mK,=4.0106m2/s of diameter...Ch. 3 - Prob. 3.138PCh. 3 - Prob. 3.139PCh. 3 - A thin circular disk is subjected to induction...Ch. 3 - An electrical cable, experiencing uniform...Ch. 3 - Prob. 3.142PCh. 3 - Prob. 3.145PCh. 3 - Consider the fuel element of Example 5.11, which...Ch. 3 - Prob. 3.147PCh. 3 - Prob. 3.148PCh. 3 - Prob. 3.149PCh. 3 - Prob. 3.150PCh. 3 - In a manufacturing process, stainless steel...Ch. 3 - Prob. 3.153PCh. 3 - Carbon steel (AISI 1010) shafts of 0.1-m diameter...Ch. 3 - A thermal energy storage unit consists of a large...Ch. 3 - Small spherical particles of diameter D=50m...Ch. 3 - A spherical vessel used as a reactor for producing...Ch. 3 - Batch processes are often used in chemical and...Ch. 3 - Consider a thin electrical heater attached to a...Ch. 3 - An electronic device, such as a power transistor...Ch. 3 - Prob. 3.161PCh. 3 - In a material processing experiment conducted...Ch. 3 - Prob. 3.165PCh. 3 - Prob. 3.166PCh. 3 - Prob. 3.167PCh. 3 - Prob. 3.168PCh. 3 - Prob. 3.173PCh. 3 - Prob. 3.174PCh. 3 - Prob. 3.175PCh. 3 - Prob. 3.176PCh. 3 - Prob. 3.177P
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- Auto Controls (a) Refer to the above figure .What kind of controller is it ? (b) simplify the block diagramto derive the closed loop transfer function of the system. (C) What are the assumptions thatare needed to make to findthe controller gain ? What arethe value of Kp , Ti and Td ?arrow_forwardAuto Controls Design a PID controller for thefollowing system so that the modified system satisfies the followingspecifications : 1. settling time ,ts = 1.96 s and % Overshoot Mp = 70.7 % Assume a non-dominant pole at s = -15 to solve the problem The plot the compensated andThen plot the uncompensated system in MATLAB. what can you see from the plot ? what is your observation ?arrow_forwardFourth year Monthly exam\3 2024-2025 Power plant Time: 1 Hr Q1. A gas turbine power plant operates on a modified Brayton cycle consisting of two-stage compression with intercooling to the initial temperature between stages, two-stage expansion with reheating to the maximum cycle temperature, and two regenerative heat exchangers. The following data is given: Inlet air temperature: 300 K Maximum cycle temperature: 1400 K Pressure ratio across each compressor stage: 4 Pressure ratio across each turbine stage: 4 Isentropic efficiency of compressors and turbines: 85% Effectiveness of each regenerator: 80% a) Draw a schematic and T-s diagram of the cycle. b) Determine the thermal efficiency of the cycle. c) Calculate the net specific work output (in kJ/kg). d) Discuss the impact of regenerators on the cycle performance. Examiner Prof. Dr. Adil Al-Kumaitarrow_forward
- Auto Controls The figure is a schematic diagram of an aircraft elevator control system. The input to the systemin the deflection angle of the control lever , and the output is the elevator angle phi.show that for each angle theta of the control lever ,there is a corresponding elevator angle phi. Then find Y(s)/theta(s) and simplify the resulting transfer function . Also note from the diagram that y and phi is relatedarrow_forwardLiquid hexane flows through a counter flow heat exchanger at 5 m3/h as shown in Figure E5.5.The hexane enters the heat exchanger at 90°C. Water, flowing at 5 m3/h, is used to cool the hexane.The water enters the heat exchanger at 15°C. The UA product of the heat exchanger is found to be2.7 kW/K. Determine the outlet temperatures of the hot and cold fluids and the heat transfer ratebetween them using LMTD method.arrow_forwardDetermine the fluid outlet temperatures and the heat transfer rate for the counter flow heatexchanger described in Problem 3 using the ε-NTU model. Assume that the properties can beevaluated at the given fluid inlet temperatures.arrow_forward
- Section View - practice Homework 0.5000 3.0000 2,0000 1.0000arrow_forwardDrawing the section view for the following multiview drawing AutoCAD you see the section pratice I need to show how to autocadarrow_forwardA boiler with 80% efficiency produces steam at 40bar and 500 C at a rate of 1.128kg/s. The temperature of the feed water is raised from 25 C to 125 C in the economizer and the ambient air is drawn to the boiler at a rate of 2.70 kg/s at 16 C. The flue gases leave the chimney at rate of 3 kg/s at 150 C with specific heat of 1.01 kJ/kg.K. The dryness fraction of steam collected in the steam drum is 0.95. 1- Determine the heat value of the fuel. 2- The equivalence evaporation. 3- Draw the heat balance sheet.arrow_forward
- A rotating shaft is made of 42 mm by 4 mm thick cold-drawn round steel tubing and has a 6 mm diameter hole drilled transversely through it. The shaft is subjected to a pulsating torque fluctuating from 20 to 160 Nm and a completely reversed bending moment of 200 Nm. The steel tubing has a minimum strength of Sut = 410 MPa (60 ksi). The static stress-concentration factor for the hole is 2.4 for bending and 1.9 for torsion. The maximum operating temperature is 400˚C and a reliability of 99.9% is to be assumed. Find the factor of safety for infinite life using the modified Goodman failure criterion.arrow_forwardI need help with a MATLAB code. This code just keeps running and does not give me any plots. I even reduced the tolerance from 1e-9 to 1e-6. Can you help me fix this? Please make sure your solution runs. % Initial Conditions rev = 0:0.001:2; g1 = deg2rad(1); g2 = deg2rad(3); g3 = deg2rad(6); g4 = deg2rad(30); g0 = deg2rad(0); Z0 = 0; w0 = [0; Z0*cos(g0); -Z0*sin(g0)]; Z1 = 5; w1 = [0; Z1*cos(g1); -Z1*sin(g1)]; Z2 = 11; w2 = [0; Z2*cos(g2); -Z2*sin(g2)]; [v3, psi3, eta3] = Nut_angle(Z2, g2, w2); plot(v3, psi3) function dwedt = K_DDE(~, w_en) % Extracting the initial condtions to a variable % Extracting the initial condtions to a variable w = w_en(1:3); e = w_en(4:7); Z = w_en(8); I = 0.060214; J = 0.015707; x = (J/I) - 1; y = Z - 1; s = Z; % Kinematic Differential Equations dedt = zeros(4,1); dedt(1) = pi*(e(3)*(s-w(2)-1) + e(2)*w(3) + e(4)*w(1)); dedt(2) = pi*(e(4)*(w(2)-1-s) + e(3)*w(1) - e(1)*w(3)); dedt(3) = pi*(-e(1)*(s-w(2)-1) - e(2)*w(1) + e(4)*w(3));…arrow_forwardalpha 1 is not zero alpha 1 can equal alpha 2 use velocity triangle to solve for alpha 1 USE MATLAB ONLY provide typed code solve for velocity triangle and dont provide copied answer Turbomachienery . GIven: vx = 185 m/s, flow angle = 60 degrees, (leaving a stator in axial flow) R = 0.5, U = 150 m/s, b2 = -a3, a2 = -b3 Find: velocity triangle , a. magnitude of abs vel leaving rotor (m/s) b. flow absolute angles (a1, a2, a3) 3. flow rel angles (b2, b3) d. specific work done e. use code to draw vel. diagram Use this code for plot % plots Velocity Tri. in Ch4 function plotveltri(al1,al2,al3,b2,b3) S1L = [0 1]; V1x = [0 0]; V1s = [0 1*tand(al3)]; S2L = [2 3]; V2x = [0 0]; V2s = [0 1*tand(al2)]; W2s = [0 1*tand(b2)]; U2x = [3 3]; U2y = [1*tand(b2) 1*tand(al2)]; S3L = [4 5]; V3x = [0 0]; V3r = [0 1*tand(al3)]; W3r = [0 1*tand(b3)]; U3x = [5 5]; U3y = [1*tand(b3) 1*tand(al3)]; plot(S1L,V1x,'k',S1L,V1s,'r',... S2L,V2x,'k',S2L,V2s,'r',S2L,W2s,'b',U2x,U2y,'g',...…arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Principles of Heat Transfer (Activate Learning wi...Mechanical EngineeringISBN:9781305387102Author:Kreith, Frank; Manglik, Raj M.Publisher:Cengage Learning
Principles of Heat Transfer (Activate Learning wi...
Mechanical Engineering
ISBN:9781305387102
Author:Kreith, Frank; Manglik, Raj M.
Publisher:Cengage Learning
Heat Transfer – Conduction, Convection and Radiation; Author: NG Science;https://www.youtube.com/watch?v=Me60Ti0E_rY;License: Standard youtube license