Chapter 9, Problem 9.62P

Consider the differential amplifier shown in Figure 9.24(a). Assume thateach resistor is $50\left(1\pm x\right)k\Omega$ . (a) Determine the worst case common-modegain $A_{CM}=v_O/v_{CM}$ , where $v_{CM}=v_1=v_2$ . (b) Evaluate $A_{CM}$ andCMRR(dB) for $x=0.01,0.02,$ and 0.05.

To determine

The value of the worst case common mode gain.

Answer to Problem 9.62P

The value of the worst case common mode gain is $\frac{2x}{1+x}$ .

Explanation of Solution

Calculation:

The given diagram is shown in Figure 1

  

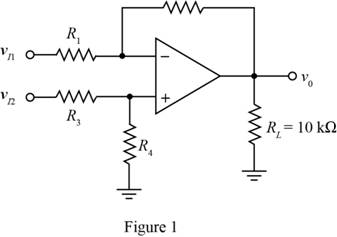
Mark the voltages and redraw the circuit.

The required diagram is shown in Figure 2

  

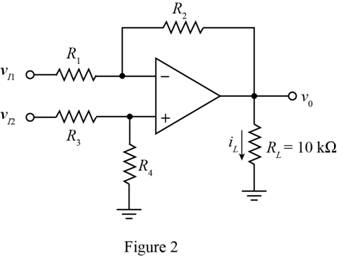
The expression for the common mode voltage gain is given by,

  $A_{CM}=\frac{v_O}{v_{CM}}$

The expression for the value of the voltage $v_1$ is given by,

  $v_1=v_2$

The expression for the voltage $v_2$ by voltage division rule is given by,

  $v_2=\left(\frac{R_4}{R_3+R_4}\right)v_{I2}$

Apply KCL at the inverting terminal.

  $\begin{array}{l}\frac{v_{I1}-v_1}{R_1}=\frac{v_1-v_O}{R_2}\\ \frac{v_{I1}{R}_2-v_1{R}_1-v_1{R}_1}{R_1{R}_2}=-\frac{v_O}{R_2}\\ v_O=-v_{I1}\left(\frac{R_2}{R_1}\right)+v_1\left(1+\frac{R_2}{R_1}\right)\end{array}$

Substitute $v_2$ for $v_1$ in the above equation.

  $v_O=-v_{I1}\left(\frac{R_2}{R_1}\right)+v_2\left(1+\frac{R_2}{R_1}\right)$

Substitute $\left(\frac{R_4}{R_3+R_4}\right)v_{I2}$ for $v_2$ in the above equation.

  $v_O=-v_{I1}\left(\frac{R_2}{R_1}\right)+\left(\left(\frac{R_4}{R_3+R_4}\right)v_{I2}\right)\left(1+\frac{R_2}{R_1}\right)$ …… (1)

The expression for the common node input voltage is given by,

  $v_{CM}=v_{I1}$

The expression for the common node input voltage is given by,

  $v_{CM}=v_{I2}$

Substitute $v_{CM}$ for $v_{I1}$ and $v_{CM}$ for $v_{I2}$ in the above equation.

  $\begin{array}{l}{v}_O=-v_{CM}\left(\frac{R_2}{R_1}\right)+\left(\left(\frac{R_4}{R_3+R_4}\right)v_{CM}\right)\left(1+\frac{R_2}{R_1}\right)\\ \frac{v_O}{v_{CM}}=\left[\left(\frac{R_4}{R_3+R_4}\right)\left(\frac{R_1+R_2}{R_1}\right)-\frac{R_2}{R_1}\right]\end{array}$

Substitute $A_{CM}$ for $\frac{v_O}{v_{CM}}$ in the above equation.

  $\begin{array}{c}{A}_{CM}=\left[\left(\frac{R_4}{R_3+R_4}\right)\left(\frac{R_1+R_2}{R_1}\right)-\frac{R_2}{R_1}\right]\\ =\frac{R_4}{R_3+R_4}\left(1-\frac{R_2{R}_3}{R_1{R}_4}\right)\end{array}$ …… (2)

In the above equation the worst case $A_{CM}$ is obtained when $\frac{R_4}{R_3+R_4}$ is maximum and $1-\frac{R_2{R}_3}{R_1{R}_4}$ is minimum. The second case arises when $R_2$ and $\frac{R_3}{R_4}$ is minimum and $R_1$ is maximum. The third case arises when the value of $R_1,R_4$ are minimum and $R_2$ , $R_3$ is minimum.

For worst condition the value of the resistance $R_1$ is given by,

  $R_1=50\left(1+x\right)$

For worst condition the value of the resistance $R_2$ is given by,

  $R_2=50\left(1-x\right)$

For worst condition the value of the resistance $R_3$ is given by,

  $R_3=50\left(1-x\right)$

For worst condition the value of the resistance $R_4$ is given by,

  $R_4=50\left(1+x\right)$

Substitute $50\left(1+x\right)$ for $R_1$ , $50\left(1-x\right)$ for $R_2$ , $50\left(1-x\right)$ for $R_3$ and $50\left(1+x\right)$ for $R_4$ in the above equation.

  $\begin{array}{c}{A}_{CM}=\frac{50\left(1+x\right)}{50\left(1-x\right)+50\left(1+x\right)}\left(1-\frac{\left(50\left(1-x\right)\right)\left(50\left(1-x\right)\right)}{\left(50\left(1+x\right)\right)\left(50\left(1+x\right)\right)}\right)\\ =\frac{2x}{1+x}\end{array}$ …… (3)

Conclusion:

Therefore, the value of the worst case common mode gain is $\frac{2x}{1+x}$ .

To determine

The value of the CMRR and the common mode gain.

Answer to Problem 9.62P

The value of $A_{CM}$ for $x$ equals to $0.01$ is $0.0198$ , for $x$ equal to $0.02$ is $0.0392$ and for $x$ equals to $0.05$ is $0.0952$ . The value of the CMRR for for $x$ equals to $0.01$ is $33.979\text{dB}$ , for $x$ equal to $0.02$ is $27.959\text{dB}$ and for $x$ equals to $0.05$ is $20\text{dB}$ .

Explanation of Solution

Calculation:

The expression for the common mode gain is given by,

  $\begin{array}{l}{v}_{CM}=\frac{v_{I2}+v_{I1}}{2}\\ v_{I1}=2v_{CM}-v_{I2}\end{array}$

The expression for the differential voltage is given by,

  $v_d=v_{I2}-v_{I1}$

Substitute $2v_{CM}-v_{I2}$ for $v_{I1}$ in the above equation.

  $\begin{array}{l}{v}_d=v_{I2}-2v_{CM}+v_{I2}\\ v_d=2v_{I2}-2v_{CM}\\ v_{I2}=\frac{v_d}{2}+v_{CM}\end{array}$

The expression for the voltage $v_{I1}$ is given by,

  $v_{I1}=v_{CM}-\frac{v_d}{2}$

The expression for the common mode voltage is given by,

  $v_{CM}=\frac{v_{I2}+v_{I1}}{2}$

Substitute $v_{CM}-\frac{v_d}{2}$ for $v_{I1}$ and $\frac{v_d}{2}+v_{CM}$ for $v_{I2}$ in the above equation.

  $\begin{array}{c}{v}_{CM}=\frac{\frac{v_d}{2}+v_{CM}-v_{CM}-\frac{v_d}{2}}{2}\\ =0\text{V}\end{array}$

Substitute $v_{CM}-\frac{v_d}{2}$ for $v_{I1}$ and $\frac{v_d}{2}+v_{CM}$ for $v_{I2}$ in equation (1).

  $v_O=-\left(v_{CM}-\frac{v_d}{2}\right)\left(\frac{R_2}{R_1}\right)+\left[\left(\frac{R_4}{R_3+R_4}\right)\left(\frac{R_1+R_2}{R_1}\right)\right]\left(\frac{v_d}{2}+v_{CM}\right)$

Substitute $0$ for $v_{CM}$ in the above equation.

  $\begin{array}{c}{v}_O=\left(\frac{v_d}{2}\right)\left(\frac{R_2}{R_1}\right)+\left[\left(\frac{R_4}{R_3+R_4}\right)\left(\frac{R_1+R_2}{R_1}\right)\right]\left(\frac{v_d}{2}\right)\\ =\left[\left(\frac{R_4}{R_3+R_4}\right)\left(\frac{R_1+R_2}{R_1}\right)+\left(\frac{R_2}{R_1}\right)\right]\frac{v_d}{2}\end{array}$

Substitute $50\left(1+x\right)$ for $R_1$ , $50\left(1-x\right)$ for $R_2$ , $50\left(1-x\right)$ for $R_3$ and $50\left(1+x\right)$ for $R_4$ in the above equation.

  $\begin{array}{l}{v}_O=\left[\left(\frac{50\left(1+x\right)}{50\left(1-x\right)+50\left(1+x\right)}\right)\left(\frac{50\left(1-x\right)+\left(50\left(1+x\right)\right)}{50\left(1-x\right)}\right)+\left(\frac{50\left(1-x\right)}{50\left(1+x\right)}\right)\right]\frac{v_d}{2}\\ v_O=\left[\frac{2}{1+x}\right]\frac{v_d}{2}\\ \frac{v_O}{v_d}=\left[\frac{2}{1+x}\right]\end{array}$

Substitute $A_d$ for $\frac{v_O}{v_d}$ in the above equation.

  $A_d=\left[\frac{1}{1+x}\right]$

The expression for the $\text{CMRR}$ is given by,

  $\text{CMRR}\left(\text{dB}\right)=20{\log }_{10}\left|\frac{A_d}{A_{CM}}\right|$

Substitute for $\frac{1}{1+x}$ for $A_d$ and $\frac{2x}{1+x}$ for $A_{CM}$ in the above equation.

  $\begin{array}{c}\text{CMRR}\left(\text{dB}\right)=20{\log }_{10}\left|\frac{\frac{1}{1+x}}{\frac{2x}{1+x}}\right|\\ =20{\log }_{10}\left|\frac{1}{2x}\right|\end{array}$ …… (4)

Substitute $0.01$ for $x$ in equation (3).

  $\begin{array}{c}{A}_{CM}=\frac{2\left(0.01\right)}{1+0.01}\\ =0.0198\end{array}$

Substitute $0.01$ for $x$ in equation (4).

  $\begin{array}{c}\text{CMRR}\left(\text{dB}\right)=20{\log }_{10}\left|\frac{1}{2\left(0.01\right)}\right|\\ =33.979\text{dB}\end{array}$

Substitute $0.02$ for $x$ in equation (3).

  $\begin{array}{c}{A}_{CM}=\frac{2\left(0.02\right)}{1+0.02}\\ =0.0392\end{array}$

Substitute $0.02$ for $x$ in equation (4).

  $\begin{array}{c}\text{CMRR}\left(\text{dB}\right)=20{\log }_{10}\left|\frac{1}{2\left(0.02\right)}\right|\\ =27.959\text{dB}\end{array}$

Substitute $0.05$ for $x$ in equation (3).

  $\begin{array}{c}{A}_{CM}=\frac{2\left(0.05\right)}{1+0.05}\\ =0.0952\end{array}$

Substitute $0.05$ for $x$ in equation (4).

  $\begin{array}{c}\text{CMRR}\left(\text{dB}\right)=20{\log }_{10}\left|\frac{1}{2\left(0.05\right)}\right|\\ =20\text{dB}\end{array}$

Conclusion:

Therefore, the value of $A_{CM}$ for $x$ equals to $0.01$ is $0.0198$ , for $x$ equal to $0.02$ is $0.0392$ and for $x$ equals to $0.05$ is $0.0952$ . The value of the CMRR for for $x$ equals to $0.01$ is $33.979\text{dB}$ , for $x$ equal to $0.02$ is $27.959\text{dB}$ and for $x$ equals to $0.05$ is $20\text{dB}$ .