
A battery with ε = 6.00 V and no internal resistance supplies current to the circuit shown in Figure P27.9. When the double-throw switch S is open as shown in the figure, the current in the battery is 1.00 mA. When the switch is closed in position a, the current in the battery is 1.20 mA. When the switch is closed in position b, the current in the battery is 2.00 mA. Find the resistances (a) R1, (b) R2, and (c) R3.
Figure P27.9 Problems 9 and 10.
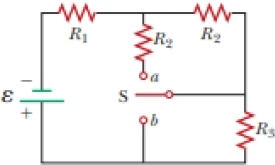
(a)
Answer to Problem 28.11P
Explanation of Solution
Given information: Emf across the battery is
Explanation:
When the switch S is open, then the three resistors
Formula to calculate the equivalent resistance across the circuit, when the switch S is open.
Here,
As the total emf across the battery is equal to the voltage across the battery.
Here,
Substitute
Formula to calculate the equivalent resistance across the circuit, when the switch S is open.
Here,
Substitute
Substitute
When the switch is closed in position
From equation (2), formula to calculate the equivalent resistance across the circuit, when the switch is closed in position
Here,
Formula to calculate the resistance when the resistors are connected in parallel.
From equation (3), formula to calculate the equivalent resistance across the circuit, when the switch is closed in position
Substitute
Substitute
Substitute
When the switch is closed in position
From equation (2), formula to calculate the equivalent resistance across the circuit, when the switch is closed in position
Here,
From equation (3), formula to calculate the equivalent resistance across the circuit, when the switch is closed in position
Substitute
Substitute
Subtract equation (14) from (5) to find
Thus, the value of the resistance
Subtract equation (14) from (10) to find
Substitute
Thus, the value of the resistance
Substitute
Thus, the value of the resistance
Conclusion:
Therefore, the value of the resistance
(b)
Answer to Problem 28.11P
Explanation of Solution
Given information: Emf across the battery is
Explanation:
From part (a) equation (17), the value of resistance
Thus, the value of the resistance
Conclusion:
Therefore, the value of the resistance
(c)
Answer to Problem 28.11P
Explanation of Solution
Given information: Emf across the battery is
Explanation:
From part (a) equation (15), the value of resistance
Thus, the value of the resistance
Conclusion:
Therefore, the value of the resistance
Want to see more full solutions like this?
Chapter 28 Solutions
Physics for Scientists and Engineers, Technology Update (No access codes included)
- In the circuit of Figure P27.25, the switch S has been open for a long time. It is then suddenly closed. Determine the time constant (a) before the switch is closed and (b) after the switch is closed. (c) Let the switch be closed at t = 0. Determine the current in the switch as a function of time. Figure P27.25 Problems 25 and 26.arrow_forwardIn the circuit of Figure P21.57, the switch S has been open for a long time. It is then suddenly closed. Take = 10.0 V, R1 = 50.0 k, R2 = 100 k, and C = 10.0 F. Determine the time constant (a) before the switch is closed and (b) after the switch is closed. (c) Let the switch be closed at t = 0. Determine the current in the switch as a function of time.arrow_forwardThe emfs in Figure P29.43 are 1 = 6.00 V and 2 = 12.0 V. The resistances are R1 = 15.0 , R2 = 30.0 , R3 = 45.0 , and R4 = 60.0 . Find the current in each resistor when the switch is a. open and b. closed.arrow_forward
- Consider a series RC circuit as in Figure P28.38 for which R = 1.00 M, C = 5.00 F, and = 30.0 V. Find (a) the time constant of the circuit and (b) the maximum charge on the capacitor after the switch is thrown closed. (c) Find the current in the resistor 10.0 s after the switch is closed.arrow_forwardThe circuit shown in Figure P28.78 is set up in the laboratory to measure an unknown capacitance C in series with a resistance R = 10.0 M powered by a battery whose emf is 6.19 V. The data given in the table are the measured voltages across the capacitor as a function of lime, where t = 0 represents the instant at which the switch is thrown to position b. (a) Construct a graph of In (/v) versus I and perform a linear least-squares fit to the data, (b) From the slope of your graph, obtain a value for the time constant of the circuit and a value for the capacitance. v(V) t(s) In (/v) 6.19 0 5.56 4.87 4.93 11.1 4.34 19.4 3.72 30.8 3.09 46.6 2.47 67.3 1.83 102.2arrow_forwardAt one instant, a current of 6.0 A flows through part of a circuit as shown in Figure P33.12. Determine the instantaneous potential difference between points A and B if the current starts to decrease at a constant rate of 1.0 102 A/s. FIGURE P33.12arrow_forward
- For the circuit shown in Figure P28.55. the ideal voltmeter reads 6.00 V and the ideal ammeter reads 3.00-k. Find (a) the value of K, (b) the emf of the battery, and (c) the voltage across the 3.00-kft resistor.arrow_forwardFigure P29.84 shows a circuit that consists of two identical emf devices. If R1 = R2 = R and the switch is closed, find an expression (in terms of R and ) for the current I that is in the branch from point a to b.arrow_forwardSwitch S shown in Figure P28.71 has been closed for a long lime, and the electric circuit carries a constant current. Take C1 = 3.00 μF, C2 = 6.00 μF, R1 = 4.00 kΩ, and R2 , = 7.00 kΩ. The power delivered to R2 , is 2.40 W. (a) Find the charge on C1 . (b) Now the switch is opened. After many milliseconds, by how much has the charge on C2 changed?arrow_forward
- A battery with emf ? and no internal resistance supplies current to the circuit shown in the figure below. When the double-throw switch S is open, the current in the battery is I0. When the switch is closed in position a, the current in the battery is Ia. When the switch is closed in position b, the current in the battery is Ib. Find the resistances (a) R1, (b) R2, and (c) R3.arrow_forwardThe switch in the circuit below has been in position a for a long time. At time t = 0 the switch is thrown to position b. You are given the data: Vb = 36 V, C = 8 μF. Vc is the voltage across the capacitor. If the charge on the capacitor at time t = 0.3 msec after the switch is thrown is 54.1 μC, what is the value of the resistor R? a) 89.71 Ω b) 44.86 Ω c) 22.43 Ω d) Not enough information.arrow_forwardYou connect a battery, resistor, and capacitor as shown in Figure, where s = 36.0 V, C = 5.00 μF, and R = 120 Q2. The switch $ is closed at t=0. (a) When the voltage across the capacitor is 8.00 V, what is the magnitude of the current in the circuit? (b) At what time t after the switch is closed is the voltage across the capacitor 8.00 V? (c) When the voltage across the capacitor is 8.00 V, at what rate is energy being stored in the capacitor? ation i0 ww Switch open q=0arrow_forward
 Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning




