The demonstration of how rapidly Rayleigh’s method converges for the uniform-diameter solid shaft.

Question

Want to see more full solutions like this?

Answer 1

Question

Chapter 7, Problem 29P

To determine

The demonstration of how rapidly Rayleigh’s method converges for the uniform-diameter solid shaft.

Expert Solution & Answer

Answer to Problem 29P

The Rayleigh method for uniform diameter shaft is converging rapidly by using a static deflection beam equation.

Explanation of Solution

Write the expression for moment of inertia.

I=π64d4 (I)

Here, the diameter of the shaft is d.

Write the expression for area of the shaft.

A=π4d2 (II)

Write the expression for weight of the shaft.

w=Aγl (III)

Here, the specific weight is γ

Write the expression for influence coefficient.

δij=bjxi6EIl(l2−bj2−xi2) (IV)

Here, the length of the shaft is l, the elastic constant is E.

Write the expression for deflection at point 1.

y1=w1δ11 (V)

Write the expression for Rayleigh method.

∑wy=w1y1 (VI)

Write the expression for Rayleigh method.

∑wy2=w1y21 (VII)

Write the expression for first critical speed.

ω1=g∑wy∑wy2 (VIII)

Draw the diagram for the two elements system.

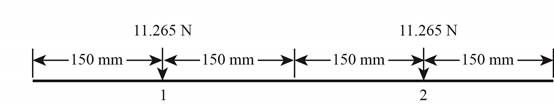
Shigley's Mechanical Engineering Design (McGraw-Hill Series in Mechanical Engineering), Chapter 7, Problem 29P , additional homework tip 1

Figure-(1)

The figure-(1) shows the required dimension.

Write the expression for the deflection at point 1 for two element.

y1=w1δ11+w2δ12 (IX)

Write the expression for the deflection at point 2 for two element.

y2=w1δ11+w2δ12 (X)

Write the expression for Rayleigh method for two element.

∑wy=w1y1+w2y2 (XI)

Write the expression for Rayleigh method for two element.

∑wy2=w1y21+w2y22 (XII)

Write the expression for first critical speed for two element.

ω2=g∑wy∑wy2 (XIII)

Draw the diagram for the three element system.

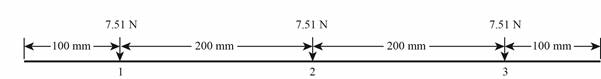
Shigley's Mechanical Engineering Design (McGraw-Hill Series in Mechanical Engineering), Chapter 7, Problem 29P , additional homework tip 2

Figure-(2)

The Figure-(2) shows all the dimensions for the three elements.

Write the expression for the deflection at point 1 for three elements

y1=w1(δ11+δ12+δ13) (XIV)

Write the expression for the deflection at point 2 for three element.

y2=w1(δ12+δ22+δ32) (XV)

Write the expression for the deflection at point 2 for three element.

y3=w1(δ13+δ32+δ33) (XVI)

Write the expression for Rayleigh method for three elements.

∑wy=w1(y1+y2+y3) (XVII)

Write the expression for Rayleigh method for three elements.

∑wy2=w1(y12+y22+y32) (XVIII)

Write the expression for first critical speed for three elements.

ω3=g∑wy∑wy2 (XIX)

Conclusion:

Substitute 25 mm for d in Equation (I).

I=π(25 mm)464=(19174.75985 mm4)(1 m41012 mm4)=1.917×10−8 m4

Substitute 25 mm for d in Equation (II).

A=π4(25 mm)2=(490.8738 mm2)(1 m2106 mm2)=4.909×10−4 m2

Substitute 4.909×10−4 m2 for A, 76.5 kN/m2 for γ and 600 mm for l in Equation (III).

w=(4.909×10−4 m2)(76.5 kN/m2)(600 mm)=(4.909×10−4 m2)(76.5 kN/m2)(1000 N1 kN)(600 mm)(1 m1000 mm)=22.53 N

Substitute 0.3 m for b1, 0.3 m for x1, 207×109 N/m2 for E, 1.917×10−8 m4 for I, 0.6 m for l, 1 for i and 1 for j in Equation (VI).

δ11=(0.3 m)(0.3 m)6(207×109 N/m2)(1.917×10−8 m4)(0.6 m)((0.6 m)2−(0.3 m)2−(0.3 m)2)=(0.09 m2)(0.18 m2)(1242×109 N/m2)(1.1502×10−8 m5)=1.134×10−6 m/N

Substitute 1.134×10−6 m/N for δ11,and 22.53 N for w1 in Equation (VII).

y1=(22.53 N)(1.134×10−6 m/N)=2.555×10−5 m

Calculate the square of the deflection at point 1 of element 1.

y12=(2.555×10−5 m)2y12=6.528×10−10 m2

Substitute 2.555×10−5 m for y1 and 22.53 N for w1 in Equation (VIII).

∑wy=(22.53 N)(2.555×10−5 m)=5.756×10−4 N⋅m

Substitute 6.528×10−10 m2 for y12 and 22.53 N for w1 in Equation (IX).

∑wy2=(22.53 N)(6.528×10−10 m2)=1.471×10−8 N⋅m2

Substitute 5.756×10−4 N⋅m for ∑wy, 9.81 m/s2 for g and 1.471×10−8 N⋅m2 for ∑wy2 in Equation (X).

ω1=9.81 m/s2(5.756×10−4 N⋅m1.471×10−8 N⋅m2)=383863.766 rad/s≈620 rad/s

Substitute 0.45 m for b1, 0.15 m for x1, 207×109 N/m2 for E, 1.917×10−8 m4 for I, 0.6 m for l, 1 for i and 1 for j in Equation (VI).

δ11=(0.45 m)(0.15 m)6(207×109 N/m2)(1.917×10−8 m4)(0.6 m)((0.6 m)2−(0.45 m)2−(0.15 m)2)=(0.0675 m2)(0.135 m2)(1242×109 N/m2)(1.1502×10−8 m5)=6.37×10−7 m/N

Substitute 0.45 m for b2, 0.15 m for x2, 207×109 N/m2 for E, 1.917×10−8 m4 for I, 0.6 m for l, 2 for i and 2 for j in Equation (VI).

δ22=(0.45 m)(0.15 m)6(207×109 N/m2)(1.917×10−8 m4)(0.6 m)((0.6 m)2−(0.45 m)2−(0.15 m)2)=(0.0675 m2)(0.135 m2)(1242×109 N/m2)(1.1502×10−8 m5)=6.37×10−7 m/N

Substitute 0.15 m for b2, 0.15 m for x1, 207×109 N/m2 for E, 1.917×10−8 m4 for I, 0.6 m for l, 1 for i and 2 for j in Equation (VI).

δ12=(0.15 m)(0.15 m)6(207×109 N/m2)(1.917×10−8 m4)(0.6 m)((0.6 m)2−(0.15 m)2−(0.15 m)2)=(0.0225 m2)(0.315 m2)(1242×109 N/m2)(1.1502×10−8 m5)=4.961×10−7 m/N

Substitute 0.15 m for b1, 0.15 m for x2, 207×109 N/m2 for E, 1.917×10−8 m4 for I, 0.6 m for l, 2 for i and 1 for j in Equation (VI).

δ21=(0.15 m)(0.15 m)6(207×109 N/m2)(1.917×10−8 m4)(0.6 m)((0.6 m)2−(0.15 m)2−(0.15 m)2)=(0.0225 m2)(0.315 m2)(1242×109 N/m2)(1.1502×10−8 m5)=4.961×10−7 m/N

Substitute 6.37×10−7 m/N for δ11,11.625 N for w1, 11.625 N for w2 and 4.961×10−7 m/N for δ12 in Equation (XI).

y1=(11.625 N)(6.37×10−7 m/N)+(11.625 N)(4.961×10−7 m/N)=1.035×10−6 m+5.7671×10−6 m=1.277×10−5 m

Substitute 6.37×10−7 m/N for δ11, 11.625 N for w1, 11.625 N for w2 and 5.67×10−7 m/N for δ12 in Equation (XII).

y2=(11.625 N)(6.37×10−7 m/N)+(11.625 N)(4.961×10−7 m/N)=1.035×10−6 m+5.7671×10−6 m=1.277×10−5 m

Calculate the square of the deflection at point 1 of element 2.

y12=(1.277×10−5 m)2=1.632×10−10 m2

Calculate the square of the deflection at point 2 of element 2.

y22=(1.277×10−5 m)2=1.632×10−10 m2

Substitute 1.277×10−5 m for y1, 11.265 N for w1, 1.277×10−5 m for y2 and 11.265 N for w2 in Equation (XIII).

∑wy=(11.265 N)(1.277×10−5 m)+(11.265 N)(1.277×10−5 m)=1.438×10−4 N⋅m+1.438×10−4 N⋅m=2.877×10−4 N⋅m

Substitute 1.632×10−10 m2 for y12, 11.265 N for w2, 1.632×10−10 m2 for y22 and 11.265 N for w1 in Equation (XIV).

∑wy2=(11.265 N)(1.632×10−10 m2)+(11.265 N)(1.632×10−10 m2)=1.838×10−9 N⋅m2+1.838×10−9 N⋅m2=3.677×10−9 N⋅m2

Substitute 2.877×10−4 N⋅m for ∑wy, 9.81 m/s2 for g and 3.677×10−9 N⋅m2 for ∑wy2 in Equation (XV).

ω2=9.81 m/s2(2.877×10−4 N⋅m3.677×10−9 N⋅m2)=767565.1346 rad/s≈876 rad/s

Substitute 0.5 m for b1, 0.1 m for x1, 207×109 N/m2 for E, 1.917×10−8 m4 for I, 0.6 m for l, 1 for i and 1 for j in Equation (VI).

δ11=(0.5 m)(0.1 m)6(207×109 N/m2)(1.917×10−8 m4)(0.6 m)((0.6 m)2−(0.5 m)2−(0.1 m)2)=(0.05 m2)(0.1 m2)(1242×109 N/m2)(1.1502×10−8 m5)=3.500×10−7 m/N

Substitute 0.5 m for b3, 0.1 m for x3, 207×109 N/m2 for E, 1.917×10−8 m4 for I, 0.6 m for l, 3 for i and 3 for j in Equation (VI).

δ33=(0.5 m)(0.1 m)6(207×109 N/m2)(1.917×10−8 m4)(0.6 m)((0.6 m)2−(0.5 m)2−(0.1 m)2)=(0.05 m2)(0.1 m2)(1242×109 N/m2)(1.1502×10−8 m5)=3.500×10−7 m/N

Substitute 0.3 m for b2, 0.3 m for x2, 207×109 N/m2 for E, 1.917×10−8 m4 for I, 0.6 m for l, 2 for i and 2 for j in Equation (VI).

δ22=(0.3 m)(0.3 m)6(207×109 N/m2)(1.917×10−8 m4)(0.6 m)((0.6 m)2−(0.3 m)2−(0.3 m)2)=(0.09 m2)(0.18 m2)(1242×109 N/m2)(1.1502×10−8 m5)=1.13×10−6 m/N

Substitute 0.3 m for b2, 0.1 m for x1, 207×109 N/m2 for E, 1.917×10−8 m4 for I, 0.6 m for l, 1 for i and 2 for j in Equation (VI).

δ12=(0.3 m)(0.1 m)6(207×109 N/m2)(1.917×10−8 m4)(0.6 m)((0.6 m)2−(0.3 m)2−(0.1 m)2)=(0.03 m2)(0.26 m2)(1242×109 N/m2)(1.1502×10−8 m5)=5.46×10−7 m/N

Substitute 0.3 m for b2, 0.1 m for x3, 207×109 N/m2 for E, 1.917×10−8 m4 for I, 0.6 m for l, 3 for i and 2 for j in Equation (VI).

δ32=(0.3 m)(0.1 m)6(207×109 N/m2)(1.917×10−8 m4)(0.6 m)((0.6 m)2−(0.3 m)2−(0.1 m)2)=(0.03 m2)(0.26 m2)(1242×109 N/m2)(1.1502×10−8 m5)=5.46×10−7 m/N

Substitute 0.1 m for b3, 0.1 m for x1, 207×109 N/m2 for E, 1.917×10−8 m4 for I, 0.6 m for l, 1 for i and 3 for j in Equation (VI).

δ13=(0.1 m)(0.1 m)6(207×109 N/m2)(1.917×10−8 m4)(0.6 m)((0.6 m)2−(0.1 m)2−(0.1 m)2)=(0.01 m2)(0.34 m2)(1242×109 N/m2)(1.1502×10−8 m5)=2.38×10−7 m/N

Substitute 3.500×10−7 m/N for δ11, 7.51 N for w1, 2.38×10−7 m/N for δ13 and 5.46×10−7 m/N for δ12 in Equation (XVI).

y1=(7.51 N)[3.500×10−7 m/N+5.46×10−7 m/N+2.38×10−7 m/N]=(7.51 N)(11.34×10−7 m/N)=8.51×10−6 m

Substitute 1.13×10−6 m/N for δ22, 7.51 N for w1, 5.46×10−7 m/N for δ32 and 5.46×10−7 m/N for δ12 in Equation (XVII).

y2=(7.51 N)[5.46×10−7 m/N+1.13×10−6 m/N+5.46×10−7 m/N]=(7.51 N)(2.22×10−6 m/N)=1.672×10−5 m

Substitute 2.38×10−7 m/N for δ13, 7.51 N for w1, 5.46×10−7 m/N for δ32 and 3.500×10−7 m/N for δ33 in Equation (XVIII).

y3=(7.51 N)[2.38×10−7 m/N+5.46×10−7 m/N+3.500×10−7 m/N]=(7.51 N)(11.34×10−7 m/N)=8.51×10−6 m

Calculate the square of the deflection at point 1 of element 3.

y12=(8.51×10−6 m)2=7.25×10−11 m2

Calculate the square of the deflection at point 2 of element 3.

y22=(1.672×10−5 m)2=2.79×10−10 m2

Calculate the square of the deflection at point 3 of element 3.

y32=(8.51×10−6 m)2=7.24×10−11 m2

Substitute 8.51×10−6 m for y1, 7.51 N for w1, 1.672×10−5 m for y2 and 8.51×10−6 m for y3 in Equation (XIX).

∑wy=(7.51 N)(8.51×10−6 m+1.672×10−5 m+8.51×10−6 m)=(7.51 N)(3.374×10−5 m)=2.535×10−4 N⋅m

Substitute 7.25×10−11 m2 for y12, 7.51 N for w1, 2.79×10−10 m2 for y22 and 7.24×10−11 m2 for y32 in Equation (XX).

∑wy2=(7.51 N)(7.25×10−11 m2+2.79×10−10 m2+7.24×10−11 m2)=(7.51 N)(4.238×10−10 m2)=3.189×10−9 N⋅m2

Substitute 2.535×10−4 N⋅m for ∑wy, 9.81 m/s2 for g and 3.189×10−9 N⋅m2 for ∑wy2 in Equation (XXI).

ω3=9.81 m/s2(2.535×10−4 N⋅m3.189×10−9 N⋅m2)=779816.5569 rad/s=883 rad/s

Since the static bending equation is available, and satisfied the moment-free and deflection-free ends, so the convergence is rapid using a static deflection beam equation.

Thus, the Rayleigh method for uniform diameter shaft is converging rapidly by using a static deflection beam equation.

Want to see more full solutions like this?

Subscribe now to access step-by-step solutions to millions of textbook problems written by subject matter experts!

Students have asked these similar questions

The shaft shown in the figure below and rotating at a speed of 1 radian / sec transmits 9 kW of power from the gear at the B point to the pulley at the D point. Manufactured from cold drawn AISI 1040 steel, the shaft is machined to the given dimensions and bedded from A and C points. The highest working temperature of the spindle is 50 ° C. At point D; Since (d2 / d3 = 1.1) and (r /d = 0.1), determine the diameter d3 of the shaft using Goodman's theory. (kb = kc = kf = 1, q = 0.8 and qs = 0.9) 200 mm 140 mm 60 mm F1= 5F Fa Kasnak 160 Ry F2 = F Dig!! Ray Ray

Bearing stress 5. Please provide proper discussion and illustration also complete solution and clear solution please thank you A solid shaft is designed to transmit 50kN @ 1800 rpm to a gear by means of 156 mm long key with square cross-section. The shaft diameter is 35.6mm. What should the cross-section be in mm2 to resist shearing, if the allowable shear stress on the key is 20 MPa?

The chain drive is mounted in the center for the system shown in figure.It can transmit 15 hp at a speed of 400 rpm and the sprocket has a pitch diameter of 6 inches. The shaft is made of ASTM-A 709 Grade 50 steel. A factor of safety of 2.5 is required. Is the design acceptable? Hint: You need to calculate torsion and bending stresses during solution.

Answer 2