Chapter 5, Problem 3P

a.

To determine

Sketch a scatter plot for the given data set.

Answer to Problem 3P

The scatter plot is

  

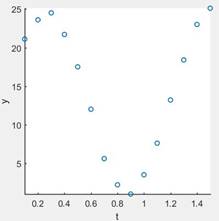
Explanation of Solution

Given: A set of the data is,

  $\begin{array}{cc}t& y\\ 0.1& 21.1\\ 0.2& 23.6\\ 0.3& 24.5\\ 0.4& 21.7\\ 0.5& 17.5\\ 0.6& 12.0\\ 0.7& 5.6\\ 0.8& 2.2\\ 0.9& 1.0\\ 1.0& 3.5\\ 1.1& 7.6\\ 1.2& 13.2\\ 1.3& 18.4\\ 1.4& 23.0\\ 1.5& 25.1\end{array}$

Calculation:

Let’s take a given data set sketch a scatter plot using MATLAB.

The function is using in the MATLAB to sketch a scatter plot is,

  $\text{scatter(t,y)}$

Program:

clc
clear
close all
t=[0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1.0 1.1 1.2 1.3 1.4 1.5];
y=[21.1 23.6 24.5 21.7 17.5 12.0 5.6 2.2 1.0 3.5 7.6 13.2 18.4 23.0 25.1];
scatter(t,y,'linewidth',1.25');
set(gca,'Linewidth',1.2,'Fontsize',12);
xlabel('t');
ylabel('y')
axis square
axis tight

Query:

• First, we have defined the given data sets.
• Then using a function “scatter (t, y)” sketch a scatter plot.

b.

To determine

Calculate the cosine function using given data set.

Answer to Problem 3P

The cosine function is,

  $y=12.05\times \cos (4.1888(t-1.5))+13.05$

Explanation of Solution

Given: A set of the data is,

  $\begin{array}{cc}t& y\\ 0.1& 21.1\\ 0.2& 23.6\\ 0.3& 24.5\\ 0.4& 21.7\\ 0.5& 17.5\\ 0.6& 12.0\\ 0.7& 5.6\\ 0.8& 2.2\\ 0.9& 1.0\\ 1.0& 3.5\\ 1.1& 7.6\\ 1.2& 13.2\\ 1.3& 18.4\\ 1.4& 23.0\\ 1.5& 25.1\end{array}$

Calculation:

First, we have to write a general equation of the cosine function,

  $y=a\cos (w(t-c))+b \mathrm{......}(1)$

Then, calculate the vertical shifting as,

  $b=\frac{1}{2}\left(max value(y)\text{ + }min value(y)\right)=13.05$

Calculate the amplitude as,

  $a=\frac{1}{2}\left(max value(y)\text{ - }min value(y)\right)=12.05$

Then, Calculate the phase shift as,

  $w=\frac{2\pi }{perod}=4.1888$

The value of c is,

  $c=time period at which y value is maximum=1.5$

Put all the value into the equation (1) then,

  $y=12.05\times \cos (4.1888(t-1.5))+13.05$

Program:

clc
clear
close all
t=[0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1.0 1.1 1.2 1.3 1.4 1.5];
y=[21.1 23.6 24.5 21.7 17.5 12.0 5.6 2.2 1.0 3.5 7.6 13.2 18.4 23.0 25.1];
b=(1/2)*(max(y)+min(y));
a=(1/2)*(max(y)-min(y));
w=2*pi/max(t);
idx=find(y==max(y));
c=t(idx);
f=(a*cos(w*(t-c)))+b;

Query:

• First, we have defined the given data sets.
• Then calculate the value of b, a, w, and c.
• Put all the values into the equation of cosine function and get the solution.

c.

To determine

Sketch a graph of the function which is found in part (b).

Answer to Problem 3P

The solution is,

  

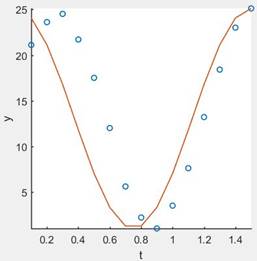
Explanation of Solution

Given: A set of the data is,

  $\begin{array}{cc}t& y\\ 0.1& 21.1\\ 0.2& 23.6\\ 0.3& 24.5\\ 0.4& 21.7\\ 0.5& 17.5\\ 0.6& 12.0\\ 0.7& 5.6\\ 0.8& 2.2\\ 0.9& 1.0\\ 1.0& 3.5\\ 1.1& 7.6\\ 1.2& 13.2\\ 1.3& 18.4\\ 1.4& 23.0\\ 1.5& 25.1\end{array}$

Calculation:

Sketch a graph of the cosine function in MATLAB using function “plot (f, t)”.

The function is found in part (b) is,

  $y=12.05\times \cos (4.1888(t-1.5))+13.05$

Program:

clc
clear
close all
t=[0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1.0 1.1 1.2 1.3 1.4 1.5];
y=[21.1 23.6 24.5 21.7 17.5 12.0 5.6 2.2 1.0 3.5 7.6 13.2 18.4 23.0 25.1];
b=(1/2)*(max(y)+min(y));
a=(1/2)*(max(y)-min(y));
w=2*pi/max(t);
idx=find(y==max(y));
c=t(idx);
f=(a*cos(w*(t-c)))+b;
scatter(t,y,'linewidth',1.25');
hold on
plot(t,f,'linewidth',1.25');
set(gca,'Linewidth',1.2,'Fontsize',12);
xlabel('t');
ylabel('y')
axis square
axis tight

Query:

• First, we have defined the given data sets.
• Then calculate the value of b, a, w, and c.
• Put all the values into the equation of cosine function and get the solution.
• Then sketch a graph.

d.

To determine

Calculate the sine function using given data set.

Answer to Problem 3P

The cosine function is,

  $y=12.05\times \sin (4.1888(t+1.5))+13.05$

Explanation of Solution

Given: A set of the data is,

  $\begin{array}{cc}t& y\\ 0.1& 21.1\\ 0.2& 23.6\\ 0.3& 24.5\\ 0.4& 21.7\\ 0.5& 17.5\\ 0.6& 12.0\\ 0.7& 5.6\\ 0.8& 2.2\\ 0.9& 1.0\\ 1.0& 3.5\\ 1.1& 7.6\\ 1.2& 13.2\\ 1.3& 18.4\\ 1.4& 23.0\\ 1.5& 25.1\end{array}$

Calculation:

First, we have to write a general equation of the cosine function,

  $y=a\sin (w(t+c))+b \mathrm{......}(1)$

Then, calculate the vertical shifting as,

  $b=\frac{1}{2}\left(max value(y)\text{ + }min value(y)\right)=13.05$

Calculate the amplitude as,

  $a=\frac{1}{2}\left(max value(y)\text{ - }min value(y)\right)=12.05$

Then, Calculate the phase shift as,

  $w=\frac{2\pi }{perod}=4.1888$

The value of c is,

  $c=time period at which y value is maximum=1.5$

Put all the value into the equation (1) then,

  $y=12.05\times \sin (4.1888(t+1.5))+13.05$

Program:

clc
clear
close all
t=[0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1.0 1.1 1.2 1.3 1.4 1.5];
y=[21.1 23.6 24.5 21.7 17.5 12.0 5.6 2.2 1.0 3.5 7.6 13.2 18.4 23.0 25.1];
b=(1/2)*(max(y)+min(y));
a=(1/2)*(max(y)-min(y));
w=2*pi/max(t);
idx=find(y==max(y));
c=t(idx);
f=(a*sin(w*(t+c)))+b;

Query:

• First, we have defined the given data sets.
• Then calculate the value of b, a, w, and c.
• Put all the values into the equation of cosine function and get the solution.

e.

To determine

Compare the results from part (b) and (c).

Answer to Problem 3P

Using reduction relation, the graph of the given data is,

  

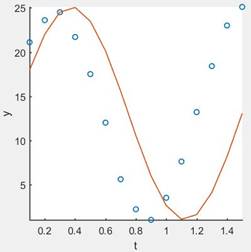
Explanation of Solution

Given: the reduction formula,

  $\sin u=\cos \left(u-\frac{\pi }{2}\right)$

Calculation:

As we can see in part (d) the sine function is,

  $y=12.05\times \sin (4.1888(t+1.5))+13.05$

Where,

  $u=4.1888(t+1.5)$

Then rewrite the function as,

  $y=\cos \left(4.1888(t+1.5)-\frac{\pi }{2}\right)+147.5$

Simplify the above function and sketch a graph.

Program:

clc
clear
close all
t=[0 25 50 75 100 125 150 175 200 225 250 275 300 325 350];
y=[190 175 155 125 110 95 105 120 140 165 185 200 195 185 165];
b=(1/2)*(max(y)+min(y));
a=(1/2)*(max(y)-min(y));
w=2*pi/max(t);
idx=find(y==max(y));
c=t(idx);
f=(a*cos(w*(t+c)-pi/2))+b;
scatter(t,y,'linewidth',1.25');
hold on
plot(t,f,'linewidth',1.25');
set(gca,'linewidth',1.2,'fontsize',12);
xlabel('t');
ylabel('y')
axis square
axis tight

Query:

• First, we have defined the given data sets.
• Then calculate the value of b, a, w, and c.
• Then using reduction relation redefine the function.
• Sketch a graph.