Chapter 4.3, Problem 23PPS

To determine

To prove : The two angles in a triangle $\Delta ABC$ are congruent that is, $\angle A\cong \angle C$ , where $\overline{BD}$ bisects $\angle B$ and $\overline{BD}\perp \overline{AC}$ .

Explanation of Solution

Given information : $\overline{BD}$ bisects $\angle B$ and $\overline{BD}\perp \overline{AC}$ .

The given diagram is:

  

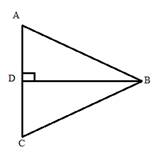
Proof :

If a line bisects an angle, then the line divides the angle into two equal and congruent parts.

If a line is perpendicular to another line, then the angles formed by the intersection of the two lines are right angles.

If there are two triangles and the non included side and two angles of one triangle are congruent to the side and the two angles of a second triangle, then the two triangles are congruent by Angle-Angle-Side (AAS) congruence.

Statements	Reasons
1. $\overline{BD}$ bisects   $\angle B$ and   $\overline{BD}\perp \overline{AC}$	Given
2. $\angle ABD\cong \angle CBD$	Definition of Angle Bisector
3.$\angle ADB\cong \angle CDB$	Definition of Perpendicular and all the right angles are congruent
4. $BD\cong BD$	Reflexive Property of Congruence
5. $\Delta ABD\cong \Delta CBD$	Angle-Angle-Side (AAS) congruence
6. $\angle A\cong \angle C$	Definition that congruent parts of congruent triangles are congruent

Hence, $\angle A\cong \angle C$