Chapter 3, Problem 77RE

To determine

To graph the rational function and show the x-andy-intercepts and asymptotes.

Explanation of Solution

Given information:

The rational function is $r\left(x\right)=\frac{3x-12}{x+1}$ .

Graph:

Factor: $r\left(x\right)=\frac{3(x-4)}{x+1}$

x-intercepts: the x-intercept are the zeros of the numerator,

  $\begin{array}{l}x-4=0\\ x=4\end{array}$

So, x-intercept is $4$ .

y-intercepts: to find y-intercept, substitute $x=0$ in the original form of the function,

  $\begin{array}{l}r\left(0\right)=\frac{3\left(0\right)-12}{\left(0\right)+1}\\ r\left(0\right)=-12\end{array}$

So, y-intercept is $-12$ .

Horizontal asymptote: the degree of numerator and denominator is same,

So the horizontal asymptote will be the division of leading coefficient of numerator and denominator,

  $\begin{array}{l}y=\frac{3}{1}\\ y=3\end{array}$

Vertical asymptote: the vertical asymptote is occurs where denominator is zero,

$\begin{array}{l}x+1=0\\ x=-1\end{array}$

So vertical asymptote is $x=-1$ .

Slant asymptote: the slant asymptote is occurs when the numerator degree is one more than denominator, using division algoritum,

  $r\left(x\right)=3-\frac{15}{x+1}$

Thus, $y=3$ is the slant asymptote.

Use the above information together with some additional values which is show in table below

To sketch the graph,

x	y
-2	18
0	-12
1	-4.5
2	-2

Thus, the graph is obtained as:

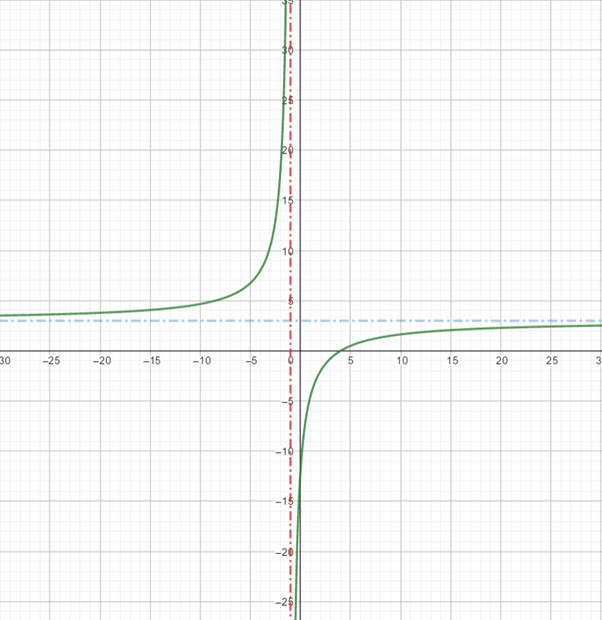
Interpretation:

From the above graph it can be observed that the x-intercept is $4$ , y-intercept is $-12$ , horizontal asymptote $y=3$ and vertical asymptote is $x=-1$ and slant asymptote is $y=3$ .