
(a)
Find the perimeter of a regular
(a)
Answer to Problem 24WE
2nsin(180n) , 2ntan(180n)
Explanation of Solution
Formula Used:
Law of Sines:
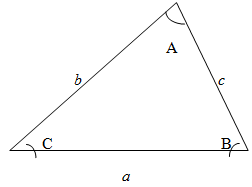
asinA=bsinB=csinC
Calculation:
The n -gon has n sides and if we join the sides to the centre of the circle, it forms n isosceles

Since there are n equal triangles, the angle to the centre of each triangle is 360°n .
Let base of each triangle is x .
In the isosceles triangle , the central angle is 360°n , the other two angles are equal since the triangle is isosceles , so each angle is 90°−180°n..............[sum of all angles is 180°] .
Use the law of sines to find x :
1sin(90−180n)=xsin(360n)⇒x=sin(2⋅180n)cos(180n).....................[multiply each side by sin(360n) and sin(90-θ)=cosθ]⇒x=2sin(180n)cos(180n)cos(180n)..................[sin2θ=2sinθcosθ]⇒x=2sin(180n)
Since the n -gon has n sides , and one of the side is x , so , the perimeter of the n -gon inscribed inside a unit circle is nx :
nx=2nsin(180n)
The n -gon circumscribed about the unit circle has n sides and if we join the sides to the centre of the circle, it forms n triangles:

Since there are n equal triangles, the angle to the centre of each triangle is 360°n .
So, the central angle of the right angled triangle that bisects the triangle is 180°n
Let base of each right angled triangle is x and height is 1.
So,
tanθ=side opposite to θside adjacent to θ⇒tan(180n)=x1⇒x=tan(180n)
Eace side of the n - gon is 2x = 2tan(180n)
Since the n -gon has n sides , and one of the side is 2tan(180n) , so , the perimeter of the n -gon circumscribed about a unit circle is:
2ntan(180n)
(b)
Find where the number is approached when n gets larger and larger:
nsin(180n) , ntan(180n)
(b)
Answer to Problem 24WE
both the numbers approaches 0 as n becomes larger and larger.
Explanation of Solution
Calculation :
As n becomes larger and larger , 180n becomes smaller and smaller. So,
n→∞⇒180n→0⇒nsin180n→0 and ntan180n→0
Hence , both the numbers approaches 0 as n becomes larger and larger.
Chapter 12 Solutions
Algebra and Trigonometry: Structure and Method, Book 2
Additional Math Textbook Solutions
University Calculus: Early Transcendentals (4th Edition)
Introductory Statistics
Pre-Algebra Student Edition
Calculus: Early Transcendentals (2nd Edition)
Elementary Statistics (13th Edition)
A First Course in Probability (10th Edition)
- Safari File Edit View History Bookmarks Window Help Ο Ω OV O mA 0 mW ర Fri Apr 4 1 222 tv A F9 F10 DII 4 F6 F7 F8 7 29 8 00 W E R T Y U S D பட 9 O G H J K E F11 + 11 F12 O P } [arrow_forwardSo confused. Step by step instructions pleasearrow_forwardIn simplest terms, Sketch the graph of the parabola. Then, determine its equation. opens downward, vertex is (- 4, 7), passes through point (0, - 39)arrow_forward
- In simplest way, For each quadratic relation, find the zeros and the maximum or minimum. a) y = x 2 + 16 x + 39 b) y = 5 x2 - 50 x - 120arrow_forwardIn simplest terms and step by step Write each quadratic relation in standard form, then fi nd the zeros. y = - 4( x + 6)2 + 36arrow_forwardIn simplest terms and step by step For each quadratic relation, find the zeros and the maximum or minimum. 1) y = - 2 x2 - 28 x + 64 2) y = 6 x2 + 36 x - 42arrow_forward
- Write each relation in standard form a)y = 5(x + 10)2 + 7 b)y = 9(x - 8)2 - 4arrow_forwardIn simplest form and step by step Write the quadratic relation in standard form, then fi nd the zeros. y = 3(x - 1)2 - 147arrow_forwardStep by step instructions The path of a soccer ball can be modelled by the relation h = - 0.1 d 2 + 0.5 d + 0.6, where h is the ball’s height and d is the horizontal distance from the kicker. a) Find the zeros of the relation.arrow_forward
 Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning
Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON
Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press
Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education
College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education





