Geometry, Student Edition
Geometry, Student Edition
1st Edition
ISBN: 9780078884849
Author: McGraw-Hill, McGraw Hill
Publisher: Glencoe/McGraw-Hill School Pub Co
bartleby

Videos

Question
Book Icon
Chapter 12.3, Problem 3CYU
To determine

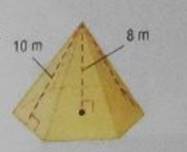
Find the Lateral area and surface area of the regular pyramid.

Expert Solution & Answer
Check Mark

Answer to Problem 3CYU

L = 207.8m2

S = 332.5m2

Explanation of Solution

Given:

  Geometry, Student Edition, Chapter 12.3, Problem 3CYU , additional homework tip 1

Formula Used: The Lateral area L of a regular Pyramid is

  L=12Pl , where l is the slant height and P is the perimeter of the base .

The Surface area S of a regular Pyramid is

  S=12Pl+B , where l is the slant height, P is the perimeter of the base and B is the area of the base .

Calculation:

The base of the pyramid is regular hexagon.As shown in the figure, find the base of the right angled triangle whose one leg is 8 m and hypotenuse is 10 m.

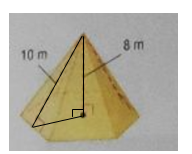
  Geometry, Student Edition, Chapter 12.3, Problem 3CYU , additional homework tip 2

Using Pythagoras Theorem :

So, the length of the base b = √102−82=√100−64=6m

Now, the base is a regular hexagon .Divide the base in 6 equal triangles with height of each triangle 6m.

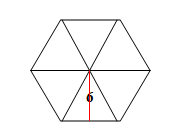
  Geometry, Student Edition, Chapter 12.3, Problem 3CYU , additional homework tip 3

Draw the right angled triangle out and find the base:

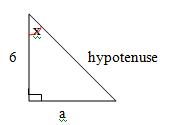
  Geometry, Student Edition, Chapter 12.3, Problem 3CYU , additional homework tip 4

Now, first find the value of x = 360°12=30°

Also,

  tanx=a6....................[tanθ=length of side opposite to θlength of side adjacent to θ]⇒tan30°=a6⇒a=6tan30°...........[multiply each side by 6]⇒a=6⋅1√3.............[tan30°=1√3]⇒a=6√3

Now, the length of base of each triangle =

  2⋅6√3=12√3⋅√3√3=4√3m

Hence , each side of the hexagon is 4√3m

Perimeter of the base = P = (length of each side )(number of sides ) = 6⋅4√3=24√3m

So, the Lateral area = L=12Pl=12(24√3)(10)=207.8m2

The area of one section of the hexagon , which is a triangle with base 4√3m and height

6 m is 12(base)(height)=12(4√3)(6)=12√3cm2

So, the area of the base = B = 6×12√3=124.71cm2 ....[there are 6 equal triangles ]

So, the surface area of the pyramid :

  S=12Pl+B=207.8+124.71=332.5m2

Hence ,

L = 207.8m2

S = 332.5m2

Previous
Chapter 12.3, Problem 2CYU
Next
Chapter 12.3, Problem 4CYU

Chapter 12 Solutions

Geometry, Student Edition

Ch. 12.1 - Prob. 1CYPCh. 12.1 - Prob. 2CYPCh. 12.1 - Prob. 3CYPCh. 12.1 - Prob. 1CYUCh. 12.1 - Prob. 2CYUCh. 12.1 - Prob. 3CYUCh. 12.1 - Prob. 4CYUCh. 12.1 - Prob. 5CYUCh. 12.1 - Prob. 6CYUCh. 12.1 - Prob. 7CYU
Ch. 12.1 - Prob. 8PPSCh. 12.1 - Prob. 9PPSCh. 12.1 - Prob. 10PPSCh. 12.1 - Prob. 11PPSCh. 12.1 - Prob. 12PPSCh. 12.1 - Prob. 13PPSCh. 12.1 - Prob. 14PPSCh. 12.1 - Prob. 15PPSCh. 12.1 - Prob. 16PPSCh. 12.1 - Prob. 17PPSCh. 12.1 - Prob. 18PPSCh. 12.1 - Prob. 19PPSCh. 12.1 - Prob. 20PPSCh. 12.1 - Prob. 21PPSCh. 12.1 - Prob. 22PPSCh. 12.1 - Prob. 23PPSCh. 12.1 - Prob. 24PPSCh. 12.1 - Prob. 25PPSCh. 12.1 - Prob. 26PPSCh. 12.1 - Prob. 27PPSCh. 12.1 - Prob. 28PPSCh. 12.1 - Prob. 29PPSCh. 12.1 - Prob. 30PPSCh. 12.1 - Prob. 31PPSCh. 12.1 - Prob. 32PPSCh. 12.1 - Prob. 33PPSCh. 12.1 - Prob. 34PPSCh. 12.1 - Prob. 35HPCh. 12.1 - Prob. 36HPCh. 12.1 - Prob. 37HPCh. 12.1 - Prob. 38HPCh. 12.1 - Prob. 39HPCh. 12.1 - Prob. 40STPCh. 12.1 - Prob. 41STPCh. 12.1 - Prob. 42STPCh. 12.1 - Prob. 43STPCh. 12.1 - Prob. 44SPRCh. 12.1 - Prob. 45SPRCh. 12.1 - Prob. 46SPRCh. 12.1 - Prob. 47SPRCh. 12.1 - Prob. 48SRCh. 12.1 - Prob. 49SRCh. 12.1 - Prob. 50SRCh. 12.2 - Prob. 1CYPCh. 12.2 - Prob. 2CYPCh. 12.2 - Prob. 3ACYPCh. 12.2 - Prob. 3BCYPCh. 12.2 - Prob. 4CYPCh. 12.2 - Prob. 1CYUCh. 12.2 - Prob. 2CYUCh. 12.2 - Prob. 3CYUCh. 12.2 - Prob. 4CYUCh. 12.2 - Prob. 5CYUCh. 12.2 - Prob. 6CYUCh. 12.2 - Prob. 7CYUCh. 12.2 - Prob. 8CYUCh. 12.2 - Prob. 9PPSCh. 12.2 - Prob. 10PPSCh. 12.2 - Prob. 11PPSCh. 12.2 - Prob. 12PPSCh. 12.2 - Prob. 13PPSCh. 12.2 - Prob. 14PPSCh. 12.2 - Prob. 15PPSCh. 12.2 - Prob. 16PPSCh. 12.2 - Prob. 17PPSCh. 12.2 - Prob. 18PPSCh. 12.2 - Prob. 19PPSCh. 12.2 - Prob. 20PPSCh. 12.2 - Prob. 21PPSCh. 12.2 - Prob. 22PPSCh. 12.2 - Prob. 23PPSCh. 12.2 - Prob. 24PPSCh. 12.2 - Prob. 25PPSCh. 12.2 - Prob. 26PPSCh. 12.2 - Prob. 27PPSCh. 12.2 - Prob. 28PPSCh. 12.2 - Prob. 29PPSCh. 12.2 - Prob. 30PPSCh. 12.2 - Prob. 31PPSCh. 12.2 - Prob. 32PPSCh. 12.2 - Prob. 33PPSCh. 12.2 - Prob. 34PPSCh. 12.2 - Prob. 35PPSCh. 12.2 - Prob. 36PPSCh. 12.2 - Prob. 37PPSCh. 12.2 - Prob. 38PPSCh. 12.2 - Prob. 39HPCh. 12.2 - Prob. 40HPCh. 12.2 - Prob. 41HPCh. 12.2 - Prob. 42HPCh. 12.2 - Prob. 43HPCh. 12.2 - Prob. 44HPCh. 12.2 - Prob. 45STPCh. 12.2 - Prob. 46STPCh. 12.2 - Prob. 47STPCh. 12.2 - Prob. 48STPCh. 12.2 - Prob. 49SPRCh. 12.2 - Prob. 50SPRCh. 12.2 - Prob. 51SPRCh. 12.2 - Prob. 52SPRCh. 12.2 - Prob. 53SPRCh. 12.2 - Prob. 54SRCh. 12.2 - Prob. 55SRCh. 12.2 - Prob. 56SRCh. 12.3 - Prob. 1CYPCh. 12.3 - Prob. 2ACYPCh. 12.3 - Prob. 2BCYPCh. 12.3 - Prob. 3ACYPCh. 12.3 - Prob. 3BCYPCh. 12.3 - Prob. 4CYPCh. 12.3 - Prob. 5ACYPCh. 12.3 - Prob. 5BCYPCh. 12.3 - Prob. 1CYUCh. 12.3 - Prob. 2CYUCh. 12.3 - Prob. 3CYUCh. 12.3 - Prob. 4CYUCh. 12.3 - Prob. 5CYUCh. 12.3 - Prob. 6CYUCh. 12.3 - Prob. 7PPSCh. 12.3 - Prob. 8PPSCh. 12.3 - Prob. 9PPSCh. 12.3 - Prob. 10PPSCh. 12.3 - Prob. 11PPSCh. 12.3 - Prob. 12PPSCh. 12.3 - Prob. 13PPSCh. 12.3 - Prob. 14PPSCh. 12.3 - Prob. 15PPSCh. 12.3 - Prob. 16PPSCh. 12.3 - Prob. 17PPSCh. 12.3 - Prob. 18PPSCh. 12.3 - Prob. 19PPSCh. 12.3 - Prob. 20PPSCh. 12.3 - Prob. 21PPSCh. 12.3 - Prob. 22PPSCh. 12.3 - Prob. 23PPSCh. 12.3 - Prob. 24PPSCh. 12.3 - Prob. 25PPSCh. 12.3 - Prob. 26PPSCh. 12.3 - Prob. 27PPSCh. 12.3 - Prob. 28PPSCh. 12.3 - Prob. 29PPSCh. 12.3 - Prob. 30PPSCh. 12.3 - Prob. 31PPSCh. 12.3 - Prob. 32PPSCh. 12.3 - Prob. 33PPSCh. 12.3 - Prob. 34PPSCh. 12.3 - Prob. 35PPSCh. 12.3 - Prob. 36PPSCh. 12.3 - Prob. 37HPCh. 12.3 - Prob. 38HPCh. 12.3 - Prob. 39HPCh. 12.3 - Prob. 40HPCh. 12.3 - Prob. 41HPCh. 12.3 - Prob. 42HPCh. 12.3 - Prob. 43STPCh. 12.3 - Prob. 44STPCh. 12.3 - Prob. 45STPCh. 12.3 - Prob. 46SPRCh. 12.3 - Prob. 47SPRCh. 12.3 - Prob. 48SPRCh. 12.3 - Prob. 49SPRCh. 12.3 - Prob. 50SPRCh. 12.3 - Prob. 51SPRCh. 12.3 - Prob. 52SPRCh. 12.3 - Prob. 53SPRCh. 12.3 - Prob. 54SPRCh. 12.3 - Prob. 55SRCh. 12.3 - Prob. 56SRCh. 12.3 - Prob. 57SRCh. 12.4 - Prob. 1ACYPCh. 12.4 - Prob. 1BCYPCh. 12.4 - Prob. 2ACYPCh. 12.4 - Prob. 2BCYPCh. 12.4 - Prob. 3CYPCh. 12.4 - Prob. 4CYPCh. 12.4 - Prob. 1CYUCh. 12.4 - Prob. 2CYUCh. 12.4 - Prob. 3CYUCh. 12.4 - Prob. 4CYUCh. 12.4 - Prob. 5CYUCh. 12.4 - Prob. 6CYUCh. 12.4 - Prob. 7CYUCh. 12.4 - Prob. 8CYUCh. 12.4 - Prob. 9CYUCh. 12.4 - Prob. 10PPSCh. 12.4 - Prob. 11PPSCh. 12.4 - Prob. 12PPSCh. 12.4 - Prob. 13PPSCh. 12.4 - Prob. 14PPSCh. 12.4 - Prob. 15PPSCh. 12.4 - Prob. 16PPSCh. 12.4 - Prob. 17PPSCh. 12.4 - Prob. 18PPSCh. 12.4 - Prob. 19PPSCh. 12.4 - Prob. 20PPSCh. 12.4 - Prob. 21PPSCh. 12.4 - Prob. 22PPSCh. 12.4 - Prob. 23PPSCh. 12.4 - Prob. 24PPSCh. 12.4 - Prob. 25PPSCh. 12.4 - Prob. 26PPSCh. 12.4 - Prob. 27PPSCh. 12.4 - Prob. 28PPSCh. 12.4 - Prob. 29PPSCh. 12.4 - Prob. 30PPSCh. 12.4 - Prob. 31PPSCh. 12.4 - Prob. 32PPSCh. 12.4 - Prob. 33PPSCh. 12.4 - Prob. 34PPSCh. 12.4 - Prob. 35PPSCh. 12.4 - Prob. 36PPSCh. 12.4 - Prob. 37PPSCh. 12.4 - Prob. 38PPSCh. 12.4 - Prob. 39PPSCh. 12.4 - Prob. 40PPSCh. 12.4 - Prob. 41PPSCh. 12.4 - Prob. 42HPCh. 12.4 - Prob. 43HPCh. 12.4 - Prob. 44HPCh. 12.4 - Prob. 45HPCh. 12.4 - Prob. 46HPCh. 12.4 - Prob. 47HPCh. 12.4 - Prob. 48STPCh. 12.4 - Prob. 49STPCh. 12.4 - Prob. 50STPCh. 12.4 - Prob. 51STPCh. 12.4 - Prob. 52SPRCh. 12.4 - Prob. 53SPRCh. 12.4 - Prob. 54SPRCh. 12.4 - Prob. 55SPRCh. 12.4 - Prob. 56SPRCh. 12.4 - Prob. 57SPRCh. 12.4 - Prob. 58SPRCh. 12.4 - Prob. 59SPRCh. 12.4 - Prob. 60SRCh. 12.4 - Prob. 61SRCh. 12.4 - Prob. 62SRCh. 12.5 - Prob. 1ACYPCh. 12.5 - Prob. 1BCYPCh. 12.5 - Prob. 2ACYPCh. 12.5 - Prob. 2BCYPCh. 12.5 - Prob. 2CCYPCh. 12.5 - Prob. 3CYPCh. 12.5 - Prob. 1CYUCh. 12.5 - Prob. 2CYUCh. 12.5 - Prob. 3CYUCh. 12.5 - Prob. 4CYUCh. 12.5 - Prob. 5CYUCh. 12.5 - Prob. 6CYUCh. 12.5 - Prob. 7CYUCh. 12.5 - Prob. 8CYUCh. 12.5 - Prob. 9CYUCh. 12.5 - Prob. 10PPSCh. 12.5 - Prob. 11PPSCh. 12.5 - Prob. 12PPSCh. 12.5 - Prob. 13PPSCh. 12.5 - Prob. 14PPSCh. 12.5 - Prob. 15PPSCh. 12.5 - Prob. 16PPSCh. 12.5 - Prob. 17PPSCh. 12.5 - Prob. 18PPSCh. 12.5 - Prob. 19PPSCh. 12.5 - Prob. 20PPSCh. 12.5 - Prob. 21PPSCh. 12.5 - Prob. 22PPSCh. 12.5 - Prob. 23PPSCh. 12.5 - Prob. 24PPSCh. 12.5 - Prob. 25PPSCh. 12.5 - Prob. 26PPSCh. 12.5 - Prob. 27PPSCh. 12.5 - Prob. 28PPSCh. 12.5 - Prob. 29PPSCh. 12.5 - Prob. 30PPSCh. 12.5 - Prob. 31PPSCh. 12.5 - Prob. 32PPSCh. 12.5 - Prob. 33PPSCh. 12.5 - Prob. 34PPSCh. 12.5 - Prob. 35PPSCh. 12.5 - Prob. 36HPCh. 12.5 - Prob. 37HPCh. 12.5 - Prob. 38HPCh. 12.5 - Prob. 39HPCh. 12.5 - Prob. 40HPCh. 12.5 - Prob. 41STPCh. 12.5 - Prob. 42STPCh. 12.5 - Prob. 43STPCh. 12.5 - Prob. 44STPCh. 12.5 - Prob. 45SPRCh. 12.5 - Prob. 46SPRCh. 12.5 - Prob. 47SPRCh. 12.5 - Prob. 48SPRCh. 12.5 - Prob. 49SRCh. 12.5 - Prob. 50SRCh. 12.5 - Prob. 51SRCh. 12.5 - Prob. 52SRCh. 12.6 - Prob. 1ACYPCh. 12.6 - Prob. 1BCYPCh. 12.6 - Prob. 2ACYPCh. 12.6 - Prob. 2BCYPCh. 12.6 - Prob. 2CCYPCh. 12.6 - Prob. 3ACYPCh. 12.6 - Prob. 3BCYPCh. 12.6 - Prob. 4CYPCh. 12.6 - Prob. 1CYUCh. 12.6 - Prob. 2CYUCh. 12.6 - Prob. 3CYUCh. 12.6 - Prob. 4CYUCh. 12.6 - Prob. 5CYUCh. 12.6 - Prob. 6CYUCh. 12.6 - Prob. 7CYUCh. 12.6 - Prob. 8CYUCh. 12.6 - Prob. 9CYUCh. 12.6 - Prob. 10PPSCh. 12.6 - Prob. 11PPSCh. 12.6 - Prob. 12PPSCh. 12.6 - Prob. 13PPSCh. 12.6 - Prob. 14PPSCh. 12.6 - Prob. 15PPSCh. 12.6 - Prob. 16PPSCh. 12.6 - Prob. 17PPSCh. 12.6 - Prob. 18PPSCh. 12.6 - Prob. 19PPSCh. 12.6 - Prob. 20PPSCh. 12.6 - Prob. 21PPSCh. 12.6 - Prob. 22PPSCh. 12.6 - Prob. 23PPSCh. 12.6 - Prob. 24PPSCh. 12.6 - Prob. 25PPSCh. 12.6 - Prob. 26PPSCh. 12.6 - Prob. 27PPSCh. 12.6 - Prob. 28PPSCh. 12.6 - Prob. 29PPSCh. 12.6 - Prob. 30PPSCh. 12.6 - Prob. 31PPSCh. 12.6 - Prob. 32PPSCh. 12.6 - Prob. 33PPSCh. 12.6 - Prob. 34PPSCh. 12.6 - Prob. 35PPSCh. 12.6 - Prob. 36PPSCh. 12.6 - Prob. 37PPSCh. 12.6 - Prob. 38PPSCh. 12.6 - Prob. 39PPSCh. 12.6 - Prob. 40PPSCh. 12.6 - Prob. 41PPSCh. 12.6 - Prob. 42PPSCh. 12.6 - Prob. 43PPSCh. 12.6 - Prob. 44PPSCh. 12.6 - Prob. 45HPCh. 12.6 - Prob. 46HPCh. 12.6 - Prob. 47HPCh. 12.6 - Prob. 48HPCh. 12.6 - Prob. 49STPCh. 12.6 - Prob. 50STPCh. 12.6 - Prob. 51STPCh. 12.6 - Prob. 52STPCh. 12.6 - Prob. 53SPRCh. 12.6 - Prob. 54SPRCh. 12.6 - Prob. 55SPRCh. 12.6 - Prob. 56SPRCh. 12.6 - Prob. 57SPRCh. 12.6 - Prob. 58SPRCh. 12.6 - Prob. 59SPRCh. 12.6 - Prob. 60SPRCh. 12.6 - Prob. 61SPRCh. 12.6 - Prob. 62SRCh. 12.6 - Prob. 63SRCh. 12.6 - Prob. 64SRCh. 12.6 - Prob. 65SRCh. 12.7 - Prob. 1ACYPCh. 12.7 - Prob. 1BCYPCh. 12.7 - Prob. 1CCYPCh. 12.7 - Prob. 2CYPCh. 12.7 - Prob. 3ACYPCh. 12.7 - Prob. 3BCYPCh. 12.7 - Prob. 1CYUCh. 12.7 - Prob. 2CYUCh. 12.7 - Prob. 3CYUCh. 12.7 - Prob. 4CYUCh. 12.7 - Prob. 5CYUCh. 12.7 - Prob. 6CYUCh. 12.7 - Prob. 7CYUCh. 12.7 - Prob. 8CYUCh. 12.7 - Prob. 9PPSCh. 12.7 - Prob. 10PPSCh. 12.7 - Prob. 11PPSCh. 12.7 - Prob. 12PPSCh. 12.7 - Prob. 13PPSCh. 12.7 - Prob. 14PPSCh. 12.7 - Prob. 15PPSCh. 12.7 - Prob. 16PPSCh. 12.7 - Prob. 17PPSCh. 12.7 - Prob. 18PPSCh. 12.7 - Prob. 19PPSCh. 12.7 - Prob. 20PPSCh. 12.7 - Prob. 21PPSCh. 12.7 - Prob. 22PPSCh. 12.7 - Prob. 23PPSCh. 12.7 - Prob. 24HPCh. 12.7 - Prob. 25HPCh. 12.7 - Prob. 26HPCh. 12.7 - Prob. 27HPCh. 12.7 - Prob. 28HPCh. 12.7 - Prob. 29HPCh. 12.7 - Prob. 30HPCh. 12.7 - Prob. 31STPCh. 12.7 - Prob. 32STPCh. 12.7 - Prob. 33STPCh. 12.7 - Prob. 34STPCh. 12.7 - Prob. 35SPRCh. 12.7 - Prob. 36SPRCh. 12.7 - Prob. 37SPRCh. 12.7 - Prob. 38SPRCh. 12.7 - Prob. 39SPRCh. 12.7 - Prob. 40SPRCh. 12.7 - Prob. 41SPRCh. 12.7 - Prob. 42SPRCh. 12.7 - Prob. 43SRCh. 12.7 - Prob. 44SRCh. 12.7 - Prob. 45SRCh. 12.8 - Prob. 1ACYPCh. 12.8 - Prob. 1BCYPCh. 12.8 - Prob. 2CYPCh. 12.8 - Prob. 3CYPCh. 12.8 - Prob. 1CYUCh. 12.8 - Prob. 2CYUCh. 12.8 - Prob. 3CYUCh. 12.8 - Prob. 4CYUCh. 12.8 - Prob. 5CYUCh. 12.8 - Prob. 6PPSCh. 12.8 - Prob. 7PPSCh. 12.8 - Prob. 8PPSCh. 12.8 - Prob. 9PPSCh. 12.8 - Prob. 10PPSCh. 12.8 - Prob. 11PPSCh. 12.8 - Prob. 12PPSCh. 12.8 - Prob. 13PPSCh. 12.8 - Prob. 14PPSCh. 12.8 - Prob. 15PPSCh. 12.8 - Prob. 16PPSCh. 12.8 - Prob. 17PPSCh. 12.8 - Prob. 18PPSCh. 12.8 - Prob. 19PPSCh. 12.8 - Prob. 20PPSCh. 12.8 - Prob. 21PPSCh. 12.8 - Prob. 22PPSCh. 12.8 - Prob. 23PPSCh. 12.8 - Prob. 24PPSCh. 12.8 - Prob. 25PPSCh. 12.8 - Prob. 26PPSCh. 12.8 - Prob. 27HPCh. 12.8 - Prob. 28HPCh. 12.8 - Prob. 29HPCh. 12.8 - Prob. 30HPCh. 12.8 - Prob. 31HPCh. 12.8 - Prob. 32HPCh. 12.8 - Prob. 33STPCh. 12.8 - Prob. 34STPCh. 12.8 - Prob. 35STPCh. 12.8 - Prob. 36STPCh. 12.8 - Prob. 37SPRCh. 12.8 - Prob. 38SPRCh. 12.8 - Prob. 39SPRCh. 12.8 - Prob. 40SPRCh. 12.8 - Prob. 41SPRCh. 12.8 - Prob. 42SPRCh. 12.8 - Prob. 43SPRCh. 12.8 - Prob. 44SRCh. 12.8 - Prob. 45SRCh. 12.8 - Prob. 46SRCh. 12.8 - Prob. 47SRCh. 12 - Prob. 1GRFCCh. 12 - Prob. 2GRFCCh. 12 - Prob. 3GRFCCh. 12 - Prob. 4GRFCCh. 12 - Prob. 5GRFCCh. 12 - Prob. 6GRFCCh. 12 - Prob. 7GRFCCh. 12 - Prob. 8GRFCCh. 12 - Prob. 9GRFCCh. 12 - Prob. 10GRFCCh. 12 - Prob. 11GRFCCh. 12 - Prob. 12GRFCCh. 12 - Prob. 1MCQCh. 12 - Prob. 2MCQCh. 12 - Prob. 3MCQCh. 12 - Prob. 4MCQCh. 12 - Prob. 5MCQCh. 12 - Prob. 6MCQCh. 12 - Prob. 7MCQCh. 12 - Prob. 8MCQCh. 12 - Prob. 9MCQCh. 12 - Prob. 10MCQCh. 12 - Prob. 11MCQCh. 12 - Prob. 12MCQCh. 12 - Prob. 13MCQCh. 12 - Prob. 14MCQCh. 12 - Prob. 15MCQCh. 12 - Prob. 16MCQCh. 12 - Prob. 17MCQCh. 12 - Prob. 18MCQCh. 12 - Prob. 19MCQCh. 12 - Prob. 20MCQCh. 12 - Prob. 1SGRCh. 12 - Prob. 2SGRCh. 12 - Prob. 3SGRCh. 12 - Prob. 4SGRCh. 12 - Prob. 5SGRCh. 12 - Prob. 6SGRCh. 12 - Prob. 7SGRCh. 12 - Prob. 8SGRCh. 12 - Prob. 9SGRCh. 12 - Prob. 10SGRCh. 12 - Prob. 11SGRCh. 12 - Prob. 12SGRCh. 12 - Prob. 13SGRCh. 12 - Prob. 14SGRCh. 12 - Prob. 15SGRCh. 12 - Prob. 16SGRCh. 12 - Prob. 17SGRCh. 12 - Prob. 18SGRCh. 12 - Prob. 19SGRCh. 12 - Prob. 20SGRCh. 12 - Prob. 21SGRCh. 12 - Prob. 22SGRCh. 12 - Prob. 23SGRCh. 12 - Prob. 24SGRCh. 12 - Prob. 25SGRCh. 12 - Prob. 26SGRCh. 12 - Prob. 27SGRCh. 12 - Prob. 28SGRCh. 12 - Prob. 29SGRCh. 12 - Prob. 30SGRCh. 12 - Prob. 31SGRCh. 12 - Prob. 32SGRCh. 12 - Prob. 33SGRCh. 12 - Prob. 34SGRCh. 12 - Prob. 35SGRCh. 12 - Prob. 36SGRCh. 12 - Prob. 37SGRCh. 12 - Prob. 38SGRCh. 12 - Prob. 39SGRCh. 12 - Prob. 40SGRCh. 12 - Prob. 41SGRCh. 12 - Prob. 42SGRCh. 12 - Prob. 1PTCh. 12 - Prob. 2PTCh. 12 - Prob. 3PTCh. 12 - Prob. 4PTCh. 12 - Prob. 5PTCh. 12 - Prob. 6PTCh. 12 - Prob. 7PTCh. 12 - Prob. 8PTCh. 12 - Prob. 9PTCh. 12 - Prob. 10PTCh. 12 - Prob. 11PTCh. 12 - Prob. 12PTCh. 12 - Prob. 13PTCh. 12 - Prob. 14PTCh. 12 - Prob. 15PTCh. 12 - Prob. 16PTCh. 12 - Prob. 17PTCh. 12 - Prob. 18PTCh. 12 - Prob. 19PTCh. 12 - Prob. 20PTCh. 12 - Prob. 1PSTCh. 12 - Prob. 2PSTCh. 12 - Prob. 3PSTCh. 12 - Prob. 4PSTCh. 12 - Prob. 5PSTCh. 12 - Prob. 6PSTCh. 12 - Prob. 1STPCh. 12 - Prob. 2STPCh. 12 - Prob. 3STPCh. 12 - Prob. 4STPCh. 12 - Prob. 5STPCh. 12 - Prob. 6STPCh. 12 - Prob. 7STPCh. 12 - Prob. 8STPCh. 12 - Prob. 9STPCh. 12 - Prob. 10STPCh. 12 - Prob. 11STPCh. 12 - Prob. 12STPCh. 12 - Prob. 13STP

Additional Math Textbook Solutions

Find more solutions based on key concepts
Integrals in cylindrical coordinates Evaluate the following integrals in cylindrical coordinates. 19.0402/2x1x2...

Calculus: Early Transcendentals (2nd Edition)

For what values of a is continuous at every x?

University Calculus: Early Transcendentals (4th Edition)

In Problems 39-62, graph each function using the techniques of shifting, compressing, stretching, and/or reflec...

Precalculus

Twenty five people, consisting of 15 women and 10 men are lined up in a random order. Find the probability that...

A First Course in Probability (10th Edition)

The square root of the given term

Pre-Algebra Student Edition

TRY IT YOURSELF 1 Find the mean of the points scored by the 51 winning teams listed on page 39.

Elementary Statistics: Picturing the World (7th Edition)

Knowledge Booster
Background pattern image
Geometry
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, geometry and related others by exploring similar questions and additional content below.
Similar questions
SEE MORE QUESTIONS
Recommended textbooks for you
Text book image
Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,
Text book image
Elementary Geometry for College Students
Geometry
ISBN:9781285195698
Author:Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:Cengage Learning
SEE MORE TEXTBOOKS
Quadrilaterals: Missing Angles and Sides; Author: rhornfeck;https://www.youtube.com/watch?v=knVj1O0L2TM;License: Standard YouTube License, CC-BY
STD IX | State Board | Types of Quadrilateral; Author: Robomate;https://www.youtube.com/watch?v=wh0KQ4UB0EU;License: Standard YouTube License, CC-BY