Geometry, Student Edition
Geometry, Student Edition
1st Edition
ISBN: 9780078884849
Author: McGraw-Hill, McGraw Hill
Publisher: Glencoe/McGraw-Hill School Pub Co
bartleby

Videos

Question
Book Icon
Chapter 12.1, Problem 39HP
To determine

To explain about the properties of cross section of a hexagonal pyramid.

Expert Solution & Answer
Check Mark

Explanation of Solution

Given Information:

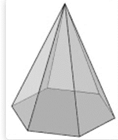
Hexagonal pyramid

  Geometry, Student Edition, Chapter 12.1, Problem 39HP , additional homework tip 1

Formula used:

A hexagonal pyramid is a pyramid with a hexagonal base upon which are erected six isosceles triangular faces that meet at a point.

Cross sections perpendicular to the base and through the vertex will be isosceles triangles.

The figure below shows about the vertical cross section:

  Geometry, Student Edition, Chapter 12.1, Problem 39HP , additional homework tip 2

There are two ways to make the cross section: The base can be cut through two opposite vertices or through the centre of two opposite sides as shown below.

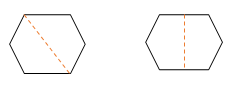
  Geometry, Student Edition, Chapter 12.1, Problem 39HP , additional homework tip 3

The cross sections will always be isosceles triangles. The length of the sides may differ, depending on the nature of cross section.

Another way of cross sectioning hexagonal pyramid is the horizontal section. Since the base of the pyramid is regular hexagon, a horizontal section will provide hexagons. However the horizontal section doesn’t separate the figure into congruent figures.

Previous
Chapter 12.1, Problem 38HP
Next
Chapter 12.1, Problem 40STP

Chapter 12 Solutions

Geometry, Student Edition

Ch. 12.1 - Prob. 1CYPCh. 12.1 - Prob. 2CYPCh. 12.1 - Prob. 3CYPCh. 12.1 - Prob. 1CYUCh. 12.1 - Prob. 2CYUCh. 12.1 - Prob. 3CYUCh. 12.1 - Prob. 4CYUCh. 12.1 - Prob. 5CYUCh. 12.1 - Prob. 6CYUCh. 12.1 - Prob. 7CYU
Ch. 12.1 - Prob. 8PPSCh. 12.1 - Prob. 9PPSCh. 12.1 - Prob. 10PPSCh. 12.1 - Prob. 11PPSCh. 12.1 - Prob. 12PPSCh. 12.1 - Prob. 13PPSCh. 12.1 - Prob. 14PPSCh. 12.1 - Prob. 15PPSCh. 12.1 - Prob. 16PPSCh. 12.1 - Prob. 17PPSCh. 12.1 - Prob. 18PPSCh. 12.1 - Prob. 19PPSCh. 12.1 - Prob. 20PPSCh. 12.1 - Prob. 21PPSCh. 12.1 - Prob. 22PPSCh. 12.1 - Prob. 23PPSCh. 12.1 - Prob. 24PPSCh. 12.1 - Prob. 25PPSCh. 12.1 - Prob. 26PPSCh. 12.1 - Prob. 27PPSCh. 12.1 - Prob. 28PPSCh. 12.1 - Prob. 29PPSCh. 12.1 - Prob. 30PPSCh. 12.1 - Prob. 31PPSCh. 12.1 - Prob. 32PPSCh. 12.1 - Prob. 33PPSCh. 12.1 - Prob. 34PPSCh. 12.1 - Prob. 35HPCh. 12.1 - Prob. 36HPCh. 12.1 - Prob. 37HPCh. 12.1 - Prob. 38HPCh. 12.1 - Prob. 39HPCh. 12.1 - Prob. 40STPCh. 12.1 - Prob. 41STPCh. 12.1 - Prob. 42STPCh. 12.1 - Prob. 43STPCh. 12.1 - Prob. 44SPRCh. 12.1 - Prob. 45SPRCh. 12.1 - Prob. 46SPRCh. 12.1 - Prob. 47SPRCh. 12.1 - Prob. 48SRCh. 12.1 - Prob. 49SRCh. 12.1 - Prob. 50SRCh. 12.2 - Prob. 1CYPCh. 12.2 - Prob. 2CYPCh. 12.2 - Prob. 3ACYPCh. 12.2 - Prob. 3BCYPCh. 12.2 - Prob. 4CYPCh. 12.2 - Prob. 1CYUCh. 12.2 - Prob. 2CYUCh. 12.2 - Prob. 3CYUCh. 12.2 - Prob. 4CYUCh. 12.2 - Prob. 5CYUCh. 12.2 - Prob. 6CYUCh. 12.2 - Prob. 7CYUCh. 12.2 - Prob. 8CYUCh. 12.2 - Prob. 9PPSCh. 12.2 - Prob. 10PPSCh. 12.2 - Prob. 11PPSCh. 12.2 - Prob. 12PPSCh. 12.2 - Prob. 13PPSCh. 12.2 - Prob. 14PPSCh. 12.2 - Prob. 15PPSCh. 12.2 - Prob. 16PPSCh. 12.2 - Prob. 17PPSCh. 12.2 - Prob. 18PPSCh. 12.2 - Prob. 19PPSCh. 12.2 - Prob. 20PPSCh. 12.2 - Prob. 21PPSCh. 12.2 - Prob. 22PPSCh. 12.2 - Prob. 23PPSCh. 12.2 - Prob. 24PPSCh. 12.2 - Prob. 25PPSCh. 12.2 - Prob. 26PPSCh. 12.2 - Prob. 27PPSCh. 12.2 - Prob. 28PPSCh. 12.2 - Prob. 29PPSCh. 12.2 - Prob. 30PPSCh. 12.2 - Prob. 31PPSCh. 12.2 - Prob. 32PPSCh. 12.2 - Prob. 33PPSCh. 12.2 - Prob. 34PPSCh. 12.2 - Prob. 35PPSCh. 12.2 - Prob. 36PPSCh. 12.2 - Prob. 37PPSCh. 12.2 - Prob. 38PPSCh. 12.2 - Prob. 39HPCh. 12.2 - Prob. 40HPCh. 12.2 - Prob. 41HPCh. 12.2 - Prob. 42HPCh. 12.2 - Prob. 43HPCh. 12.2 - Prob. 44HPCh. 12.2 - Prob. 45STPCh. 12.2 - Prob. 46STPCh. 12.2 - Prob. 47STPCh. 12.2 - Prob. 48STPCh. 12.2 - Prob. 49SPRCh. 12.2 - Prob. 50SPRCh. 12.2 - Prob. 51SPRCh. 12.2 - Prob. 52SPRCh. 12.2 - Prob. 53SPRCh. 12.2 - Prob. 54SRCh. 12.2 - Prob. 55SRCh. 12.2 - Prob. 56SRCh. 12.3 - Prob. 1CYPCh. 12.3 - Prob. 2ACYPCh. 12.3 - Prob. 2BCYPCh. 12.3 - Prob. 3ACYPCh. 12.3 - Prob. 3BCYPCh. 12.3 - Prob. 4CYPCh. 12.3 - Prob. 5ACYPCh. 12.3 - Prob. 5BCYPCh. 12.3 - Prob. 1CYUCh. 12.3 - Prob. 2CYUCh. 12.3 - Prob. 3CYUCh. 12.3 - Prob. 4CYUCh. 12.3 - Prob. 5CYUCh. 12.3 - Prob. 6CYUCh. 12.3 - Prob. 7PPSCh. 12.3 - Prob. 8PPSCh. 12.3 - Prob. 9PPSCh. 12.3 - Prob. 10PPSCh. 12.3 - Prob. 11PPSCh. 12.3 - Prob. 12PPSCh. 12.3 - Prob. 13PPSCh. 12.3 - Prob. 14PPSCh. 12.3 - Prob. 15PPSCh. 12.3 - Prob. 16PPSCh. 12.3 - Prob. 17PPSCh. 12.3 - Prob. 18PPSCh. 12.3 - Prob. 19PPSCh. 12.3 - Prob. 20PPSCh. 12.3 - Prob. 21PPSCh. 12.3 - Prob. 22PPSCh. 12.3 - Prob. 23PPSCh. 12.3 - Prob. 24PPSCh. 12.3 - Prob. 25PPSCh. 12.3 - Prob. 26PPSCh. 12.3 - Prob. 27PPSCh. 12.3 - Prob. 28PPSCh. 12.3 - Prob. 29PPSCh. 12.3 - Prob. 30PPSCh. 12.3 - Prob. 31PPSCh. 12.3 - Prob. 32PPSCh. 12.3 - Prob. 33PPSCh. 12.3 - Prob. 34PPSCh. 12.3 - Prob. 35PPSCh. 12.3 - Prob. 36PPSCh. 12.3 - Prob. 37HPCh. 12.3 - Prob. 38HPCh. 12.3 - Prob. 39HPCh. 12.3 - Prob. 40HPCh. 12.3 - Prob. 41HPCh. 12.3 - Prob. 42HPCh. 12.3 - Prob. 43STPCh. 12.3 - Prob. 44STPCh. 12.3 - Prob. 45STPCh. 12.3 - Prob. 46SPRCh. 12.3 - Prob. 47SPRCh. 12.3 - Prob. 48SPRCh. 12.3 - Prob. 49SPRCh. 12.3 - Prob. 50SPRCh. 12.3 - Prob. 51SPRCh. 12.3 - Prob. 52SPRCh. 12.3 - Prob. 53SPRCh. 12.3 - Prob. 54SPRCh. 12.3 - Prob. 55SRCh. 12.3 - Prob. 56SRCh. 12.3 - Prob. 57SRCh. 12.4 - Prob. 1ACYPCh. 12.4 - Prob. 1BCYPCh. 12.4 - Prob. 2ACYPCh. 12.4 - Prob. 2BCYPCh. 12.4 - Prob. 3CYPCh. 12.4 - Prob. 4CYPCh. 12.4 - Prob. 1CYUCh. 12.4 - Prob. 2CYUCh. 12.4 - Prob. 3CYUCh. 12.4 - Prob. 4CYUCh. 12.4 - Prob. 5CYUCh. 12.4 - Prob. 6CYUCh. 12.4 - Prob. 7CYUCh. 12.4 - Prob. 8CYUCh. 12.4 - Prob. 9CYUCh. 12.4 - Prob. 10PPSCh. 12.4 - Prob. 11PPSCh. 12.4 - Prob. 12PPSCh. 12.4 - Prob. 13PPSCh. 12.4 - Prob. 14PPSCh. 12.4 - Prob. 15PPSCh. 12.4 - Prob. 16PPSCh. 12.4 - Prob. 17PPSCh. 12.4 - Prob. 18PPSCh. 12.4 - Prob. 19PPSCh. 12.4 - Prob. 20PPSCh. 12.4 - Prob. 21PPSCh. 12.4 - Prob. 22PPSCh. 12.4 - Prob. 23PPSCh. 12.4 - Prob. 24PPSCh. 12.4 - Prob. 25PPSCh. 12.4 - Prob. 26PPSCh. 12.4 - Prob. 27PPSCh. 12.4 - Prob. 28PPSCh. 12.4 - Prob. 29PPSCh. 12.4 - Prob. 30PPSCh. 12.4 - Prob. 31PPSCh. 12.4 - Prob. 32PPSCh. 12.4 - Prob. 33PPSCh. 12.4 - Prob. 34PPSCh. 12.4 - Prob. 35PPSCh. 12.4 - Prob. 36PPSCh. 12.4 - Prob. 37PPSCh. 12.4 - Prob. 38PPSCh. 12.4 - Prob. 39PPSCh. 12.4 - Prob. 40PPSCh. 12.4 - Prob. 41PPSCh. 12.4 - Prob. 42HPCh. 12.4 - Prob. 43HPCh. 12.4 - Prob. 44HPCh. 12.4 - Prob. 45HPCh. 12.4 - Prob. 46HPCh. 12.4 - Prob. 47HPCh. 12.4 - Prob. 48STPCh. 12.4 - Prob. 49STPCh. 12.4 - Prob. 50STPCh. 12.4 - Prob. 51STPCh. 12.4 - Prob. 52SPRCh. 12.4 - Prob. 53SPRCh. 12.4 - Prob. 54SPRCh. 12.4 - Prob. 55SPRCh. 12.4 - Prob. 56SPRCh. 12.4 - Prob. 57SPRCh. 12.4 - Prob. 58SPRCh. 12.4 - Prob. 59SPRCh. 12.4 - Prob. 60SRCh. 12.4 - Prob. 61SRCh. 12.4 - Prob. 62SRCh. 12.5 - Prob. 1ACYPCh. 12.5 - Prob. 1BCYPCh. 12.5 - Prob. 2ACYPCh. 12.5 - Prob. 2BCYPCh. 12.5 - Prob. 2CCYPCh. 12.5 - Prob. 3CYPCh. 12.5 - Prob. 1CYUCh. 12.5 - Prob. 2CYUCh. 12.5 - Prob. 3CYUCh. 12.5 - Prob. 4CYUCh. 12.5 - Prob. 5CYUCh. 12.5 - Prob. 6CYUCh. 12.5 - Prob. 7CYUCh. 12.5 - Prob. 8CYUCh. 12.5 - Prob. 9CYUCh. 12.5 - Prob. 10PPSCh. 12.5 - Prob. 11PPSCh. 12.5 - Prob. 12PPSCh. 12.5 - Prob. 13PPSCh. 12.5 - Prob. 14PPSCh. 12.5 - Prob. 15PPSCh. 12.5 - Prob. 16PPSCh. 12.5 - Prob. 17PPSCh. 12.5 - Prob. 18PPSCh. 12.5 - Prob. 19PPSCh. 12.5 - Prob. 20PPSCh. 12.5 - Prob. 21PPSCh. 12.5 - Prob. 22PPSCh. 12.5 - Prob. 23PPSCh. 12.5 - Prob. 24PPSCh. 12.5 - Prob. 25PPSCh. 12.5 - Prob. 26PPSCh. 12.5 - Prob. 27PPSCh. 12.5 - Prob. 28PPSCh. 12.5 - Prob. 29PPSCh. 12.5 - Prob. 30PPSCh. 12.5 - Prob. 31PPSCh. 12.5 - Prob. 32PPSCh. 12.5 - Prob. 33PPSCh. 12.5 - Prob. 34PPSCh. 12.5 - Prob. 35PPSCh. 12.5 - Prob. 36HPCh. 12.5 - Prob. 37HPCh. 12.5 - Prob. 38HPCh. 12.5 - Prob. 39HPCh. 12.5 - Prob. 40HPCh. 12.5 - Prob. 41STPCh. 12.5 - Prob. 42STPCh. 12.5 - Prob. 43STPCh. 12.5 - Prob. 44STPCh. 12.5 - Prob. 45SPRCh. 12.5 - Prob. 46SPRCh. 12.5 - Prob. 47SPRCh. 12.5 - Prob. 48SPRCh. 12.5 - Prob. 49SRCh. 12.5 - Prob. 50SRCh. 12.5 - Prob. 51SRCh. 12.5 - Prob. 52SRCh. 12.6 - Prob. 1ACYPCh. 12.6 - Prob. 1BCYPCh. 12.6 - Prob. 2ACYPCh. 12.6 - Prob. 2BCYPCh. 12.6 - Prob. 2CCYPCh. 12.6 - Prob. 3ACYPCh. 12.6 - Prob. 3BCYPCh. 12.6 - Prob. 4CYPCh. 12.6 - Prob. 1CYUCh. 12.6 - Prob. 2CYUCh. 12.6 - Prob. 3CYUCh. 12.6 - Prob. 4CYUCh. 12.6 - Prob. 5CYUCh. 12.6 - Prob. 6CYUCh. 12.6 - Prob. 7CYUCh. 12.6 - Prob. 8CYUCh. 12.6 - Prob. 9CYUCh. 12.6 - Prob. 10PPSCh. 12.6 - Prob. 11PPSCh. 12.6 - Prob. 12PPSCh. 12.6 - Prob. 13PPSCh. 12.6 - Prob. 14PPSCh. 12.6 - Prob. 15PPSCh. 12.6 - Prob. 16PPSCh. 12.6 - Prob. 17PPSCh. 12.6 - Prob. 18PPSCh. 12.6 - Prob. 19PPSCh. 12.6 - Prob. 20PPSCh. 12.6 - Prob. 21PPSCh. 12.6 - Prob. 22PPSCh. 12.6 - Prob. 23PPSCh. 12.6 - Prob. 24PPSCh. 12.6 - Prob. 25PPSCh. 12.6 - Prob. 26PPSCh. 12.6 - Prob. 27PPSCh. 12.6 - Prob. 28PPSCh. 12.6 - Prob. 29PPSCh. 12.6 - Prob. 30PPSCh. 12.6 - Prob. 31PPSCh. 12.6 - Prob. 32PPSCh. 12.6 - Prob. 33PPSCh. 12.6 - Prob. 34PPSCh. 12.6 - Prob. 35PPSCh. 12.6 - Prob. 36PPSCh. 12.6 - Prob. 37PPSCh. 12.6 - Prob. 38PPSCh. 12.6 - Prob. 39PPSCh. 12.6 - Prob. 40PPSCh. 12.6 - Prob. 41PPSCh. 12.6 - Prob. 42PPSCh. 12.6 - Prob. 43PPSCh. 12.6 - Prob. 44PPSCh. 12.6 - Prob. 45HPCh. 12.6 - Prob. 46HPCh. 12.6 - Prob. 47HPCh. 12.6 - Prob. 48HPCh. 12.6 - Prob. 49STPCh. 12.6 - Prob. 50STPCh. 12.6 - Prob. 51STPCh. 12.6 - Prob. 52STPCh. 12.6 - Prob. 53SPRCh. 12.6 - Prob. 54SPRCh. 12.6 - Prob. 55SPRCh. 12.6 - Prob. 56SPRCh. 12.6 - Prob. 57SPRCh. 12.6 - Prob. 58SPRCh. 12.6 - Prob. 59SPRCh. 12.6 - Prob. 60SPRCh. 12.6 - Prob. 61SPRCh. 12.6 - Prob. 62SRCh. 12.6 - Prob. 63SRCh. 12.6 - Prob. 64SRCh. 12.6 - Prob. 65SRCh. 12.7 - Prob. 1ACYPCh. 12.7 - Prob. 1BCYPCh. 12.7 - Prob. 1CCYPCh. 12.7 - Prob. 2CYPCh. 12.7 - Prob. 3ACYPCh. 12.7 - Prob. 3BCYPCh. 12.7 - Prob. 1CYUCh. 12.7 - Prob. 2CYUCh. 12.7 - Prob. 3CYUCh. 12.7 - Prob. 4CYUCh. 12.7 - Prob. 5CYUCh. 12.7 - Prob. 6CYUCh. 12.7 - Prob. 7CYUCh. 12.7 - Prob. 8CYUCh. 12.7 - Prob. 9PPSCh. 12.7 - Prob. 10PPSCh. 12.7 - Prob. 11PPSCh. 12.7 - Prob. 12PPSCh. 12.7 - Prob. 13PPSCh. 12.7 - Prob. 14PPSCh. 12.7 - Prob. 15PPSCh. 12.7 - Prob. 16PPSCh. 12.7 - Prob. 17PPSCh. 12.7 - Prob. 18PPSCh. 12.7 - Prob. 19PPSCh. 12.7 - Prob. 20PPSCh. 12.7 - Prob. 21PPSCh. 12.7 - Prob. 22PPSCh. 12.7 - Prob. 23PPSCh. 12.7 - Prob. 24HPCh. 12.7 - Prob. 25HPCh. 12.7 - Prob. 26HPCh. 12.7 - Prob. 27HPCh. 12.7 - Prob. 28HPCh. 12.7 - Prob. 29HPCh. 12.7 - Prob. 30HPCh. 12.7 - Prob. 31STPCh. 12.7 - Prob. 32STPCh. 12.7 - Prob. 33STPCh. 12.7 - Prob. 34STPCh. 12.7 - Prob. 35SPRCh. 12.7 - Prob. 36SPRCh. 12.7 - Prob. 37SPRCh. 12.7 - Prob. 38SPRCh. 12.7 - Prob. 39SPRCh. 12.7 - Prob. 40SPRCh. 12.7 - Prob. 41SPRCh. 12.7 - Prob. 42SPRCh. 12.7 - Prob. 43SRCh. 12.7 - Prob. 44SRCh. 12.7 - Prob. 45SRCh. 12.8 - Prob. 1ACYPCh. 12.8 - Prob. 1BCYPCh. 12.8 - Prob. 2CYPCh. 12.8 - Prob. 3CYPCh. 12.8 - Prob. 1CYUCh. 12.8 - Prob. 2CYUCh. 12.8 - Prob. 3CYUCh. 12.8 - Prob. 4CYUCh. 12.8 - Prob. 5CYUCh. 12.8 - Prob. 6PPSCh. 12.8 - Prob. 7PPSCh. 12.8 - Prob. 8PPSCh. 12.8 - Prob. 9PPSCh. 12.8 - Prob. 10PPSCh. 12.8 - Prob. 11PPSCh. 12.8 - Prob. 12PPSCh. 12.8 - Prob. 13PPSCh. 12.8 - Prob. 14PPSCh. 12.8 - Prob. 15PPSCh. 12.8 - Prob. 16PPSCh. 12.8 - Prob. 17PPSCh. 12.8 - Prob. 18PPSCh. 12.8 - Prob. 19PPSCh. 12.8 - Prob. 20PPSCh. 12.8 - Prob. 21PPSCh. 12.8 - Prob. 22PPSCh. 12.8 - Prob. 23PPSCh. 12.8 - Prob. 24PPSCh. 12.8 - Prob. 25PPSCh. 12.8 - Prob. 26PPSCh. 12.8 - Prob. 27HPCh. 12.8 - Prob. 28HPCh. 12.8 - Prob. 29HPCh. 12.8 - Prob. 30HPCh. 12.8 - Prob. 31HPCh. 12.8 - Prob. 32HPCh. 12.8 - Prob. 33STPCh. 12.8 - Prob. 34STPCh. 12.8 - Prob. 35STPCh. 12.8 - Prob. 36STPCh. 12.8 - Prob. 37SPRCh. 12.8 - Prob. 38SPRCh. 12.8 - Prob. 39SPRCh. 12.8 - Prob. 40SPRCh. 12.8 - Prob. 41SPRCh. 12.8 - Prob. 42SPRCh. 12.8 - Prob. 43SPRCh. 12.8 - Prob. 44SRCh. 12.8 - Prob. 45SRCh. 12.8 - Prob. 46SRCh. 12.8 - Prob. 47SRCh. 12 - Prob. 1GRFCCh. 12 - Prob. 2GRFCCh. 12 - Prob. 3GRFCCh. 12 - Prob. 4GRFCCh. 12 - Prob. 5GRFCCh. 12 - Prob. 6GRFCCh. 12 - Prob. 7GRFCCh. 12 - Prob. 8GRFCCh. 12 - Prob. 9GRFCCh. 12 - Prob. 10GRFCCh. 12 - Prob. 11GRFCCh. 12 - Prob. 12GRFCCh. 12 - Prob. 1MCQCh. 12 - Prob. 2MCQCh. 12 - Prob. 3MCQCh. 12 - Prob. 4MCQCh. 12 - Prob. 5MCQCh. 12 - Prob. 6MCQCh. 12 - Prob. 7MCQCh. 12 - Prob. 8MCQCh. 12 - Prob. 9MCQCh. 12 - Prob. 10MCQCh. 12 - Prob. 11MCQCh. 12 - Prob. 12MCQCh. 12 - Prob. 13MCQCh. 12 - Prob. 14MCQCh. 12 - Prob. 15MCQCh. 12 - Prob. 16MCQCh. 12 - Prob. 17MCQCh. 12 - Prob. 18MCQCh. 12 - Prob. 19MCQCh. 12 - Prob. 20MCQCh. 12 - Prob. 1SGRCh. 12 - Prob. 2SGRCh. 12 - Prob. 3SGRCh. 12 - Prob. 4SGRCh. 12 - Prob. 5SGRCh. 12 - Prob. 6SGRCh. 12 - Prob. 7SGRCh. 12 - Prob. 8SGRCh. 12 - Prob. 9SGRCh. 12 - Prob. 10SGRCh. 12 - Prob. 11SGRCh. 12 - Prob. 12SGRCh. 12 - Prob. 13SGRCh. 12 - Prob. 14SGRCh. 12 - Prob. 15SGRCh. 12 - Prob. 16SGRCh. 12 - Prob. 17SGRCh. 12 - Prob. 18SGRCh. 12 - Prob. 19SGRCh. 12 - Prob. 20SGRCh. 12 - Prob. 21SGRCh. 12 - Prob. 22SGRCh. 12 - Prob. 23SGRCh. 12 - Prob. 24SGRCh. 12 - Prob. 25SGRCh. 12 - Prob. 26SGRCh. 12 - Prob. 27SGRCh. 12 - Prob. 28SGRCh. 12 - Prob. 29SGRCh. 12 - Prob. 30SGRCh. 12 - Prob. 31SGRCh. 12 - Prob. 32SGRCh. 12 - Prob. 33SGRCh. 12 - Prob. 34SGRCh. 12 - Prob. 35SGRCh. 12 - Prob. 36SGRCh. 12 - Prob. 37SGRCh. 12 - Prob. 38SGRCh. 12 - Prob. 39SGRCh. 12 - Prob. 40SGRCh. 12 - Prob. 41SGRCh. 12 - Prob. 42SGRCh. 12 - Prob. 1PTCh. 12 - Prob. 2PTCh. 12 - Prob. 3PTCh. 12 - Prob. 4PTCh. 12 - Prob. 5PTCh. 12 - Prob. 6PTCh. 12 - Prob. 7PTCh. 12 - Prob. 8PTCh. 12 - Prob. 9PTCh. 12 - Prob. 10PTCh. 12 - Prob. 11PTCh. 12 - Prob. 12PTCh. 12 - Prob. 13PTCh. 12 - Prob. 14PTCh. 12 - Prob. 15PTCh. 12 - Prob. 16PTCh. 12 - Prob. 17PTCh. 12 - Prob. 18PTCh. 12 - Prob. 19PTCh. 12 - Prob. 20PTCh. 12 - Prob. 1PSTCh. 12 - Prob. 2PSTCh. 12 - Prob. 3PSTCh. 12 - Prob. 4PSTCh. 12 - Prob. 5PSTCh. 12 - Prob. 6PSTCh. 12 - Prob. 1STPCh. 12 - Prob. 2STPCh. 12 - Prob. 3STPCh. 12 - Prob. 4STPCh. 12 - Prob. 5STPCh. 12 - Prob. 6STPCh. 12 - Prob. 7STPCh. 12 - Prob. 8STPCh. 12 - Prob. 9STPCh. 12 - Prob. 10STPCh. 12 - Prob. 11STPCh. 12 - Prob. 12STPCh. 12 - Prob. 13STP

Additional Math Textbook Solutions

Find more solutions based on key concepts
TRY IT YOURSELF 1 Find the mean of the points scored by the 51 winning teams listed on page 39.

Elementary Statistics: Picturing the World (7th Edition)

29-36. Total and Annual Returns. Compute the total and annual returns on the following investments. 29. Five ye...

Using and Understanding Mathematics: A Quantitative Reasoning Approach (6th Edition)

Siblings The histogram shows the distribution of the numbers of siblings (brothers and sisters) for 2000 adults...

Introductory Statistics

A pair of fair dice is rolled. What is the probability that the second die lands on a higher value than does th...

A First Course in Probability (10th Edition)

Find the areas of the surfaces generated by revolving the curves in Exercises 13–23 about the indicated axes. I...

University Calculus: Early Transcendentals (4th Edition)

Sine substitution Evaluate the following integrals. 11. 01/2x21x2dx

Calculus: Early Transcendentals (2nd Edition)

Knowledge Booster
Background pattern image
Geometry
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, geometry and related others by exploring similar questions and additional content below.
Recommended textbooks for you
Text book image
Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,
Text book image
Elementary Geometry for College Students
Geometry
ISBN:9781285195698
Author:Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:Cengage Learning
SEE MORE TEXTBOOKS
Maths - Geometry - What is an Angle - English; Author: Bodhaguru;https://www.youtube.com/watch?v=bsuDcCjFq6c;License: Standard YouTube License, CC-BY
What is an Angle? | Different Types of Angles | Geometry | Math | LetsTute; Author: Let'stute;https://www.youtube.com/watch?v=aGejx2fRCHU;License: Standard Youtube License