Chapter 12, Problem 12.12P

During radiant heat treatment of a thin-film material, its shape, which may be hemispherical (a) or spherical (b), is maintained by a relatively low air pressure (as in the case of a rubber balloon). Irradiation on the film is due to emission from a radiant heater of area $A_h=0.0052m^2$ , which emits diffusely with an intensity of ${\text{I}}_{e,h}=169,000W/m^2·sr.$

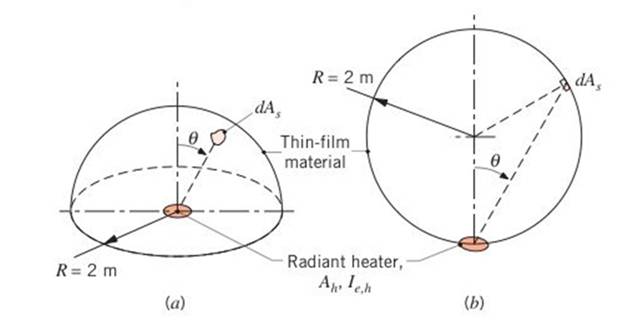
(a) Obtain an expression for the irradiation on the film as a function of the zenith angle $\theta$ .
(b) Based on the expressions derived in part (a), which shape provides the more uniform irradiation G and hence provides better quality control for the treatment process?