Chapter 10.9, Problem 109RP

(a)

To determine

The mass flow rate of air in the gas-turbine cycle.

Answer to Problem 109RP

The mass flow rate of air in the gas-turbine cycle is $\text{220}\text{.1}\text{kg}/\text{s}$.

Explanation of Solution

Show the T-s diagram as in Figure (1).

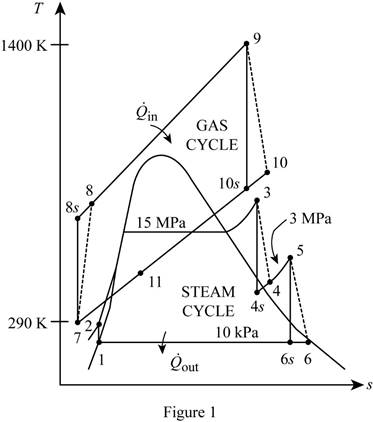
Express Prandtl number at state 8s.

$P_{r_{8s}}=\frac{P_{8s}}{P_7}{P}_{r_7}$ (I)

Here, pressure at state 8s is $P_{8s}$, pressure at state 7 is $P_7$ and Prandtl number at state 7 is $P_{r_7}$.

Express enthalpy at state 8.

$h_8=h_7+\frac{h_{8s}-h_7}{{\eta }_C}$ (II)

Here, enthalpy at state 7 is $h_7$, enthalpy at state 8s is $h_{8s}$ and the compressor efficiency is ${\eta }_C$.

Express Prandtl number at state 10s.

$P_{r_{10s}}=\frac{P_{10s}}{P_9}{P}_{r_9}$ (III)

Here, pressure at state 10s is $P_{10s}$, pressure at state 9 is $P_9$ and Prandtl number at state 9 is $P_{r_9}$.

Express enthalpy at state 10.

$h_{10}=h_9-{\eta }_T\left(h_9-h_{10s}\right)$ (IV)

Here, enthalpy at state 9 is $h_9$, compressor of the gas and steam turbine is ${\eta }_T$ and enthalpy at state 10s is $h_{10s}$.

Express enthalpy at state 1.

$h_1=h_{f\text{@10}\text{kPa}}$ (V)

Here, enthalpy of saturation liquid at pressure of $\text{10}\text{kPa}$ is $h_{f\text{@10}\text{kPa}}$.

Express specific volume at state 1.

$v_1=v_{f\text{@10}\text{kPa}}$ (VI)

Here, specific volume of saturation liquid at pressure of $\text{10}\text{kPa}$ is $v_{f\text{@10}\text{kPa}}$.

Express initial work input.

$w_{\text{pI,in}}=v_1\left(P_2-P_1\right)$ (VII)

Here, pressure at state 2 and 1 is $P_2\text{and}{P}_1$ respectively.

Express enthalpy at state 2.

$h_2=h_1+w_{\text{pI,in}}$ (VIII)

Express quality at state 4s.

$x_{4s}=\frac{s_{4s}-s_{f\text{@3}\text{MPa}}}{s_{fg\text{@3}\text{MPa}}}$ (IX)

Here, entropy at state 4s is $s_{4s}$, entropy at saturation liquid and evaporation at pressure of $\text{3}\text{MPa}$ is $s_{f\text{@3}\text{MPa}}\text{and}{s}_{fg\text{@3}\text{MPa}}$ respectively.

Express enthalpy at state 4s.

$h_{4s}=h_{f\text{@3}\text{MPa}}+x_{4s}{h}_{fg\text{@3}\text{MPa}}$ (X)

Here, enthalpy at saturation liquid and evaporation at pressure of $\text{3}\text{MPa}$ is $h_{f\text{@3}\text{MPa}}\text{and}{h}_{fg\text{@3}\text{MPa}}$ respectively.

Express enthalpy at state 4.

$h_4=h_3-{\eta }_T\left(h_3-h_{4s}\right)$ (XI)

Here, enthalpy at state 3 is $h_3$ and enthalpy at state 4s is $h_{4s}$.

Express quality at state 6s.

$x_{6s}=\frac{s_{6s}-s_{f\text{@10}\text{kPa}}}{s_{fg\text{@10}\text{kPa}}}$ (XII)

Here, entropy at state 6s is $s_{6s}$, entropy at saturation liquid and evaporation at pressure of $10\text{kPa}$ is $s_{f\text{@10}\text{kPa}}\text{and}{s}_{fg\text{@10}\text{kPa}}$ respectively.

Express enthalpy at state 6s.

$h_{6s}=h_{f\text{@10}\text{MPa}}+x_{6s}{h}_{fg\text{@10}\text{MPa}}$ (XIII)

Here, enthalpy at saturation liquid and evaporation at pressure of $10\text{MPa}$ is $h_{f\text{@10}\text{MPa}}\text{and}{h}_{fg\text{@10}\text{MPa}}$ respectively.

Express enthalpy at state 6.

$h_6=h_5-{\eta }_T\left(h_5-h_{6s}\right)$ (XIV)

Here, enthalpy at state 5 is $h_5$ and enthalpy at state 6s is $h_{6s}$.

Express the mass flow rate of air in the gas-turbine cycle from energy balance equation.

${\dot{m}}_{\text{air}}=\frac{h_3-h_2}{h_{10}-h_{11}}{\dot{m}}_s$ (XV)

Here, enthalpy at state 10 is $h_{10}$, enthalpy at state 11 is $h_{11}$, enthalpy at state 3 and 2 is $h_3\text{and}{h}_2$ respectively, and mass flow rate of steam is ${\dot{m}}_s$.

Conclusion:

Refer Table A-17, “ideal gas properties of air”, and write the enthalpy at state 7 and Prandtl number at state 7 corresponding to temperature at state 7 of $\text{290}\text{K}$.

$\begin{array}{l}{h}_7=290.16\text{kJ}/\text{kg}\\ P_{r_7}=1.2311\end{array}$

Substitute $8$ for $\frac{P_{8s}}{P_7}$ and $1.2311$ for $P_{r_7}$ in Equation (I).

$\begin{array}{c}{P}_{r_{8s}}=8\left(1.2311\right)\\ =9.849\end{array}$

Refer Table A-17, “ideal gas properties of air”, and write the enthalpy at state 8s corresponding to Prandtl number at state 8s of $9.849$ using an interpolation method.

Write the formula of interpolation method of two variables.

$y_2=\frac{\left(x_2-x_1\right)\left(y_3-y_1\right)}{\left(x_3-x_1\right)}+y_1$ (XVI)

Here, the variables denote by x and y is Prandtl number at state8s and enthalpy at state 8s respectively.

Show the enthalpy at state 8s corresponding to Prandtl number as in Table (1).

Prandtl number at state 8s $P_{r_{8s}}$	Enthalpy at state 8s $h_{8s}\left(\text{kJ}/\text{kg}\right)$
9.684 $\left(x_1\right)$	523.63 $\left(y_1\right)$
9.849 $\left(x_2\right)$	$\left(y_2=?\right)$
10.37 $\left(x_3\right)$	533.98 $\left(y_3\right)$

Substitute $\text{9}\text{.684,}\text{9}\text{.849}\text{and}\text{10}\text{.37}$ for $x_1,x_2\text{and}{x}_3$ respectively, $\text{523}\text{.63}\text{kJ}/\text{kg}$ for $y_1$ and $\text{533}\text{.98}\text{kJ}/\text{kg}$ for $y_3$ in Equation (XVI).

$\begin{array}{c}{y}_2=\frac{\left(\text{9}\text{.849}-\text{9}\text{.684}\right)\left(\text{533}\text{.98}\text{kJ}/\text{kg}-\text{523}\text{.63}\text{kJ}/\text{kg}\right)}{\left(\text{10}\text{.37}-\text{9}\text{.684}\right)}+\text{523}\text{.63}\text{kJ}/\text{kg}\\ =\text{526}\text{.12}\text{kJ}/\text{kg}\\ =h_{8s}\end{array}$

Thus, enthalpy at state 8s corresponding to Prandtl number at state 8s of $9.849$ is,

$h_{8s}=\text{526}\text{.12}\text{kJ}/\text{kg}$

Substitute $290.16\text{kJ}/\text{kg}$ for $h_7$, $\text{526}\text{.12}\text{kJ}/\text{kg}$ for $h_{8s}$ and $0.85$ for ${\eta }_C$ in Equation (II).

$\begin{array}{c}{h}_8=290.16\text{kJ}/\text{kg}+\left[\frac{\text{526}\text{.12}\text{kJ}/\text{kg}-290.16\text{kJ}/\text{kg}}{0.85}\right]\\ =567.76\text{kJ}/\text{kg}\end{array}$

Refer Table A-17, “ideal gas properties of air”, and write the enthalpy at state 9 and Prandtl number at state 9 corresponding to temperature at state 9 of $\text{1400}\text{K}$.

$\begin{array}{l}{h}_9=1515.42\text{kJ}/\text{kg}\\ P_{r_9}=450.5\end{array}$

Here, enthalpy at state 9 is $h_9$ and Prandtl number at state 9 is $P_{r_9}$.

Substitute $\frac{1}{8}$ for $\frac{P_{10s}}{P_9}$ and $450.5$ for $P_{r_9}$ in Equation (III).

$\begin{array}{c}{P}_{r_{10s}}=\frac{1}{8}\left(450.5\right)\\ =56.3\end{array}$

Refer Table A-17, “ideal gas properties of air”, and write the enthalpy at state 10s corresponding to Prandtl number at state 10s of $56.3$ using an interpolation method.

Show the enthalpy at state 10s corresponding to Prandtl number as in Table (2).

Prandtl number at state 10s $P_{r_{10s}}$	Enthalpy at state 10s $h_{10s}\left(\text{kJ}/\text{kg}\right)$
52.59 $\left(x_1\right)$	843.98 $\left(y_1\right)$
56.3 $\left(x_2\right)$	$\left(y_2=?\right)$
57.60 $\left(x_3\right)$	866.08 $\left(y_3\right)$

Use excels and substitutes the values from Table (II) in Equation (XVI) to get,

$h_{10s}=\text{860}\text{.35}\text{kJ}/\text{kg}$

Here, enthalpy at state 10s is $h_{10s}$.

Substitute $\text{1515}\text{.42}\text{kJ}/\text{kg}$ for $h_9$, $0.90$ for ${\eta }_T$ and $\text{860}\text{.35}\text{kJ}/\text{kg}$ for $h_{10s}$ in Equation (IV).

$\begin{array}{c}{h}_{10}=\text{1515}\text{.42}\text{kJ}/\text{kg}-\left(0.90\right)\left(\text{1515}\text{.42}\text{kJ}/\text{kg}-\text{860}\text{.35}\text{kJ}/\text{kg}\right)\\ =925.86\text{kJ}/\text{kg}\end{array}$

Refer Table A-17, “ideal gas properties of air”, and write the enthalpy at state 11 corresponding to temperature at state 11 of $\text{520}\text{K}$.

$h_{11}=523.63\text{kJ}/\text{kg}$

Here, enthalpy at state 11 is $h_{11}$.

Refer Table A-5, “saturated water-pressure table”, and write the properties at pressure of $\text{10}\text{kPa}$.

$\begin{array}{l}{h}_{f\text{@10}\text{kPa}}=191.81\text{kJ}/\text{kg}\\ v_{f\text{@10}\text{kPa}}=0.00101{\text{m}}^{\text{3}}/\text{kg}\end{array}$

Substitute $191.81\text{kJ}/\text{kg}$ for $h_{f\text{@10}\text{kPa}}$ in Equation (V).

$h_1=191.81\text{kJ}/\text{kg}$

Substitute $0.00101{\text{m}}^{\text{3}}/\text{kg}$ for $v_{f\text{@10}\text{kPa}}$ in Equation (VI).

$v_1=0.00101{\text{m}}^{\text{3}}/\text{kg}$

Substitute $0.00101{\text{m}}^{\text{3}}/\text{kg}$ for $v_1$, $\text{15}\text{MPa}$ for $P_2$ and $\text{10}\text{kPa}$ for $P_1$ in Equation (VII).

$\begin{array}{c}{w}_{\text{pI,in}}=\left(0.00101{\text{m}}^{\text{3}}/\text{kg}\right)\left(\text{15}\text{MPa}-\text{10}\text{kPa}\right)\\ =\left(0.00101{\text{m}}^{\text{3}}/\text{kg}\right)\left(\text{15,000}\text{kPa}-\text{10}\text{kPa}\right)\frac{\text{kJ}}{\text{kPa}\cdot {\text{m}}^{\text{3}}}\\ =15.14\text{kJ}/\text{kg}\end{array}$

Substitute $\text{191}\text{.81}\text{kJ}/\text{kg}$ for $h_1$ and $15.14\text{kJ}/\text{kg}$ for $w_{\text{pI,in}}$ in Equation (VIII).

$\begin{array}{c}{h}_2=\text{191}\text{.81}\text{kJ}/\text{kg}+15.14\text{kJ}/\text{kg}\\ =206.95\text{kJ}/\text{kg}\end{array}$

Refer Table A-6, “superheated water”, and write the properties corresponding to pressure at state 3 of $\text{15}\text{MPa}$ and temperature at state 3 of $\text{450°C}$.

$\begin{array}{l}{h}_3=3157.9\text{kJ}/\text{kg}\\ s_3=6.1428\text{kJ}/\text{kg}\cdot \text{K}\end{array}$

Here, enthalpy and entropy at state 3 is $h_3\text{and}{s}_3$ respectively.

Due to throttling process, entropy at state 3 is equal to entropy at state 4s.

$\begin{array}{c}{s}_3=s_{4s}\\ =6.1428\text{kJ}/\text{kg}\cdot \text{K}\end{array}$

Refer Table A-5, “saturated water-pressure table”, and write the properties corresponding to pressure of $\text{3}\text{MPa}\left(\text{3000}\text{kPa}\right)$.

$\begin{array}{l}{s}_{f@3\text{MPa}}=2.6454\text{kJ}/\text{kg}\cdot \text{K}\\ s_{fg@3\text{MPa}}=3.5402\text{kJ}/\text{kg}\cdot \text{K}\\ h_{f@3\text{MPa}}=1008.3\text{kJ}/\text{kg}\\ h_{fg@3\text{MPa}}=1794.9\text{kJ}/\text{kg}\end{array}$

Substitute $6.1428\text{kJ}/\text{kg}\cdot \text{K}$ for $s_{4s}$, $2.6454\text{kJ}/\text{kg}\cdot \text{K}$ for $s_{f@3\text{MPa}}$ and $3.5402\text{kJ}/\text{kg}\cdot \text{K}$ for $s_{fg@3\text{MPa}}$ in Equation (IX).

$\begin{array}{c}{x}_{4s}=\frac{6.1428\text{kJ}/\text{kg}\cdot \text{K}-2.6454\text{kJ}/\text{kg}\cdot \text{K}}{3.5402\text{kJ}/\text{kg}\cdot \text{K}}\\ =0.9880\end{array}$

Substitute $0.9880$ for $x_{4s}$, $1008.3\text{kJ}/\text{kg}$ for $h_{f@3\text{MPa}}$ and $1794.9\text{kJ}/\text{kg}$ for $h_{fg@3\text{MPa}}$ in Equation (X).

$\begin{array}{c}{h}_{4s}=1008.3\text{kJ}/\text{kg}+\left(0.9880\right)\left(1794.9\text{kJ}/\text{kg}\right)\\ =2781.7\text{kJ}/\text{kg}\end{array}$

Substitute $3157.9\text{kJ}/\text{kg}$ for $h_3$, $0.90$ for ${\eta }_T$ and $2781.7\text{kJ}/\text{kg}$ for $h_{4s}$ in Equation (XI).

$\begin{array}{c}{h}_4=3157.9\text{kJ}/\text{kg}-\left(0.90\right)\left(3157.9\text{kJ}/\text{kg}-2781.7\text{kJ}/\text{kg}\right)\\ =2819.4\text{kJ}/\text{kg}\end{array}$

Refer Table A-6, “superheated water”, and write the properties corresponding to pressure at state 5 of $\text{3}\text{MPa}$ and temperature at state 5 of $\text{500°C}$.

$\begin{array}{l}{h}_5=3457.2\text{kJ}/\text{kg}\\ s_5=7.2359\text{kJ}/\text{kg}\cdot \text{K}\end{array}$

Here, enthalpy and entropy at state 5 is $h_5\text{and}{s}_5$ respectively.

Due to throttling process, entropy at state 5 is equal to entropy at state 6s.

$\begin{array}{c}{s}_5=s_{6s}\\ =7.2359\text{kJ}/\text{kg}\cdot \text{K}\end{array}$

Refer Table A-5, “saturated water-pressure table”, and write the properties corresponding to pressure of $\text{10}\text{kPa}$.

$\begin{array}{l}{s}_{f@10\text{kPa}}=0.6492\text{kJ}/\text{kg}\cdot \text{K}\\ s_{fg@10\text{kPa}}=7.4996\text{kJ}/\text{kg}\cdot \text{K}\\ h_{f@10\text{kPa}}=191.81\text{kJ}/\text{kg}\\ h_{fg@10\text{kPa}}=2392.1\text{kJ}/\text{kg}\end{array}$

Substitute $7.2359\text{kJ}/\text{kg}\cdot \text{K}$ for $s_{6s}$, $0.6492\text{kJ}/\text{kg}\cdot \text{K}$ for $s_{f@10\text{kPa}}$ and $7.4996\text{kJ}/\text{kg}\cdot \text{K}$ for $s_{fg@10\text{kPa}}$ in Equation (XII).

$\begin{array}{c}{x}_{6s}=\frac{7.2359\text{kJ}/\text{kg}\cdot \text{K}-0.6492\text{kJ}/\text{kg}\cdot \text{K}}{7.4996\text{kJ}/\text{kg}\cdot \text{K}}\\ =0.8783\end{array}$

Substitute $0.8783$ for $x_{6s}$, $191.81\text{kJ}/\text{kg}$ for $h_{f@10\text{kPa}}$ and $2392.1\text{kJ}/\text{kg}$ for $h_{fg@10\text{kPa}}$ in Equation (XIII).

$\begin{array}{c}{h}_{6s}=191.81\text{kJ}/\text{kg}+\left(0.8783\right)\left(2392.1\text{kJ}/\text{kg}\right)\\ =2292.7\text{kJ}/\text{kg}\end{array}$

Substitute $\text{3457}\text{.2}\text{kJ}/\text{kg}$ for $h_5$, $0.90$ for ${\eta }_T$ and $\text{2292}\text{.7}\text{kJ}/\text{kg}$ for $h_{6s}$ in Equation (XIV).

$\begin{array}{c}{h}_6=\text{3457}\text{.2}\text{kJ}/\text{kg}-\left(0.90\right)\left(\text{3457}\text{.2}\text{kJ}/\text{kg}-\text{2292}\text{.7}\text{kJ}/\text{kg}\right)\\ =\text{2409}\text{.1}\text{kJ}/\text{kg}\end{array}$

Substitute $\text{3157}\text{.9}\text{kJ}/\text{kg}$ for $h_3$, $\text{206}\text{.95}\text{kJ}/\text{kg}$ for $h_2$, $\text{925}\text{.862}\text{kJ}/\text{kg}$ for $h_{10}$, $\text{523}\text{.63}\text{kJ}/\text{kg}$ for $h_{11}$, and $\text{30}\text{kg}/\text{s}$ for ${\dot{m}}_s$ in Equation (V).

$\begin{array}{c}{\dot{m}}_{\text{air}}=\frac{\text{3157}\text{.9}\text{kJ}/\text{kg}-\text{206}\text{.95}\text{kJ}/\text{kg}}{\text{925}\text{.862}\text{kJ}/\text{kg}-\text{523}\text{.63}\text{kJ}/\text{kg}}\left(\text{30}\text{kg}/\text{s}\right)\\ =\text{220}\text{.1}\text{kg}/\text{s}\end{array}$

Hence, the mass flow rate of air in the gas-turbine cycle is $\text{220}\text{.1}\text{kg}/\text{s}$.

(b)

To determine

The rate of total heat input.

Answer to Problem 109RP

The rate of total heat input is $\text{227,700}\text{kW}$.

Explanation of Solution

Express the rate of total heat input.

${\dot{Q}}_{\text{in}}={\dot{m}}_{\text{air}}\left(h_9-h_8\right)+{\dot{m}}_s\left(h_5-h_4\right)$ (XVII)

Conclusion:

Substitute $\text{220}\text{.1}\text{kg}/\text{s}$ for ${\dot{m}}_{\text{air}}$, $\text{30}\text{kg}/\text{s}$ for ${\dot{m}}_s$, $\text{1515}\text{.42}\text{kJ}/\text{kg}$ for $h_9$, $\text{567}\text{.76}\text{kJ}/\text{kg}$ for $h_8$, $\text{3457}\text{.2}\text{kJ}/\text{kg}$ for $h_5$ and $\text{2819}\text{.4}\text{kJ}/\text{kg}$ for $h_4$ in Equation (XVII).

$\begin{array}{c}{\dot{Q}}_{\text{in}}=\left\{\begin{array}{c}\left(\text{220}\text{.1}\text{kg}/\text{s}\right)\left[\left(\text{1515}\text{.42}-567.76\right)\text{kJ}/\text{kg}\right]+\left(\text{30}\text{kg}/\text{s}\right)\\ \left[\left(\text{3457}\text{.2}-2819.4\right)\text{kJ}/\text{kg}\right]\end{array}\right\}\\ =227,700\text{kJ}/\text{s}\left[\frac{\text{kW}}{\text{kJ}/\text{s}}\right]\\ =227,700\text{kW}\end{array}$

Hence, the rate of total heat input is $\text{227,700}\text{kW}$.

(c)

To determine

The thermal efficiency of the combined cycle.

Answer to Problem 109RP

The thermal efficiency of the combined cycle is $\text{48}\text{.2\%}$.

Explanation of Solution

Express the rate of total heat output.

${\dot{Q}}_{\text{out}}={\dot{m}}_{\text{air}}\left(h_{11}-h_7\right)+{\dot{m}}_s\left(h_6-h_1\right)$ (XVIII)

Express the thermal efficiency of the combined cycle.

${\eta }_{\text{th}}=1-\frac{{\dot{Q}}_{\text{out}}}{{\dot{Q}}_{\text{in}}}$ (XIX)

Conclusion:

Substitute $\text{220}\text{.1}\text{kg}/\text{s}$ for ${\dot{m}}_{\text{air}}$, $\text{30}\text{kg}/\text{s}$ for ${\dot{m}}_s$, $\text{523}\text{.63}\text{kJ}/\text{kg}$ for $h_{11}$, $\text{290}\text{.16}\text{kJ}/\text{kg}$ for $h_7$, $\text{2409}\text{.1}\text{kJ}/\text{kg}$ for $h_6$ and $\text{191}\text{.81}\text{kJ}/\text{kg}$ for $h_1$ in Equation (XVIII).

$\begin{array}{c}{\dot{Q}}_{\text{out}}=\left\{\begin{array}{c}\left(\text{220}\text{.1}\text{kg}/\text{s}\right)\left[\left(\text{523}\text{.63}-290.16\right)\text{kJ}/\text{kg}\right]+\left(\text{30}\text{kg}/\text{s}\right)\\ \left[\left(\text{2409}\text{.1}-191.81\right)\text{kJ}/\text{kg}\right]\end{array}\right\}\\ =117,900\text{kJ}/\text{s}\left[\frac{\text{kW}}{\text{kJ}/\text{s}}\right]\\ =117,900\text{kW}\end{array}$

Substitute $117,900\text{kW}$ for ${\dot{Q}}_{\text{out}}$ and $227,700\text{kW}$ for ${\dot{Q}}_{\text{in}}$ in Equation (XIX).

$\begin{array}{c}{\eta }_{\text{th}}=1-\frac{117,900\text{kW}}{227,700\text{kW}}\\ =0.482\\ =48.2\%\end{array}$

Hence, the thermal efficiency of the combined cycle is $\text{48}\text{.2\%}$.