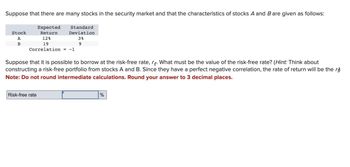
Suppose that there are many stocks in the security market and that the characteristics of stocks A and B are given as follows:StockABExpectedReturnStandardDeviation12%19Correlation = -13%9Suppose that it is possible to borrow at the risk-free rate, r. What must be the value of the risk-free rate? (Hint: Think aboutconstructing a risk-free portfolio from stocks A and B. Since they have a perfect negative correlation, the rate of return will be the rNote: Do not round intermediate calculations. Round your answer to 3 decimal places.Risk-free rate%

Explanation To find the risk-free rate, we constructed a portfolio using two stocks (A and B) with a…

Answered: Suppose that there are many stocks in…

EBK CONTEMPORARY FINANCIAL MANAGEMENT

14th Edition

ISBN:9781337514835

Author:MOYER

Publisher:MOYER

Chapter8: Analysis Of Risk And Return

Section: Chapter Questions

Problem 13QTD

See similar textbooks

Similar questions

Suppose you observe the following situation on two securities:Security Beta Expected Return Pete Corp. 0.8 0.12 Repete Corp. 1.1 0.16 Assume these two securities are correctly priced. Based on the CAPM, what is the return on the market?
Suppose that there are many stocks in the security market and that the characteristics of stocks A and B are given as follows: Stock A B Expected Return 9% 19 Correlation = -1 Standard Deviation 5% 12 Suppose that it is possible to borrow at the risk-free rate, rf. What must be the value of the risk-free rate? (Hint: Think about constructing a risk-free portfolio from stocks A and B.) (Do not round intermediate calculations. Round your answer to 3 decimal places.) Risk-free rate %
Assume that security returns are generated by the single-index model,Ri = αi + βiRM + eiwhere Ri is the excess return for security i and RM is the market’s excess return. The risk-free rate is 2%. Suppose also that there are three securities A, B, and C, characterized by the following data: Security βi E(Ri) σ(ei) A 0.7 7 % 20 % B 0.9 9 6 C 1.1 11 15 a. If σM = 16%, calculate the variance of returns of securities A, B, and C. b. Now assume that there are an infinite number of assets with return characteristics identical to those of A, B, and C, respectively. What will be the mean and variance of excess returns for securities A, B, and C? (Enter the variance answers as a percent squared and mean as a percentage. Do not round intermediate calculations. Round your answers to the nearest whole number.)
Assume that security returns are generated by the single-index model, Ri = αi + βiRM + ei where Ri is the excess return for security i and RM is the market’s excess return. The risk-free rate is 3%. Suppose also that there are three securities A, B, and C, characterized by the following data: Security βi E(Ri) σ(ei) A 1.4 14 % 23 % B 1.6 16 14 C 1.8 18 17 a. If σM = 22%, calculate the variance of returns of securities A, B, and C. b. Now assume that there are an infinite number of assets with return characteristics identical to those of A, B, and C, respectively. What will be the mean and variance of excess returns for securities A, B, and C? (Enter the variance answers as a percent squared and mean as a percentage. Do not round intermediate calculations. Round your answers to the nearest whole number.)
Suppose that there are many stocks in the security market and that the characteristics of stocks A and B are given as follows: Stock A B Expected Return 10% Standard Deviation 5% 9 18 Correlation = -1 Risk-free rate Suppose that it is possible to borrow at the risk-free rate, rf. What must be the value of the risk-free rate? (Hint: Think about constructing a risk-free portfolio from stocks A and B.) Note: Do not round intermediate calculations. Round your answer to 3 decimal places. %
1
Suppose that there are many stocks in the security market and that the characteristics of stocks A and B are given as follows: Stock Expected Return Standard Deviation A 12 % 4 % B 19 12 Correlation = –1 Suppose that it is possible to borrow at the risk-free rate, rf. What must be the value of the risk-free rate? (Hint: Think about constructing a risk-free portfolio from stocks A and B.)
Suppose that there are many stocks in the security market and that the characteristics of stocks A and B are given as follows: Stock A B Expected Return 11% 17 Correlation -1 Risk-free rate Standard Deviation 6% 9 Suppose that it is possible to borrow at the risk-free rate, r. What must be the value of the risk-free rate? (Hint: Think about constructing a risk-free portfolio from stocks A and B.) (Do not round intermediate calculations. Round your answer to 3 decimal places.) %
Is it possible to construct a portfolio of real-world stocks that has a required return equalto the risk-free rate? Explain.
Suppose that there are many stocks in the security market and that the characteristics of stocks A and B are given as follows: Expected Return 11% 17 Correlation = -1 Stock A B Standard Deviation 6% 9 Suppose that it is possible to borrow at the risk-free rate, rf. What must be the value of the risk-free rate? (Hint: Think about constructing a risk-free portfolio from stocks A and B.) Note: Do not round intermediate calculations. Round your answer to 3 decimal places. Risk-free rate
Assume that security returns are generated by the single-index model, Ri a BiRM + ei where R₁ is the excess return for security /and Ry is the market's excess return. The risk-free rate is 3%. Suppose also that there are three securities A, B, and C, characterized by the following data: Security Bi E(Ri} #{@į) A 1.5 61 298 B 1.7 8 15 C 1.9 10 24 a. If oy 26%, calculate the variance of returns of securities A, B, and C. Answer is complete but not entirely correct. Variance Security A 1,521 Security B 1,609 Security C 2,440 b. Now assume that there are an infinite number of assets with return characteristics identical to those of A, B, and C, respectively. What will be the mean and variance of excess returns for securities A, B, and C? (Enter the variance answers as a percent squared and mean as a percentage. Do not round intermediate calculations. Round your answers to the nearest whole number.)
Assume that security returns are generated by the single-index model, Ri = alphai + BetaiRM + ei where Ri is the excess return for security i and RM is the market's excess return. The risk-free rate is 2%. Suppose also that there are three securities A, B, and C, characterized by the following data. Security Betai E(Ri) sigma(ei) A 1.4 15% 28% B 1.6 17% 14% C 1.8 19% 23% a. If simaM = 24%, calculate the variance of returns of securities A, B, and C (round to whole number). Variance Security A Security B Security C b. Now assume that there are an infinite number of assets with return characteristics identical to those of A, B, and C, respectively. What will be the mean and variance of excess returns for securities A, B, and C (enter the variance answers as a whole number decimal and the mean as a whole number percentage)? Mean Variance Security A ?% Security B ?% Security C ?%

SEE MORE QUESTIONS

Recommended textbooks for you

EBK CONTEMPORARY FINANCIAL MANAGEMENT

Finance

ISBN:

9781337514835

Author:

MOYER

Publisher:

CENGAGE LEARNING - CONSIGNMENT

Intermediate Financial Management (MindTap Course…

Finance

ISBN:

9781337395083

Author:

Eugene F. Brigham, Phillip R. Daves

Publisher:

Cengage Learning

EBK CONTEMPORARY FINANCIAL MANAGEMENT

Finance

ISBN:

9781337514835

Author:

MOYER

Publisher:

CENGAGE LEARNING - CONSIGNMENT

Intermediate Financial Management (MindTap Course…

Finance

ISBN:

9781337395083

Author:

Eugene F. Brigham, Phillip R. Daves

Publisher:

Cengage Learning

SEE MORE TEXTBOOKS