
Concept explainers
a.
Show that the points
a.
Answer to Problem 53E
The points
Explanation of Solution
Given information:
Let
Given figure,
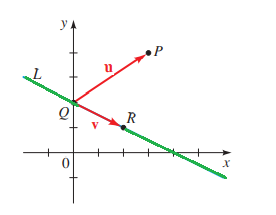
Calculation:
We have to verify that
Consider the firuge,
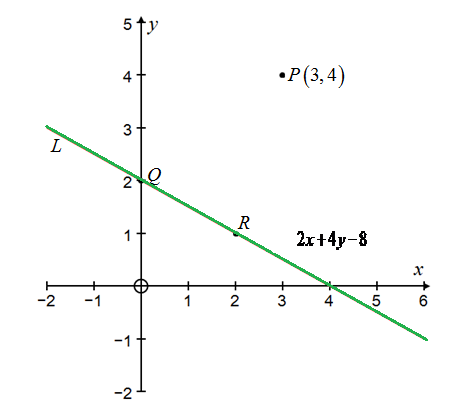
Now substitute
Now substitute
Hence, the points
b.
Find
b.
Answer to Problem 53E
Explanation of Solution
Given information:
Let
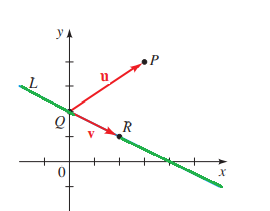
Calculation:
We have to given that
Consider the firuge,
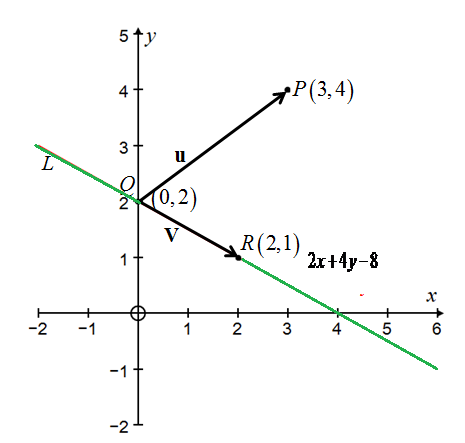
Projection of vector
Vector
Now dot product of vector
Now substitute the calculated values in equation,
Hence,
c.
Find the distance.
c.
Answer to Problem 53E
Explanation of Solution
Given information:
Let
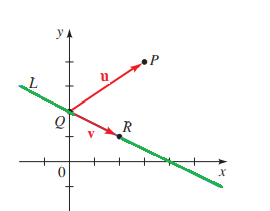
Sketch a graph that explains why
Calculation:
Consider projection vector
We have calculated
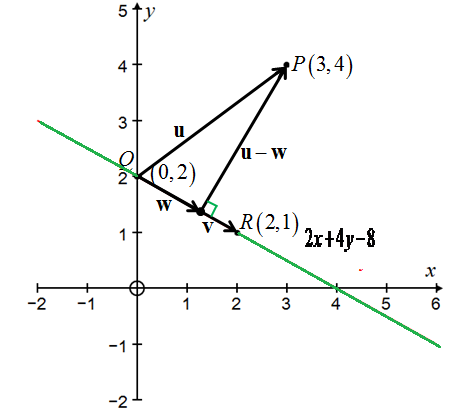
Vector
The distance
The distance the point
d.
Write a short paragraph.
d.
Answer to Problem 53E
The magnitude of this component represents the distance between the point and the line.
Explanation of Solution
Given information:
Let
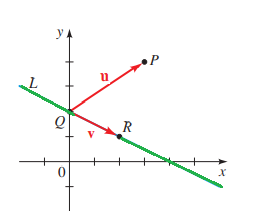
Write a short paragraph describing the steps to measure the distance from a given point to a given line.
Calculation:
The distance from a given point to a given line.
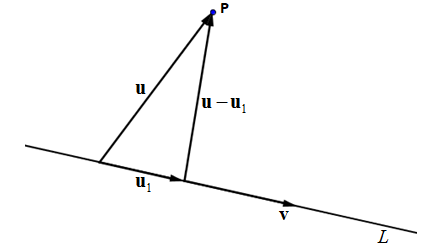
The distance between a line and point is the perpendicular distance between them. A
The component
The orthogonal component
This component is perpendicular to
Hence, the magnitude of this component represent the distance between the point and the line.
Chapter 9 Solutions
Precalculus: Mathematics for Calculus - 6th Edition
- Given lim x-4 f (x) = 1,limx-49 (x) = 10, and lim→-4 h (x) = -7 use the limit properties to find lim→-4 1 [2h (x) — h(x) + 7 f(x)] : - h(x)+7f(x) 3 O DNEarrow_forward17. Suppose we know that the graph below is the graph of a solution to dy/dt = f(t). (a) How much of the slope field can you sketch from this information? [Hint: Note that the differential equation depends only on t.] (b) What can you say about the solu- tion with y(0) = 2? (For example, can you sketch the graph of this so- lution?) y(0) = 1 y ANarrow_forward(b) Find the (instantaneous) rate of change of y at x = 5. In the previous part, we found the average rate of change for several intervals of decreasing size starting at x = 5. The instantaneous rate of change of fat x = 5 is the limit of the average rate of change over the interval [x, x + h] as h approaches 0. This is given by the derivative in the following limit. lim h→0 - f(x + h) − f(x) h The first step to find this limit is to compute f(x + h). Recall that this means replacing the input variable x with the expression x + h in the rule defining f. f(x + h) = (x + h)² - 5(x+ h) = 2xh+h2_ x² + 2xh + h² 5✔ - 5 )x - 5h Step 4 - The second step for finding the derivative of fat x is to find the difference f(x + h) − f(x). - f(x + h) f(x) = = (x² x² + 2xh + h² - ])- = 2x + h² - 5h ])x-5h) - (x² - 5x) = ]) (2x + h - 5) Macbook Proarrow_forward
- Evaluate the integral using integration by parts. Sx² cos (9x) dxarrow_forwardLet f be defined as follows. y = f(x) = x² - 5x (a) Find the average rate of change of y with respect to x in the following intervals. from x = 4 to x = 5 from x = 4 to x = 4.5 from x = 4 to x = 4.1 (b) Find the (instantaneous) rate of change of y at x = 4. Need Help? Read It Master Itarrow_forwardVelocity of a Ball Thrown into the Air The position function of an object moving along a straight line is given by s = f(t). The average velocity of the object over the time interval [a, b] is the average rate of change of f over [a, b]; its (instantaneous) velocity at t = a is the rate of change of f at a. A ball is thrown straight up with an initial velocity of 128 ft/sec, so that its height (in feet) after t sec is given by s = f(t) = 128t - 16t². (a) What is the average velocity of the ball over the following time intervals? [3,4] [3, 3.5] [3, 3.1] ft/sec ft/sec ft/sec (b) What is the instantaneous velocity at time t = 3? ft/sec (c) What is the instantaneous velocity at time t = 7? ft/sec Is the ball rising or falling at this time? O rising falling (d) When will the ball hit the ground? t = sec Need Help? Read It Watch Itarrow_forward
- Find the slope of the tangent line to the graph of the function at the given point. f(x) = -4x + 5 at (-1, 9) m Determine an equation of the tangent line. y = Need Help? Read It Watch It SUBMIT ANSWERarrow_forwardFind the slope of the tangent line to the graph of the function at the given point. f(x) = 5x-4x² at (-1, -9) m Determine an equation of the tangent line. y = Need Help? Read It Master It SUBMIT ANSWERarrow_forwardFor what value of A and B the function f(x) will be continuous everywhere for the given definition?..arrow_forward
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning





