Concept explainers
Videos
(a)
ToShow: Successive bricks must extend no more than (starting at the top)
12,14,16 and 18 Of their length beyond the one below.
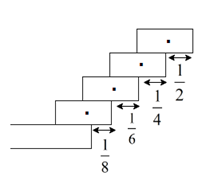
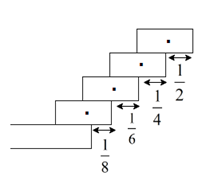
(a)
Explanation of Solution
Given data:
System is in equilibrium.
Formula used:
To remain in equilibrium, centre of mass of above system must lie just above the right edge.
For one brick over 2
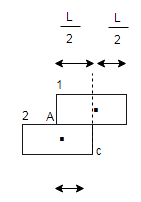
To remain in equilibrium, centre of mass of brick 1 must lie above the right edge of brick 2 or before. So, for maximum extension overhanging part must be equal to L2 .
Brick is of uniform thickness, so centre of mass is at mid-point.
For two bricks (1,2) over 3
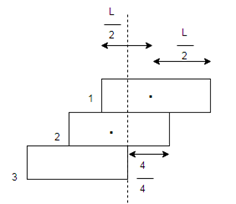
For equilibrium of brick 1 and 2, and for maximum extension,centre of mass of brick 1 and 2 must lie above the edge of 3 bricks.
xcm(1,2,3)=3L4×M+L4×M+0.M3M=L3
Distance of centre of mass of 1, 2 and 3 from the right edge of brick 3:
=L2−L3=L6
Centre of mass of brick 1,2 and 3must lie above the right edge of brick 4, so distance between right edges of brick three and 4=16
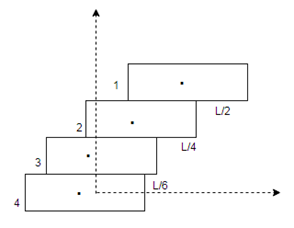
Above system remains in equilibrium when C.M of mass of bricks 1,2,3,4 lies above the right edge of table.
First find the C.M of brick 1,2,3,4
W.r.t C.M of brick 4 .
Let C.M of brick is origin.
x− Coordinate of C.M of brick 4:xcm(4)=0
x− Coordinate of C.M of brick 3:xcm(3)=L6
x− Coordinate of C.M of brick 2:xcm(2)=L6+L4
=5L12
x− Coordinate of C.M of brick 1:xcm(1)=L6+L4+L2
=1112L
With the help of previous solution, you can say that distance between C.M of brick 1 and 2 must be equal to R2 . For the center of mass of brick 1 and 2 to lie just abovethe right edge of brick 3 , centre of mass of brick 1 and 2 must be equidistance to vertical line. Hence the overhanging part of brick 2 should be equal to L4 .
For equilibrium of 1,2,3 over 4 . These three brick remain in equilibrium with maximum overhanging part only when C.M of brick 1 , brick 2 and brick 3 must be above the right edge of brick four.
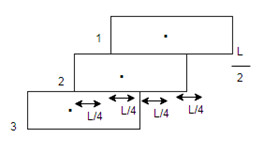
Let C.M of brick 3 as origin
xcm(1,2,3)=Mxcm(1)+Mxcm(2)+Mxcm(3)3M
xcm(1): ( x -coordinate of centre of mass of brick 1) =3L4
xcm(2): ( x -coordinate of centre of mass of brick 2) =L4
xcm(3): ( x -coordinate of centre of mass of brick 3) =0
M : Mass of each brick.
xcm(1,2,3,4)=M.xcm(1)+Mxcm(2)+Mxcm(3)+Mxcm(4)4M=(M)×(11L2)+M(5L12)+M(L6)+M(0)4M=3L8
Distance between right edges of brick 4 and xcm(1,2,3,4)
=L2−3L8=L8
Hence distance between right edge of table and right edge of 4th brick =L8
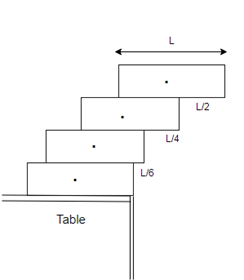
Hence hanging part of brick 1,2,3,4th are L2,L4,L6,L8 to remain in equilibrium with maximum extend.
Conclusion:
Over hanging part of brick 1,2,3,4th are L2,L4,L6,L8 , etc. to remain equilibrium and with maximum extend.
(b)
Whether the top brick is completely beyond the base.
(b)
Answer to Problem 38P
Solution:
Yes
Explanation of Solution
(c)
Ageneral formula for the maximum total distance spanned by n bricks if they are to remain stable.
(c)
Answer to Problem 38P
Solution:
The general formula of maximum total spanned by n bricks =n∑i=1(L2i) .
Explanation of Solution
Given data:
You can use previous result.
Formula used:
Find out the general term of the series with the help of previous result.
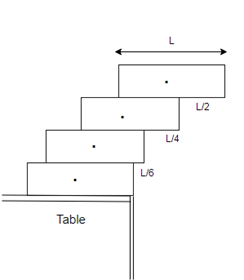
Overhanging part of 1st brick =L2=L2×1
Overhanging part of 2nd brick =L4=L2×2
Overhanging part of 3rd brick L6=L2×3
Overhanging part of 4th brick =L8=L2×4
From above it becomes clear that overhanging part of nth brick = L2n
Hence, maximum span length obtained with the help of n brick.
=L2+L2×2+L2×3+L2×4+...L2n=n∑i=1L2i
Hence, maximum span length obtained with the help of n brick
=n∑i=1(L2i)
Conclusion:
Maximum span length obtained with the help of n brick
=n∑i=1(L2i)
(d)
The minimum number of bricks each 0.30 m long and uniform is needed if the arch is to span 1.0 m .
(d)
Answer to Problem 38P
Solution:
35 bricks
Explanation of Solution
Given data: Span length =1.0 m
Length of brick =0.30 m
Formula used:
Maximum span length (overhanging part)
Obtain from n brick
=n∑i=1L2i
Calculation:
The span length of arch =1.0 m
Length of one side span length =1.0 m2=0.5 m
Let number of required bricksbe n to develop 0.5 m overhanging part.
n∑i=1L2i≥0.5L=0.30 m(given)n∑i=10.302i≥0.50n∑i=112i≥53
12+14+16+18+110+112... to n≥53
Do the sum of left side until you obtain the sum is either greater than or equal to 5/3
12+14+16+18+110+112+114+116+118+120+122+124126+128(n=14)=1.625
If n=15 15∑i=112i=1.659
If n=16 16∑i=112i=1.690=53=1.666
Form the above solution it becomes clear that
For n=15, overhanging part is less 0.5
For n=16, overhanging is just greater than 0.5
Hence, required number of bricks =2×16+(1)+(2)
Multiply 2 both sides
1 is for brick on the top and 2 is for the base of each side.
2×16+(1)+(2)
=35
Conclusion:
Minimum no of brick to developed span length 1.0 m and arch as given in figure =35 .
Chapter 9 Solutions
Physics: Principles with Applications
Additional Science Textbook Solutions
Cosmic Perspective Fundamentals
College Physics: A Strategic Approach (3rd Edition)
Campbell Biology (11th Edition)
Chemistry: An Introduction to General, Organic, and Biological Chemistry (13th Edition)
Microbiology: An Introduction
Physics for Scientists and Engineers: A Strategic Approach, Vol. 1 (Chs 1-21) (4th Edition)
- You want to fabricate a soft microfluidic chip like the one below. How would you go about fabricating this chip knowing that you are targeting a channel with a square cross-sectional profile of 200 μm by 200 μm. What materials and steps would you use and why? Disregard the process to form the inlet and outlet. Square Cross Sectionarrow_forward1. What are the key steps involved in the fabrication of a semiconductor device. 2. You are hired by a chip manufacturing company, and you are asked to prepare a silicon wafer with the pattern below. Describe the process you would use. High Aspect Ratio Trenches Undoped Si Wafer P-doped Si 3. You would like to deposit material within a high aspect ratio trench. What approach would you use and why? 4. A person is setting up a small clean room space to carry out an outreach activity to educate high school students about patterning using photolithography. They obtained a positive photoresist, a used spin coater, a high energy light lamp for exposure and ordered a plastic transparency mask with a pattern on it to reduce cost. Upon trying this set up multiple times they find that the full resist gets developed, and they are unable to transfer the pattern onto the resist. Help them troubleshoot and find out why pattern of transfer has not been successful. 5. You are given a composite…arrow_forwardTwo complex values are z1=8 + 8i, z2=15 + 7 i. z1∗ and z2∗ are the complex conjugate values. Any complex value can be expessed in the form of a+bi=reiθ. Find r and θ for (z1-z∗2)/z1+z2∗. Find r and θ for (z1−z2∗)z1z2∗ Please show all stepsarrow_forward
- An electromagnetic wave is traveling through vacuum in the positive x direction. Its electric field vector is given by E=E0sin(kx−ωt)j^,where j^ is the unit vector in the y direction. If B0 is the amplitude of the magnetic field vector, find the complete expression for the magnetic field vector B→ of the wave. What is the Poynting vector S(x,t), that is, the power per unit area associated with the electromagnetic wave described in the problem introduction? Give your answer in terms of some or all of the variables E0, B0, k, x, ω, t, and μ0. Specify the direction of the Poynting vector using the unit vectors i^, j^, and k^ as appropriate. Please explain all stepsarrow_forwardAnother worker is performing a task with an RWL of only 9 kg and is lifting 18 kg, giving him an LI of 2.0 (high risk). Questions:What is the primary issue according to NIOSH?Name two factors of the RWL that could be improved to reduce risk.If the horizontal distance is reduced from 50 cm to 30 cm, how does the HM change and what effect would it have?arrow_forwardTwo complex values are z1=8 + 8i, z2=15 + 7 i. z1∗ and z2∗ are the complex conjugate values. Any complex value can be expessed in the form of a+bi=reiθ. Find r and θ for z1z2∗. Find r and θ for z1/z2∗? Find r and θ for (z1−z2)∗/z1+z2∗. Find r and θ for (z1−z2)∗/z1z2∗ Please explain all steps, Thank youarrow_forward
- An ac series circuit consists of a voltage source of frequency 60 Hz and voltage amplitude V, a 505-Ω resistor, and a capacitor of capacitance 7.2 μF. What must be the source voltage amplitude V for the average electrical power consumed in the resistor to be 236 W? There is no inductance in the circuit.arrow_forwardAn L−R−C series circuit has R= 280 Ω . At the frequency of the source, the inductor has reactance XLL= 905 Ω and the capacitor has reactance XC= 485 Ω . The amplitude of the voltage across the inductor is 445 V . What is the amplitude of the voltage across the resistor and the capacitor? What is the voltage amplitude of the source? What is the rate at which the source is delivering electrical energy to the circuit?arrow_forwardA 0.185 H inductor is connected in series with a 98.5 Ω resistor and an ac source. The voltage across the inductor is vL=−(12.5V)sin[(476rad/s)t]vL. Derive an expression for the voltage vR across the resistor. Express your answer in terms of the variables L, R, VL (amplitude of the voltage across the inductor), ω, and t. What is vR at 2.13 ms ? Please explain all stepsarrow_forward
- A worker lifts a box under the following conditions:Horizontal distance (H): 30 cmInitial height (V): 60 cmVertical travel (D): 50 cmTorso rotation (A): 30°Frequency: 3 times/minute for 1 hourGrip: Good Question:What is the RWL for this task?What does this value mean in terms of occupational safety?arrow_forwardCan someone helparrow_forwardCan someone help mearrow_forward
College PhysicsPhysicsISBN:9781305952300Author:Raymond A. Serway, Chris VuillePublisher:Cengage Learning
University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON
Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley
College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON