Chapter 5.1, Problem 76E

To determine

To graph: The given trigonometric expression and determine which trigonometric function is equal to the expression. Then verify the resulting identity algebraically.

Explanation of Solution

Given information:

The following trigonometric expression:

  $\sin x(\cot x+\tan x)$

Graph:

Sketch the graph using graphing utility.

Step 1: Press WINDOW button to access the Window editor.

Step 2: Press $\boxed{\text{Y=}}$ button.

Step 3: Enter the expression $\sin x(\cot x+\tan x)$ which is required to graph.

Step 4: Press GRAPH button to graph the function.

The graph is obtained as:

  

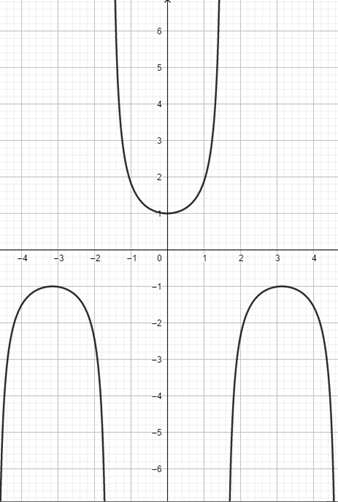
Interpretation:

According to the above graph, it can be observed that the given expression has the same graph as obtained forsecant function. Hence, $y=\sec x$

Formula used:

The following identities:

The Pythagorean identity:

  ${\sec }^{2}x=1+{\tan }^{2}x$

The Quotient identity:

  $\tan x=\frac{\sin x}{\cos x}$

The Reciprocal identities:

  $\cot x=\frac{1}{\tan x}$ and $\sec x=\frac{1}{\cos x}$

Proof:

The given trigonometric expression can be simplified as follows:

  $\begin{array}{l}y=\sin x(\cot x+\tan x)\text{ [Original expression]}\\ =\sin x(\frac{1}{\tan x}\text{+}\tan x)\text{ [Reciprocal identity] }\\ =\sin x(\frac{1\text{+}{\tan }^{2}x}{\tan x})\text{ [Add fractions]}\\ =\sin x(\frac{{\sec }^{2}x}{\tan x})\text{ [Pythagorean identity]}\\ =\frac{\sin x\cdot \frac{1}{{\cos }^{2}x}}{\frac{\sin x}{\tan x}}\text{ [Reciprocal and Quotient identity]}\\ =\frac{\sin x\cos x}{{\cos }^{2}x\sin x}\text{ [Simplify]}\\ =\frac{1}{\cos x}\text{ [Divide out common factors]}\\ =\sec x\text{ [Reciprocal identity]}\end{array}$

Therefore, $\text{y}=\sec x$ .

The simplified expression is same as the expression obtained from the graph. Hence, verified.