Chapter 4.1, Problem 5E

To determine

To Find: the absolute extreme value of the given graph, also, explain according to Extreme value theorem.

Answer to Problem 5E

Maximum at $x=b$ , minimum at $x=c_2$ .

Also, the extreme value theorem applies, so both the minimum and maximum exist.

Explanation of Solution

Given information:

The graph is:

  

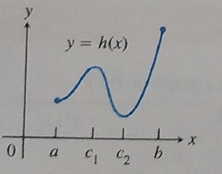
Concept Used:

The extreme values are the points where any x values minimum and maximum value of y is obtained.

Extreme value theorem: For any real valued function if it is continuous in a closed interval then it must attain a maximum and a minimum both at least once.

From the given graph it can be seen that the function is continuous in a closed interval $\left[a,b\right]$ . So, by extreme value theorem the function must attain a maximum and a minimum value.

The maximum value (value of y ) is obtained at $x=b$ and minimum at $x=c_2$ .

Conclusion:

The extreme values are:

Maximum at $x=b$ , minimum at $x=c_2$ .