Chapter 4, Problem 79P

(a)

To determine

The magnitude of contact force between the two masses.

Answer to Problem 79P

The magnitude of contact force between the two masses is $\underset{\_}{0\text{N}}$.

Explanation of Solution

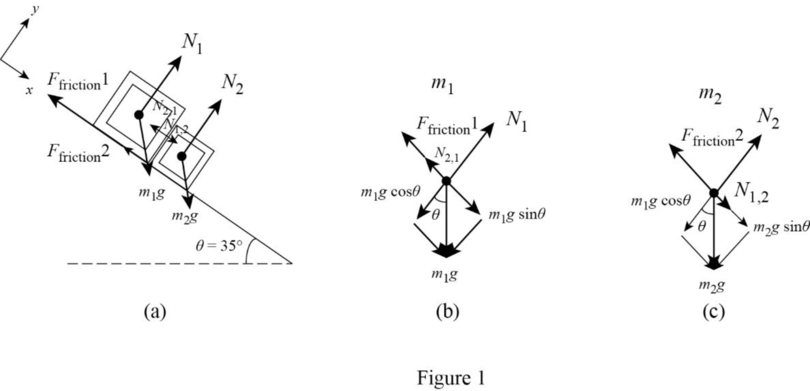
Write the expression for the Newton’s second law of motion.

    $F=ma$        (I)

Here, $F$ is the force, $m$ is the mass, $a$ is the acceleration of the object.

If the plane is frictionless, then the only force on the two masses are the normal forces and the weight with the frictional forces equalling zero. Since there is no force directed up the incline, the normal force between the two blocks must be zero.

Conclusion:

Therefore, the magnitude of contact force between the two masses is $\underset{\_}{0\text{N}}$.

(b)

To determine

The magnitude of contact force between two masses if the coefficient of kinetic friction between the masses and the plane are equal.

Answer to Problem 79P

The magnitude of contact force between two masses if the coefficient of kinetic friction between the masses and the plane are equal is $\underset{\_}{0\text{N}}$.

Explanation of Solution

Write the expression for the Newton’s law for the mass $m_1$ along horizontal direction.

    $\begin{array}{c}{\displaystyle \sum F_{x,1}}=m_{1}g\sin \theta -F_{\text{friction,1}}+N_{21}\\ =m_1{a}_{x,1}\end{array}$        (II)

Here, ${\displaystyle \sum F_{x,1}}$ is the force along the horizontal direction for mass $m_1$, $m_1$ is the mass, $g$ is the acceleration due to gravity, $N_{21}$ is the contact force between the masses, $a_x$ is the acceleration along the horizontal direction, $\theta$ is the angle of inclination.

Write the expression for the Newton’s law for the mass $m_1$ along vertical direction.

    $\begin{array}{c}{\displaystyle \sum F_{y,1}}=N_1-m_{1}g\cos \theta \\ =0\\ N_1=m_{1}g\cos \theta \end{array}$        (III)

Here, ${\displaystyle \sum F_{y,1}}$ is the force along the vertical direction for mass $m_1$.

Use equation (III) to write the expression for $F_{\text{friction,1}}$.

    $\begin{array}{c}{F}_{\text{friction,1}}={\mu }_1{N}_1\\ ={\mu }_1{m}_{1}g\cos \theta \end{array}$        (III)

Here, $F_{\text{friction,1}}$ is the frictional force of mass $m_1$, ${\mu }_1$ is the coefficient of kinetic friction.

Use equation (III) in (II) to solve for $a_{x,1}$.

    $\begin{array}{c}{m}_{1}g\sin \theta -{\mu }_1{m}_{1}g\sin \theta +N_{21}=m_1{a}_{x,1}\\ g\sin \theta -{\mu }_{1}g\cos \theta +\frac{N_{21}}{m_1}=a_{x,1}\end{array}$        (IV)

Write the expression for the Newton’s law for the mass $m_2$ along horizontal direction.

    $\begin{array}{c}{\displaystyle \sum F_{x,2}}=m_{2}g\sin \theta -F_{\text{friction,2}}-N_{12}\\ =m_2{a}_{x,2}\end{array}$        (V)

Here, ${\displaystyle \sum F_{x,2}}$ is the force along the horizontal direction for mass $m_2$, $m_2$ is the mass, $g$ is the acceleration due to gravity, $N_{12}$ is the contact force between the masses, $a_x$ is the acceleration along the horizontal direction, $\theta$ is the angle of inclination.

Write the expression for the Newton’s law for the mass $m_2$ along vertical direction.

    $\begin{array}{c}{\displaystyle \sum F_{y,2}}=N_2-m_{2}g\cos \theta \\ =0\\ N_2=m_{2}g\cos \theta \end{array}$        (VI)

Here, ${\displaystyle \sum F_{y,2}}$ is the force along the vertical direction for mass $m_1$.

Use equation (VI) to write the expression for $F_{\text{friction,2}}$.

    $\begin{array}{c}{F}_{\text{friction,2}}={\mu }_2{N}_2\\ ={\mu }_2{m}_{2}g\cos \theta \end{array}$        (VII)

Here, $F_{\text{friction,2}}$ is the frictional force of mass $m_2$, ${\mu }_2$ is the coefficient of kinetic friction.

Use equation (VII) in (V) to solve for $a_{x,2}$.

    $\begin{array}{c}{m}_{2}g\sin \theta -{\mu }_2{m}_{2}g\sin \theta -N_{12}=m_2{a}_{x,2}\\ g\sin \theta -{\mu }_{2}g\cos \theta -\frac{N_{12}}{m_2}=a_{x,2}\end{array}$        (VIII)

Write the expression for the Newton’s third law of motion.

    $\begin{array}{c}{N}_{12}=-N_{21}\\ \left|N_{12}\right|=\left|N_{21}\right|\end{array}$        (IX)

If the normal force is non-zero, then the acceleration must be equal.

    $a_{x,1}=a_{x,2}$        (X)

Use equation (VIII) and (IV) in (X) and it becomes,

    $g\sin \theta -{\mu }_{1}g\cos \theta +\frac{N_{21}}{m_1}=g\sin \theta -{\mu }_{2}g\cos \theta -\frac{N_{12}}{m_2}$        (XI)

The coefficient of kinetic friction between the masses and the plane are equal.

    ${\mu }_1={\mu }_2$        (XII)

Use equation (XII) in (XI) to solve for the contact forces between the two masses.

    $\frac{N_{21}}{m_1}=\frac{N_{12}}{m_2}$        (XIII)

The only way this can be true with $N_{12}=-N_{21}$ and the contact force between the two blocks is zero.

Conclusion:

Therefore, the magnitude of contact force between two masses if the coefficient of kinetic friction between the masses and the plane are equal is $\underset{\_}{0\text{N}}$.

(c)

To determine

If ${\mu }_1=0.15$ and ${\mu }_2=0.25$, the magnitude of contact force between the two blocks.

Answer to Problem 79P

The magnitude of contact force between the two blocks is $\underset{\_}{N_{12}=4.5\text{N}}$.

Explanation of Solution

Use equation (IX) and (XI) to solve for $N_{12}$

    $\begin{array}{c}-{\mu }_{1}g\cos \theta +\frac{N_{21}}{m_1}=-{\mu }_{2}g\cos \theta -\frac{N_{12}}{m_2}\\ \frac{N_{12}}{m_1}+\frac{N_{12}}{m_2}={\mu }_{1}g\cos \theta -{\mu }_{2}g\cos \theta \\ N_{12}\left(\frac{1}{m_2}+\frac{1}{m_1}\right)=\left({\mu }_1-{\mu }_2\right)g\cos \theta \\ N_{12}=\frac{\left({\mu }_1-{\mu }_2\right)g\cos \theta }{\left(\frac{1}{m_2}+\frac{1}{m_1}\right)}\end{array}$        (XIV)

Conclusion:

Substitute ${\mu }_1=0.15$, ${\mu }_2$ for $0.25$, $9.8\text{m}/{\text{s}}^2$ for $g$, $35°$ for $\theta$, $9\text{kg}$ for $m_2$, $15\text{kg}$ for $m_1$ in equation (XIV) to solve for $N_{12}$.

    $\begin{array}{c}{N}_{12}=\frac{\left(0.15-0.25\right)\left(9.8\text{m}/{\text{s}}^2\right)\cos 35°}{\frac{1}{15\text{kg}}+\frac{1}{9\text{kg}}}\\ =4.5\text{N}\end{array}$

Therefore, the magnitude of contact force between the two blocks is $\underset{\_}{N_{12}=4.5\text{N}}$.