Fox and McDonald's Introduction to Fluid Mechanics
9th Edition
ISBN: 9781118912652
Author: Philip J. Pritchard, John W. Mitchell
Publisher: WILEY
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 2, Problem 6P
When an incompressible, nonviscous fluid flows against a plate in a plane (two-dimensional) flow, an exact solution for the equations of motion for this flow is u = Ax, υ = −Ay, with A > 0 for the sketch shown. The coordinate origin is located at the stagnation point 0, where the flow divides and the local velocity is zero. Plot the streamlines in the flow.
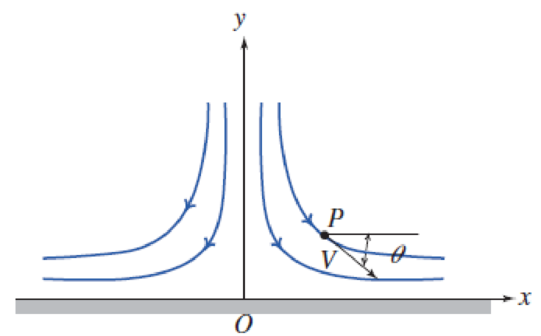
P2.6
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
3.7. In Fig. 3.4b is shown the unsteady-state streamlines of a cylinder moving
with a uniform velocity U in the fluid at rest. Calculate and draw the un-
steady-state streamlines at an interval of 10° (0 = 10°). The tangential com-
ponent of velocity on the surface of the cylinder in a uniform stream, U.,
is given by r = -2U sin 0.
2. Consider a stream function given by = (²+x²).
(a) Does this flow satisfy conservation of mass? Show your work.
(b) Plot the streamlines for this flow. Let K= 2. Be sure to indicate the direction of the flow.
(c) Is this flow irrotational? If so, find the velocity potential for this flow. If not, show that a
velocity potential does not exist.
(d) Describe the flow represented by this stream function.
The velocity components of a flow field are given by:
= 2x² – xy + z²,
v = x² – 4xy + y²,
w = 2xy – yz + y²
(i) Prove that it is a case of possible steady incompressible fluid flow
(ii) Calculate the velocity and acceleration at the point (2,1,3)
Chapter 2 Solutions
Fox and McDonald's Introduction to Fluid Mechanics
Ch. 2 - For the velocity fields given below, determine:...Ch. 2 - For the velocity fields given below, determine:...Ch. 2 - A viscous liquid is sheared between two parallel...Ch. 2 - For the velocity field V=Ax2yi+Bxy2j, where A = 2...Ch. 2 - A fluid flow has the following velocity...Ch. 2 - When an incompressible, nonviscous fluid flows...Ch. 2 - For the free vortex flow the velocities are t =...Ch. 2 - For the forced vortex flow the velocities are t =...Ch. 2 - A velocity field is specified as V=axyi+by2j,...Ch. 2 - A velocity field is given by V=ax3i+bxy3j, where a...
Ch. 2 - The velocity for a steady, incompressible flow in...Ch. 2 - The flow field for an atmospheric flow is given by...Ch. 2 - For the velocity field V=AxiAyj,, where A = 2s 1....Ch. 2 - A velocity field in polar coordinates is given...Ch. 2 - The flow of air near the Earths surface is...Ch. 2 - A velocity field is given by V=aytibxj, where a =...Ch. 2 - Air flows downward toward an infinitely wide...Ch. 2 - Consider the flow described by the velocity field...Ch. 2 - Consider the velocity field V = axi + by(1 + ct)...Ch. 2 - Consider the flow field given in Eulerian...Ch. 2 - A velocity field is given by V=axti+byj, where A =...Ch. 2 - Consider the garden hose of Fig. 2.5. Suppose the...Ch. 2 - Consider the velocity field of Problem 2.18. Plot...Ch. 2 - Streaklines are traced out by neutrally buoyant...Ch. 2 - Consider the flow field V=axti+bj, where a = 1/s2...Ch. 2 - A flow is described by velocity field V=ay2i+bj,...Ch. 2 - Tiny hydrogen bubbles are being used as tracers to...Ch. 2 - A flow is described by velocity field V=ai+bxj,...Ch. 2 - A flow is described by velocity field V=ayi+btj,...Ch. 2 - A flow is described by velocity field V=ati+bj,...Ch. 2 - The variation with temperature of the viscosity of...Ch. 2 - The variation with temperature of the viscosity of...Ch. 2 - Some experimental data for the viscosity of helium...Ch. 2 - The velocity distribution for laminar flow between...Ch. 2 - What is the ratio between the viscosities of air...Ch. 2 - Calculate velocity gradients and shear stress for...Ch. 2 - A very large thin plate is centered in a gap of...Ch. 2 - A female freestyle ice skater, weighing 100 lbf,...Ch. 2 - A block of mass 10 kg and measuring 250 mm on each...Ch. 2 - A 73-mm-diameter aluminum (SG = 2.64) piston of...Ch. 2 - A vertical gap 25 mm wide of infinite extent...Ch. 2 - A cylinder 8 in. in diameter and 3 ft long is...Ch. 2 - Crude oil at 20C fills the space between two...Ch. 2 - The piston in Problem 2.40 is traveling at...Ch. 2 - A block of mass M slides on a thin film of oil....Ch. 2 - A block 0.1 m square, with 5 kg mass, slides down...Ch. 2 - A torque of 4 N m is required to rotate the...Ch. 2 - A circular disk of diameter d is slowly rotated in...Ch. 2 - The fluid drive shown transmits a torque T for...Ch. 2 - A block that is a mm square slides across a flat...Ch. 2 - In a food-processing plant, honey is pumped...Ch. 2 - SAE 10W-30 oil at 100C is pumped through a tube L...Ch. 2 - The lubricant has a kinematic viscosity of 2:8105...Ch. 2 - Calculate the approximate viscosity of the oil....Ch. 2 - Calculate the approximate power lost in friction...Ch. 2 - Fluids of viscosities 1 = 0.1 Ns/m2 and 2 = 0.15...Ch. 2 - A concentric cylinder viscometer may be formed by...Ch. 2 - A concentric cylinder viscometer is driven by a...Ch. 2 - A shaft with outside diameter of 18 mm turns at 20...Ch. 2 - A shock-free coupling for a low-power mechanical...Ch. 2 - A proposal has been made to use a pair of parallel...Ch. 2 - The cone and plate viscometer shown is an...Ch. 2 - A viscometer is used to measure the viscosity of a...Ch. 2 - A concentric-cylinder viscometer is shown. Viscous...Ch. 2 - Design a concentric-cylinder viscometer to measure...Ch. 2 - A cross section of a rotating bearing is shown....Ch. 2 - Small gas bubbles form in soda when a bottle or...Ch. 2 - You intend to gently place several steel needles...Ch. 2 - According to Folsom [6], the capillary rise h...Ch. 2 - Calculate and plot the maximum capillary rise of...Ch. 2 - Calculate the maximum capillary rise of water...Ch. 2 - Calculate the maximum capillary depression of...Ch. 2 - Water usually is assumed to be incompressible when...Ch. 2 - The viscous boundary layer velocity profile shown...Ch. 2 - In a food industry process, carbon tetrachloride...Ch. 2 - What is the Reynolds number of water at 20C...Ch. 2 - A supersonic aircraft travels at 2700 km/hr at an...Ch. 2 - SAE 30 oil at 100C flows through a 12-mm-diameter...Ch. 2 - A seaplane is flying at 100 mph through air at...Ch. 2 - An airliner is cruising at an altitude of 5.5 km...
Additional Engineering Textbook Solutions
Find more solutions based on key concepts
Test Scores #1 Write a program that dynamically allocates an array large enough to hold a user-defined number o...
Starting Out with C++ from Control Structures to Objects (9th Edition)
Describe the purpose of the access key attribute and how it supports accessibility.
Web Development and Design Foundations with HTML5 (8th Edition)
What are the two basic types of stationary spot-welding machines?
Degarmo's Materials And Processes In Manufacturing
What is a condition-controlled loop?
Starting Out with Programming Logic and Design (5th Edition) (What's New in Computer Science)
In Exercises 39 through 44, write a program to carry out the stated task. Cost of Electricity The cost of the e...
Introduction To Programming Using Visual Basic (11th Edition)
Create a VB application that lets the user enter his or her weight (in pounds) and height (in inches). If the u...
Starting Out With Visual Basic (8th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- The figure below shows 4 streamlines corresponding to a free vortex flow. The streamlines are concentric eircles, and the velocity magnitude Valong each streamline is given by V = K/r, where r is the radial distance from the center, and K is a constant. Assume the flow to be steady, incompressible and inviscid. Neglect the effects of gravity. If K = 20 m2/s and the fluid density is 1000 kg/m, what is the magnitude of the static pressure difference AP between 2 streamlines at radial distances r = 3 m and r = 4 m? Ap = 1 КРа. C r Вarrow_forwarda) A Newtonian fluid flows with external force in the y-direction in space between two stationary parallel plates that are separated by a distance 2D as shown in Figure 1. The length and the width of each plate are L and W, respectively. 2D Center Line Figure 1 Flow between parallel plates Derive the velocity profile for system using shell momentum balance. State all your postulate and assumptions.arrow_forwardQ.2 A flow is described by the stream function v = 25xv, The coordinates of the point at which velocity vector has a magnitude of 4 units and makes an angle 150 ° with the X-axis is A x=1.0, y=0.5774 B X=0.5774, Y=1.0 WRONG C X=1, Y=-0.5774 D X=-1, Y=0.5774arrow_forward
- 3.4 Consider a steady, incompressible, 2D velocity field for motion parallel to the X-axis with constant shear. The shear rate is du/dy Ay. Obtain an expression for the velocity field V. Calculate the rate of rotation. Evaluate the stream function %3D for this flow field. Ay Ay + В і, о, Ay + By+ C 6. Ans: V= 2arrow_forward1. For incompressible flows, their velocity field 2. In the case of axisymmetric 2D incompressible flows, where is Stokes' stream function, and u = VXS, S(r, z, t) = Uz = where {r, y, z} are the cylindrical coordinates in which the flow is independent on the coordinate and hence 1 Ꭷ r dr 1 dy r dz Show that in spherical coordinates {R, 0, 0} with the same z axis, this result reads Y(R, 0, t) R sin 0 S(R, 0, t) UR uo Y(r, z, t) r = = -eq, and Up = = 1 ay R2 sin Ꮎ ᎧᎾ 1 ƏY R sin Ꮎ ᎧR -eq 2 (1) (2) (3)arrow_forwardFlow through a converging nozzle can be approximated by a one-dimensional velocity distribution u=vo (1+2). For the nozzle shown below, assume that the velocity varies linearly from u = vo at the entrance to u = 3v, at the exit. Compute the acceleration at the entrance and exit if vo=10m/s and L = 1m. x=0 X u= :326 x=Larrow_forward
- Q.5 The velocity components in x and y direction 2 are given by u = Axy° - xy; v = > ху; v — ху = xy² – 3/4 .4 y*. The value of A for a possible flow field involving an incompressible fluid is: A -3/4 В 3 C 4/3 D -4/3arrow_forwardCourse: Fluid Mechanicsarrow_forwardPlease don't provide handwritten solution.arrow_forward
- 3.14. Find the vorticity in polar coordinates for the following velocity com- ponents (a) v=rsin 0, ve = 2r cos 0 (b) v = cos 0, 11=0arrow_forward3. The velocity components of a two-dimensional incompressible fluid flow are prescribed as v=-3x²² u=-1 i) Determine the stream function. ii) Using excel plot the stream function for y=-5 and y=-20 from x = 0 to x = 5.arrow_forwardSolve correctly please.arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Elements Of ElectromagneticsMechanical EngineeringISBN:9780190698614Author:Sadiku, Matthew N. O.Publisher:Oxford University Press
Mechanics of Materials (10th Edition)Mechanical EngineeringISBN:9780134319650Author:Russell C. HibbelerPublisher:PEARSON
Thermodynamics: An Engineering ApproachMechanical EngineeringISBN:9781259822674Author:Yunus A. Cengel Dr., Michael A. BolesPublisher:McGraw-Hill Education
Control Systems EngineeringMechanical EngineeringISBN:9781118170519Author:Norman S. NisePublisher:WILEY
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Engineering Mechanics: StaticsMechanical EngineeringISBN:9781118807330Author:James L. Meriam, L. G. Kraige, J. N. BoltonPublisher:WILEY
Elements Of Electromagnetics
Mechanical Engineering
ISBN:9780190698614
Author:Sadiku, Matthew N. O.
Publisher:Oxford University Press
Mechanics of Materials (10th Edition)
Mechanical Engineering
ISBN:9780134319650
Author:Russell C. Hibbeler
Publisher:PEARSON
Thermodynamics: An Engineering Approach
Mechanical Engineering
ISBN:9781259822674
Author:Yunus A. Cengel Dr., Michael A. Boles
Publisher:McGraw-Hill Education
Control Systems Engineering
Mechanical Engineering
ISBN:9781118170519
Author:Norman S. Nise
Publisher:WILEY
Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning
Engineering Mechanics: Statics
Mechanical Engineering
ISBN:9781118807330
Author:James L. Meriam, L. G. Kraige, J. N. Bolton
Publisher:WILEY
Introduction to Kinematics; Author: LearnChemE;https://www.youtube.com/watch?v=bV0XPz-mg2s;License: Standard youtube license