Statistics for Business and Economics (13th Edition)
13th Edition
ISBN: 9780134506593
Author: James T. McClave, P. George Benson, Terry Sincich
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 14.9, Problem 14.57ACI
Modeling the deposit share of a retail bank. Exploratory research published in the Journal of Professional Services Marketing (Vol. 5, 1990) examined the relationship between deposit share of a retail bank and several marketing variables. Quarterly deposit share data were collected for 5 consecutive years for each of nine retail banking institutions. The model analyzed took the following form:
E(Yt) = β0 + β1Pt−1 + β2St−1 + β3Dt−1
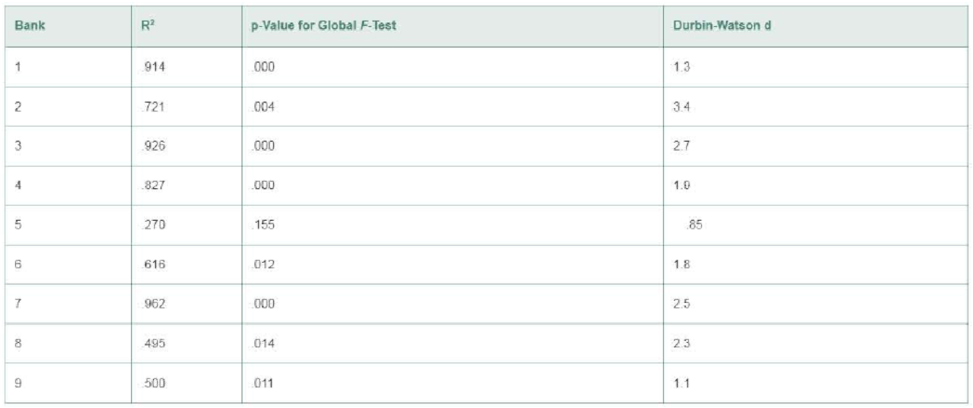
where Yt = deposit share of a bank In quarter t (t = 1, 2, ... , 20), Pt−1 = expenditures on promotion-related activities in quarter t – 1, St−1 = expenditures on service- related activities in quarter t − 1, and Dt−1 = expenditures on distribution-related activities in quarter t – 1. A separate model was fit for each bank with the results shown in the table.
a. Interpret the values of R2 for each bank.
b. Test the overall adequacy of the model for each bank using α = .01.
c. Conduct the Durbin-Watson d-test for
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
A study was conducted to see if a person's income will affect their well-being. We want to create a model with Y = well-being index, and X =
income in $1,000. The regression model is:
y = beta0 + beta1*x
%D
y = beta0 + beta1*x + error
%3D
y_hat = beta0_hat + beta1_hat*x + error
O y_hat = beta0_hat + beta1_hat*x
An experiment is conducted to see the effect of light intensity on plant growth, what is the dependent variable in this scenario?
A researcher conducted a study about the hemoglobin levels among menopausal and non-menopausal women. With study of 20 women total, the following information was collected: Hemoglobin Level (g/dl), Age, and Menopausal Status (yes/no). The researcher conducted a multiple logistic regression analysis to find the effects of hemoglobin level, and age on menopausal status. The summary of the analysis is attached as photo.
Please help me determine the predicted probability of being menopausal for a 53-year-old woman whose hemoglobin level is 15.
Chapter 14 Solutions
Statistics for Business and Economics (13th Edition)
Ch. 14.1 - Explain in words how to construct a simple index.Ch. 14.1 - Explain in words how to calculate the following...Ch. 14.1 - Explain in words the difference between Laspeyres...Ch. 14.1 - The table below gives the prices for three...Ch. 14.1 - Refer to Exercise 14.4. The next table gives the...Ch. 14.1 - Annual median family income. The table below lists...Ch. 14.1 - Annual U.S. craft beer production. While overall...Ch. 14.1 - Quarterly single-family housing starts. The...Ch. 14.1 - Spot price of natural gas. The table shown in the...Ch. 14.1 - Employment in farm and nonfarm categories....
Ch. 14.1 - GOP personal consumption expenditures. The gross...Ch. 14.1 - GDP personal consumption expenditures (contd)....Ch. 14.1 - Weekly earnings for workers. The table in the next...Ch. 14.1 - Production and price of metals. The level or price...Ch. 14.2 - Describe the effect of selecting an exponential...Ch. 14.2 - A monthly time series is shown in the table to the...Ch. 14.2 - Annual U.S. craft beer production. Refer to the...Ch. 14.2 - Foreign fish production. Overfishing and pollution...Ch. 14.2 - Yearly price of gold. The price of gold is used by...Ch. 14.2 - Personal consumption in transportation. There has...Ch. 14.2 - OPEC crude oil imports. The data in the table...Ch. 14.2 - SP 500 Stock Index. Standard Poors 500 Composite...Ch. 14.5 - How does the choice of the smoothing constant w...Ch. 14.5 - Refer to Exercise 14.4 (p. 14-9). The table with...Ch. 14.5 - Annual U.S. craft beer production. Refer to...Ch. 14.5 - Quarterly single-family housing starts. Refer to...Ch. 14.5 - Consumer Price Index. The CPI measures the...Ch. 14.5 - OPEC crude oil imports. Refer to the annual OPEC...Ch. 14.5 - SP 500 Stock Index. Refer to the quarterly...Ch. 14.5 - SP 500 Stock Index (contd). Refer to Exercise...Ch. 14.5 - Monthly gold prices. The fluctuation of gold...Ch. 14.6 - Annual U.S. craft beer production. Refer to the...Ch. 14.6 - Annual U.S. craft beer production (contd). Refer...Ch. 14.6 - SP 500 Stock Index. Refer to your exponential...Ch. 14.6 - SP 500 Stock Index (contd). Refer to your Holt...Ch. 14.6 - Monthly gold prices. Refer to the monthly gold...Ch. 14.6 - US school enrollments. The next table reports...Ch. 14.8 - The annual price of a finished product (in cents...Ch. 14.8 - Retail sales in Quarters 14 over a 10-year period...Ch. 14.8 - What advantage do regression forecasts have over...Ch. 14.8 - Mortgage interest rates. The level at which...Ch. 14.8 - Price of natural gas. Refer to Exercise 14.9 (p....Ch. 14.8 - A gasoline tax on carbon emissions. In an effort...Ch. 14.8 - Predicting presidential elections. Researchers at...Ch. 14.8 - Life insurance policies in force. The table below...Ch. 14.8 - Graphing calculator sales. The next table presents...Ch. 14.8 - Prob. 14.47ACICh. 14.9 - Define autocorrelation. Explain why it is...Ch. 14.9 - For each case, indicate the decision regarding the...Ch. 14.9 - What do the following Durbin-Watson statistics...Ch. 14.9 - Company donations to charity. Refer to the Journal...Ch. 14.9 - Forecasting monthly car and truck sales. Forecasts...Ch. 14.9 - Predicting presidential elections. Refer to the...Ch. 14.9 - Mortgage interest rates. Refer to the data on...Ch. 14.9 - Price of natural gas. Refer to the annual data on...Ch. 14.9 - Life insurance policies in force. Refer to the...Ch. 14.9 - Modeling the deposit share of a retail bank....Ch. 14 - Insured Social Security workers. Workers insured...Ch. 14 - Insured Social Security workers (contd). Refer to...Ch. 14 - Retail prices of food items. In 1990, the average...Ch. 14 - Demand for emergency room services. With the...Ch. 14 - Mortgage interest rates. Refer to the annual...Ch. 14 - Price of Abbott Labs stock. The yearly closing...Ch. 14 - Price o f Abbott Labs stock (contd). Refer to...Ch. 14 - Prob. 14.65ACICh. 14 - Prob. 14.66ACICh. 14 - Quarterly GOP values (contd). Refer to Exercise...Ch. 14 - Prob. 14.68ACICh. 14 - Prob. 14.69ACICh. 14 - Prob. 14.70ACICh. 14 - IBM stock prices. Refer to Example 14.1 (p. 14-5)...Ch. 14 - Prob. 14.72ACI
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, statistics and related others by exploring similar questions and additional content below.Similar questions
- We have data on Lung Capacity of persons and we wish to build a multiple linear regression model that predicts Lung Capacity based on the predictors Age and Smoking Status. Age is a numeric variable whereas Smoke is a categorical variable (0 if non-smoker, 1 if smoker). Here is the partial result from STATISTICA. b* Std.Err. of b* Std.Err. N=725 of b Intercept Age Smoke 0.835543 -0.075120 1.085725 0.555396 0.182989 0.014378 0.021631 0.021631 -0.648588 0.186761 Which of the following statements is absolutely false? A. The expected lung capacity of a smoker is expected to be 0.648588 lower than that of a non-smoker. B. The predictor variables Age and Smoker both contribute significantly to the model. C. For every one year that a person gets older, the lung capacity is expected to increase by 0.555396 units, holding smoker status constant. D. For every one unit increase in smoker status, lung capacity is expected to decrease by 0.648588 units, holding age constant.arrow_forwardIn a simple linear regression model, the correlation coefficient between x and y is 0.8. What can you say about the strength and direction of the relationship between x and y?arrow_forwardA study was conducted to see if a person's income will affect their well-being. We want to create a model with Y = well-being index, and X = income in $1,000. The dependent variable in this study is: Income in $1,000 Well-being index Neitherarrow_forward
- Look at the following regression table where the dependent variable is the demand for illegal massage services in a city in the United States. Specifically, the dependent variable is the number of customers per hour (Models 1 and 2) or per day (Models 3 and 4). (a) Explain why the coefficient for Population/1,000 in Model 2 is very different from the one in Model 4?(b) Can you reject H0 in Model 1 if H0 : βP opulation/1,000 = 0.01, H1 : βPopulation/1,000 6= 0.01, and α = 0.01?arrow_forwardWhy would the male lifespan not be the dependent variable?arrow_forwardAssume we have data demonstrating a strong linear link between the amount of fertilizer applied to certain plants and their yield. Which is the independent variable in this research question?arrow_forward
- I set up a multiple linear regression model to explain returns on BA as a linear function of market returns, and size and value. Note that the data is in levels ( not in logs) I interpret the beta of market as Select one: a. the impact of a one unit variation of market on BA returns on average b. the impact of a one unit % chnage in market on the BA returns on average keeping size and value fixed c. the relation between BA returns and market d. the impact of a one percentage variation of market on BA returns on average in percentage e. the impact of a one unit variation in market on the BA returns on average keeping size and value fixedarrow_forwardA regression was run to determine if there is a relationship between the happiness index (y) and life expectancy in years of a given country (x). The results of the regression were: ý=a+bx a=0.137 b=0.082 (a) Write the equation of the Least Squares Regression line of the form (b) Which is a possible value for the correlation coefficient, r? O-1.338 O-0.84 O 1.338 O 0.84 (c) If a country increases its life expectancy, the happiness index will O increase O decreasearrow_forwardIn the simple linear regression model, if there is a very strong correlation between the independent and dependent variables, then the correlation coefficient should be a) close to either -1 or +1 b) close to zero c) close to -1 d) close to +1 ( don't hand writing solution)arrow_forward
- A major brokerage company has an office in Miami, Florida. The manager of the office is evaluated based on the number of new clients generated each quarter. Data were collected that show the number of new customers added during each quarter between 2015 and 2018. A multiple regression model was developed with the number of new customers as the dependent and the following four independent variables: Period (1, …, 16): A variable that measures the trend; Q1 = 1 for first quarter, Q1 = 0 otherwise; Q2 = 1 for second quarter, Q2 = 0 otherwise; Q3 = 1 for third quarter, Q3 = 0 otherwise. Questions: 1. Explain each of the four slopes (Period, Q1, Q2, Q3). 2. How many new customers would you expect in the second quarter of the following year (2019)?arrow_forwardCaterpillar, Inc., manufactures and sells heavy construction equipment worldwide. The performance of Caterpillar’s stock is likely to be strongly influenced by the economy. For example, during the Great Recession, the value of Caterpillar’s stock plunged dramatically. Monthly data for Caterpillar’s risk-adjusted return (R − Rf) and the risk-adjusted market return (RM − Rf) are collected for a five-year period (n = 60). A portion of the data is shown in the accompanying table. (You may find it useful to reference the t table.) Month Year R_Rf RM_Rf Jan 2012 0.0510 0.0403 Feb 2012 -0.0682 0.0304 Mar 2012 -0.0360 -0.0083 Apr 2012 -0.1446 -0.0635 May 2012 -0.0318 0.0387 Jun 2012 -0.0091 0.0117 Jul 2012 0.0188 0.0188 Aug 2012 0.0073 0.0232 Sept 2012 -0.0154 -0.0209 Oct 2012 0.0101 0.0018 Nov 2012 0.0504 0.0062 Dec 2012 0.1036 0.0497 Jan 2013 -0.0619 0.0104 Feb 2013 -0.0595 0.0350 Mar 2013 -0.0273 0.0172 Apr 2013 0.0193 0.0202 May 2013 -0.0390 -0.0154…arrow_forwardDistress in EMSworkers. The Journal consulting and Clinical Psychology reported on a study of emergency service (EMS) rescue workers who responded to the I-880 freeway collapse during a San Francisco earthquake. The goal of the study was to identify the predictors of symptomatic distress in the EMS workers. One of the distress variables studied was the Global Symptom Index (GSI). Several models for GSI, y, were considered based on the following independent variables: x1 = Critical Incident Exposure scale (CIE) x2 = Hogan Personality Inventory—Adjustment scale (HPI-A) x3 = Years of experience (EXP) x4 = Locus of Control scale (LOC) x5 = Social Support scale (SS) x6 = Dissociative Experiences scale (DES) x7 = Peritraumatic Dissociation Experiences Questionnaire, self-report (PDEQ-SR) (a) Write a first-order model for E(y) as a function of the first five independent variables, x1 –x5. (b) The model of part a, fitted to data collected for n = 147 EMS workers, yielded the following results:…arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
Big Ideas Math A Bridge To Success Algebra 1: Stu...
Algebra
ISBN:9781680331141
Author:HOUGHTON MIFFLIN HARCOURT
Publisher:Houghton Mifflin Harcourt
Time Series Analysis Theory & Uni-variate Forecasting Techniques; Author: Analytics University;https://www.youtube.com/watch?v=_X5q9FYLGxM;License: Standard YouTube License, CC-BY
Operations management 101: Time-series, forecasting introduction; Author: Brandoz Foltz;https://www.youtube.com/watch?v=EaqZP36ool8;License: Standard YouTube License, CC-BY