
In a laboratory experiment, an electron with a kinetic energy of 50.5 keV is shot toward another electron initially at rest (Fig. P11.50). (1 eV = 1.602 × 10−19 J) The collision is elastic. The initially moving electron is deflected by the collision.
- a. Is it possible for the initially stationary electron to remain at rest after the collision? Explain.
- b. The initially moving electron is detected at an angle of 40.0° from its original path. What is the speed of each electron after the collision?
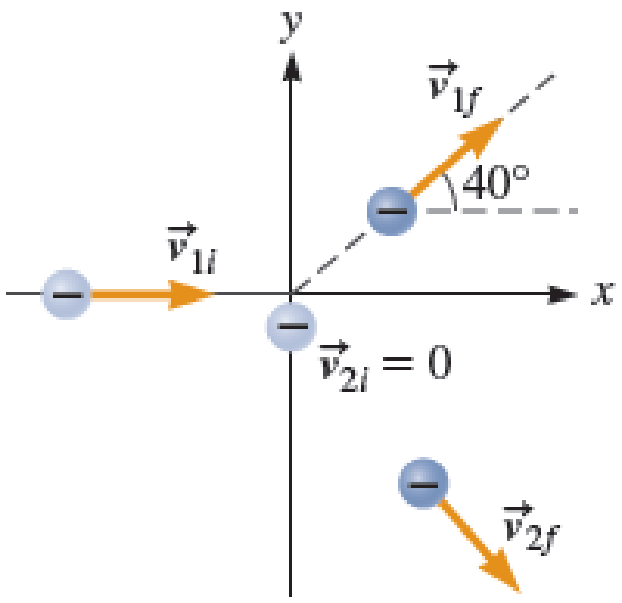
FIGURE P11.50
(a)
Whether it is possible for the initially stationary electron to remain at rest after the collision.
Answer to Problem 50PQ
It is not possible for the initially stationary electron to remain at rest after the collision.
Explanation of Solution
The elastic collision is characterized by the conservation of momentum as well as kinetic energy. For an elastic collision initial momentum of the system before collision should be equal to the momentum of the system after collision.
In the given situation, the initial momentum of the system in
Conclusion:
Thus, it is not possible for the initially stationary electron to remain at rest after the collision.
(b)
The speed of each electron after the collision.
Answer to Problem 50PQ
The speed of initially moving electron after the collision is
Explanation of Solution
The given situation is a two-dimensional elastic collision between two particles of equal mass with initial velocity for one of the particle equal to zero. For such a collision, the angle between the final velocities of the particles after the collision will be equal to
Here,
Rearrange the above equation for
It is given that the value of
Substitute
Write the expression for the conservation of momentum in
Here,
Write the expression for
Here,
Substitute
Write the expression for
Here,
Substitute
Put equations (II) and (III) in equation (I).
The momentum in
Write the expression for the conservation of momentum in
Here,
Write the expression for
Substitute
Write the expression for
The negative sign is due to the fact that the electron 2 moves in negative
Substitute
Put equations (VI) and (VII) in equation (V) and rearrange it for
Put the above equation in equation (IV) and rearrange it for
Put equation (IX) in equation (VIII).
Write the equation for the initial kinetic energy of electron 1.
Here,
Rewrite the above equation for
Conclusion:
It is given that the initial kinetic energy of electron 1 is
Substitute
Substitute
Substitute
Therefore, the speed of initially moving electron after the collision is
Want to see more full solutions like this?
Chapter 11 Solutions
EBK PHYSICS FOR SCIENTISTS AND ENGINEER
- An unstable particle with mass m = 3.34 1027 kg is initially at rest. The particle decays into two fragments that fly off along the x axis with velocity components u1 = 0.987c and u2 = 0.868c. From this information, we wish to determine the masses of fragments 1 and 2. (a) Is the initial system of the unstable particle, which becomes the system of the two fragments, isolated or nonisolated? (b) Based on your answer to part (a), what two analysis models are appropriate for this situation? (c) Find the values of for the two fragments after the decay. (d) Using one of the analysis models in part (b), find a relationship between the masses m1 and m2 of the fragments. (e) Using the second analysis model in part (b). find a second relationship between the masses m1 and m2. (f) Solve the relationships in parts (d) and (c) simultaneously for the masses m1 and m2.arrow_forwardA Carbon-14 nucleus is initially at rest but emits two particles of radiation; one with momentum 5.8x10-21kgm/s[E], and another with momentum 4.5x10-21kgm/s[S]. If the residual Carbon-14 mass is 2.3x10-26kg; a. Determine the residual Carbon 14 momentum magnitude b. Determine the residual Carbon 14 momentum direction c. determine the residual Carbon 14 velocity.arrow_forwardTwo blocks of the same mass move along the same line in opposite directions. The first block is moving with speed v. The blocks collide, stick together, and move with speed 0.100v in the direction of the velocity of the first block before the collision. What was the speed of the second block before the collision? a. 1.20v b. 0.800v c. 10.0v d. 0.900v e. 0.00varrow_forward
- 1. In inertial reference frame A, the energy and the momentum of a particle are measured to be 41 MeV and 40 MeV, respectively. In an inertial reference frame B, the energy of the same particle is measured to be 20 MeV while the momentum is unspecified. What is the mass of the particle in inertial reference frame B? А. 1 MeV В. З МeV С. 6 MeV D. 9 MeV 2. A photon is scattered by an unknown particle of mass 3.0 x 10-43 [kg). A sensor detects the final energy of this photon to be 5.0 x 10-43 [kg]. What is the final momentum of the photon? A. 1.0 x 10-43 (kg] B. 3.0 x 10-43 (kg] C. 5.0 x 10-43 D. 6.4 x 10-43 (kg] [kg] 3. What is the mass of the system of pho- tons shown in figure? A. 0 E = 2m 0 = 30° B. V6m 90° С. 5m D. 3/2m E = 3marrow_forwardElectrons got ejected out as if they were ping pong balls by the light particle (photon). Electrons were not moving initially. Then, it was moving later because of the light. a. What is the momentum of the electron initially (p=mv) Hint: Is it moving initially? b. Does the electron have momentum after being hit by the light particle? Hint: Is it moving after being hit? c. What can you infer about light particles and momentum? Hint: Where is the electron getting its velocity from?arrow_forwarda. If the Pressure produced by the gas cylinder of the motor of the rocket is governed by the below equation: P = sin* (V)cos³(V) And the energy is given by: V2 W = i. If V2=2V1, Find W. ii. What will be W if V2=4V1. iii. Use Matlab or excel to plot the Energy in the interval [0, 2]arrow_forward
- n unstable particle at rest breaks up into two fragments of unequal mass. The mass of the lighter fragment is equal to 3.60 ✕ 10−28 kg and that of the heavier fragment is 1.75 ✕ 10−27 kg. If the lighter fragment has a speed of 0.893c after the breakup, what is the speed of the heavier fragment? carrow_forwardElectron capture is a variant on beta-radiation. The lightest nucleus to decay by electron capture is 7Be -- beryllium-7. The daughter nucleus is 7Li -- lithium-7. The electron is transformed into a massless particle (a neutrino): e − + 7 B e + ⟶ 7 L i + ν The initial electron is bound in the atom, so the beryllium mass includes the electron. In fact, since the electron starts bound in the atom, a more-accurate statement of the nuclear reaction is probably: 7 B e ⟶ 7 L i + ν The masses are beryllium: 7.016929 u, and lithium: 7.016003 u, and refer to the neutral atom as a whole. (Use uc and uc2 as your momentum and energy units -- but carry them along in your calculation.) The initial beryllium atom is stationary. Calculate the speed of the final lithium nucleus in km/s. (You will make life much easier for yourself if you recognize that practically all the energy released goes into the lighter particle. c = 300,000 km/s)arrow_forwardElectron capture is a variant on beta-radiation. The lightest nucleus to decay by electron capture is 7Be -- beryllium-7. The daughter nucleus is 7Li -- lithium-7. The electron is transformed into a massless particle (a neutrino): e − + 7 B e + ⟶ 7 L i + ν The initial electron is bound in the atom, so the beryllium mass includes the electron. In fact, since the electron starts bound in the atom, a more-accurate statement of the nuclear reaction is probably: 7 B e ⟶ 7 L i + ν The masses are beryllium: 7.016929 u, and lithium: 7.016003 u, and refer to the neutral atom as a whole. (Use uc and uc2 as your momentum and energy units -- but carry them along in your calculation.) The initial beryllium atom is stationary. Calculate the speed of the final lithium nucleus in km/s. (all the energy released goes into the lighter particle. c = 300,000 km/s)arrow_forward
- An evacuated tube uses a potential difference of Delta*V = 0.24kV to accelerate electrons, which then hit a copper plate and produce X-rays Part A: write expression for non relativistic speed of these electrons v in terms of e ,delta v and m assuming that he electrons start from rest. Part b: calculate the non relativistic speed of these electrons b in m/aarrow_forward%oV +令自 A Imssb1.mutah.edu.jo/mod/q Determine the energy stored ( in units of mJ) by C4 when C; = 20 µF, C2 = 10 µF, C3 = 14 µF, C4 = 30 µF, and Vo = 69.1 V. C, Select one: A. 0.74 B. 74.49 C. 5.09 D. 1.19 E. 7.64 العربية الإنجليزية II ... +arrow_forwardA cargo truck weighing 5,500 kg accelerates from 4.2 m / s to 7.8 m / s in 15.0 seconds applying a constant force. a. What change does the momentum experience? b. What is the intensity of the force exerted?arrow_forward
 Modern PhysicsPhysicsISBN:9781111794378Author:Raymond A. Serway, Clement J. Moses, Curt A. MoyerPublisher:Cengage Learning
Modern PhysicsPhysicsISBN:9781111794378Author:Raymond A. Serway, Clement J. Moses, Curt A. MoyerPublisher:Cengage Learning Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers, Technology ...PhysicsISBN:9781305116399Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning College PhysicsPhysicsISBN:9781938168000Author:Paul Peter Urone, Roger HinrichsPublisher:OpenStax College
College PhysicsPhysicsISBN:9781938168000Author:Paul Peter Urone, Roger HinrichsPublisher:OpenStax College Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning





