
Two bumper cars at the county fair are sliding toward one another (Fig. P11.54). Initially, bumper car 1 is traveling to the east at 5.62 m/s, and bumper car 2 is traveling 60.0° south of west at 10.00 m/s. After they collide, bumper car 1 is observed to be traveling to the west with a speed of 3.14 m/s. Friction is negligible between the cars and the ground. a. If the masses of bumper cars 1 and 2 are 596 kg and 625 kg respectively, what is the velocity of bumper car 2 immediately after the collision? b. What is the kinetic energy lost in the collision?
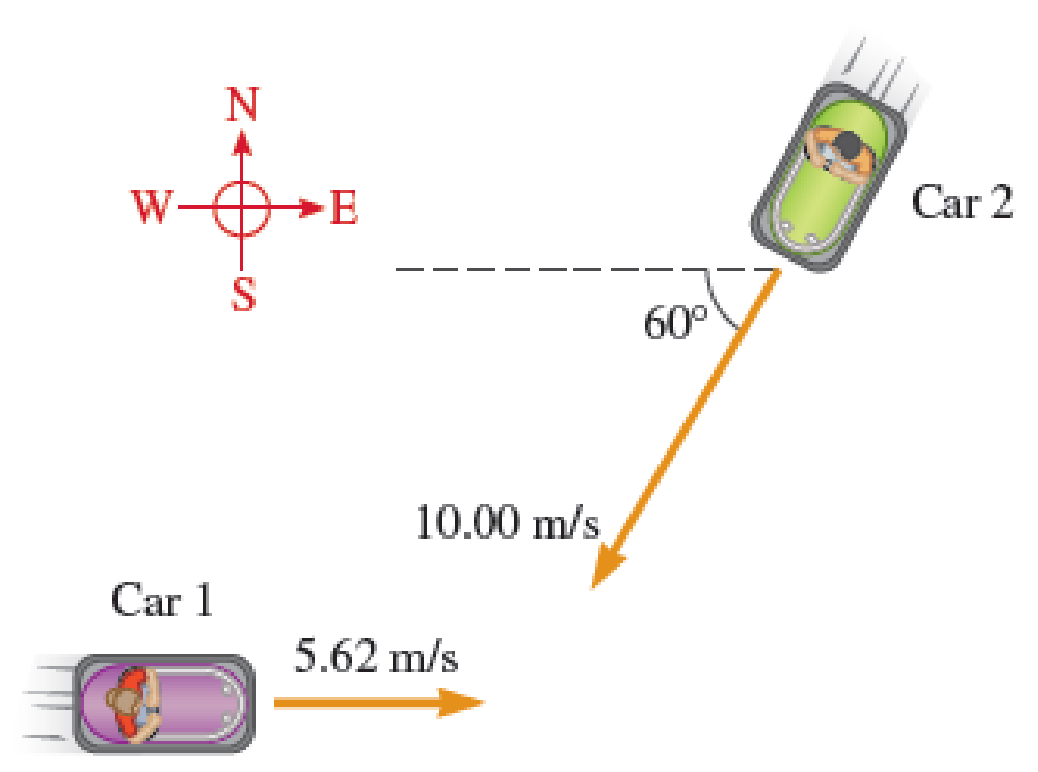
FIGURE P11.54 Problems 54 and 55.
(a)
Velocity of bumper car 2 after collision.
Answer to Problem 54PQ
Velocity of bumper car 2 after collision is
Explanation of Solution
Positive x axis points to the east and the positive y axis points to the north. Apply law of conservation of momentum. The momentum of cars before collision must be equal to the momentum of cars after collision.
Here,
Elaborate equation (I) in terms of mass and velocity.
Here,
Apply the same condition of conservation of momentum in the y direction also. Here only car 2 travels in the y direction.
The initial total momentum in the y direction is equal to the final momentum in the y direction.
Here,
Elaborate equation (III) in terms of mass and velocity.
Here,
Write the equation to find the final velocity of second car.
Here,
Conclusion:
Substitute
Substitute
Substitute
Therefore, velocity of bumper car 2 after collision is
(b)
Kinetic energy lost during the collision.
Answer to Problem 54PQ
The kinetic energy lost is
Explanation of Solution
Write the equation to find the resultant final speed of car 2 after collision.
Here,
The kinetic energy lost is equal to the difference between the kinetic energy before collision and after collision.
Write the equation to find the kinetic energy lost.
Here,
Conclusion:
Substitute
Substitute
Therefore, the kinetic energy lost is
Want to see more full solutions like this?
Chapter 11 Solutions
EBK PHYSICS FOR SCIENTISTS AND ENGINEER
- A rocket has total mass Mi = 360 kg, including Mfuel = 330 kg of fuel and oxidizer. In interstellar space, it starts from rest at the position x = 0, turns on its engine at time t = 0, and puts out exhaust with relative speed ve = 1 500 m/s at the constant rate k = 2.50 kg/s. The fuel will last for a burn time of Tb = Mfuel/k = 330 kg/(2.5 kg/s) = 132 s. (a) Show that during the burn the velocity of the rocket as a function of time is given by v(t)=veln(1ktMi) (b) Make a graph of the velocity of the rocket as a function of time for times running from 0 to 132 s. (c) Show that the acceleration of the rocket is a(t)=kveMikt (d) Graph the acceleration as a function of time. (c) Show that the position of the rocket is x(t)=ve(Mikt)ln(1ktMi)+vet (f) Graph the position during the burn as a function of time.arrow_forwardTwo bumper cars at the county fair are sliding toward one another (see figure below). Initially, bumper car 1 is traveling to the east at 5.62 m/s, and bumper car 2 is traveling 60.0° south of west at 10.00 m/s. After they collide, bumper car 1 is observed to be traveling to the west with a speed of 3.10 m/s. Friction is negligible between the cars and the ground. E Car 2 60 10.00 m/s Car 1 5.62 m/s (a) If the masses of bumper cars 1 and 2 are 600 kg and 631 kg respectively, what is the velocity of bumper car 2 immediately after the collision? (Express your answer in vector form. Enter your answer to at least three significant figures.) m/s (b) What is the kinetic energy lost in the collision?arrow_forwardTwo bumper cars at the county fair are sliding toward one another (see figure below). Initially, bumper car 1 is traveling to the east at 5.62 m/s, and bumper car 2 is traveling 60.0° south of west at 10.00 m/s. After they collide, bumper car 1 is observed to be traveling to the west with a speed of 3.24 m/s. Friction is negligible between the cars and the ground. (a) If the masses of bumper cars 1 and 2 are 588 kg and 633 kg respectively, what is the velocity of bumper car 2 immediately after the collision? (Express your answer in vector form. Enter your answer to at least three significant figures.) (b) What is the kinetic energy lost in the collision?arrow_forward
- In a baseball game, one of the players graps the ball and lessens the influence of the ball by moving his hand back. The ball touches the player's hand at a speed of 140.4 km/h in the horizontal direction. The ball is 152 gr. The player moves his hand back with an average speed of 8 m/s over 14 cm in the horizontal direction. In this way, the player has caused the ball to stop. Calculate the average impulsive force applied on the hand of the player.arrow_forwardTwo asteroids of equal mass collide in an ellastic collision. Asteroid A has an initial velocity of 40 m/s. After the collision, asteroid A deflects an an angle of 30° above its initial direction. Asteroid B is initially at rest. After the collision, asteroid B travels at 45° below the original direction of asteroid A. What are the final speeds of the two asteroids?arrow_forwardA 791 N man stands in the middle of a frozen pond of radius 9.0 m. He is unable to get to the other side because of a lack of friction between his shoes and the ice. To overcome this difficulty, he throws his 1.2 kg physics textbook horizontally toward the north shore at a speed of 9.0 m/s. How long does it take him to reach the south shore?arrow_forward
- A father (mF = 90 kg) and his daughter (mD= 42 kg) stand on a flat frozen lake of negligible friction. They hold a 12 m long rope stretched between them. The father and daughter then pull the rope to bring them together. If the father is initially standing at the origin, how far from the origin will they meet?arrow_forwardA 1.1 g pebble is stuck in a tread of a 0.74 m diameter automobile tire, held in place by static friction that can be at most 3.5 N . The car starts from rest and gradually accelerates on a straight road. How fast is the car moving when the pebble flies out of the tire tread?arrow_forward41. An arrow (m = 5.0 g) is fired at a styrofoam block (m = 150 g) sitting on the edge of a frictionlesstable. The tabletop is 1.1 m above ground. The arrow enters the block horizontally at va1 = 35 m/sand passes through to the other side, exiting horizontally at va2 = 15 m/s. a) Determine the speed of the styrofoam after the arrow exits the block. b) Determine where the Styrofoam will land with respect to the arrow. Assume the arrow passedright through the centre of mass of the block, and both objects drop the same vertical distance tothe ground.arrow_forward
- The carbon isotope 14C is used for carbon dating of archaeological artefacts. 14C decays to 14N by a process known as beta decay, in which the nucleus emits an electron (also known as a beta particle) and a subatomic particle called a neutrino. In one such decay, the electron and the neutrino are emitted in opposite directions to each other. The electron has a speed of 5 x 10 m/s and the neutrino has a momentum of 5 x 1024 kg m/s. n as What is the recoil speed of the resulting 14N nucleus? (Take the mass of the carbon-14 and nitrogen-14 nuclei to be 2.34 x 10 26 kg and the mass of the electron to be 9.11 x 1031 kg. If you were aware of such things and concerned, please ignore relativistic effects.)arrow_forwardAn object with mass m1 = 3.00 kg is moving along the positive x axis with a speed v1i = 2 m/s straight towards two objects with masses m2 = 2.00 kg and m3 = 4.00 kg, which are initially at rest. When they collide, object 1 comes to rest and object 2 moves away with a speed of v2f = 1.5 m/s at an angle of 50 degrees above the x axis. What is the direction of the velocity of the center of mass of the system comprised of all three objects after the collision? A) Along the x axis B) A an angle of 50 degrees above the x axis C) At an angle of 50 degrees below the x axis D) At an angle > 0 degrees and <50 degrees above the x axis E) At an angle>0 degrees and <50 degrees below the x axis The correct answer is A but I am confused why it is A, if you could explain the justification as to why the answer is option A.arrow_forwardThe carbon isotope 14C is used for carbon dating of archaeological artefacts. 14C decays to 14N by a process known as beta decay, in which the nucleus emits an electron (also known as a beta particle) and a subatomic particle called a neutrino. In one such decay, the electron and the neutrino are emitted in opposite directions to each other. The electron has a speed of 4 x 107 m/s and the neutrino has a momentum of 8 × 10-24 kg m/s. What is the recoil speed (in m/s) of the resulting 14N nucleus? (Take the mass of the carbon-14 and nitrogen-14 nuclei to be 2.34 x 10-26 kg and the mass of the electron to be 9.11 x 1031 kg. If you were aware of such things and concerned, please ignore relativistic effects.)arrow_forward
 Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Principles of Physics: A Calculus-Based TextPhysicsISBN:9781133104261Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Physics for Scientists and Engineers with Modern ...PhysicsISBN:9781337553292Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning


