
Concept explainers
Using the information obtained from a study on heart rate, the following data was obtained for the hypothesis test:
- A doctor is testing the claim that the average heart rate of patients is more than 65 bpm.
- Assume the significance level is α=0.01.
- The z− test statistic is calculated as 3.01.
What is the p−value and conclusion for this hypothesis test?
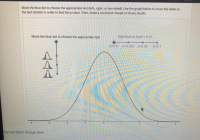
Move the blue dot to choose the appropriate test (left-, right, or two-tailed). Use the graph below to move the slider to the test statistic in order to find the p-value. Then, make a conclusion based on those results.
Select the correct answer below:
a. The p− value is 0.0013. Fail to reject the null hypothesis H0. The doctor does NOT have sufficient evidence that the average heart rate of patients is more than 65 bpm.
b. The p− value is 0.0013. Reject the null hypothesis H0. The doctor has sufficient evidence that the average heart rate of patients is more than 65 bpm.
c. The p− value is 0.9987. Fail to reject the null hypothesis H0. The doctor does NOT have sufficient evidence that the average heart rate of patients is more than 65 bpm.
d. The p− value is 0.9987. Reject the null hypothesis H0. The doctor has sufficient evidence that the average heart rate of patients is more than 65 bpm
Trending nowThis is a popular solution!
Step by stepSolved in 2 steps with 2 images

- State the Result: A hypothesis test was conducted at the alpha = 0.01 level of significance. The test resulted in a p-value of 0.044.arrow_forwardYou wish to test the following daim (Ha) at a significance level of a = 0.05. Ho:P1 P2 You obtain a sample from the first population with 422 successes and 126 failures. You obtain a sample from the second population with 385 successes and 176 failures. critical value = [three decimal accuracy] test statistic = [three decimal accuracy]arrow_forwardYou may need to use the appropriate technology to answer this question. Submitted The following table contains observed frequencies for a sample of 200. Column Variable Row Variable A B C P 20 44 50 Q 30 26 30 Test for independence of the row and column variables using a = 0.05. State the null and alternative hypotheses. Ho: The column variable is independent of the row variable. H: The column variable is not independent of the row variable. Ho: Variable P is not independent of variable Q. H: Variable P is independent of variable Q. Ho: Variable P is independent of variable Q. H: Variable P is not independent of variable Q. : The column variable is not independent of the row variable. Ho: : The column variable is independent of the row variable. Find the value of the test statistic. (Round your answer to three decimal places.) Find the p-value. (Round your answer to four decimal places.) p-value %3D State your conclusion. Reject Ho. We conclude that the column and row variables are…arrow_forward
- For your study on the food consumption of teenage males, you randomlyselected 20 males and asked each how many 12-ounce servings of soda he drinks eachday. The results are listed below. At alpha = 0.1, is there enough evidence to support theclaim that teenage males drink fewer than five 12-ounce servings of soda per day? Noneed to check the criteria –just do the hypothesis test.3.3 4.1 3.5 2.7 3.4 3.3 4.8 3.4 5.2 3.22.5 3.8 5.0 1.9 3.9 5.3 2.9 1.8 4.2 2.2arrow_forwardA radio show producer believes that a new proposed format would be preferred by only 25% of their current listeners. A survey of 100 current listeners showed that 17 favored the new format. Find the test statistic z and test the claim. H0:p= 0.25. use the significance level a=.05.arrow_forwardYou wish to test the following claim (Ha) at a significance level of a = Ho: P₁ = P2 Ha: P₁ P2 You obtain a sample from the first population with 120 successes and 403 failures. You obtain a sample from the second population with 39 successes and 278 failures. The test statistic is... in the critical region O not in the critical region critical value = ± test statistic = This test statistic leads to a decision to... reject the null hypothesis fail to reject the null hypothesis = 0.005. O There is not sufficient evidence to support second population proportion. [three decimal accuracy] [three decimal accuracy] As such, the final conclusion is that... There is sufficient evidence to support that the first population proportion is not equal to the second population proportion. first population proportion not equal to thearrow_forward
- The test statistic of z=1.54 is obtained when testing the claim that p=0.723. a. Identify the hypothesis test as being two-tailed, left-tailed, or right-tailed. b. Find the P-value. c. Using a significance level of a=0.10, should we reject H0 or should we fail to reject H0?arrow_forwardUse a significance level of a=0.05 to test the claim that μ=19. The sample data consists of 15 scores for which x=20.4 and s=4.8. State the null and alternative hypotheses, compute the value of the test statistic, and find the P-value for the sample. State your conclusions about the claim.arrow_forwardA sample of 100 yielded a test statistic of 2.1. For a level of significance of 0.05, the null hypothesis was rejected. What was the sample proportion for the sample of .1 100? What was the p-value for this test? 0.864arrow_forward
 MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc
MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning
Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON
Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman
The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman
Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman





