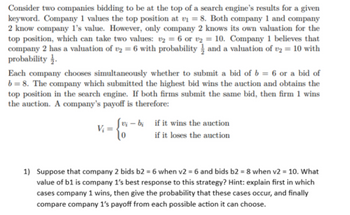
Consider two companies bidding to be at the top of a search engine's results for a given keyword. Company 1 values the top position at v₁ = 8. Both company 1 and company 2 know company 1's value. However, only company 2 knows its own valuation for the top position, which can take two values: v2 = 6 or v₂ = 10. Company 1 believes that company 2 has a valuation of 2 = 6 with probability and a valuation of v2 = 10 with probability. Each company chooses simultaneously whether to submit a bid of b = 6 or a bid of b= 8. The company which submitted the highest bid wins the auction and obtains the top position in the search engine. If both firms submit the same bid, then firm 1 wins the auction. A company's payoff is therefore: V₁ = [v₁ - bį if it wins the auction To if it loses the auction 1) Suppose that company 2 bids b2 = 6 when v2 = 6 and bids b2 = 8 when v2 = 10. What value of b1 is company 1's best response to this strategy? Hint: explain first in which cases company 1 wins, then give the probability that these cases occur, and finally compare company 1's payoff from each possible action it can choose.

A reserve price is a minimum price set by the auctioneer. If no bidder is willing to pay the reserve price, the item is unsold at a profit of $0 for the auctioneer. If only one bidder values the item at or above the reserve price, that bidder pays the reserve price. An auctioneer faces two bidders, each with a value of either $39 or $104, with both values equally probable. Without a reserve price, the second highest bid will be the price paid by the winning bidder. The following table lists the four possible combinations of bidder values. Each combination is equally likely to occur. On the following table, indicate the price paid by the winning bidder with and without the stated reserve price. Bidder 1 Value Bidder 2 Value Probability Price Without Reserve? Price with $104 Reserve Price? ($) ($) ($) $39 $39 0.25           $39 $104 0.25           $104 $39 0.25           $104 $104 0.25             Without a reserve price, the expected price is…

In Homework 1, we saw that there is no strict dominant strategy equilibrium in an inspection game. Thus, the players must randomize and play a mixed strategy in equilibrium. Suppose the agent shirks with probability p₁ and works with probability (1 - p₁), while the principal inspects with probability p2 and does not inspect with probability (1-P2). Find the mixed strategy equilibrium for this game. I NI S (0, -k) (w, -w) W (w c, p-w-k)| (wc, p-w) Table 1: Payoff matrix for the inspection game

None