
A 15-cm-thick mesh filter is being used to filter uniformly sized microorganisms from a 10 m3/minute air stream, effectively reducing the concentration from 200 microorganisms/m3 to 10/m3 over a 100-hour fermentation period. New regulations mandate a reduction to 1/m3 . If the air velocity and ambient conditions do not change, what depth of the same type of filter is required?
Given:
The flow rate of the air stream, Q = 10 m3/min
The initial and final concentration of the microorganism in this airstream is:

Fermentation period, t = 100 h
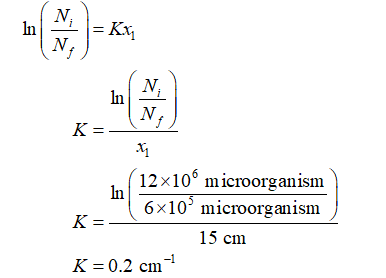
The thickness of the mesh filter, x1 = 15 cm
The number of particles at initial as well as at the final conditions are calculated as:
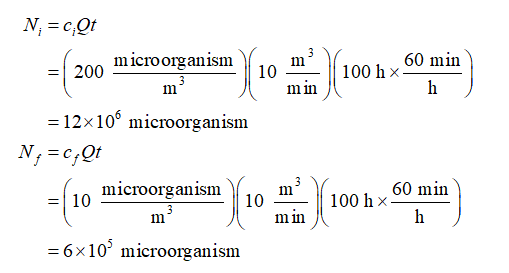
Calculate the value of constant K that relates this number of microorganisms to the mesh depth as:
Step by stepSolved in 6 steps with 6 images

- Steel surface can be hardened by carburization. Such process is done at 1000°C and the concentration gradient (dc/dx) is -8.23x1029 atoms/m4. The coefficient of diffusion (D0) for Fe is 20x10-6 m2/s (See table 1). Estimate the flux of carbon atoms into the steel in this process.arrow_forwardA 0.02 M feed solution containing a macromolecular solute is to be concentrated to 0.1 M concentration by batch ultrafiltration at 25℃. The solute rejection is 95% . If the upstream pressure is 3.5 atm and the downstream pressure is essentially atmospheric, calculate the effective pressure driving force at the beginning and at the end of the process. Also estimate the fractional reduction in the solvent flux at the end of the process. (assume fractional reduction in flux = fractional reduction in driving force).arrow_forward2) Filtration involves the separation of solid particles from fluid through a filtering medium and ranges from simple straining to more complex operations. Depending on the objective of the filtration process, the fluid, solid or both may be useful outputs of the overall process(a)Describe the basic principles offiltration as applied in the chemical industry. Using a suitable example, explain with clear illustrationshow filtration technique can be used to separate solids from filtrates at industrial scalearrow_forward
- This method of leak detection is simple and practical as it does not require expensive equipment, sometimes the use of antifreeze is required. c) ultrasonic detection b) ultraviolet light d) simple auditory inspection a) soap solution Which of the following groups of refrigerants has the greatest impact on the ozone layer? b) HCFC d) HFC a) CFC c) HCarrow_forwardIn the process of drying carrots using a parallel flow drying system, the material with a moisture content of 85% enters the drying system at a rate of 500 Kg / hour to produce dry carrots with a moisture content of 20%. If it is known that hot air used as the drying medium has a humidity ratio of 0.006 Kg of water per Kg of dry air and the flow of hot air enters the system at a rate of 200 Kg of air for each dry matter produced, calculate the ratio of humidity to air leaving the system assuming that the system is under steady state conditions.arrow_forward1.6 Properties: Diffusivity Estimate the molecular diameter and diffusion coefficient for the proteins ribonuclease (MW 13,700 Da), hemoglobin (MW 68,000), and urease (MW 480,000), assuming the molecules are spherical and the density of each protein molecule is 1.3 g/cm³.arrow_forward
- An evaporation-crystallization process (as shown below) is used to obtain solid potassium sulfate from an aqueous salt solution. The feed contains 18.7 wt% K2SO4. The wet filter cake contains solid sulfate crystals and a 40.0 wt% salt solution. The filtrate is recycled to mix with the feed. The evaporator has a capacity of 175 kg of water evaporated per second. The filtrate has 40.0 wt% salt solution, as well, and from the water entering the evaporator, 45.0% is evaporated. In the wet filter cake, there is 10 kg of the solid crystals for every 1 kg of the salt solution. a.) Calculate the fresh feed rate. (Include units) b.) Determine the production rate of crystal. (Include units) c.) Calculate the recycle ratio. (kg recycle/kg feed) Include unitsarrow_forwardA double effect evaporator is used to concentrate an 18% CuSO4 solution. At the end of evaporation, 26,000 kg/h of 50% CuSO4 solution were obtained. If the water evaporated in the second effect is half the water evaporated in the first effect, determine: a) The amount of solution fed into the first effect. b) The amount of water evaporated in the first and second effect. c) The amount of solution fed into the second effect, as well as its composition. Problem diagram 18% CuSO4 82% H₂O M₁ M₂ M3 X CuSO4 Y H₂O A 2 M4 = 0.5 M₂ M5 H₂O steam M6 M2 M6 is the water that condenses 26 000 kg/hr 50% CuSO4 50% H2₂Oarrow_forwardAn evaporation-crystallization process (as shown below) is used to obtain solid potassium sulfate from an aqueous salt solution. The feed contains 18.7 wt% K2SO4. The wet filter cake contains solid sulfate crystals and a 40.0 wt% salt solution. The filtrate is recycled to mix with the feed. The evaporator has a capacity of 175 kg of water evaporated per second. The filtrate has 40.0 wt% salt solution, as well, and from the water entering the evaporator, 45.0% is evaporated. In the wet filter cake, there is 10 kg of the solid crystals for every 1 kg of the salt solution. a.) Calculate the fresh feed rate. (Include units) b.) Determine the production rate of crystal. (Include units) c.) Calculate the recycle ratio. (kg recycle/kg feed) Include unitsarrow_forward
- Reverse Osmosis is used by water bottling companies to produce desalinized water from sea water. Giventhe figure below, determine the fraction of brine leaving the RO cell that is recycled.arrow_forwardThe following consideration(s) is/are relevant to the choice of refrigerant (SELECT ALL that apply): Vapor pressure Environmental factors Toxicity and corrosion properties Water is usually used as the refrigerantarrow_forwardTo make an instant tomato soup, a company makes soup powder from liquid step by a two-step process: concentrating the soup by removing water using a membrane to reach a concentration of 45% water, followed by a spray drying process which leads to a final moisture content of 3%. If the initial liquid tomato soup contains 9.0% solids and fat and enters the membrane separator at 4000 kg/hr, calculate: The flow-rate of the concentrated soup after membrane separation: kg/hr The flow-rate of water removed by the spray drying process (the second stage): kg/hrarrow_forward
 Introduction to Chemical Engineering Thermodynami...Chemical EngineeringISBN:9781259696527Author:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark SwihartPublisher:McGraw-Hill Education
Introduction to Chemical Engineering Thermodynami...Chemical EngineeringISBN:9781259696527Author:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark SwihartPublisher:McGraw-Hill Education Elementary Principles of Chemical Processes, Bind...Chemical EngineeringISBN:9781118431221Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. BullardPublisher:WILEY
Elementary Principles of Chemical Processes, Bind...Chemical EngineeringISBN:9781118431221Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. BullardPublisher:WILEY Elements of Chemical Reaction Engineering (5th Ed...Chemical EngineeringISBN:9780133887518Author:H. Scott FoglerPublisher:Prentice Hall
Elements of Chemical Reaction Engineering (5th Ed...Chemical EngineeringISBN:9780133887518Author:H. Scott FoglerPublisher:Prentice Hall
 Industrial Plastics: Theory and ApplicationsChemical EngineeringISBN:9781285061238Author:Lokensgard, ErikPublisher:Delmar Cengage Learning
Industrial Plastics: Theory and ApplicationsChemical EngineeringISBN:9781285061238Author:Lokensgard, ErikPublisher:Delmar Cengage Learning Unit Operations of Chemical EngineeringChemical EngineeringISBN:9780072848236Author:Warren McCabe, Julian C. Smith, Peter HarriottPublisher:McGraw-Hill Companies, The
Unit Operations of Chemical EngineeringChemical EngineeringISBN:9780072848236Author:Warren McCabe, Julian C. Smith, Peter HarriottPublisher:McGraw-Hill Companies, The





