
- (a) Explain the polar
coordinate system . - (b) Graph the points with polar coordinates (2, π/3) and (−1, 3π/4).
- (c) State the equations that relate the rectangular coordinates of a point to its polar coordinates.
- (d) Find rectangular coordinates for (2, π/3).
- (e) Find polar coordinates for P(−2, 2).
(a)
To describe: The polar coordinate system.
Explanation of Solution
The coordinate system
In polar coordinate system r shows the distance of the point and
In polar coordinate system take
In polar coordinate system negative r signifies that the polar coordinate
The below figure shows the polar coordinates
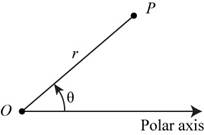
Figure (1)
In the above figure, the point P is r unit away adjoining with angle
(b)
To sketch: The graph of the polar coordinates.
Explanation of Solution
The below graph shows the polar coordinates
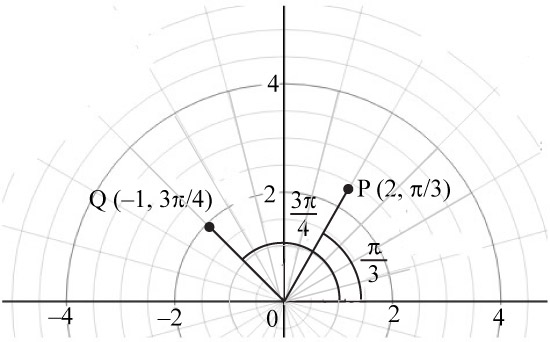
Figure (2)
In the above graph, point
(c)
To describe: The equations that relate the rectangular coordinates and polar coordinates of a point with each other.
Explanation of Solution
Use the equations
Use the equations
(d)
To find: The rectangular coordinate of the point.
Answer to Problem 1RCC
The rectangular coordinate of the point
Explanation of Solution
Given:
The value of polar coordinate is
Calculation:
Use the equations
The formula to calculate the x coordinate is,
Substitute 2 for r and
The value of the x coordinate is 1.
The formula to calculate the y coordinate is,
Substitute 2 for r and
The value of the y coordinate is
Thus, the rectangular coordinate of the point
(e)
To find: The polar coordinate of the point.
Answer to Problem 1RCC
The polar coordinate of the point
Explanation of Solution
Given:
The value of rectangular coordinate is
Calculation:
Use the equations
The formula to calculate the r is,
Substitute
The value of r is
The formula to calculate the value of
Substitute
The value of
Thus, the rectangular coordinate of the point
Want to see more full solutions like this?
Chapter 8 Solutions
Precalculus: Mathematics for Calculus (Standalone Book)
- Can you solve question 3,4,5 and 6 for this questionarrow_forwardwater at a rate of 2 m³/min. of the water height in this tank? 16) A box with a square base and an open top must have a volume of 256 cubic inches. Find the dimensions of the box that will minimize the amount of material used (the surface area). 17) A farmer wishes toarrow_forward#14 Sand pours from a chute and forms a conical pile whose height is always equal to its base diameter. The height o the pile increases at a rate of 5 feet/hour. Find the rate of change of the volume of the sand in the conical pile when the height of the pile is 4 feet.arrow_forward
- (d)(65in(x)-5 cos(x) dx mins by 5x-2x² 3x+1 dx -dx 20 Evaluate each the following indefinite integralsarrow_forward19 Evaluate each the following definite integrals: a) લ b) (+3) 6) (2-2)(+33) dxarrow_forward#11 If a snowball melts so its surface area decreases at a rate of 1cm²/min, find the rate at which the diameter decreases when the diameter is 6 cm.arrow_forward
- Use Deritivitve of the inverse to solve thisarrow_forwardEvaluate the following Limits: e6x-1 Lim +0Sin3x 7x-5x2 2x-1+ Cos 4x +6 c) Lim b) Lim + x³-x2 X-0 1-e' 4x d) Lim 6x²-3 X+0 6x+2x² Find the derivatives of the following functions using the Limit definition of derivativearrow_forward15A cylindrical tank with radius 8 m is being filled with water at a rate of 2 m³/min. What is the rate of change of the water height in this tank? 6)A box with a square base and an open top must box that will minimiarrow_forward
- #12 The radius of a sphere increases at a rate of 3 in/sec. How fast is the volume increasing when the diameter is 24arrow_forward84 256 cubic inches. Find the dimensions of the of material used (the surface area). A farmer wishes to enclose a rectangular plot using 200 m of fencing material. One side of the land borders a river and does not need fencing. What is the largest area that can be enclosed? For the function y=x³-3x²-1, use derivatives to: 3 b) 2x - 6x2 (a) determine the intarrow_forwardCan you solve this 6 questions numerical method and teach me how to solve it and what we use.arrow_forward
 Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage LearningAlgebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage LearningAlgebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage

