Single Variable Calculus: Concepts and Contexts, Enhanced Edition

4th Edition

ISBN: 9781337687805

Author: James Stewart

Publisher: Cengage Learning

See similar textbooks

Concept explainers

Angles in Circles

Angles within a circle are feasible to create with the help of different properties of the circle such as radii, tangents, and chords. The radius is the distance from the center of the circle to the circumference of the circle. A tangent is a line made p…

Arcs in Circles

A circular arc is the arc of a circle formed by two distinct points. It is a section or segment of the circumference of a circle. A straight line passing through the center connecting the two distinct ends of the arc is termed a semi-circular arc.

Videos

Question

Chapter 8, Problem 12P

(a)

To determine

To show: That $12xn+xn+1+xn+2+xn+3=2$ , and find a similar equation for the $y$ -coordinates.

(a)

Expert Solution

Answer to Problem 12P

It is proved that $12xn+xn+1+xn+2+xn+3=2$ , and similar equation in terms of $y$ is $12yn+yn+1+yn+2+yn+3=32$ .

Explanation of Solution

Given:

The vertices of the square are $P1(0,1)$ , $P2(1,1)$ , $P3(1,0)$ , and $P4(0,0)$ .

Calculation:

Draw the diagram as follows.

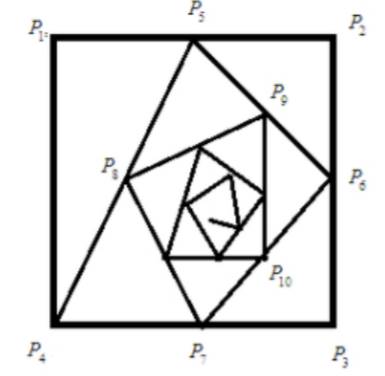
Single Variable Calculus: Concepts and Contexts, Enhanced Edition, Chapter 8, Problem 12P

$Pn(xn,yn)$ is the midpoint of $Pn−4$ and $Pn−3$ . So, $xn=12(xn−4+xn−3)$ for $n≥5$ .

Use the induction on $n$ , to prove the result.

Let $n=1$ ,

Substitute $n=1$ in the equation $12xn+xn+1+xn+2+xn+3=2$ .

$12x1+x1+1+x1+2+x1+3=212x1+x2+x3+x4=212(0)+(1)+(1)+(0)=22=2 (True)$

Assume the result is true for $n=k−1$ .

$12xk−1+xk−1+1+xk−1+2+xk−1+3=212xk−1+xk+xk+1+xk+2=2$

Assume the result is true for $n=k$ .

$12xk+xk+1+xk+2+xk+3=2$

Since $Pk+3(xk+3,yk+3)$ is the midpoint of $Pk+3−4$ , and $Pk+3−3$ .

$12xk+xk+1+xk+2+12(xk+3−4+xk+3−3)=212xk+xk+1+xk+2+12(xk−1+xk)=212xk−1+xk+xk+1+xk+2=2$

Since the result is true for $n=k−1$ .

$12xk−1+xk+xk+1+xk+2=22=2$

Therefore, by the induction, the result $12xn+xn+1+xn+2+xn+3=2$ is true for all $n$ .

$Pn(xn,yn)$ is the midpoint of $Pn−4$ and $Pn−3$ . So, $yn=12(yn−4+yn−3)$ for $n≥5$ .

Consider $12yn+yn+1+yn+2+yn+3$ .

Let $n=1$ ,

Substitute $n=1$ in the equation $12yn+yn+1+yn+2+yn+3$ .

$12yn+yn+1+yn+2+yn+3=12y1+y1+1+y1+2+y1+3=12y1+y2+y3+y4=12(1)+(1)+(0)+(0)=32$

Assume the result is true for $n=k−1$ .

Substitute $n=k−1$ in the equation $12yn+yn+1+yn+2+yn+3$ .

$12yk−1+yk+yk+1+yk+2=32$

Assume the result is true for $n=k$ .

Substitute $n=k$ in the equation $12yn+yn+1+yn+2+yn+3$ .

$12yk+yk+1+yk+2+yk+3=32$

Since $Pk+3(xk+3,yk+3)$ is the midpoint of $Pk+3−4$ , and $Pk+3−3$ .

$12yk+yk+1+yk+2+12(yk+3−4+yk+3−3)=3212yk+yk+1+yk+2+12(yk−1+yk)=3212yk−1+yk+yk+1+yk+2=32$

Since the result is true for $n=k−1$ .

$12yk−1+yk+yk+1+yk+2=3232=32$

Therefore, by the induction, the result $12yn+yn+1+yn+2+yn+3=32$ is true for all $n$ .

Therefore, it is proved that $12xn+xn+1+xn+2+xn+3=2$ , and similar equation in terms of $y$ is $12yn+yn+1+yn+2+yn+3=32$ .

(b)

To determine

To find: The coordinate of $P$ .

(b)

Expert Solution

Answer to Problem 12P

The point $P$ is $(47, 37)$ .

Explanation of Solution

Given:

The vertices of the square are $P1(0,1)$ , $P2(1,1)$ , $P3(1,0)$ , and $P4(0,0)$ .

Calculation:

To find the $x$ coordinate for $P$ , take the limit tends to infinity for the relation $12xn+xn+1+xn+2+xn+3=2$ .

$limn→∞(12xn+xn+1+xn+2+xn+3)=limn→∞(2)limn→∞(12xn)+limn→∞(xn+1)+limn→∞(xn+2)+limn→∞(xn+3)=limn→∞(2)12limn→∞(xn)+limn→∞(xn+1)+limn→∞(xn+2)+limn→∞(xn+3)=2$

Since the values of the above all limits are equal.

$12limn→∞(xn)+limn→∞(xn)+limn→∞(xn)+limn→∞(xn)=2limn→∞(xn)(12+1+1+1)=272limn→∞(xn)=2limn→∞(xn)=47$

To find the $y$ coordinate for $P$ , take the limit tends to infinity for the relation $12yn+yn+1+yn+2+yn+3=32$ .

$limn→∞(12yn+yn+1+yn+2+yn+3)=limn→∞(32)limn→∞(12yn)+limn→∞(yn+1)+limn→∞(yn+2)+limn→∞(yn+3)=limn→∞(32)12limn→∞(yn)+limn→∞(yn+1)+limn→∞(yn+2)+limn→∞(yn+3)=32$

Since the values of the above all limits are equal.

$12limn→∞(yn)+limn→∞(yn)+limn→∞(yn)+limn→∞(yn)=32limn→∞(yn)(12+1+1+1)=3272limn→∞(xn)=32limn→∞(yn)=37$

Therefore, the point $P$ is $(47, 37)$ .

Chapter 8, Problem 11P

Chapter 8, Problem 14P

Chapter 8 Solutions

Single Variable Calculus: Concepts and Contexts, Enhanced Edition

Ch. 8.1 - Prob. 1E Ch. 8.1 - Prob. 2E Ch. 8.1 - Prob. 3E Ch. 8.1 - Prob. 4E Ch. 8.1 - Prob. 5E Ch. 8.1 - Prob. 6E Ch. 8.1 - Prob. 7E Ch. 8.1 - Prob. 8E Ch. 8.1 - Prob. 9E Ch. 8.1 - Prob. 10E

Ch. 8.1 - Prob. 11E Ch. 8.1 - Prob. 12E Ch. 8.1 - Prob. 13E Ch. 8.1 - Prob. 14E Ch. 8.1 - Prob. 15E Ch. 8.1 - Prob. 16E Ch. 8.1 - Prob. 17E Ch. 8.1 - Prob. 18E Ch. 8.1 - Prob. 19E Ch. 8.1 - Prob. 20E Ch. 8.1 - Prob. 21E Ch. 8.1 - Prob. 22E Ch. 8.1 - Prob. 23E Ch. 8.1 - Prob. 24E Ch. 8.1 - Prob. 25E Ch. 8.1 - Prob. 26E Ch. 8.1 - Prob. 27E Ch. 8.1 - Prob. 28E Ch. 8.1 - Prob. 29E Ch. 8.1 - Prob. 30E Ch. 8.1 - Prob. 31E Ch. 8.1 - Prob. 32E Ch. 8.1 - Prob. 33E Ch. 8.1 - Prob. 34E Ch. 8.1 - Prob. 35E Ch. 8.1 - Prob. 36E Ch. 8.1 - Prob. 37E Ch. 8.1 - Prob. 38E Ch. 8.1 - Prob. 39E Ch. 8.1 - Prob. 40E Ch. 8.1 - Prob. 41E Ch. 8.1 - Prob. 42E Ch. 8.1 - Prob. 43E Ch. 8.1 - Prob. 44E Ch. 8.1 - Prob. 45E Ch. 8.1 - Prob. 46E Ch. 8.1 - Prob. 47E Ch. 8.1 - Prob. 48E Ch. 8.1 - Prob. 49E Ch. 8.1 - Prob. 50E Ch. 8.1 - Prob. 51E Ch. 8.1 - Prob. 52E Ch. 8.1 - Prob. 53E Ch. 8.1 - Prob. 54E Ch. 8.1 - Prob. 55E Ch. 8.1 - Prob. 56E Ch. 8.1 - Prob. 57E Ch. 8.1 - Prob. 58E Ch. 8.1 - Prob. 59E Ch. 8.1 - Prob. 60E Ch. 8.2 - Prob. 1E Ch. 8.2 - Prob. 2E Ch. 8.2 - Prob. 3E Ch. 8.2 - Prob. 4E Ch. 8.2 - Prob. 5E Ch. 8.2 - Prob. 6E Ch. 8.2 - Prob. 7E Ch. 8.2 - Prob. 8E Ch. 8.2 - Prob. 9E Ch. 8.2 - Prob. 10E Ch. 8.2 - Prob. 11E Ch. 8.2 - Prob. 12E Ch. 8.2 - Prob. 13E Ch. 8.2 - Prob. 14E Ch. 8.2 - Prob. 15E Ch. 8.2 - Prob. 16E Ch. 8.2 - Prob. 17E Ch. 8.2 - Prob. 18E Ch. 8.2 - Prob. 19E Ch. 8.2 - Prob. 20E Ch. 8.2 - Prob. 21E Ch. 8.2 - Prob. 22E Ch. 8.2 - Prob. 23E Ch. 8.2 - Prob. 24E Ch. 8.2 - Prob. 25E Ch. 8.2 - Prob. 26E Ch. 8.2 - Prob. 27E Ch. 8.2 - Prob. 28E Ch. 8.2 - Prob. 29E Ch. 8.2 - Prob. 30E Ch. 8.2 - Prob. 31E Ch. 8.2 - Prob. 32E Ch. 8.2 - Prob. 33E Ch. 8.2 - Prob. 34E Ch. 8.2 - Prob. 35E Ch. 8.2 - Prob. 36E Ch. 8.2 - Prob. 37E Ch. 8.2 - Prob. 38E Ch. 8.2 - Prob. 39E Ch. 8.2 - Prob. 40E Ch. 8.2 - Prob. 41E Ch. 8.2 - Prob. 42E Ch. 8.2 - Prob. 43E Ch. 8.2 - Prob. 44E Ch. 8.2 - Prob. 45E Ch. 8.2 - Prob. 46E Ch. 8.2 - Prob. 47E Ch. 8.2 - Prob. 48E Ch. 8.2 - Prob. 49E Ch. 8.2 - Prob. 50E Ch. 8.2 - Prob. 51E Ch. 8.2 - Prob. 52E Ch. 8.2 - Prob. 53E Ch. 8.2 - Prob. 54E Ch. 8.2 - Prob. 55E Ch. 8.2 - Prob. 56E Ch. 8.2 - Prob. 57E Ch. 8.2 - Prob. 58E Ch. 8.2 - Prob. 59E Ch. 8.2 - Prob. 60E Ch. 8.2 - Prob. 61E Ch. 8.2 - Prob. 62E Ch. 8.2 - Prob. 63E Ch. 8.2 - Prob. 64E Ch. 8.2 - Prob. 65E Ch. 8.2 - Prob. 66E Ch. 8.2 - Prob. 67E Ch. 8.2 - Prob. 68E Ch. 8.3 - Prob. 1E Ch. 8.3 - Prob. 2E Ch. 8.3 - Prob. 3E Ch. 8.3 - Prob. 4E Ch. 8.3 - Prob. 5E Ch. 8.3 - Prob. 6E Ch. 8.3 - Prob. 7E Ch. 8.3 - Prob. 8E Ch. 8.3 - Prob. 9E Ch. 8.3 - Prob. 10E Ch. 8.3 - Prob. 11E Ch. 8.3 - Prob. 12E Ch. 8.3 - Prob. 13E Ch. 8.3 - Prob. 14E Ch. 8.3 - Prob. 15E Ch. 8.3 - Prob. 16E Ch. 8.3 - Prob. 17E Ch. 8.3 - Prob. 18E Ch. 8.3 - Prob. 19E Ch. 8.3 - Prob. 20E Ch. 8.3 - Prob. 21E Ch. 8.3 - Prob. 22E Ch. 8.3 - Prob. 23E Ch. 8.3 - Prob. 24E Ch. 8.3 - Prob. 25E Ch. 8.3 - Prob. 26E Ch. 8.3 - Prob. 27E Ch. 8.3 - Prob. 28E Ch. 8.3 - Prob. 29E Ch. 8.3 - Prob. 30E Ch. 8.3 - Prob. 31E Ch. 8.3 - Prob. 32E Ch. 8.3 - Prob. 33E Ch. 8.3 - Prob. 34E Ch. 8.3 - Prob. 35E Ch. 8.3 - Prob. 36E Ch. 8.3 - Prob. 37E Ch. 8.3 - Prob. 38E Ch. 8.3 - Prob. 39E Ch. 8.3 - Prob. 40E Ch. 8.3 - Prob. 41E Ch. 8.3 - Prob. 42E Ch. 8.3 - Prob. 43E Ch. 8.3 - Prob. 44E Ch. 8.3 - Prob. 45E Ch. 8.3 - Prob. 46E Ch. 8.4 - Prob. 1E Ch. 8.4 - Prob. 2E Ch. 8.4 - Prob. 3E Ch. 8.4 - Prob. 4E Ch. 8.4 - Prob. 5E Ch. 8.4 - Prob. 6E Ch. 8.4 - Prob. 7E Ch. 8.4 - Prob. 8E Ch. 8.4 - Prob. 9E Ch. 8.4 - Prob. 10E Ch. 8.4 - Prob. 11E Ch. 8.4 - Prob. 12E Ch. 8.4 - Prob. 13E Ch. 8.4 - Prob. 14E Ch. 8.4 - Prob. 15E Ch. 8.4 - Prob. 16E Ch. 8.4 - Prob. 17E Ch. 8.4 - Prob. 18E Ch. 8.4 - Prob. 19E Ch. 8.4 - Prob. 20E Ch. 8.4 - Prob. 21E Ch. 8.4 - Prob. 22E Ch. 8.4 - Prob. 23E Ch. 8.4 - Prob. 24E Ch. 8.4 - Prob. 25E Ch. 8.4 - Prob. 26E Ch. 8.4 - Prob. 27E Ch. 8.4 - Prob. 28E Ch. 8.4 - Prob. 29E Ch. 8.4 - Prob. 30E Ch. 8.4 - Prob. 31E Ch. 8.4 - Prob. 32E Ch. 8.4 - Prob. 33E Ch. 8.4 - Prob. 34E Ch. 8.4 - Prob. 35E Ch. 8.4 - Prob. 36E Ch. 8.4 - Prob. 37E Ch. 8.4 - Prob. 38E Ch. 8.4 - Prob. 39E Ch. 8.4 - Prob. 40E Ch. 8.4 - Prob. 41E Ch. 8.4 - Prob. 42E Ch. 8.5 - Prob. 1E Ch. 8.5 - Prob. 2E Ch. 8.5 - Prob. 3E Ch. 8.5 - Prob. 4E Ch. 8.5 - Prob. 5E Ch. 8.5 - Prob. 6E Ch. 8.5 - Prob. 7E Ch. 8.5 - Prob. 8E Ch. 8.5 - Prob. 9E Ch. 8.5 - Prob. 10E Ch. 8.5 - Prob. 11E Ch. 8.5 - Prob. 12E Ch. 8.5 - Prob. 13E Ch. 8.5 - Prob. 14E Ch. 8.5 - Prob. 15E Ch. 8.5 - Prob. 16E Ch. 8.5 - Prob. 17E Ch. 8.5 - Prob. 18E Ch. 8.5 - Prob. 19E Ch. 8.5 - Prob. 20E Ch. 8.5 - Prob. 21E Ch. 8.5 - Prob. 22E Ch. 8.5 - Prob. 23E Ch. 8.5 - Prob. 24E Ch. 8.5 - Prob. 25E Ch. 8.5 - Prob. 26E Ch. 8.5 - Prob. 27E Ch. 8.5 - Prob. 28E Ch. 8.5 - Prob. 29E Ch. 8.5 - Prob. 30E Ch. 8.5 - Prob. 31E Ch. 8.5 - Prob. 32E Ch. 8.5 - Prob. 33E Ch. 8.5 - Prob. 34E Ch. 8.5 - Prob. 35E Ch. 8.5 - Prob. 36E Ch. 8.6 - Prob. 1E Ch. 8.6 - Prob. 2E Ch. 8.6 - Prob. 3E Ch. 8.6 - Prob. 4E Ch. 8.6 - Prob. 5E Ch. 8.6 - Prob. 6E Ch. 8.6 - Prob. 7E Ch. 8.6 - Prob. 8E Ch. 8.6 - Prob. 9E Ch. 8.6 - Prob. 10E Ch. 8.6 - Prob. 11E Ch. 8.6 - Prob. 12E Ch. 8.6 - Prob. 13E Ch. 8.6 - Prob. 14E Ch. 8.6 - Prob. 15E Ch. 8.6 - Prob. 16E Ch. 8.6 - Prob. 17E Ch. 8.6 - Prob. 18E Ch. 8.6 - Prob. 19E Ch. 8.6 - Prob. 20E Ch. 8.6 - Prob. 21E Ch. 8.6 - Prob. 22E Ch. 8.6 - Prob. 23E Ch. 8.6 - Prob. 24E Ch. 8.6 - Prob. 25E Ch. 8.6 - Prob. 26E Ch. 8.6 - Prob. 27E Ch. 8.6 - Prob. 28E Ch. 8.6 - Prob. 29E Ch. 8.6 - Prob. 30E Ch. 8.6 - Prob. 31E Ch. 8.6 - Prob. 32E Ch. 8.6 - Prob. 33E Ch. 8.6 - Prob. 34E Ch. 8.6 - Prob. 35E Ch. 8.6 - Prob. 36E Ch. 8.6 - Prob. 37E Ch. 8.6 - Prob. 38E Ch. 8.6 - Prob. 39E Ch. 8.6 - Prob. 40E Ch. 8.7 - Prob. 1E Ch. 8.7 - Prob. 2E Ch. 8.7 - Prob. 3E Ch. 8.7 - Prob. 4E Ch. 8.7 - Prob. 5E Ch. 8.7 - Prob. 6E Ch. 8.7 - Prob. 7E Ch. 8.7 - Prob. 8E Ch. 8.7 - Prob. 9E Ch. 8.7 - Prob. 10E Ch. 8.7 - Prob. 11E Ch. 8.7 - Prob. 12E Ch. 8.7 - Prob. 13E Ch. 8.7 - Prob. 14E Ch. 8.7 - Prob. 15E Ch. 8.7 - Prob. 16E Ch. 8.7 - Prob. 17E Ch. 8.7 - Prob. 18E Ch. 8.7 - Prob. 19E Ch. 8.7 - Prob. 20E Ch. 8.7 - Prob. 21E Ch. 8.7 - Prob. 22E Ch. 8.7 - Prob. 23E Ch. 8.7 - Prob. 24E Ch. 8.7 - Prob. 25E Ch. 8.7 - Prob. 26E Ch. 8.7 - Prob. 27E Ch. 8.7 - Prob. 28E Ch. 8.7 - Prob. 29E Ch. 8.7 - Prob. 30E Ch. 8.7 - Prob. 31E Ch. 8.7 - Prob. 32E Ch. 8.7 - Prob. 33E Ch. 8.7 - Prob. 34E Ch. 8.7 - Prob. 35E Ch. 8.7 - Prob. 36E Ch. 8.7 - Prob. 37E Ch. 8.7 - Prob. 38E Ch. 8.7 - Prob. 39E Ch. 8.7 - Prob. 40E Ch. 8.7 - Prob. 41E Ch. 8.7 - Prob. 42E Ch. 8.7 - Prob. 43E Ch. 8.7 - Prob. 44E Ch. 8.7 - Prob. 45E Ch. 8.7 - Prob. 46E Ch. 8.7 - Prob. 47E Ch. 8.7 - Prob. 48E Ch. 8.7 - Prob. 49E Ch. 8.7 - Prob. 50E Ch. 8.7 - Prob. 51E Ch. 8.7 - Prob. 52E Ch. 8.7 - Prob. 53E Ch. 8.7 - Prob. 54E Ch. 8.7 - Prob. 55E Ch. 8.7 - Prob. 56E Ch. 8.7 - Prob. 57E Ch. 8.7 - Prob. 58E Ch. 8.7 - Prob. 59E Ch. 8.7 - Prob. 60E Ch. 8.7 - Prob. 61E Ch. 8.7 - Prob. 62E Ch. 8.7 - Prob. 63E Ch. 8.7 - Prob. 64E Ch. 8.7 - Prob. 65E Ch. 8.7 - Prob. 66E Ch. 8.7 - Prob. 67E Ch. 8.7 - Prob. 68E Ch. 8.7 - Prob. 69E Ch. 8.7 - Prob. 70E Ch. 8.8 - Prob. 1E Ch. 8.8 - Prob. 2E Ch. 8.8 - Prob. 3E Ch. 8.8 - Prob. 4E Ch. 8.8 - Prob. 5E Ch. 8.8 - Prob. 6E Ch. 8.8 - Prob. 7E Ch. 8.8 - Prob. 8E Ch. 8.8 - Prob. 9E Ch. 8.8 - Prob. 10E Ch. 8.8 - Prob. 11E Ch. 8.8 - Prob. 12E Ch. 8.8 - Prob. 13E Ch. 8.8 - Prob. 14E Ch. 8.8 - Prob. 15E Ch. 8.8 - Prob. 16E Ch. 8.8 - Prob. 17E Ch. 8.8 - Prob. 18E Ch. 8.8 - Prob. 19E Ch. 8.8 - Prob. 20E Ch. 8.8 - Prob. 21E Ch. 8.8 - Prob. 22E Ch. 8.8 - Prob. 23E Ch. 8.8 - Prob. 24E Ch. 8.8 - Prob. 25E Ch. 8.8 - Prob. 26E Ch. 8.8 - Prob. 27E Ch. 8.8 - Prob. 28E Ch. 8.8 - Prob. 29E Ch. 8.8 - Prob. 30E Ch. 8.8 - Prob. 31E Ch. 8.8 - Prob. 32E Ch. 8.8 - Prob. 33E Ch. 8 - Prob. 1RCC Ch. 8 - Prob. 2RCC Ch. 8 - Prob. 3RCC Ch. 8 - Prob. 4RCC Ch. 8 - Prob. 5RCC Ch. 8 - Prob. 6RCC Ch. 8 - Prob. 7RCC Ch. 8 - Prob. 8RCC Ch. 8 - Prob. 9RCC Ch. 8 - Prob. 10RCC Ch. 8 - Prob. 11RCC Ch. 8 - Prob. 12RCC Ch. 8 - Prob. 1RQ Ch. 8 - Prob. 2RQ Ch. 8 - Prob. 3RQ Ch. 8 - Prob. 4RQ Ch. 8 - Prob. 5RQ Ch. 8 - Prob. 6RQ Ch. 8 - Prob. 7RQ Ch. 8 - Prob. 8RQ Ch. 8 - Prob. 9RQ Ch. 8 - Prob. 10RQ Ch. 8 - Prob. 11RQ Ch. 8 - Prob. 12RQ Ch. 8 - Prob. 13RQ Ch. 8 - Prob. 14RQ Ch. 8 - Prob. 15RQ Ch. 8 - Prob. 16RQ Ch. 8 - Prob. 17RQ Ch. 8 - Prob. 18RQ Ch. 8 - Prob. 19RQ Ch. 8 - Prob. 20RQ Ch. 8 - Prob. 1RE Ch. 8 - Prob. 2RE Ch. 8 - Prob. 3RE Ch. 8 - Prob. 4RE Ch. 8 - Prob. 5RE Ch. 8 - Prob. 6RE Ch. 8 - Prob. 7RE Ch. 8 - Prob. 8RE Ch. 8 - Prob. 9RE Ch. 8 - Prob. 10RE Ch. 8 - Prob. 11RE Ch. 8 - Prob. 12RE Ch. 8 - Prob. 13RE Ch. 8 - Prob. 14RE Ch. 8 - Prob. 15RE Ch. 8 - Prob. 16RE Ch. 8 - Prob. 17RE Ch. 8 - Prob. 18RE Ch. 8 - Prob. 19RE Ch. 8 - Prob. 20RE Ch. 8 - Prob. 21RE Ch. 8 - Prob. 22RE Ch. 8 - Prob. 23RE Ch. 8 - Prob. 24RQ Ch. 8 - Prob. 25RQ Ch. 8 - Prob. 26RQ Ch. 8 - Prob. 27RQ Ch. 8 - Prob. 28RQ Ch. 8 - Prob. 29RQ Ch. 8 - Prob. 30RQ Ch. 8 - Prob. 31RQ Ch. 8 - Prob. 32RQ Ch. 8 - Prob. 33RQ Ch. 8 - Prob. 34RQ Ch. 8 - Prob. 35RQ Ch. 8 - Prob. 36RQ Ch. 8 - Prob. 37RQ Ch. 8 - Prob. 38RQ Ch. 8 - Prob. 39RQ Ch. 8 - Prob. 40RQ Ch. 8 - Prob. 41RQ Ch. 8 - Prob. 42RQ Ch. 8 - Prob. 43RQ Ch. 8 - Prob. 44RQ Ch. 8 - Prob. 45RQ Ch. 8 - Prob. 46RQ Ch. 8 - Prob. 47RQ Ch. 8 - Prob. 48RQ Ch. 8 - Prob. 49RQ Ch. 8 - Prob. 50RQ Ch. 8 - Prob. 1P Ch. 8 - Prob. 2P Ch. 8 - Prob. 3P Ch. 8 - Prob. 4P Ch. 8 - Prob. 5P Ch. 8 - Prob. 6P Ch. 8 - Prob. 7P Ch. 8 - Prob. 8P Ch. 8 - Prob. 9P Ch. 8 - Prob. 10P Ch. 8 - Prob. 11P Ch. 8 - Prob. 12P Ch. 8 - Prob. 14P Ch. 8 - Prob. 15P Ch. 8 - Prob. 16P

Knowledge Booster

$Calculus$

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.

That 1 2 x n + x n + 1 + x n + 2 + x n + 3 = 2 , and find a similar equation for the y -coordinates.

Concept explainers

Videos

Answer to Problem 12P

Explanation of Solution

To find: The coordinate of P<m:math><m:mi>P</m:mi></m:math> .

Answer to Problem 12P

Explanation of Solution

Chapter 8 Solutions

To find: The coordinate of $P$ .