Chapter 6.2, Problem 47E

a.

To determine

To find: The possible values of T . Also, compute the probabilities that T can take and show these probabilities in a table.

Explanation of Solution

Given:

  

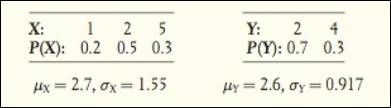
Calculation:

The possible values of T are:

  $\begin{array}{l}1+2=3\\ 1+4=5\\ 2+2=4\\ 2+4=6\end{array}$

And,

  $\begin{array}{l}5+2=7\\ 5+4=9\end{array}$

The probabilities can be calculated as:

  $\begin{array}{l}P\left(T=3\right)=P\left(X=1\right)\times P\left(Y=2\right)=0.2\left(0.7\right)=0.14\\ P\left(T=4\right)=P\left(X=2\right)\times P\left(Y=2\right)=0.5\left(0.7\right)=0.35\\ P\left(T=5\right)=P\left(X=1\right)\times P\left(Y=4\right)=0.2\left(0.3\right)=0.06\\ P\left(T=6\right)=P\left(X=2\right)\times P\left(Y=4\right)=0.5\left(0.3\right)=0.15\end{array}$

Similarly,

  $\begin{array}{l}P\left(T=7\right)=P\left(X=5\right)\times P\left(Y=2\right)=0.3\left(0.7\right)=0.21\\ P\left(T=9\right)=P\left(X=5\right)\times P\left(Y=4\right)=0.3\left(0.3\right)=0.09\end{array}$

Thus, the table is:

Value	Probability
3	0.14
4	0.35
5	0.06
6	0.15
7	0.21
9	0.09

b.

To determine

To show: The mean of T is equal to ${\mu }_X+{\mu }_Y$

Explanation of Solution

The mean of T be calculated as:

  $\begin{array}{c}{\mu }_T={\displaystyle \sum x\times P\left(x\right)}\\ =3\left(0.14\right)+4\left(0.35\right)+5\left(0.06\right)+6\left(0.15\right)+7\left(0.21\right)+9\left(0.09\right)\\ =5.3\end{array}$

Now,

  $\begin{array}{c}{\mu }_X+{\mu }_Y=2.7+2.6\\ =5.3\end{array}$

Hence, proved.

c.

To determine

To confirm: Whether ${\sigma }_T^2={\sigma }_X^2+{\sigma }_Y^2$ . Also, show that ${\sigma }_T={\sigma }_X+{\sigma }_Y$

Explanation of Solution

The variance of T be calculated as:

  $\begin{array}{c}{\sigma }_T=\sqrt{{\displaystyle \sum x^2\times P\left(x\right)-{\left({\displaystyle \sum x\times P\left(x\right)}\right)}^2}}\\ =\sqrt{\left(3^2\times 0.14+4^2\times 0.35+\mathrm{.......}+9^2\times 0.09\right)-{\left(5.3\right)}^2}\\ =3.25\end{array}$

Now,

  $\begin{array}{c}{\sigma }_X^2+{\sigma }_Y^2={1.55}^2+{0.917}^2\\ =3.2433\end{array}$

It could be shown as:

  $\begin{array}{c}{\sigma }_T={\sigma }_X+{\sigma }_Y\\ =1.55+0.917\\ =2.467\end{array}$

Thus, it could be concluded that ${\sigma }_T\ne {\sigma }_X+{\sigma }_Y$