Chapter 6.2, Problem 17E

To determine

To calculate: The integral ${\displaystyle {\int }_{-2}^1\left|x\right|}dx$ with the help of the graph of the integrand.

Answer to Problem 17E

The value of the integral ${\displaystyle {\int }_{-2}^1\left|x\right|}dx$ is $\frac{5}{2}\text{units}$ .

Explanation of Solution

Given:

The integral is ${\displaystyle {\int }_{-2}^1\left|x\right|}dx$ .

Concept used:

If a nonnegative function $y=f\left(x\right)$ is integrable over the interval $\left[a,b\right]$ , then the area $A$ lies below the curve $y=f\left(x\right)$ that is bounded from $a\text{ to }b$ is equal to the integral of $f$ from a to b that can be mathematically expressed as $A={\displaystyle {\int }_a^{b}f\left(x\right)dx}$ .

Formula used:

The area of the triangle is as follows:

  $A=\frac{1}{2}\cdot b\cdot h$

Here, the expression $b$ denotes the base of the triangle and h denotes the height of the triangle.

Calculation:

In the given integral, the integrand function is $f\left(x\right)=\left|x\right|$ that would be two straight lines as the function $f\left(x\right)=\left|x\right|$ is defined as

  $\left|x\right|=\{\begin{array}{c}x,x\ge 0\\ -x,x<0\end{array}$

For values of x greater than or equal to 0 the value of function will be positive and for the values of x less than 0 the value of function will be negative.

  $f\left(x\right)=x$ is a straight line passing through center and having coordinates (1, 1), (2, 2) etc.

  $f\left(x\right)=-x$ is a straight line passing through center and having coordinates (-1, -1), (-2, -2) etc.

Now draw the graph of $f\left(x\right)=\left|x\right|$ and make bounds of $x=-2\text{ and }x=1$ as shown in Figure 1.

  

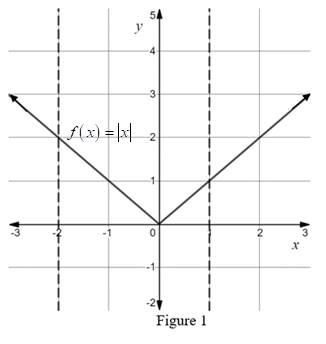
It can be seen that the area under a function is two triangles. The height of the left triangle is 2 units and the base is 2 units.

The height of the right triangle is on the left side is 1 unit and the length of the base is 1 unit.

The area of the region bounded by the $f\left(x\right)=\left|x\right|$ , $x=-2\text{ and }x=1$ is the sum of area of two triangles.

Use the formula of the triangle to obtain the area of the triangle.

  $\begin{array}{c}A=\frac{1}{2}\cdot 2\cdot 2+\frac{1}{2}\cdot 1\cdot 1\\ =2+\frac{1}{2}\\ =\frac{5}{2}\end{array}$

Therefore, by the concept the value of the integral is equal to the area of the sum of two triangles.

The value of the integral ${\displaystyle {\int }_{-2}^1\left|x\right|}dx$ is $\frac{5}{2}\text{units}$ .

Conclusion:

Thus, the value of the integral ${\displaystyle {\int }_{-2}^1\left|x\right|}dx$ is $\frac{5}{2}\text{units}$ .