Chapter 6.2, Problem 10P

(a)

To determine

Find the z interval for $x<30$.

Answer to Problem 10P

The z interval for $x<30$ is $z<0.65$.

Explanation of Solution

Calculation:

Z score:

The number of standard deviations the original measurement x is from the value of mean $\mu$ is measured using the z-score or z value. The formula for z score is,

$z=\frac{x-\mu }{\sigma }$

In the formula, x is the raw score, $\mu$ is the mean and $\sigma$ is the standard deviation.

The variable x is the weight of a fawn in kilograms. The weight of the body is normally distributed with mean $\mu =27.2\text{kilograms}$ and standard deviation $\sigma =4.3\text{kilograms}$. Then the z score is,

$z=\frac{x-27.2}{4.3}$

The z interval is,

Consider $x<30$

Subtract 27.2 on both sides of the inequality.

$\begin{array}{c}x-27.2<30-27.2\\ x-27.2<2.8\end{array}$

Divide 4.3 on both sides of the inequality.

$\begin{array}{c}\frac{x-27.2}{4.3}<\frac{2.8}{4.3}\\ z<0.65\end{array}$

Hence, the z interval for $x<30$ is $z<0.65$.

(b)

To determine

Find the z interval for $19<x$.

Answer to Problem 10P

The z interval for $19<x$ is $z>-1.91$.

Explanation of Solution

Calculation:

For the z interval consider,

$\begin{array}{c}19<x\\ x>19\end{array}$

Subtract 27.2 on both sides of the inequality.

$\begin{array}{c}x-27.2>19-27.2\\ x-27.2>-8.2\end{array}$

Divide 4.3 on both sides of the inequality.

$\begin{array}{c}\frac{x-27.2}{4.3}>\frac{-8.2}{4.3}\\ z>-1.91\end{array}$

Hence, the z interval for $19<x$ is $z>-1.91$.

(c)

To determine

Find the z interval for $32<x<35$.

Answer to Problem 10P

The z interval for $32<x<35$ is $1.12<z<1.81$.

Explanation of Solution

Calculation:

For the z interval consider,

$32<x<35$

Subtract 27.2 for each part of the inequality.

$\begin{array}{c}32-27.2<x-27.2<35-27.2\\ 4.8<x<7.8\end{array}$

Divide 4.3 for each part of the inequality.

$\begin{array}{c}\frac{4.8}{4.3}<\frac{x-27.2}{4.3}<\frac{7.8}{4.3}\\ 1.12<z<1.81\end{array}$

Hence, the z interval for $32<x<35$ is $1.12<z<1.81$.

(d)

To determine

Find the x interval for $-2.17<z$.

Answer to Problem 10P

The x interval for $-2.17<z$ is $x>17.9$.

Explanation of Solution

Calculation:

The z score is,

$\begin{array}{l}z=\frac{x-27.2}{4.3}\\ x=27.2+4.3z\end{array}$

For the x interval consider,

$\begin{array}{c}-2.17<z\\ z>-2.17\end{array}$

Multiply 4.3 on both sides of the inequality.

$\begin{array}{c}4.3z>-2.17\left(4.3\right)\\ 4.3z>-9.331\end{array}$

Add 27.2 on both sides of the inequality.

$\begin{array}{c}27.2+4.3z>-9.331+27.2\\ x>17.9\end{array}$

Hence, the x interval for $-2.17<z$ is $x>17.9$.

(e)

To determine

Find the x interval for $z<1.28$.

Answer to Problem 10P

The x interval for $z<1.28$ is $x<32.7$.

Explanation of Solution

Calculation:

For the x interval consider,

$z<1.28$

Multiply 4.3 on both sides of the inequality.

$\begin{array}{c}4.3z<1.28\left(4.3\right)\\ 4.3z<5.504\end{array}$

Add 27.2 on both sides of the inequality.

$\begin{array}{c}27.2+4.3z<5.504+27.2\\ x<32.7\end{array}$

Hence, the x interval for $z<1.28$ is $x<32.7$.

(f)

To determine

Find the x interval for $-1.99<z<1.44$.

Answer to Problem 10P

The x interval for $-1.99<z<1.44$ is $18.6<x<33.4$.

Explanation of Solution

Calculation:

For the x interval consider,

$-1.99<z<1.44$

Multiply 4.3 for each part of the inequality.

$\begin{array}{c}-1.99\left(4.3\right)<4.3z<1.44\left(4.3\right)\\ -8.557<4.3z<6.192\end{array}$

Add 27.2 for each part of the inequality.

$\begin{array}{c}-8.557+27.2<27.2+4.3z<27.2+6.192\\ 18.6<x<33.4\end{array}$

Hence, the x interval for $-1.99<z<1.44$ is $18.6<x<33.4$.

(g)

To determine

Identify whether the fawn weighing 14 kilograms is unusually small animal or not using z values and Figure 6-15.

Answer to Problem 10P

Yes, the fawn weighing 14 kilograms is unusually small animal.

Explanation of Solution

Calculation:

The weight of the fawn is 14 kilograms. The z score is,

$\begin{array}{c}z=\frac{x-27.2}{4.3}\\ =\frac{14-27.2}{4.3}\\ =\frac{-13.2}{4.3}\\ =-3.07\end{array}$

The weight of the fawn is 14 kilograms is 3.07 standard deviations below the mean value. The z score value is less than –3 indicating that the value is very unusual.

The figure 6-15 is the standard normal distribution curve. The z value is located on the curve as below.

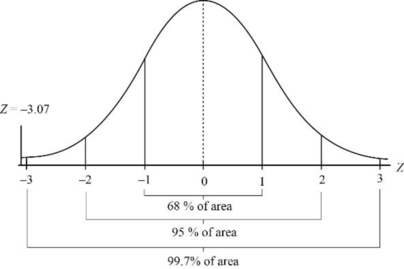
The z value that is far from the mean (zero) is considered as unusual. From the figure it can be observed that z value is very far from the value of mean indicating that it is very unusual. This shows that, fawn weighing 14 kilograms is unusually very small animal.

(h)

To determine

Explain whether the value of z for weight of the fawn would be closer to 0, –2, or 3 when fawn is unusually large.

Answer to Problem 10P

The value of z for weight of the fawn would be closer to 3 when fawn is unusually large.

Explanation of Solution

The z value that is far from the mean (zero) is considered as unusual. If the value is closer to –3 is usually very small and value closer to 3 is usually very large.

If the value of z for weight of the fawn is closer to 3 then the weight of the fawn usually would be very large.