Chapter 6, Problem 6P

To determine

Find the equations for slope and deflection of the beam using direct integration method.

Answer to Problem 6P

For segment AB:

The equation for slope is $\underset{\_}{\frac{PaL}{6EI}\left(1-\frac{3x^2}{L^2}\right)}$.

The equation for deflection is $\underset{\_}{\frac{PaxL}{6EI}\left(1-\frac{x^2}{L^2}\right)}$.

For segment BC:

The equation for slope is $\underset{\_}{\frac{PL}{EI}\left(\frac{x^2}{2L}-x\left(1+\frac{a}{L}\right)+\frac{L}{2}+\frac{2a}{3}\right)}$.

The equation for deflection is $\underset{\_}{\frac{PL}{EI}\left[\frac{x^3}{6L}-\frac{x^2}{2}\left(1+\frac{a}{L}\right)+x\left(\frac{L}{2}+\frac{2a}{3}\right)-\frac{L}{6}\left(L+a\right)\right]}$.

Explanation of Solution

Calculation:

Consider flexural rigidity EI of the beam is constant.

Draw the free body diagram of the beam as in Figure (1).

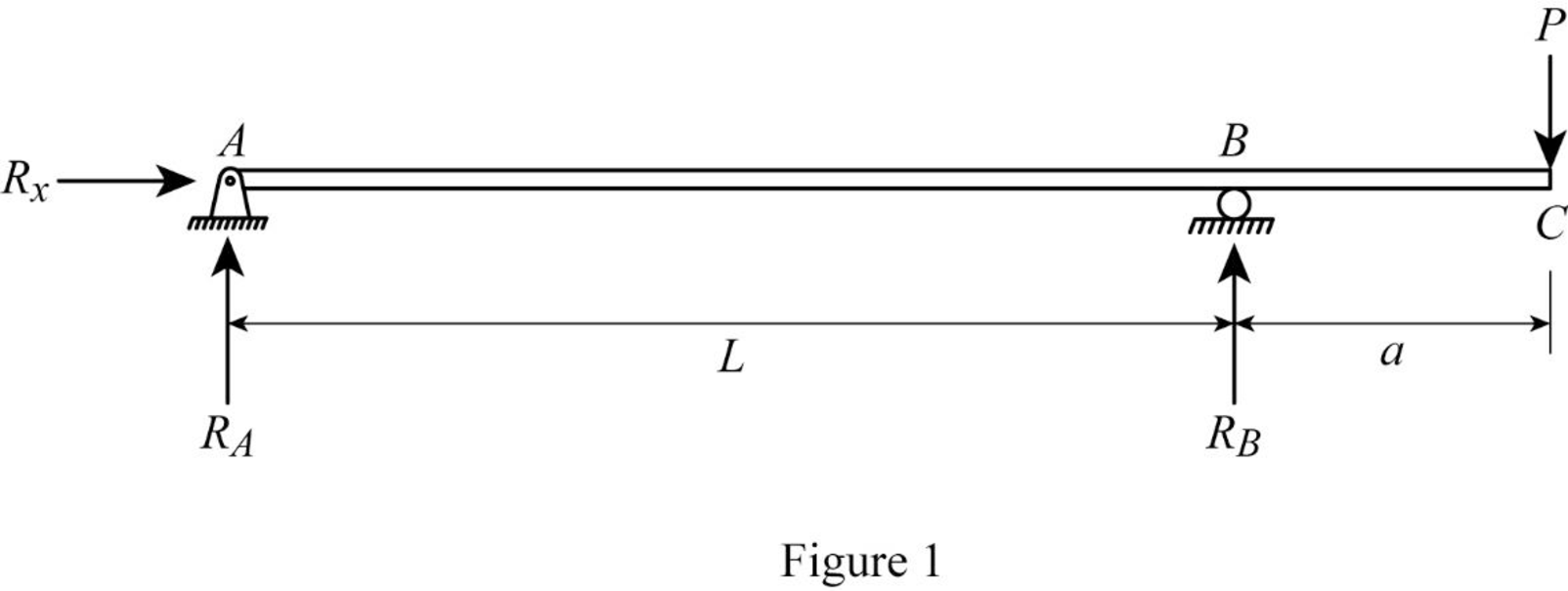
Refer Figure (1),

Consider upward force is positive and downward force is negative.

Consider clockwise  is negative and counterclowise is positive.

Determine the support reaction at A using the relation;

$\begin{array}{l}{\displaystyle \sum M_B=0}\\ -R_A\times L-\left(P\times a\right)=0\\ R_A=-\frac{Pa}{L}\\ R_A=\frac{Pa}{L}(\downarrow )\end{array}$

Determine the support reaction at B using the relation;

$\begin{array}{l}{\displaystyle \sum V=0}\\ R_A+R_B-P=0\\ R_B=P-\left(-\frac{Pa}{L}\right)\\ R_B=\frac{PL+Pa}{L}\\ R_B=P\left(1+\frac{a}{L}\right)\end{array}$

Show the reaction values as in Figure (2).

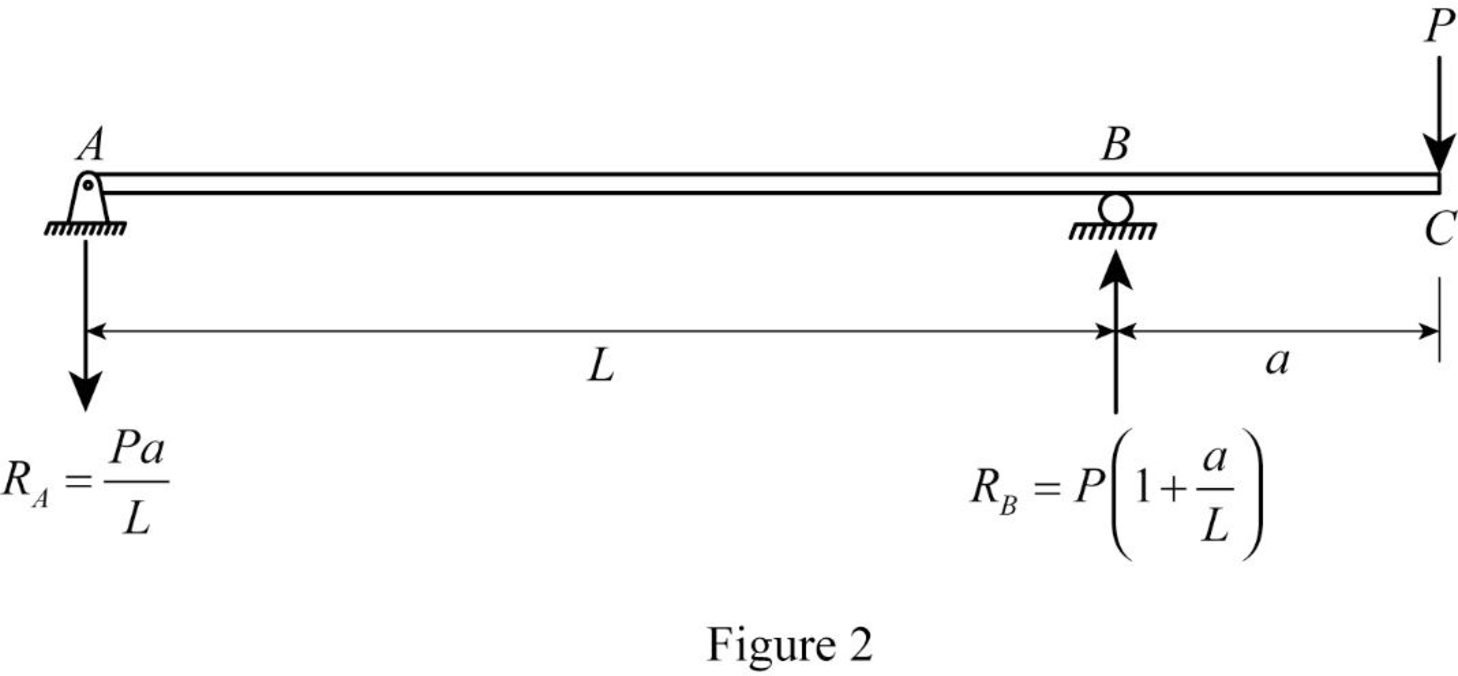
Take a section at a distance of x.

Show the section as in Figure (2).

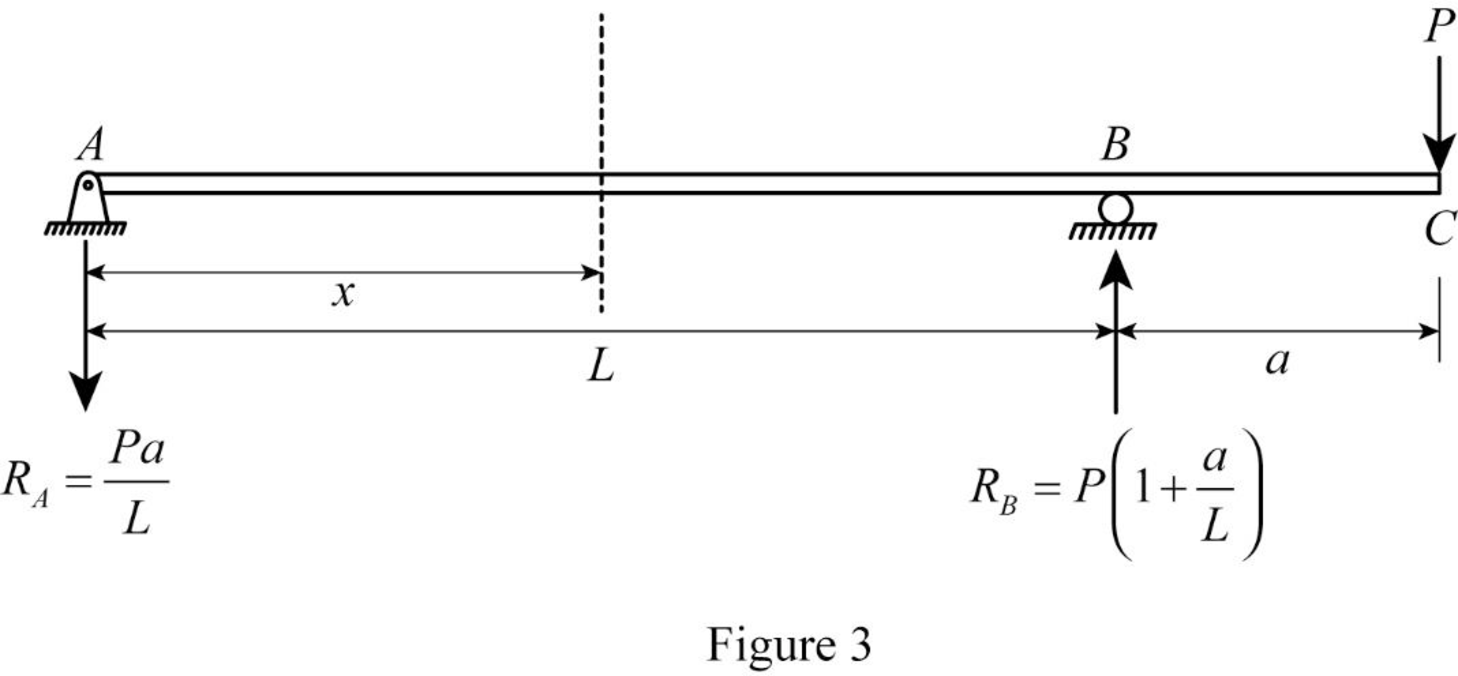
Consider the segment AB:

Refer Figure (2),

Write the equation for bending moment at x distance.

$\begin{array}{c}{M}_x=-R_A\left(x\right)\\ =-\frac{Pa}{L}\left(x\right)\\ =-\frac{Pax}{L}\end{array}$

Write the equation for $\frac{M}{EI}$ as follows:

$\frac{d^{2}y}{d{x}^2}=\frac{1}{EI}\left(-\frac{Pax}{L}\right)$        (1)

Write the equation for slope as follows:

Integrate Equation (1) with respect to x.

$\begin{array}{c}\frac{dy}{dx}=\theta =-{\displaystyle \int \frac{1}{EI}\left(\frac{Pa}{L}\right)\left(xdx\right)}\\ =-\frac{Pa}{EIL}\left(\frac{x^2}{2}\right)+C_1\end{array}$        (2)

Write the equation for deflection as follows:

Integrate Equation (2) with respect to x.

$\begin{array}{c}y={\displaystyle \int \left[-\frac{Pa}{EIL}\left(\left(\frac{x^2}{2}\right)\right)+C_1\right]}dx\\ =-\frac{Pa}{2EIL}\left(\frac{x^3}{3}\right)+C_{1}x+C_2\end{array}$        (3)

Find the integration constants $C_1\text{and}{C}_2$:

Write the boundary conditions as follows:

$\text{At}x=0;y=0$:

Apply the above boundary conditions in Equation (3):

$\begin{array}{l}0=-\frac{Pa}{2EIL}\left(\frac{\left(0^3\right)}{3}\right)+C_1\left(0\right)+C_2\\ C_2=0\end{array}$

Write the boundary conditions as follows:

$\text{At}x=L;y=0$:

Apply the above boundary conditions in Equation (3):

$\begin{array}{l}0=-\frac{Pa}{2EIL}\left(\frac{L^3}{3}\right)+C_1\left(L\right)+0\\ C_1\left(L\right)=\frac{Pa{L}^2}{6EI}\\ C_1=\frac{PaL}{6EI}\end{array}$

Find the equation for slope.

Substitute $\frac{PaL}{6EI}$ for $C_1$ in Equation (2).

$\begin{array}{c}\theta =-\frac{Pa}{EIL}\left(\frac{x^2}{2}\right)+\frac{PaL}{6EI}\\ =\frac{PaL}{6EI}\left(1-\frac{3x^2}{L^2}\right)\end{array}$

Thus, the equation for slope is $\underset{\_}{\frac{PaL}{6EI}\left(1-\frac{3x^2}{L^2}\right)}$.

Find the equation for deflection.

Substitute $\frac{PaL}{6EI}$ for $C_1$ and 0 for $C_2$ in Equation (3).

$\begin{array}{c}y=-\frac{Pa}{2EIL}\left(\frac{x^3}{3}\right)+\left(\frac{PaL}{6EI}\right)x+0\\ =-\frac{Pa}{2EIL}\left(\frac{x^3}{3}\right)+\left(\frac{PaxL}{6EI}\right)\\ =\frac{PaxL}{6EI}\left(1-\frac{x^2}{L^2}\right)\end{array}$

Thus, the equation for deflection is $\underset{\_}{\frac{PaxL}{6EI}\left(1-\frac{x^2}{L^2}\right)}$.

Consider segment BC;

Show the distance at a distance of x as in Figure (4).

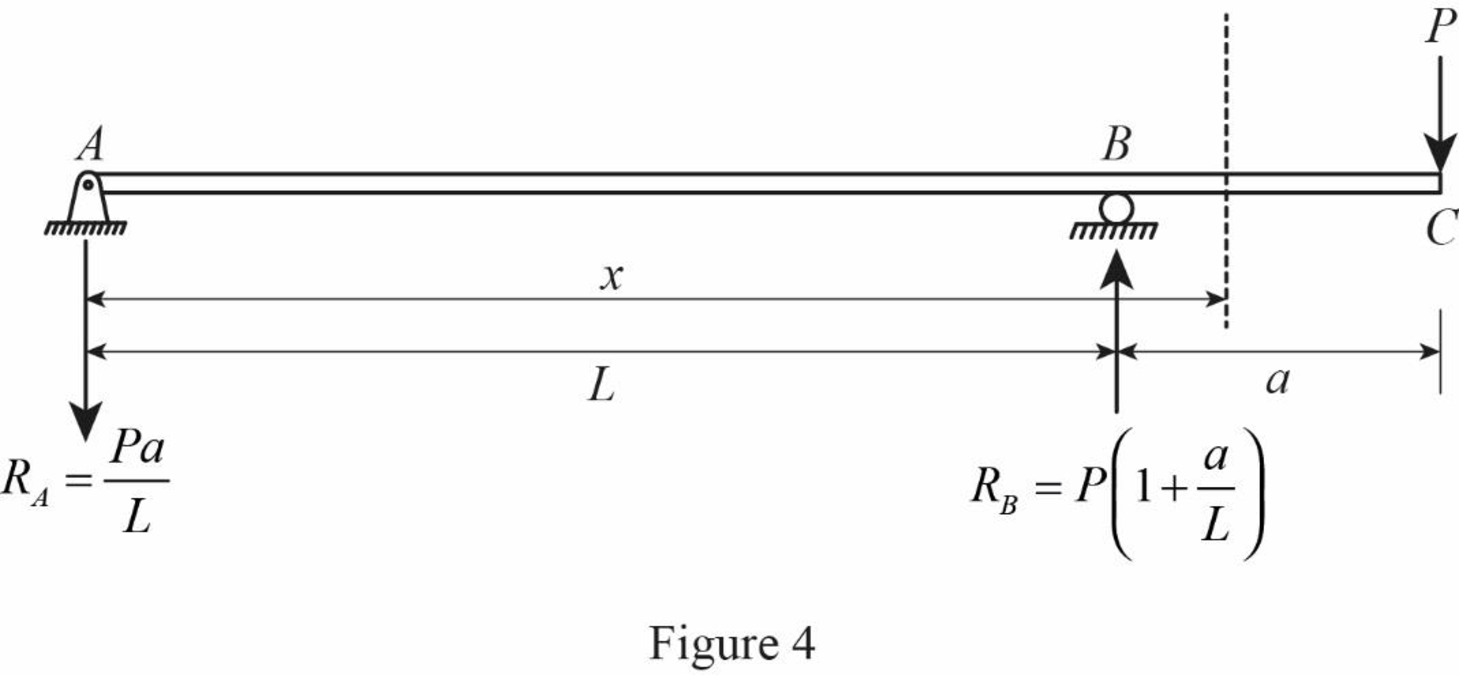
Refer Figure (2),

For segment BC the limit should be $L\le x\le \left(L+a\right)$.

Write the equation for bending moment at x distance.

$M_x=-P\left(L+a-x\right)$

Write the equation for $\frac{M}{EI}$ as follows:

$\begin{array}{c}\frac{d^{2}y}{d{x}^2}=\frac{1}{EI}\left(-P\left(L+a-x\right)\right)\\ =-\frac{P}{EI}\left(L+a-x\right)\end{array}$        (4)

Write the equation for slope as follows:

Integrate Equation (4) with respect to x.

$\begin{array}{c}\frac{dy}{dx}=\theta =-{\displaystyle \int \frac{P}{EI}\left(L+a-x\right)}dx\\ =-\frac{P}{EI}\left(Lx+ax-\frac{x^2}{2}\right)+C_3\end{array}$        (5)

Write the equation for deflection as follows:

Integrate Equation (5) with respect to x.

$\begin{array}{c}y={\displaystyle \int \left[-\frac{P}{EI}\left(Lx+ax-\frac{x^2}{2}\right)+C_3\right]}dx\\ =-\frac{P}{EI}\left(\frac{L{x}^2}{2}+\frac{a{x}^2}{2}-\frac{x^3}{6}\right)+C_{3}x+C_4\end{array}$        (6)

Write the boundary conditions as follows:

$\text{At}x=L$

The slope at left and right of support B is equal.

${\theta }_{AB}={\theta }_{BC}$

Substitute $\frac{PaL}{6EI}\left(1-\frac{3x^2}{x^2}\right)$ for ${\theta }_{AB}$ and $-\frac{P}{EI}\left(Lx+ax-\frac{x^2}{2}\right)+C_3$ for ${\theta }_{BC}$.

${\left[\frac{PaL}{6EI}\left(1-\frac{3x^2}{x^2}\right)\right]}_{x=L}={\left[-\frac{P}{EI}\left(Lx+ax-\frac{x^2}{2}\right)+C_3\right]}_{x=L}$

Apply the above boundary conditions in the above Equation

$\begin{array}{l}\frac{PaL}{6EI}\left(1-\frac{3L^2}{L^2}\right)=-\frac{P}{EI}\left(L\times L+aL-\frac{L^2}{2}\right)+C_3\\ -\frac{PaL}{6EI}\left(-2\right)=-\frac{P}{EI}\left(\frac{L^2}{2}+aL\right)+C_3\\ C_3=\frac{PaL}{3EI}+\frac{P{L}^2}{2EI}-\frac{PaL}{EI}\\ C_3=\frac{PL}{EI}\left(\frac{a}{3}+\frac{L}{2}-a\right)\end{array}$

$C_3=\frac{PL}{EI}\left(\frac{2a}{3}+\frac{L}{2}\right)$

Substitute $\frac{PL}{EI}\left(\frac{2a}{3}+\frac{L}{2}\right)$ for $C_3$ in Equation (5).

$\begin{array}{c}\theta =-\frac{P}{EI}\left(Lx+ax-\frac{x^2}{2}\right)+\frac{PL}{EI}\left(\frac{2a}{3}+\frac{L}{2}\right)\\ =\frac{PL}{EI}\left(\frac{2a}{3}+\frac{L}{2}-x-\frac{ax}{L}+\frac{x^2}{2L}\right)\\ =\frac{PL}{EI}\left(\frac{2a}{3}+\frac{L}{2}-x\left(1+\frac{a}{L}\right)+\frac{x^2}{2L}\right)\\ =\frac{PL}{EI}\left(\frac{x^2}{2L}-x\left(1+\frac{a}{L}\right)+\frac{L}{2}+\frac{2a}{3}\right)\end{array}$

Hence, the Equation for slope is $\underset{\_}{\frac{PL}{EI}\left(\frac{x^2}{2L}-x\left(1+\frac{a}{L}\right)+\frac{L}{2}+\frac{2a}{3}\right)}$.

Write the boundary conditions as follows:

$\text{At}x=L;y=0$:

Apply the above boundary conditions in Equation (6):

$\begin{array}{l}-\frac{P}{EI}\left(\frac{L\times L^2}{2}+\frac{a{L}^2}{2}-\frac{L^3}{6}\right)+\left(\frac{PL}{EI}\left(\frac{2a}{3}+\frac{L}{2}\right)\right)L+C_4=0\\ -\frac{P}{EI}\left(\frac{L^3}{3}+\frac{a{L}^2}{2}\right)+\frac{P{L}^2}{EI}\left(\frac{2a}{3}+\frac{L}{2}\right)+C_4=0\\ -\frac{P{L}^2}{EI}\left(\frac{L}{3}+\frac{a}{2}-\frac{2a}{3}-\frac{L}{2}\right)+C_4=0\\ -\frac{P{L}^2}{EI}\left(-\frac{L}{6}-\frac{a}{6}\right)+C_4=0\end{array}$

$\begin{array}{l}\frac{P{L}^2}{EI}\left(\frac{L}{6}+\frac{a}{6}\right)+C_4=0\\ C_4=-\frac{P{L}^2}{6EI}\left(L+a\right)\end{array}$

Substitute $\frac{PL}{EI}\left(\frac{2a}{3}+\frac{L}{2}\right)$ for $C_3$ and $-\frac{P{L}^2}{6EI}\left(L+a\right)$ for $C_4$ in Equation (6).

$\begin{array}{c}y=-\frac{P}{EI}\left(\frac{L{x}^2}{2}+\frac{a{x}^2}{2}-\frac{x^3}{6}\right)+\frac{PL}{EI}\left(\frac{2a}{3}+\frac{L}{2}\right)x-\frac{P{L}^2}{6EI}\left(L+a\right)\\ =-\frac{PL}{EI}\left(\frac{L{x}^2}{2}+\frac{a{x}^2}{2}-\frac{x^3}{6}\right)+\frac{PL}{EI}\left(\frac{2a}{3}+\frac{L}{2}\right)x-\frac{P{L}^2}{6EI}\left(L+a\right)\\ =\frac{PL}{EI}\left[\frac{x^3}{6L}-\frac{x^2}{2}\left(1+\frac{a}{L}\right)+x\left(\frac{L}{2}+\frac{2a}{3}\right)-\frac{L}{6}\left(L+a\right)\right]\end{array}$

Hence, the Equation for deflection is $\underset{\_}{\frac{PL}{EI}\left[\frac{x^3}{6L}-\frac{x^2}{2}\left(1+\frac{a}{L}\right)+x\left(\frac{L}{2}+\frac{2a}{3}\right)-\frac{L}{6}\left(L+a\right)\right]}$.