Calculus Volume 3
16th Edition
ISBN: 9781938168079
Author: Gilbert Strang, Edwin Jed Herman
Publisher: OpenStax
expand_more
expand_more
format_list_bulleted
Concept explainers
Videos
Textbook Question
Chapter 5.5, Problem 2SP
Hot air balloons
Rot all ballooning is a relaxing. peaceful pastime that many people enjoy. Many balloonist gatherings take place around the world, Such as the Albuquerque International Balloon Fiesta. The Albuquerque event is the largest hot air balloon festival in the world, with ovet 500 balloons participating each year.
Figure 5.61 Balloons lift off at the 2001 Albuquerque international Balloon Fiesta. (credit: David Herrera, Flickr)
As the name implies, hot air balloons use hot air to generate lift. (Hot air is less dense than cooler air, so the balloon floats as long as the hot air stays hot.) The heat is generated by a propane burner suspended below the opening of the basket. Once the balloon takes off, the pilot controls the altitude of the balloon, either by using the burner to heat the air and ascend or by using a vent near the top of the balloon to release heated air and descend. The pilot has very little control over where the balloon goes, however—balloons are at the mercy of the winds. The uncertainty over where we will end up is one of the reasons balloonists are attracted to the sport.
In this project we use tnple integrals to learn more about hot air balloons. We model the balloon in two pieces. The top of the balloon is modeled by a half sphere of radius 28 feet. The bottom of the balloon is modeled by a fnistum of a cone (think of an ice cream cone with the pointy end cut off). The radius of the large end of the fnistum is 28 feet and the radius of the small end of the fnistum is 6 feet. A graph of our balloon model and a cross-sectional diagram showing the dimensions are shown in the following figure.
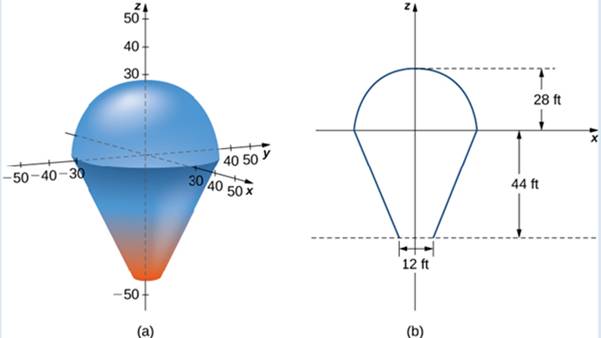
FIgure 5.62 (a)Useahalfsphetetomodeltheroppartoftheballoonandafrnsnunofaconeomodel
the bonom pan of the balloon. (b) A cross section of the balloon shong its dimensions.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
HELP PLS. I have no idea how to do this. I need to do page 196 and 197 using data from page 200. Can you please write it down and explain so I can understand
Accounting
please Only reply if you are going to help with full
question.
A paper investigated the driving behavior of teenagers by observing their vehicles as they left a high school parking lot and
then again at a site approximately mile from the school. Assume that it is reasonable to regard the teen drivers in this
study as representative of the population of teen drivers.
Amount by Which Speed
Limit Was Exceeded
Female
Driver
Male
Driver
1.3
-0.2
1.3
0.5
0.9
1.1
2.1
0.7
0.7
1.1
1.3
1.2
3
0.1
1.3
0.9
0.6
0.5
2.1
0.5
(a) Use a .01 level of significance for any hypothesis tests. Data consistent with summary quantities appearing in the paper
are given in the table. The measurements represent the difference between the observed vehicle speed and the posted
speed limit (in miles per hour) for a sample of male teenage drivers and a sample of female teenage drivers. (Use
Hmales Hremales Round your test statistic to two decimal places. Round your degrees of freedom down to the nearest…
easure.
Chapter 5 Solutions
Calculus Volume 3
Ch. 5.1 - In the following exercises, use the midpoint rule...Ch. 5.1 - In the following exercises, use the midpoint rule...Ch. 5.1 - In the following exercises, estimate the volume of...Ch. 5.1 - In the following exercises, estimate the volume of...Ch. 5.1 - In the following exercises, estimate the volume of...Ch. 5.1 - In the following exercises, estimate the volume of...Ch. 5.1 - In the following exercises, estimate the volume of...Ch. 5.1 - In the following exercises, estimate the volume of...Ch. 5.1 - In the following exercises, estimate the volume of...Ch. 5.1 - In the following exercises, estimate the volume of...
Ch. 5.1 - In the following exercises, estimate the volume of...Ch. 5.1 - In the following exercises, estimate the volume of...Ch. 5.1 - In the following exercises, calculate the...Ch. 5.1 - In the following exercises, calculate the...Ch. 5.1 - In the following exercises, calculate the...Ch. 5.1 - In the following exercises, calculate the...Ch. 5.1 - In the following exercises, calculate the...Ch. 5.1 - In the following exercises, calculate the...Ch. 5.1 - In the following exercises, calculate the...Ch. 5.1 - In the following exercises, calculate the...Ch. 5.1 - In the following exercises, evaluate the iterated...Ch. 5.1 - In the following exercises, evaluate the iterated...Ch. 5.1 - In the following exercises, evaluate the iterated...Ch. 5.1 - In the following exercises, evaluate the iterated...Ch. 5.1 - In the following exercises, evaluate the iterated...Ch. 5.1 - In the following exercises, evaluate the iterated...Ch. 5.1 - In the following exercises, evaluate the iterated...Ch. 5.1 - In the following exercises, evaluate the iterated...Ch. 5.1 - In the following exercises, evaluate the iterated...Ch. 5.1 - In the following exercises, evaluate the iterated...Ch. 5.1 - In the following exercises, evaluate the iterated...Ch. 5.1 - In the following exercises, evaluate the iterated...Ch. 5.1 - In the following exercises, evaluate the iterated...Ch. 5.1 - In the following exercises, evaluate the iterated...Ch. 5.1 - function over the given rectangles. 35....Ch. 5.1 - function over the given rectangles. 36....Ch. 5.1 - function over the given rectangles. 37....Ch. 5.1 - function over the given rectangles. 38....Ch. 5.1 - Let f and g be two continuous functions such that...Ch. 5.1 - In the following exercises, use property y. of...Ch. 5.1 - In the following exercises, use property y. of...Ch. 5.1 - In the following exercises, use property y. of...Ch. 5.1 - In the following exercises, use property y. of...Ch. 5.1 - Let f and g be two continuous functions such that...Ch. 5.1 - In the following exercises, use property y. of...Ch. 5.1 - In the following exercises, use property y. of...Ch. 5.1 - In the following exercises, use property y. of...Ch. 5.1 - In the following exercises, use property y. of...Ch. 5.1 - In the following exercises, the function f is...Ch. 5.1 - In the following exercises, the function f is...Ch. 5.1 - In the following exercises, the function f is...Ch. 5.1 - In the following exercises, the function f is...Ch. 5.1 - [T] Consider the function f(x,y)=ex2y2where...Ch. 5.1 - [T] Consider the function f(x,y)=sin(x2)cos(y2) ....Ch. 5.1 - In the following exercises, the functions fnare...Ch. 5.1 - In the following exercises, the functions fnare...Ch. 5.1 - In the following exercises, the functions fnare...Ch. 5.1 - In the following exercises, the functions fnare...Ch. 5.1 - An isotherm map is a chart connecting points...Ch. 5.2 - In the following exercises, specify whether the...Ch. 5.2 - In the following exercises, specify whether the...Ch. 5.2 - In the following exercises, specify whether the...Ch. 5.2 - In the following exercises, specify whether the...Ch. 5.2 - wIn the following exercises, specify whether the...Ch. 5.2 - In the following exercises, specify whether the...Ch. 5.2 - In the following exercises, specify whether the...Ch. 5.2 - In the following exercises, specify whether the...Ch. 5.2 - In the following exercises, specify whether the...Ch. 5.2 - In the following exercises, specify whether the...Ch. 5.2 - In the following exercises, specify whether the...Ch. 5.2 - In the following exercises, specify whether the...Ch. 5.2 - In the following exercises, specify whether the...Ch. 5.2 - In the following exercises, specify whether the...Ch. 5.2 - In the following exercises, evaluate the double...Ch. 5.2 - In the following exercises, evaluate the double...Ch. 5.2 - In the following exercises, evaluate the double...Ch. 5.2 - In the following exercises, evaluate the double...Ch. 5.2 - In the following exercises, evaluate the double...Ch. 5.2 - In the following exercises, evaluate the double...Ch. 5.2 - Evaluate the iterated integrals. 80. 012x3x(x+ y...Ch. 5.2 - Evaluate the iterated integrals. 81....Ch. 5.2 - Evaluate the iterated integrals. 82....Ch. 5.2 - Evaluate the iterated integrals. 83....Ch. 5.2 - Evaluate the iterated integrals. 84. 01 1 y 2 1 y...Ch. 5.2 - Evaluate the iterated integrals. 85. 01/2 14 y 2...Ch. 5.2 - Evaluate the iterated integrals. 86. Let D be the...Ch. 5.2 - Evaluate the iterated integrals. 87. Let D be the...Ch. 5.2 - yEvaluate the iterated integrals. 88. a. Show that...Ch. 5.2 - Evaluate the iterated integrals. 89. a. Show that...Ch. 5.2 - The region D bounded by x=0,y=x5+1 , and S y=3x2...Ch. 5.2 - The legion D bounded by y = cos x. y = 4 cos x....Ch. 5.2 - Find the area A(D) of the region...Ch. 5.2 - Let D be the region bounded by y = 1, y = x. y =...Ch. 5.2 - Find the average value of the function f(x. y) =...Ch. 5.2 - Find the average value of the function f(x. y) =-x...Ch. 5.2 - In the following exercises, change the order of...Ch. 5.2 - In the following exercises, change the order of...Ch. 5.2 - In the following exercises, change the order of...Ch. 5.2 - In the following exercises, change the order of...Ch. 5.2 - The region D is shown in the following figure....Ch. 5.2 - The region D is given in the following figure....Ch. 5.2 - Find the volume of the solid under the surface...Ch. 5.2 - Find the volume of the solid tinder the plane...Ch. 5.2 - Find the volume of the solid tinder the plane z=xy...Ch. 5.2 - Find the volume of the solid under the surface z =...Ch. 5.2 - Let g be a positive, increasing, and...Ch. 5.2 - Let g be a positive, increasing, and...Ch. 5.2 - Find the volume of the solid situated in the first...Ch. 5.2 - Find the volume of the solid situated in the first...Ch. 5.2 - Find the volume of the solid bounded by the planes...Ch. 5.2 - Find the volume of the solid bounded by the planes...Ch. 5.2 - Let S1 and S2 , be the solids situated in the...Ch. 5.2 - Let S and 5, be the solids situated in the first...Ch. 5.2 - Let S1 and S2 be the solids situated in the first...Ch. 5.2 - Let S1 and S2 be the solids situated in the first...Ch. 5.2 - [T] The following figure shows the region D...Ch. 5.2 - [T] The region D bounded by the curves y=cosx,x=0...Ch. 5.2 - Suppose that (X. Y) is the outcome of an...Ch. 5.2 - Consider X and Y two random variables of...Ch. 5.2 - [T] The Reuleaux triangle consists of an...Ch. 5.2 - [T] Show that the area of the lunes of Alhazen,...Ch. 5.3 - In the following exercises, express the region D...Ch. 5.3 - In the following exercises, express the region D...Ch. 5.3 - In the following exercises, express the region D...Ch. 5.3 - In the following exercises, express the region D...Ch. 5.3 - In the following exercises, express the region D...Ch. 5.3 - In the following exercises, express the region D...Ch. 5.3 - In the following exercises, the graph of the polar...Ch. 5.3 - In the following exercises, the graph of the polar...Ch. 5.3 - In the following exercises, the graph of the polar...Ch. 5.3 - In the following exercises, the graph of the polar...Ch. 5.3 - In the following exercises, the graph of the polar...Ch. 5.3 - In the following exercises, the graph of the polar...Ch. 5.3 - In the following exercises, evaluate the double...Ch. 5.3 - In the following exercises, evaluate the double...Ch. 5.3 - In the following exercises, evaluate the double...Ch. 5.3 - In the following exercises, evaluate the double...Ch. 5.3 - In the following exercises, evaluate the double...Ch. 5.3 - In the following exercises, evaluate the double...Ch. 5.3 - In the following exercises, evaluate the double...Ch. 5.3 - In the following exercises, evaluate the double...Ch. 5.3 - In the following exercises, evaluate the double...Ch. 5.3 - In the following exercises, evaluate the double...Ch. 5.3 - In the following exercises, the integrals have...Ch. 5.3 - In the following exercises, the integrals have...Ch. 5.3 - In the following exercises, the integrals have...Ch. 5.3 - In the following exercises, the integrals have...Ch. 5.3 - In the following exercises, convert the integrals...Ch. 5.3 - In the following exercises, convert the integrals...Ch. 5.3 - In the following exercises, convert the integrals...Ch. 5.3 - In the following exercises, convert the integrals...Ch. 5.3 - Evaluate the integral DffrdAwhere D is the region...Ch. 5.3 - Find the area of the region D bounded by the polar...Ch. 5.3 - Evaluate the integral DrdA, where D is the region...Ch. 5.3 - Find the total area of the region enclosed by the...Ch. 5.3 - Find the area of the region D, which is the region...Ch. 5.3 - Find the area of the region D. which is the region...Ch. 5.3 - Determine the average value of the function f(x....Ch. 5.3 - Determine the average value of the function...Ch. 5.3 - Find the volume of the solid situated in the first...Ch. 5.3 - Find the volume of the solid bounded by the...Ch. 5.3 - a. Find the volume of the solid S1 bounded by the...Ch. 5.3 - a. Find the volume of the solid S1 inside the unit...Ch. 5.3 - For the following two exercises, consider a...Ch. 5.3 - For the following two exercises, consider a...Ch. 5.3 - Find the volume of the solid that lies tinder the...Ch. 5.3 - Find the volume of the solid that lies under the...Ch. 5.3 - Find the volume of the solid that lies under the...Ch. 5.3 - Find the volume of the solid that lies under the...Ch. 5.3 - A radial function f is a function whose value at...Ch. 5.3 - Use the information from the preceding exercise to...Ch. 5.3 - Let f(x,y)=F(r)rbe a continuous radial function...Ch. 5.3 - Apply the preceding exercise to calculate the...Ch. 5.3 - Let f be a continuous function that can be...Ch. 5.3 - Apply the preceding exercise to calculate the...Ch. 5.3 - Let f be a continuous function that can be...Ch. 5.3 - Evaluate Dff arctan (yx)x2+y2dA. where...Ch. 5.3 - A spherical cap is the region of a sphere that...Ch. 5.3 - In statistics, the joint density for two...Ch. 5.3 - The double improper integral e( x2 +y 2/2 )dxdymay...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, change the order of...Ch. 5.4 - In the following exercises, change the order of...Ch. 5.4 - In the following exercises, change the order of...Ch. 5.4 - In the following exercises, change the order of...Ch. 5.4 - Let F. G and H be continuous functions on [a,b]...Ch. 5.4 - Let F. G. and H be differential functions on...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - In the following exercises, evaluate the triple...Ch. 5.4 - The solid E bounded by y2+z2=9,x=0 . x = 5 is...Ch. 5.4 - The solid E bounded by y=x,x=4,y=0 , and z = 1 is...Ch. 5.4 - [T] The volume of a solid E is given by the...Ch. 5.4 - [T] The volume of a solid E is given by the...Ch. 5.4 - In the following exercises, use two circular...Ch. 5.4 - In the following exercises, use two circular...Ch. 5.4 - In the following exercises, use two circular...Ch. 5.4 - In the following exercises, use two circular...Ch. 5.4 - Set up the integral that gives the volume of the...Ch. 5.4 - Set up the integral that gives the volume of the...Ch. 5.4 - Find the average value of the function f(x. y, z)...Ch. 5.4 - Find the average value of the function...Ch. 5.4 - Find the volume of the solid E that lies under the...Ch. 5.4 - Find the volume of the solid E that lies under the...Ch. 5.4 - Consider the pyramid with the base in the xv...Ch. 5.4 - Consider the pyramid with the base in the xy...Ch. 5.4 - The solid E bounded by the sphere of equation...Ch. 5.4 - The solid E bounded by the equation 9x2+4y2+z2=1...Ch. 5.4 - Find the volume of the prism with vertices (0, 0....Ch. 5.4 - Find the volume of the prism with vertices (0. 0....Ch. 5.4 - The solid E bounded by z= 10—2x—y and situated in...Ch. 5.4 - The solid E bounded by z=1x2 and situated in the...Ch. 5.4 - The midpoint rule for the triple integral...Ch. 5.4 - [T] a. Apply the midpoint rule to approximate...Ch. 5.4 - Suppose that the temperature in degrees Celsius at...Ch. 5.4 - Suppose that the temperature in degrees Fahrenheit...Ch. 5.4 - Show that the volume of a right square pyramid of...Ch. 5.4 - Show that the volume of a regular right hexagonal...Ch. 5.4 - Show that the volume of a regular right hexagonal...Ch. 5.4 - If the charge density at an arbitraiy point (x, y....Ch. 5.5 - Hot air balloons Rot all ballooning is a relaxing....Ch. 5.5 - Hot air balloons Rot all ballooning is a relaxing....Ch. 5.5 - Hot air balloons Rot all ballooning is a relaxing....Ch. 5.5 - In the following exercises, evaluate the triple...Ch. 5.5 - In the following exercises, evaluate the triple...Ch. 5.5 - In the following exercises, evaluate the triple...Ch. 5.5 - In the following exercises, evaluate the triple...Ch. 5.5 - In the following exercises, evaluate the triple...Ch. 5.5 - In the following exercises, evaluate the triple...Ch. 5.5 - a. Let B be a cylindrical shell with inner radius...Ch. 5.5 - a. Let B be a cylindrical shell with inner radius...Ch. 5.5 - In the following exercises, the boundaries of the...Ch. 5.5 - In the following exercises, the boundaries of the...Ch. 5.5 - In the following exercises, the boundaries of the...Ch. 5.5 - In the following exercises, the boundaries of the...Ch. 5.5 - In the following exercises, the function f and...Ch. 5.5 - In the following exercises, the function f and...Ch. 5.5 - In the following exercises, the function f and...Ch. 5.5 - In the following exercises, the function f and...Ch. 5.5 - In the following exercises, find the volume of the...Ch. 5.5 - In the following exercises, find the volume of the...Ch. 5.5 - In the following exercises, find the volume of the...Ch. 5.5 - In the following exercises, find the volume of the...Ch. 5.5 - In the following exercises, find the volume of the...Ch. 5.5 - In the following exercises, find the volume of the...Ch. 5.5 - In the following exercises, find the volume of the...Ch. 5.5 - In the following exercises, find the volume of the...Ch. 5.5 - [T] Use a computer algebra system (CAS) to graph...Ch. 5.5 - [T] Use a CAS to graph the solid whose volume is...Ch. 5.5 - 267. Convert the integral into an integral in...Ch. 5.5 - Convert the integral 020x 01 ( xy+z) dzdxdy into...Ch. 5.5 - f(x,y,z)=1,B={(x,y,z)x2+y2+z290,z0}Ch. 5.5 - 270. f(x,y,z)=1x2+y2+z2,B={(x,y,z)x2+y2+z29,y0,z0}Ch. 5.5 - f(x,y,z)=x2+y2. B is bounded above by the...Ch. 5.5 - f(x. y, z) = z. B is bounded above by the half...Ch. 5.5 - Show that if F(,,)=f()g()h() is a continuous...Ch. 5.5 - a. A function F is said to have spherical svmmetiy...Ch. 5.5 - a. Let B be the region between the upper...Ch. 5.5 - In the following exercises, the function f and...Ch. 5.5 - In the following exercises, the function f and...Ch. 5.5 - In the following exercises, the function f and...Ch. 5.5 - In the following exercises, the function f and...Ch. 5.5 - In the following exercises, find the volume of the...Ch. 5.5 - In the following exercises, find the volume of the...Ch. 5.5 - Use spherical coordinates to find the volume of...Ch. 5.5 - Use spherical coordinates to find the volume of...Ch. 5.5 - Convert the integral f44f16 y 216y2f16 x 2 y...Ch. 5.5 - Convert the integral 2f24 x 2f4x2 x 2+ y...Ch. 5.5 - Convert the integral 2f24 x 2f4x2 x 2+ y...Ch. 5.5 - [T] Use a CAS to graph the solid whose volume is...Ch. 5.5 - [T] Use a CAS to graph the solid whose volume is...Ch. 5.5 - [T] Use a CAS to evaluate the integral...Ch. 5.5 - [T] a. Evaluate the integral Ee x 2 + y 2 + z 2...Ch. 5.5 - Express the volume of the solid inside the sphere...Ch. 5.5 - Express the volume of the solid inside the sphere...Ch. 5.5 - The power emitted by an antenna has a power...Ch. 5.5 - Use the preceding exercise to find the total power...Ch. 5.5 - A charge cloud contained in a sphere B of radius r...Ch. 5.5 - Use the preceding exercise to find the total...Ch. 5.6 - In the following exercises, the region R occupied...Ch. 5.6 - In the following exercises, the region R occupied...Ch. 5.6 - In the following exercises, the region R occupied...Ch. 5.6 - In the following exercises, the region R occupied...Ch. 5.6 - In the following exercises, the region R occupied...Ch. 5.6 - In the following exercises, the region R occupied...Ch. 5.6 - In the following exercises, the region R occupied...Ch. 5.6 - In the following exercises, the region R occupied...Ch. 5.6 - In the following exercises, the region R occupied...Ch. 5.6 - In the following exercises, the region R occupied...Ch. 5.6 - In the following exercises, the region R occupied...Ch. 5.6 - In the following exercises, the region R occupied...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - In the following exercises, consider a lamina...Ch. 5.6 - Let Q be the solid unit cube. Find the mass of the...Ch. 5.6 - Let Q be the solid unit hemisphere. Find the mass...Ch. 5.6 - The solid Q of constant density I is situated...Ch. 5.6 - Find the mass of the solid...Ch. 5.6 - Consider the solid Q={(x,y,z)0x1,0y2,0z3} with the...Ch. 5.6 - [T] The solid Q has the mass given by the triple N...Ch. 5.6 - The solid Q is bounded by the planes...Ch. 5.6 - The solid Q is bounded by the planes x+y+z=3 . and...Ch. 5.6 - Let Q be the solid situated outside the sphere...Ch. 5.6 - The mass of a solid is given by 0f20f4x2 x 2+ y...Ch. 5.6 - Let Q be the solid bounded above the cone x2+y2=z2...Ch. 5.6 - The solid Q={(x,y,z)0x2+y216,x0,y0,0zx} has the...Ch. 5.6 - The solid Q is bounded by the cylinder + = a2. the...Ch. 5.6 - Let Q be a solid of constant density k. where k >...Ch. 5.6 - The solid Q has the mass given by the triple...Ch. 5.6 - The solid Q has the moment of inertia Ixabout...Ch. 5.6 - The solid Q has the mass given by the triple...Ch. 5.6 - A solid Q has a volume given by DabdAdz. where D...Ch. 5.6 - Consider the solid enclosed by the cylinder...Ch. 5.6 - [T] The average density of a solid Q is defined as...Ch. 5.6 - Show that the moments of inertia Ix,Iy. and...Ch. 5.7 - In the following exercises, the function...Ch. 5.7 - In the following exercises, the function...Ch. 5.7 - In the following exercises, the function...Ch. 5.7 - In the following exercises, the function...Ch. 5.7 - In the following exercises, the function...Ch. 5.7 - In the following exercises, the function...Ch. 5.7 - In the following exercises, determine whether...Ch. 5.7 - In the following exercises, determine whether...Ch. 5.7 - In the following exercises, determine whether...Ch. 5.7 - In the following exercises, determine whether...Ch. 5.7 - In the following exercises, determine whether...Ch. 5.7 - In the following exercises, determine whether...Ch. 5.7 - In the following exercises, the transformations...Ch. 5.7 - In the following exercises, the transformations...Ch. 5.7 - In the following exercises, the transformations...Ch. 5.7 - In the following exercises, the transformations...Ch. 5.7 - In the following exercises, the transformations...Ch. 5.7 - In the following exercises, the transformations...Ch. 5.7 - In the following exercises, the transformation...Ch. 5.7 - In the following exercises, the transformation...Ch. 5.7 - In the following exercises, the transformation...Ch. 5.7 - In the following exercises, the transformation...Ch. 5.7 - In the following exercises, find the Jacobian J of...Ch. 5.7 - In the following exercises, find the Jacobian J of...Ch. 5.7 - In the following exercises, find the Jacobian J of...Ch. 5.7 - In the following exercises, find the Jacobian J of...Ch. 5.7 - In the following exercises, find the Jacobian J of...Ch. 5.7 - In the following exercises, find the Jacobian J of...Ch. 5.7 - In the following exercises, find the Jacobian J of...Ch. 5.7 - In the following exercises, find the Jacobian J of...Ch. 5.7 - In the following exercises, find the Jacobian J of...Ch. 5.7 - In the following exercises, find the Jacobian J of...Ch. 5.7 - The triangular region R with the vertices...Ch. 5.7 - The triangular region R with the vertices (0, 0)....Ch. 5.7 - In the following exercises, use the transformation...Ch. 5.7 - In the following exercises, use the transformation...Ch. 5.7 - In the following exercises, use the transformation...Ch. 5.7 - In the following exercises, use the transformation...Ch. 5.7 - In the following exercises, use the transformation...Ch. 5.7 - In the following exercises, use the transformation...Ch. 5.7 - In the following exercises, use the transformation...Ch. 5.7 - In the following exercises, use the transformation...Ch. 5.7 - The circular annulus sector R bounded by the...Ch. 5.7 - The solid R bounded by the circular cylinder...Ch. 5.7 - Show that Rf( x 2 3 + y 2 3 )dA=21501f()dp. where...Ch. 5.7 - Show that Rf( 16 x 2 +4y+ x 2 )dv=201f()2dp. where...Ch. 5.7 - [T] Find the area of the region bounded by the...Ch. 5.7 - [T] Find the area of the region bounded by the...Ch. 5.7 - Evaluate the triple integral...Ch. 5.7 - Evaluate the triple integral...Ch. 5.7 - A transformation T:R2R2,T(u,v)=(x,y)of the form x...Ch. 5.7 - The transformation T:R2T(u,v)=(x,y) . where...Ch. 5.7 - [T] Find the region S in the uv-plane whose image...Ch. 5.7 - [T] The transformations T : R P. i = 1,.... 4....Ch. 5.7 - [T] The transformation...Ch. 5.7 - [T] Find transformations...Ch. 5.7 - Use the transformation, x=au,y=av,z=cw and...Ch. 5.7 - Find the volume of a football whose shape is a...Ch. 5.7 - [T] Lamé ovals (or superellipses) are plane curves...Ch. 5.7 - [T] Lamé ovals have been consistently used by...Ch. 5 - True or False? Justify your answer with a proof or...Ch. 5 - True or False? Justify your answer with a proof or...Ch. 5 - True or False? Justify your answer with a proof or...Ch. 5 - True or False? Justify your answer with a proof or...Ch. 5 - True or False? Justify your answer with a proof or...Ch. 5 - True or False? Justify your answer with a proof or...Ch. 5 - True or False? Justify your answer with a proof or...Ch. 5 - True or False? Justify your answer with a proof or...Ch. 5 - True or False? Justify your answer with a proof or...Ch. 5 - True or False? Justify your answer with a proof or...Ch. 5 - True or False? Justify your answer with a proof or...Ch. 5 - True or False? Justify your answer with a proof or...Ch. 5 - True or False? Justify your answer with a proof or...Ch. 5 - For the following problems, find the specified...Ch. 5 - For the following problems, find the specified...Ch. 5 - For the following problems, find the specified...Ch. 5 - For the following problems, find the specified...Ch. 5 - For the following problems, find the center of...Ch. 5 - For the following problems, find the center of...Ch. 5 - For the following problems, find the center of...Ch. 5 - For the following problems, find the center of...Ch. 5 - The following problems examine Mount Holly in the...Ch. 5 - The following problems examine Mount Holly in the...Ch. 5 - The following problems consider the temperature...Ch. 5 - [T] The density of Earth’s layers is displayed in...Ch. 5 - The following problems concern the Theorem of...Ch. 5 - The following problems concern the Theorem of...
Additional Math Textbook Solutions
Find more solutions based on key concepts
For a population containing N=902 individual, what code number would you assign for a. the first person on the ...
Basic Business Statistics, Student Value Edition
In Exercises 17–20, refer to the sample of body temperatures (degrees Fahrenheit) in table below. (The body tem...
Elementary Statistics (13th Edition)
Show that 34=12 using each of the following models. a. Repeated-addition number line b. Rectangular array c. Ar...
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Find T, N, and κ for the plane curves in Exercises 1–4.
3. r(t) = (2t + 3)i + (5 − t2)j
University Calculus: Early Transcendentals (4th Edition)
Disks/washers about the y-axis Let R be the region bounded by the following curves. Use the disk or washer meth...
Calculus: Early Transcendentals (2nd Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- 2 please give me the full solution and answer( this is for my reviewer only) thank youarrow_forwardDiscribe a specific time in real life where a population being surveyed is appropriate. What data are you trying to collect, and give reasons why surveying the population is appropriate. 1. Describe the situation 2. Support why surveying the population is appropriate, using details.arrow_forwardA cylinder with a 7-centimeter radius and a height of 10 cm is submerged in a tank of water that is 50 cm wide and 40 cm long (see the figure below). How much does the water level rise? Round to the nearest hundredth of a centimeter.arrow_forward
- MOVEABLE PECES m%3D Positive, Negative, Zero, Undefined? PRACTICE I Help Improve Office - Noarrow_forwardI need help solving this.arrow_forwardDownload the file interceptor.ipynb. Instructions how to download notebook files are posted here. Open this file in Jupyter Notebook and play the interceptor game. Once you win, enter below the data displayed on the final game screen. You can also click on the Binder button below to launch an interactive version of the interceptor notebook. The game may take a a couple minutes to load and it may run slowly in Binder. launch binder Enter the intial target position in the form [p1, p2]: 50 40- 30- 20- 10- time: 3.20 target distance: 211.95 missile en route 0 -300 -200 -100 0 100 200 300 Select missile launch time toarrow_forward
- login.i-ready.com/student/dashboard/home A bicycle tire is 28 inches in diameter. Approximately how far does the bicycle move forward each time the wheel goes around? (Use 4 as an approximation for .) 88 inches 44 inches 64 inches 56 inches Done -> My Progress 62% complete < Copynight 2021 by Currioulum Associates. All rights reserved. These materials, or any portion thereof, may not be eproduced or shared in any manner yoo o 11:25 AM P Type here to search 5/5/2021arrow_forwardSection A Practice Prabiemsarrow_forwardAn architect creates a 3-dimensional scale model of a building. The model requires 1,200 square centimeters of siding. The model is 20 centimeters tall. The real building will be 10 meters tall. How much siding will be required for the real building? O 200 square meters O 300 square meters O 600 square meters O 1,200 square metersarrow_forward
- Hi please answer d & e. Please show full solution. Thank you!arrow_forwardPlease box answer and write it in the same format as the question. Do not type it out only hand written. Chapter 2.4 , Question 5arrow_forwardA playhouse includes a 15-foot slide, and due to safety regulations, the base of the slide is 12 feet from the base of the playhouse structure. 15 feet x feet 12 feet How tall is the top of the slide? O 1.7 feet O 3 feet O 9 feet O 19.2 feet A Calculatorarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Algebra: Structure And Method, Book 1AlgebraISBN:9780395977224Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. ColePublisher:McDougal Littell
Holt Mcdougal Larson Pre-algebra: Student Edition...
Algebra
ISBN:9780547587776
Author:HOLT MCDOUGAL
Publisher:HOLT MCDOUGAL
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Big Ideas Math A Bridge To Success Algebra 1: Stu...
Algebra
ISBN:9781680331141
Author:HOUGHTON MIFFLIN HARCOURT
Publisher:Houghton Mifflin Harcourt
Algebra: Structure And Method, Book 1
Algebra
ISBN:9780395977224
Author:Richard G. Brown, Mary P. Dolciani, Robert H. Sorgenfrey, William L. Cole
Publisher:McDougal Littell
Probability & Statistics (28 of 62) Basic Definitions and Symbols Summarized; Author: Michel van Biezen;https://www.youtube.com/watch?v=21V9WBJLAL8;License: Standard YouTube License, CC-BY
Introduction to Probability, Basic Overview - Sample Space, & Tree Diagrams; Author: The Organic Chemistry Tutor;https://www.youtube.com/watch?v=SkidyDQuupA;License: Standard YouTube License, CC-BY