
Concept explainers
In a process that manufactures bearings, 90% of the bearings meet a thickness specification. A shipment contains 500 bearings. A shipment is acceptable if at least 440 of the 500 bearings meet the specification. Assume that each shipment contains a random sample of bearings.
a. What is the
b. What is the probability that more than 285 out of 300 shipments are acceptable?
c. What proportion of bearings must meet the specification in order that 99% of the shipments are acceptable?
a.
Find the probability that a given shipment is acceptable.
Answer to Problem 11E
The probability that a given shipment is acceptableis 0.9418.
Explanation of Solution
Given info:
About 90% of the bearings meet a thickness specification. In total there are 500 bearings. If at least 440 of 500 bearings meet specification then the shipment is acceptable.
Calculation:
The non-defective bearings in a shipment is denoted as X. Then X follows Binomial with parameters
The normal distribution can be used to approximate the binomial distribution with,
Mean:
Substitute 500 for n and 0.9 for p in the above equation.
Standard deviation:
Substitute 500 for n and 0.9 for p in the above equation.
The approximate binomial probability using the normal distribution is,
By using continuity correction, the value 0.5 is subtracted from the value of 440.
Substitute 440 for a,
The formula to convert X values into z score is,
Substitute 450for np and6.7082for
The above probability can be obtained by finding the areas to the left of –1.57.
The shaded region represents the area to the right of –1.57 is shown below:
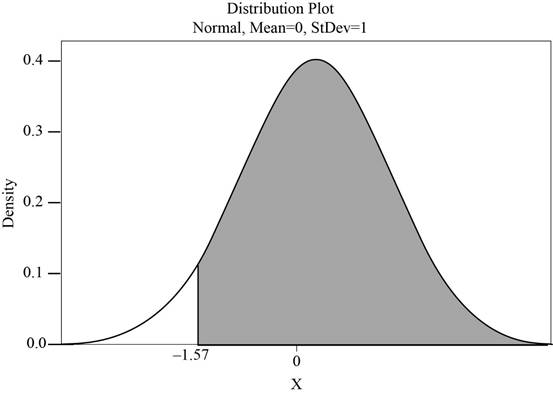
Use Table A.2: Cumulative Normal Distribution to find the area.
Procedure:
For z at –1.57,
- Locate –1.5 in the left column of the Table A.2.
- Obtain the value in the corresponding row below 0.07.
That is,
Then,
Thus, the probability that a given shipment is acceptable is 0.9418.
b.
Find probability that more than 285 out of 300 shipments are acceptable.
Answer to Problem 11E
The probability that more than 285 out of 300 shipments are acceptable is 0.2327.
Explanation of Solution
Calculation:
The random variable Y is defined as the number of shipments out of 300 that are acceptable.
From part (a), the probability that a given shipment is acceptable is 0.9418.
Then, the random variable Y follows binomial with
The normal distribution can be used to approximate the binomial distribution with,
Mean:
Substitute 300 for n and 0.9418 for p in the above equation.
Standard deviation:
Substitute n as 300 and 0.9418 for p in the above equation.
The approximate binomial probability using the normal distribution is,
By using continuity correction, the value 0.5 is added from the value of 285.
Substitute 285 for a,
The formula to convert X values into z score is,
Substitute 282.54for np and4.0551for
The above probability can be obtained by finding the areas to the left of 0.73.
The shaded region represents the area to the right of 0.73 is shown below:
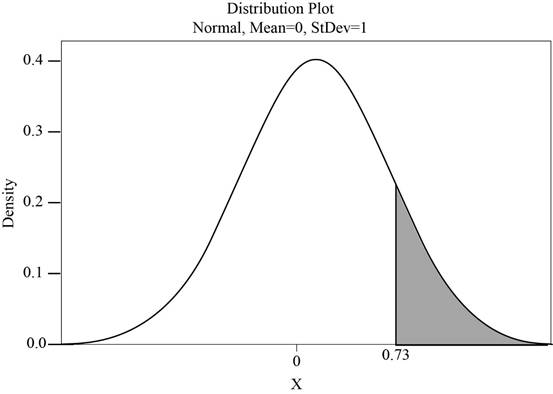
Use Table A.2: Cumulative Normal Distribution to find the area.
Procedure:
For z at 0.73,
- Locate0.7 in the left column of the Table A.2.
- Obtain the value in the corresponding row below 0.03.
That is,
Then,
Thus, the probability that more than 285 out of 300 shipments are acceptable is 0.2327.
c.
Find proportion of bearings must meet the specification in order that 99% of the shipments are acceptable.
Answer to Problem 11E
The proportion of bearings must meet the specification in order that 99% of the shipments are acceptable is 0.909.
Explanation of Solution
Calculation:
The required proportion of defective bearings is denoted as p and the number of defective bearings is denoted as X.
Then, the random variable X follows binomial with parameters
The normal distribution can be used to approximate the binomial distribution with,
Mean:
Substitute 500 for n in the above equation.
Standard deviation:
Substitute n as 500 in the above equation.
The probability that a shipment is acceptable is
The approximate binomial probability using the normal distribution is,
By using continuity correction, the value 0.5 is added from the value of 285.
Substitute 440 for a,
The formula to convert X values into z score is,
Substitute 500p for np and
The value of the random variable X for the top 0.99 is same as the value of X for the top 0.01. The area to the left of X is 0.01 and right of X is 0.99.
The shaded region represents of the area of 99% of the shipments that are acceptable is shown below:
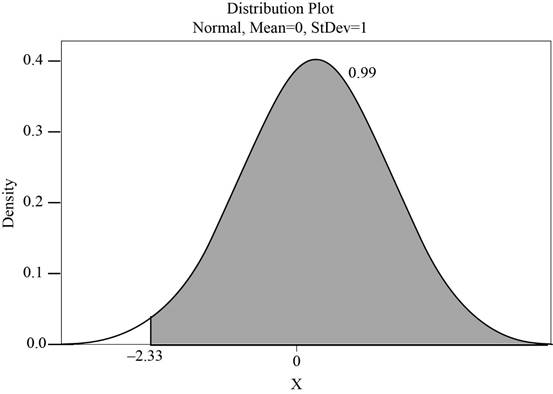
Use Table A.2: Cumulative Normal Distribution to find the critical value.
Procedure:
- Locate an approximate area of 0.0100 in the body of the A.2 table. The area closest to 0.0100 is 0.0099.
- Move left until the first column and note the value as –2.3.
- Move upward until the top row reached and note the value as 0.03.
Thus, the corresponding z-score is –2.33.
The z-score can be expressed in terms of p as follows:
Taking square on both sides,
Implies,
That is,
The formula for solving the quadratic equation of the form
Substitute
On solving this quadratic equation the value of p is,
Since 0.841 is a spurious root.
Thus, the proportion of bearings must meet the specification in order that 99% of the shipments are acceptable is 0.909.
Want to see more full solutions like this?
Chapter 4 Solutions
Statistics for Engineers and Scientists
- Please show as much work as possible to clearly show the steps you used to find each solution. If you plan to use a calculator, please be sure to clearly indicate your strategy. Consider the following game. It costs $3 each time you roll a six-sided number cube. If you roll a 6 you win $15. If you roll any other number, you receive nothing. a) Find the expected value of the game. b) If you play this game many times, will you expect to gain or lose money?arrow_forward= 12:02 WeBWorK / 2024 Fall Rafeek MTH23 D02 / 9.2 Testing the Mean mu / 3 38 WEBWORK Previous Problem Problem List Next Problem 9.2 Testing the Mean mu: Problem 3 (1 point) Test the claim that the population of sophomore college students has a mean grade point average greater than 2.2. Sample statistics include n = 71, x = 2.44, and s = 0.9. Use a significance level of a = 0.01. The test statistic is The P-Value is between : The final conclusion is < P-value < A. There is sufficient evidence to support the claim that the mean grade point average is greater than 2.2. ○ B. There is not sufficient evidence to support the claim that the mean grade point average is greater than 2.2. Note: You can earn partial credit on this problem. Note: You are in the Reduced Scoring Period. All work counts for 50% of the original. Preview My Answers Submit Answers You have attempted this problem 0 times. You have unlimited attempts remaining. . Oli wwm01.bcc.cuny.eduarrow_forwardThere are four white, fourteen blue and five green marbles in a bag. A marble is selected from the bag without looking. Find the odds of the following: The odds against selecting a green marble. The odds in favour of not selecting a green marble The odds in favor of the marble selected being either a white or a blue marble. What is true about the above odds? Explainarrow_forward
- Please show as much work as possible to clearly show the steps you used to find each solution. If you plan to use a calculator, please be sure to clearly indicate your strategy. 1. The probability of a soccer game in a particular league going into overtime is 0.125. Find the following: a. The odds in favour of a game going into overtime. b. The odds in favour of a game not going into overtime. c. If the teams in the league play 100 games in a season, about how many games would you expect to go into overtime?arrow_forwardexplain the importance of the Hypothesis test in a business setting, and give an example of a situation where it is helpful in business decision making.arrow_forwardA college wants to estimate what students typically spend on textbooks. A report fromthe college bookstore observes that textbooks range in price from $22 to $186. Toobtain a 95% confidence level for a confidence interval estimate to plus or minus $10,how many students should the college survey? (We may estimate the populationstandard deviation as (range) ÷ 4.)arrow_forward
- In a study of how students give directions, forty volunteers were given the task ofexplaining to another person how to reach a destination. Researchers measured thefollowing five aspects of the subjects’ direction-giving behavior:• whether a map was available or if directions were given from memory without a map,• the gender of the direction-giver,• the distances given as part of the directions,• the number of times directions such as “north” or “left” were used,• the frequency of errors in directions. Identify each of the variables in this study, and whether each is quantitative orqualitative. For each quantitative variable, state whether it is discrete or continuous. Was this an observational study or an experimental study? Explain your answer.arrow_forwardexplain the difference between the confident interval and the confident level. provide an example to show how to correctly interpret a confidence interval.arrow_forwardSketch to scale the orbit of Earth about the sun. Graph Icarus’ orbit on the same set of axesWhile the sun is the center of Earth’s orbit, it is a focus of Icarus’ orbit. There aretwo points of intersection on the graph. Based on the graph, what is the approximate distance between the two points of intersection (in AU)?arrow_forward
- The diameters of ball bearings are distributed normally. The mean diameter is 67 millimeters and the standard deviation is 3 millimeters. Find the probability that the diameter of a selected bearing is greater than 63 millimeters. Round to four decimal places.arrow_forwardSuppose you like to keep a jar of change on your desk. Currently, the jar contains the following: 22 Pennies 27 Dimes 9 Nickels 30 Quarters What is the probability that you reach into the jar and randomly grab a penny and then, without replacement, a dime? Express as a fraction or a decimal number rounded to four decimal places.arrow_forwardA box contains 14 large marbles and 10 small marbles. Each marble is either green or white. 9 of the large marbles are green, and 4 of the small marbles are white. If a marble is randomly selected from the box, what is the probability that it is small or white? Express as a fraction or a decimal number rounded to four decimal places.arrow_forward
 Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL
Holt Mcdougal Larson Pre-algebra: Student Edition...AlgebraISBN:9780547587776Author:HOLT MCDOUGALPublisher:HOLT MCDOUGAL College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning
College Algebra (MindTap Course List)AlgebraISBN:9781305652231Author:R. David Gustafson, Jeff HughesPublisher:Cengage Learning Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill



