
Concept explainers
A solid plastic sphere of radius R1 = 8.00 cm is concentric with an aluminum spherical shell with inner radius R2 = 14.0 cm and outer radius R3 = 17.0 cm (Fig. P25.67). Electric field measurements are made at two points: At a radial distance of 34.0 cm from the center, the electric field has magnitude 1.70 × 103 N/C and is directed radially outward, and at a radial distance of 12.0 cm from the center, the electric field has magnitude 9.10 × 104 N/C and is directed radially inward. What are the net charges on
- a. the plastic sphere and
- b. the aluminum spherical shell?
- c. What are the charges on the inner and outer surfaces of the aluminum spherical shell?
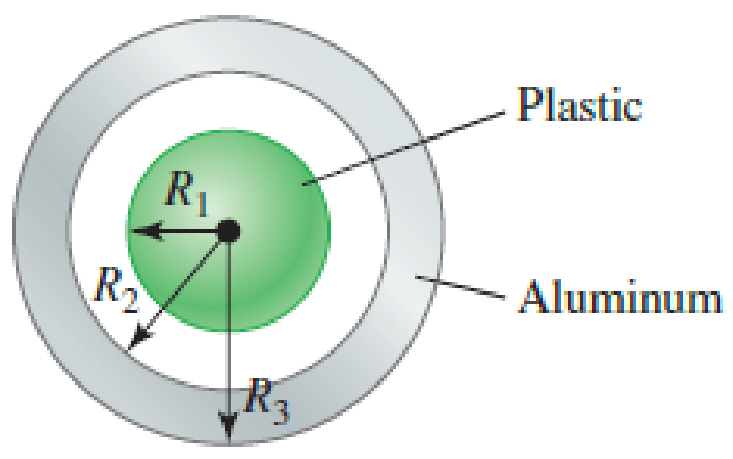
FIGURE P25.67
(a)
The net charge on the plastic sphere.
Answer to Problem 67PQ
The net charge on the plastic sphere is
Explanation of Solution
Write the expression for Gauss’s law.
Here,
Rewrite the above equation in terms of the volume charge density.
Here,
Substitute
Here,
Conclusion:
Rearrange the above equation to find the charge in the sphere.
Substitute
Therefore, the net charge on the plastic sphere is
(b)
The net charge on the aluminum spherical shell.
Answer to Problem 67PQ
The net charge on the aluminum spherical shell is
Explanation of Solution
Write the expression for total charge enclosed.
Conclusion:
Substitute
Substitute
Therefore, the net charge on the aluminum spherical shell is
(c)
The charge on the inner and outer surface of the aluminum spherical shell.
Answer to Problem 67PQ
The charge on the inner and outer surface of the aluminum spherical shell are
Explanation of Solution
Write the expression for charge on the inner surface of the aluminum spherical shell.
Write the expression for charge on the inner surface of the aluminum spherical shell.
Conclusion:
Substitute
Substitute
Therefore, the charge on the inner and outer surface of the aluminum spherical shell are
Want to see more full solutions like this?
Chapter 25 Solutions
Webassign Printed Access Card For Katz's Physics For Scientists And Engineers: Foundations And Connections, 1st Edition, Single-term
- The nonuniform charge density of a solid insulating sphere of radius R is given by = cr2 (r R), where c is a positive constant and r is the radial distance from the center of the sphere. For a spherical shell of radius r and thickness dr, the volume element dV = 4r2dr. a. What is the magnitude of the electric field outside the sphere (r R)? b. What is the magnitude of the electric field inside the sphere (r R)?arrow_forwardA total charge Q is distributed uniformly on a metal ring of radius R. a. What is the magnitude of the electric field in the center of the ring at point O (Fig. P24.61)? b. What is the magnitude of the electric field at the point A lying on the axis of the ring a distance R from the center O (same length as the radius of the ring)? FIGURE P24.61arrow_forwardA Ping-Pong paddle with surface area 3.80 102 m2 is placed in a uniform electric field of magnitude 1.10 106 N/C. a. What is the magnitude of the electric flux through the paddle when the electric field is parallel to the paddles surface? b. What is the magnitude of the electric flux through the paddle when the electric field is perpendicular to the paddles surface?arrow_forward
- stro Imssb1.mutah.edu.jo/ A charge of 24.4 pC is distributed uniformly on a spherical surface (r1 = 2.0 cm), and a second charge of -8.6 pC is distributed uniformly on a concentric thin spherical surface (r2 = 4.0 cm). Determine the magnitude of the electric field (in units of N/C) at a distance of 5.0 cm from the center of the two surfaces.arrow_forwardA cylindrical metal can has a height of 27 cm and a radius of 11 cm. The electric field is directed outward along the entire surface of the can (including the top and bottom), with a uniform magnitude 4.0 x 10^5 N/C. What is the surface area of the cylinder? What is the total flux on the sphere?arrow_forwardA conductive spherical shell has an outer radius of 20 cm and an inner radius of 15 cm. In the center of the spherical shell, there is an insulating sphere with a radius of 10 cm. The magnitude of the electric field at a distance of 12.5 cm from the center of this system is 1785 N /C and its direction is radially outward. At a point 35 cm from the center, the magnitude of the electric field is 132 N /C and the direction appears to be inward in the radius direction. (ke=9,00x109 Nm2/C2) a) Find the charge of the insulating sphere? b) Find the net charge of the conductive spherical shellI? c) Find the charges on the inner and outer surfaces of the spherical shell? d) Find the outer surface charge density of the shell?arrow_forward
- The volume charge density ρ for a spherical charge distribution of radius R= 6.00 mm is not uniform. (Figure 1) shows ρ as a function of the distance r from the center of the distribution. a)Calculate the electric field at r = 1.00 mm. b)Calculate the electric field at r = 1.00 mm.arrow_forwardPositive charge is distributed in a sphere of radius R that is centered at the origin. Inside the sphere, the electric field is Ē(r) = kr-1/4 f, where k is a positive constant. There is no charge outside the sphere. a) How is the charge distributed inside the sphere? In particular, find an equation for the charge density, p. b) Determine the electric field, E(r), for r > R (outside the sphere). c) What is the potential difference between the center of the sphere (r = 0) and the surface of the sphere (r = R)? d) What is the energy stored in this electric charge configuration?arrow_forwardThe volume charge density ρ for a spherical charge distribution of radius R = 6.00 mm is not uniform. The figure shows ρ as a function of the distance r from the center of the distribution. a) Calculate the electric field at r = 5.00 mm .Express your answer with the appropriate units. b) Calculate the electric field at r = 7.00 mm .Express your answer with the appropriate unitsarrow_forward
- A long, thin straight wire with a linear charge density λwire = +3.17 nC/m runs down the center of a thin, hollow metal cylindrical shell of radius 1.55 cm. The cylindrical shell has a linear charge density λshell = +6.97 nC/m.(Figure 1) What is the electric field strength at a radius of 1.04 cmcm inside the cylindrical shell? What is the electric field strength at a radius of 2.29 cmcm outside the cylindrical shell?arrow_forwardA long, nonconducting, solid cylinder of radius 4.0 cm has a nonuniform volume charge density p that is a function of radial distance r from the cylinder axis: p = Ar². For A = 2.5 µC/m³, what is the magnitude of the electric field at r = 3.0 cm. Give your answer in N/C. Yanıtınızarrow_forwardA solid plastic sphere of radius R1 = 1.00 cm is concentric with an aluminum spherical shell with inner radius R2 = 14.0 cm and outer radius R3 = 17.0 cm. Electricfield measurements are made at two points: At a radial distance of 34.0 cmfrom the center, the electric field has magnitude 1.70 x 103 N/C and is directedradially outward, and at a radial distance of 12.0 cm from the center, the electric field has magnitude 9.10 x 104 N/C and is directed radially inward. What are the net charges on a. the plastic sphere and b. the aluminum spherical shell? c. What are the charges on the inner and outer surfaces of the aluminum spherical shell?arrow_forward
 Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
Physics for Scientists and Engineers: Foundations...PhysicsISBN:9781133939146Author:Katz, Debora M.Publisher:Cengage Learning
