2nd Edition

ISBN: 9780134601823

Author: ETKINA, Eugenia, Planinšič, G. (gorazd), Van Heuvelen, Alan

Publisher: Pearson,

Concept explainers

One of the fundamental properties is the electromagnetic property. Charge cannot be destroyed by any process and this contributes formally to the law of charge conservation.

Videos

Textbook Question

Chapter 18, Problem 33P

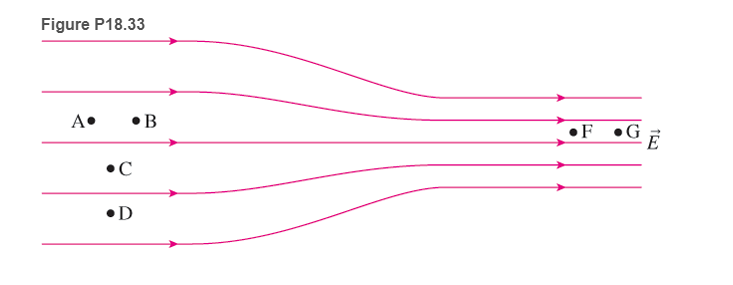
* Figure P18.33 shows E → field lines in a region of space Note that the distances between A and B, between C and D, and between F and G are all equal. (a) Rank the magnitudes of the E → field at points A to G from largest to smallest. (b) Rank the electric potentials at points A to G from largest to smallest. (c) A small negatively charged object is moved (I) from A to B, (II) from C to D, (III) from A to D, and (IV) from F to G Rank the work done on the object in these four cases from largest to smallest.

Chapter 18, Problem 33P, 33. * Figure P18.33 shows field lines in a region of space Note that the distances between A and B,

Expert Solution & Answer

Want to see the full answer?

Check out a sample textbook solution

See solution

Chapter 18, Problem 32P

Chapter 18, Problem 34P

Students have asked these similar questions

suggest a reason ultrasound cleaning is better than cleaning by hand?

Checkpoint 4 The figure shows four orientations of an electric di- pole in an external electric field. Rank the orienta- tions according to (a) the magnitude of the torque on the dipole and (b) the potential energy of the di- pole, greatest first. (1) (2) E (4)

What is integrated science. What is fractional distillation What is simple distillation

Chapter 18 Solutions

College Physics

Ch. 18 - Review Question 18.1 How do you estimate the...Ch. 18 - Review Question 18.2 You have a point-like object...Ch. 18 - Review Question 18.3 Compare the work needed to...Ch. 18 - Review Question 18.4 Imagine that you have an...Ch. 18 - Review Question 18.5 In this section you read that...Ch. 18 - Review Question 18.6 What are the differences...Ch. 18 - Review Question 18.7 A parallel plate capacitor...Ch. 18 - Review Question 18.8 Why do heart contractions...Ch. 18 - 1 What does the field at point A, which is a...Ch. 18 - Why can you shield an object from an external...

Ch. 18 - If you place a block made of a conducting material...Ch. 18 - 4. If you place a block made of a dielectric...Ch. 18 - 5. Two identical positive charges are located at a...Ch. 18 - An electric dipole is placed between the...Ch. 18 - 7. A positive charge is fixed at some distance d...Ch. 18 - Figure Q18.8 shows E field lines in a region of...Ch. 18 - How do we use the model of the electric field to...Ch. 18 - Describe a procedure to determine the E field at...Ch. 18 - What does it mean if the E field at a certain...Ch. 18 - A very small positive charge is placed at one...Ch. 18 - 13. How do we create an E field with parallel...Ch. 18 - 14. Draw a sketch of the field lines caused by...Ch. 18 - 15. Draw a sketch of the field lines caused by...Ch. 18 - 16. Jim thinks that E field lines are the paths...Ch. 18 - Can E field lines cross? Explain why or why not.Ch. 18 - An electron moving horizontally from left to right...Ch. 18 - 19. (a) What does it mean if the electric...Ch. 18 - 20. Explain how grounding works. Ch. 18 - 21. Explain how shielding works. Ch. 18 - 22. Explain the difference between the microscopic...Ch. 18 - Explain why, for charged objects submerged in a...Ch. 18 - 24. What does it mean if the dielectric constant k...Ch. 18 - What is the dielectric constant of a metal?Ch. 18 - Describe the relation between the quantities E...Ch. 18 - If the V field in a region is constant, what is...Ch. 18 - 28. Why are uncharged pieces of a dielectric...Ch. 18 - 29. Draw equipotential surfaces and label them in...Ch. 18 - Show a charge arrangement and a point in space...Ch. 18 - 31. Explain what happens when you place a...Ch. 18 - (a) Explain what happens when you place a...Ch. 18 - 33. Explain why the excess charge on an electrical...Ch. 18 - Draw a microscopic representation of the charge...Ch. 18 - 1. * (a) Construct a graph of the magnitude of the...Ch. 18 - * A uranium nucleus has 92 protons. (a) Determine...Ch. 18 - 3. The electron and the proton in a hydrogen atom...Ch. 18 - * Use the superposition principle to draw E field...Ch. 18 - 5. * Use the superposition principle to draw ...Ch. 18 - * E field lines for a field created by an...Ch. 18 - 7. * Two objects with charges C are 50 cm from...Ch. 18 - 8. * charged object is 6.0 cm along a horizontal...Ch. 18 - 9. ** charged object is 4.0 cm along a horizontal...Ch. 18 - 10. **A distance d separates two objects, each...Ch. 18 - 11. * A point-like charged object with a charge +...Ch. 18 - 12. * A 3.0-g aluminum foil ball with a charge of ...Ch. 18 - 13. ** (a) If the string in the previous problem...Ch. 18 - * EST Using Earths E field for flight Earth has an...Ch. 18 - * An electron moving with a speed v0 enters a...Ch. 18 - 10-9 C hangs freely from a 1.0-m-long thread. What...Ch. 18 - 17. A 0.50-g oil droplet with charge is in a...Ch. 18 - 19. * Equation Jeopardy 1 The equations below...Ch. 18 - * Equation Jeopardy 2 The equations below describe...Ch. 18 - 21. During a lightning flash. of charge moves...Ch. 18 - 22. * (a) Construct a graph of the V field created...Ch. 18 - * A horizontal distance d separates two objects...Ch. 18 - * Two objects with charges qand+q are separated by...Ch. 18 - * Four objects with the same charge q are placed...Ch. 18 - 26. Spark jumps to nose An electric spark jumps...Ch. 18 - 27. * Two charged point-like objects are...Ch. 18 - BIO Electric field in body cell The electric...Ch. 18 - * Equation Jeopardy 3 The equation below describes...Ch. 18 - 31. * Equation Jeopardy 4 The equation below...Ch. 18 - 32. * While a sphere with positive charge remains...Ch. 18 - 33. * Figure P18.33 shows field lines in a region...Ch. 18 - 34. * A metal sphere has no charge on it. A...Ch. 18 - 35. ** EST A Van de Graaff generator of radius...Ch. 18 - ** A metal ball of radius R1 has a charge Q. Later...Ch. 18 - 37. * Positively charged metal sphere A is placed...Ch. 18 - *Two small metal spheres A and B have different...Ch. 18 - 39. * An electric dipole such as a water molecule...Ch. 18 - 10-7C at its head and an equal magnitude negative...Ch. 18 - 41. BIO Body cell membrane electric field (a)...Ch. 18 - 42. ** Earth's electric field Earth has an...Ch. 18 - 43. You have a parallel plate capacitor. (a)...Ch. 18 - 44. * A capacitor of capacitance C with a vacuum...Ch. 18 - 45. * A capacitor of capacitance C with a vacuum...Ch. 18 - How does the capacitance of a parallel plate...Ch. 18 - BIO EST Axon capacitance The long thin cylindrical...Ch. 18 - 48. ** Sphere capacitance A metal sphere of radius...Ch. 18 - * BIO EST Capacitance of red blood cell Assume...Ch. 18 - BIO Defibrillator During ventricular fibrillation...Ch. 18 - * EST The dielectric strength of air is 3106V/m....Ch. 18 - * Charged cloud causes electric field on Earth The...Ch. 18 - *BIO Hearts dipole charge The heart has a dipole...Ch. 18 - 55. * In a hot water heater, water warms when...Ch. 18 - 56. ** EST Lightning warms water A lightning flash...Ch. 18 - 57 * Four charged particles A, B, C, and D are...Ch. 18 - 59. ** A small object of unknown mass and charge...Ch. 18 - 61. * BIO Electrophoresis Electrophoresis is used...Ch. 18 - 62. * BIO Energy stored in axon electric field An...Ch. 18 - BIO Electric discharge by eels In several aquatic...Ch. 18 - BIO Electric discharge by eels In several aquatic...Ch. 18 - BIO Electric discharge by eels In several aquatic...Ch. 18 - BIO Electric discharge by eels In several aquatic...Ch. 18 - BIO Electric discharge by eels In several aquatic...Ch. 18 - BIO Electric discharge by eels In several aquatic...Ch. 18 - Electrostatic precipitator (esp) Electrostatic...Ch. 18 - Electrostatic precipitator (esp) Electrostatic...Ch. 18 - Electrostatic precipitator (esp) Electrostatic...Ch. 18 - Electrostatic precipitator (esp) Electrostatic...Ch. 18 - Electrostatic precipitator (esp) Electrostatic...

Additional Science Textbook Solutions

Find more solutions based on key concepts

1. What are the main organs of the skeletal system?

Human Anatomy & Physiology (2nd Edition)

Plants use the process of photosynthesis to convert the energy in sunlight to chemical energy in the form of su...

Campbell Essential Biology with Physiology (5th Edition)

The following data were obtained from a disk-diffusion test. Antibiotic Zone of Inhibition A 15 mm B 0 mm c 7 m...

Microbiology: An Introduction

Two culture media were inoculated with four different bacteria. After incubation, the following results were ob...

Microbiology: An Introduction

Predict the type of reaction (if any) that occurs between each pair of substances. Write balanced molecular equ...

Introductory Chemistry (6th Edition)

5. When the phenotype of heterozygotes is intermediate between the phenotypes of the two homozygotes, this patt...

Biology: Life on Earth (11th Edition)

Knowledge Booster

Learn more about

Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.