
The structure stiffness matrix for the frame.
Answer to Problem 16.1P
The structure stiffness matrix for the frame is shown below.
Explanation of Solution
Concept Used:
Write the expression for direction cosine in x-direction.
Here, coordinate of x at near end of the member is
Write the expression for direction cosine in x-direction.
Here, coordinate of y at near end of the member is
Write the global stiffness matrix for the member.
Here, cross-sectional area of the member is
Calculation:
The free body diagram of the frame is shown below.
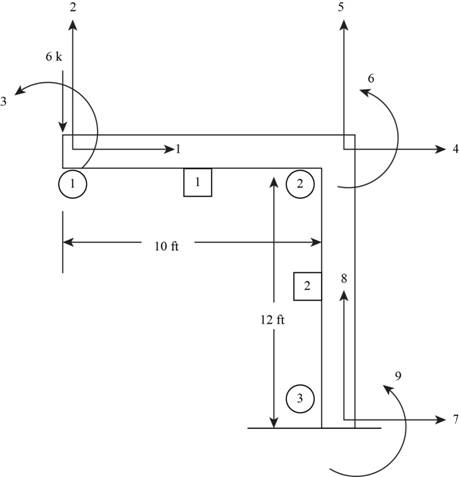
Figure (1)
Consider the member-1.
Calculate direction cosine in x-direction.
Substitute
Calculate direction cosine in y-direction.
Substitute
The stiffness matrix for member-1 is shown below.
Substitute
Calculate the value of
Substitute
Calculate the value of
Substitute
Calculate the value of
Substitute
Calculate the value of
Substitute
Calculate the value of
Substitute
Substitute
Consider the member-2.
Calculate direction cosine in x-direction.
Substitute
Calculate direction cosine in y-direction.
Substitute
The stiffness matrix for member-1 is shown below.
Substitute
Calculate the value of
Substitute
Calculate the value of
Substitute
Calculate the value of
Substitute
Calculate the value of
Substitute
Calculate the value of
Substitute
Substitute
The final matrix of the frame by assembling matrices (III) and (IV) is shown below.
Conclusion:
The structure stiffness matrix for the frame is shown below.
Want to see more full solutions like this?
Chapter 16 Solutions
Structural Analysis (10th Edition)
- I need a real solution, not artificial intelligencearrow_forwardI need detailed help solving this exercise from homework of Applied Mechanics. I do not really understand how to do, please do it step by step, not that long but clear. Thank you!arrow_forwardI need detailed help solving this exercise from homework of Applied Mechanics. I do not really understand how to do, please do it step by step, not that long but clear. Thank you!arrow_forward
- I need detailed help solving this exercise from homework of Applied Mechanics. I do not really understand how to do, please do it step by step, not that long but clear. Thank you!arrow_forwardI need detailed help solving this exercise from homework of Applied Mechanics. I do not really understand how to do, please do it step by step, not that long but clear. Thank you!arrow_forwardI need detailed help solving this exercise from homework of Applied Mechanics. I do not really understand how to do, please do it step by step, not that long but clear. Thank you!arrow_forward
- I need detailed help solving this exercise from homework of Applied Mechanics. I do not really understand how to do, please do it step by step, not that long but clear. Thank you!arrow_forwardI need detailed help solving this exercise from homework of Applied Mechanics. I do not really understand how to do, please do it step by step, not that long but clear. Thank you!arrow_forwardI need detailed help solving this exercise from homework of Applied Mechanics. I do not really understand how to do, please do it step by step, not that long but clear. Thank you!arrow_forward
- I need detailed help solving this exercise from homework of Applied Mechanics. I do not really understand how to do, please do it step by step, not that long but clear. Thank you!arrow_forwardI need detailed help solving this exercise from homework of Applied Mechanics. I do not really understand how to do, please do it step by step, not that long but clear. Thank you!arrow_forwardI need detailed help solving this exercise from homework of Applied Mechanics. I do not really understand how to do, please do it step by step, not that long but clear. Thank you!arrow_forward

 Structural Analysis (10th Edition)Civil EngineeringISBN:9780134610672Author:Russell C. HibbelerPublisher:PEARSON
Structural Analysis (10th Edition)Civil EngineeringISBN:9780134610672Author:Russell C. HibbelerPublisher:PEARSON Principles of Foundation Engineering (MindTap Cou...Civil EngineeringISBN:9781337705028Author:Braja M. Das, Nagaratnam SivakuganPublisher:Cengage Learning
Principles of Foundation Engineering (MindTap Cou...Civil EngineeringISBN:9781337705028Author:Braja M. Das, Nagaratnam SivakuganPublisher:Cengage Learning Fundamentals of Structural AnalysisCivil EngineeringISBN:9780073398006Author:Kenneth M. Leet Emeritus, Chia-Ming Uang, Joel LanningPublisher:McGraw-Hill Education
Fundamentals of Structural AnalysisCivil EngineeringISBN:9780073398006Author:Kenneth M. Leet Emeritus, Chia-Ming Uang, Joel LanningPublisher:McGraw-Hill Education
 Traffic and Highway EngineeringCivil EngineeringISBN:9781305156241Author:Garber, Nicholas J.Publisher:Cengage Learning
Traffic and Highway EngineeringCivil EngineeringISBN:9781305156241Author:Garber, Nicholas J.Publisher:Cengage Learning





