Calculus Volume 2
17th Edition
ISBN: 9781938168062
Author: Gilbert Strang, Edwin Jed Herman
Publisher: OpenStax
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 1.3, Problem 163E
The graph of
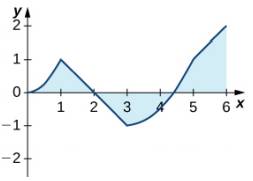
a. Over which intervals is
b. Over which intervals is
c. What is the average value of
Expert Solution & Answer
Trending nowThis is a popular solution!
Students have asked these similar questions
из
Review the deck below and determine its total square footage (add its deck and backsplash square footage
together to get the result). Type your answer in the entry box and click Submit.
126 1/2"
5" backsplash
A
158"
CL
79"
B
26"
Type your
answer here.
Refer to page 311 for a sequence of functions defined on a given interval.
Instructions:
•
Analyze whether the sequence converges pointwise and/or uniformly on the given interval.
• Discuss the implications of uniform convergence for integration and differentiation of the
sequence.
•
Provide counterexamples if any condition fails.
Link: [https://drive.google.com/file/d/1wKSrun-GlxirS3IZ9qo Hazb9tC440 AZF/view?usp=sharing]
Refer to page 310 for a matrix and its associated system of differential equations.
Instructions:
• Find the eigenvalues of the given matrix and classify the stability of the system (e.g., stable,
•
unstable, saddle point).
Discuss the geometric interpretation of eigenvalues in the context of system behavior.
•
Provide conditions under which the system exhibits periodic solutions.
Link: [https://drive.google.com/file/d/1wKSrun-GlxirS3IZ9qoHazb9tC440 AZF/view?usp=sharing]
Chapter 1 Solutions
Calculus Volume 2
Ch. 1.1 - State whether the given sums are equal or unequal....Ch. 1.1 - In the following exercises, use the rules for sums...Ch. 1.1 - In the following exercises, use the rules for sums...Ch. 1.1 - Suppose that i=1100ai=15 and i=1100bi=12 . In the...Ch. 1.1 - Suppose that i=1100ai=15 and i=1100bi=12 . In the...Ch. 1.1 - Suppose that i=1100ai=15 and i=1100bi=12 . In the...Ch. 1.1 - Suppose that i=1100ai=15 and i=1100bi=12 . In the...Ch. 1.1 - In the following exercises, use summation...Ch. 1.1 - In the following exercises, use summation...Ch. 1.1 - In the following exercises, use summation...
Ch. 1.1 - In the following exercises, use summation...Ch. 1.1 - Let Ln denote the left-endpoint sum using n...Ch. 1.1 - Let Ln denote the left-endpoint sum using n...Ch. 1.1 - Let Ln denote the left-endpoint sum using n...Ch. 1.1 - Let Ln denote the left-endpoint sum using n...Ch. 1.1 - Let Ln denote the left-endpoint sum using n...Ch. 1.1 - Let Ln denote the left-endpoint sum using n...Ch. 1.1 - Let Ln denote the left-endpoint sum using n...Ch. 1.1 - Let Ln denote the left-endpoint sum using n...Ch. 1.1 - Compute the left and right Riemann sums—L4 and R4,...Ch. 1.1 - Compute the left and right Riemann sums—L6 and R6,...Ch. 1.1 - Compute the left and right Riemann sums—L4 and R4,...Ch. 1.1 - Compute the left and right Riemann sums—L6 and R6,...Ch. 1.1 - Express the following endpoint sums in sigma...Ch. 1.1 - Express the following endpoint sums in sigma...Ch. 1.1 - Express the following endpoint sums in sigma...Ch. 1.1 - Express the following endpoint sums in sigma...Ch. 1.1 - In the following exercises, graph the function...Ch. 1.1 - In the following exercises, graph the function...Ch. 1.1 - In the following exercises, graph the function...Ch. 1.1 - In the following exercises, graph the function...Ch. 1.1 - In the following exercises, graph the function...Ch. 1.1 - In the following exercises, graph the function...Ch. 1.1 - Let tj denote the time that it took Tejay van...Ch. 1.1 - Let rj denote the total rainfall in Portland on...Ch. 1.1 - Let dj denote the hours of daylight and j denote...Ch. 1.1 - To help get in shape, Joe gets a new pair of...Ch. 1.1 - The following table gives approximate values of...Ch. 1.1 - The following table gives the approximate increase...Ch. 1.1 - The following table gives the approximate increase...Ch. 1.1 - The following {able gives the percent growth of...Ch. 1.1 - wIn the following exercises, estimate the areas...Ch. 1.1 - In the following exercises, estimate the areas...Ch. 1.1 - In the following exercises, estimate the areas...Ch. 1.1 - In the following exercises, estimate the areas...Ch. 1.1 - [T] Use a computer algebra system to compute the...Ch. 1.1 - [T] Use a computer algebra system to computer the...Ch. 1.1 - [T] Use a computer algebra system to compute the...Ch. 1.1 - In the following exercises, use a calculator or a...Ch. 1.1 - In the following exercises, use a calculator or a...Ch. 1.1 - In the following exercises, use a calculator at a...Ch. 1.1 - In the following exercises, use a calculator at a...Ch. 1.1 - In the following exercises, use a calculator at a...Ch. 1.1 - In the following exercises, use a calculator at a...Ch. 1.1 - In the following exercises, use a calculator at a...Ch. 1.1 - In the following exercises, use a calculator at a...Ch. 1.1 - For each Of the three graphs: a. Obtain a lower...Ch. 1.1 - In the previous exercise, explain why L(A) gets no...Ch. 1.1 - A unit circle is made up of n wedges equivalent to...Ch. 1.2 - In the following exercises, express the limits as...Ch. 1.2 - In the following exercises, express the limits as...Ch. 1.2 - In the following exercises, express the limits as...Ch. 1.2 - In the following exercises, express the limits as...Ch. 1.2 - In the following exercises, given Ln or Rn as...Ch. 1.2 - In the following exercises, given Ln or Rn as...Ch. 1.2 - In the following exercises, given Ln or Rn as...Ch. 1.2 - In the following exercises, given Ln or Rn as...Ch. 1.2 - In the following exercises, given Ln or Rn as...Ch. 1.2 - In the following exercises, given Ln or Rn as...Ch. 1.2 - In the following exercises, evaluate the integrals...Ch. 1.2 - In the following exercises, evaluate the integrals...Ch. 1.2 - In the following exercises, evaluate the integrals...Ch. 1.2 - In the following exercises, evaluate the integrals...Ch. 1.2 - In the following exercises, evaluate the integrals...Ch. 1.2 - In the following exercises, evaluate the integrals...Ch. 1.2 - In the following exercises, evaluate the integral...Ch. 1.2 - In the following exercises, evaluate the integral...Ch. 1.2 - In the following exercises, evaluate the integral...Ch. 1.2 - In the following exercises, evaluate the integral...Ch. 1.2 - In the following exercises, evaluate the integral...Ch. 1.2 - In the following exercises, evaluate the integral...Ch. 1.2 - In the following exercises, evaluate the integral...Ch. 1.2 - In the following exercises, evaluate the integral...Ch. 1.2 - In the following exercises, use averages of values...Ch. 1.2 - In the following exercises, use averages of values...Ch. 1.2 - In the following exercises, use averages of values...Ch. 1.2 - In the following exercises, use averages of values...Ch. 1.2 - Suppose that 04f(x)dx=5 and 02f(x)dx=3 , and...Ch. 1.2 - Suppose that 04f(x)dx=5 and 02f(x)dx=3 , and...Ch. 1.2 - Suppose that 04f(x)dx=5 and 02f(x)dx=3 , and...Ch. 1.2 - Suppose that 04f(x)dx=5 and 02f(x)dx=3 , and...Ch. 1.2 - Suppose that 04f(x)dx=5 and 02f(x)dx=3 , and...Ch. 1.2 - Suppose that 04f(x)dx=5 and 02f(x)dx=3 , and...Ch. 1.2 - In the following exercises, use the identity...Ch. 1.2 - In the following exercises, use the identity...Ch. 1.2 - In the following exercises, use the identity...Ch. 1.2 - In the following exercises, use the identity...Ch. 1.2 - In the following exercises, given that...Ch. 1.2 - In the following exercises, given that...Ch. 1.2 - In the following exercises, given that...Ch. 1.2 - In the following exercises, given that...Ch. 1.2 - In the following exercises, given that...Ch. 1.2 - In the following exercises, given that...Ch. 1.2 - In the following exercises, use the comparison...Ch. 1.2 - In the following exercises, use the comparison...Ch. 1.2 - In the following exercises, use the comparison...Ch. 1.2 - In the following exercises, use the comparison...Ch. 1.2 - In the following exercises, use the comparison...Ch. 1.2 - In the following exercises, use the comparison...Ch. 1.2 - In the following exercises, find 1112 average...Ch. 1.2 - In the following exercises, find 1112 average...Ch. 1.2 - In the following exercises, find 1112 average...Ch. 1.2 - In the following exercises, find 1112 average...Ch. 1.2 - In the following exercises, find 1112 average...Ch. 1.2 - In the following exercises, find 1112 average...Ch. 1.2 - In the following exercises, approximate the...Ch. 1.2 - In the following exercises, approximate the...Ch. 1.2 - In the following exercises, approximate the...Ch. 1.2 - In the following exercises, approximate the...Ch. 1.2 - In the following exercises, compute the average...Ch. 1.2 - In the following exercises, compute the average...Ch. 1.2 - In the following exercises, compute the average...Ch. 1.2 - In the following exercises, compute the average...Ch. 1.2 - In the following exercises, compute the average...Ch. 1.2 - In the following exercises, compute the average...Ch. 1.2 - Show that the average value of sin2t over [0, 2 ]...Ch. 1.2 - Show that the average value of cos2t over [0, 2 ]...Ch. 1.2 - Explain why the graphs of a quadratic function...Ch. 1.2 - Suppose that parabola p(x)=ax2+bx+c opens downward...Ch. 1.2 - Suppose [a, b} can be subdivided into subintervals...Ch. 1.2 - Suppose f and g are continuous functions such that...Ch. 1.2 - Suppose the average value of f over [a, b] is 1...Ch. 1.2 - Suppose that [11. b] can be partitioned, taking...Ch. 1.2 - Suppose that for each i such that 1iN one has...Ch. 1.2 - Suppose that for each i such that 1iN one has...Ch. 1.2 - [T] Compute the left and right Riemann sums L10...Ch. 1.2 - [T] Compute the left and right Riemann sums, L10...Ch. 1.2 - If 151+t4dt=41.7133... , what is 151+u4du ?Ch. 1.2 - Estimate 01tdt using the left and light endpoint...Ch. 1.2 - Estimate 01tdt by comparison with the area of a...Ch. 1.2 - From the graph of sin(2(x) shown: a. Explain why...Ch. 1.2 - If f is 1-periodic (f(t+1)=f(t)) , odd, and...Ch. 1.2 - If f is 1-periodic and 01f(t)dt=A , is it...Ch. 1.3 - A Parachutist in Free Fall Figure 1.30 Skydivers...Ch. 1.3 - A Parachutist in Free Fall Figure 1.30 Skydivers...Ch. 1.3 - A Parachutist in Free Fall Figure 1.30 Skydivers...Ch. 1.3 - A Parachutist in Free Fall Figure 1.30 Skydivers...Ch. 1.3 - A Parachutist in Free Fall Figure 1.30 Skydivers...Ch. 1.3 - A Parachutist in Free Fall Figure 1.30 Skydivers...Ch. 1.3 - A Parachutist in Free Fall Figure 1.30 Skydivers...Ch. 1.3 - Consider two athletes running at variable speeds...Ch. 1.3 - Two mountain climbers start their climb at base...Ch. 1.3 - To get on a certain toll road a driver has to take...Ch. 1.3 - Set 1x(1t)dt . Find F’(2) and the average value of...Ch. 1.3 - In the following exercises, use the Fundamental...Ch. 1.3 - In the following exercises, use the Fundamental...Ch. 1.3 - In the following exercises, use the Fundamental...Ch. 1.3 - In the following exercises, use the Fundamental...Ch. 1.3 - In the following exercises, use the Fundamental...Ch. 1.3 - In the following exercises, use the Fundamental...Ch. 1.3 - In the following exercises, use the Fundamental...Ch. 1.3 - In the following exercises, use the Fundamental...Ch. 1.3 - In the following exercises, use the Fundamental...Ch. 1.3 - In the following exercises, use the Fundamental...Ch. 1.3 - In the following exercises, use the Fundamental...Ch. 1.3 - In the following exercises, use the Fundamental...Ch. 1.3 - The graph of y=0xf(t)dt , where f is a piecewise...Ch. 1.3 - The graph of y=0xf(t)dt , where {is a piecewise...Ch. 1.3 - The graph of y=0xl(t)dt , where l is a piecewise...Ch. 1.3 - The graph of y=0xl(t)dt , where l is a piecewise...Ch. 1.3 - In the following exercises, use a calculator to...Ch. 1.3 - In the following exercises, use a calculator to...Ch. 1.3 - In the following exercises, use a calculator to...Ch. 1.3 - In the following exercises, use a calculator to...Ch. 1.3 - In the following exercises, use a calculator to...Ch. 1.3 - In the following exercises, use a calculator to...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, evaluate each definite...Ch. 1.3 - In the following exercises, use the evaluation...Ch. 1.3 - In the following exercises, use the evaluation...Ch. 1.3 - In the following exercises, use the evaluation...Ch. 1.3 - In the following exercises, use the evaluation...Ch. 1.3 - In the following exercises, identify the mats 0f...Ch. 1.3 - In the following exercises, identify the mats 0f...Ch. 1.3 - In the following exercises, identify the mats 0f...Ch. 1.3 - In the following exercises, identify the mats 0f...Ch. 1.3 - Suppose that the number of hours of daylight en a...Ch. 1.3 - Suppose the rate of gasoline consumption in the...Ch. 1.3 - Explain why, if f is continuous aver [a, b], there...Ch. 1.3 - Explain why, if fits continuous over [a, b] and is...Ch. 1.3 - Kepler's first law states that the planets move in...Ch. 1.3 - A point on an ellipse with major axis length 2a...Ch. 1.3 - As implied earlier, according to Kepler's laws,...Ch. 1.3 - The force of gravitational attraction between the...Ch. 1.3 - The displacement from rest of a mass attached to a...Ch. 1.4 - Use basic integration formulas to compute the...Ch. 1.4 - Use basic integration formulas to compute the...Ch. 1.4 - Use basic integration formulas to compute the...Ch. 1.4 - Use basic integration formulas to compute the...Ch. 1.4 - Use basic integration formulas to compute the...Ch. 1.4 - Use basic integration formulas to compute the...Ch. 1.4 - Write an integral that expresses the increase in...Ch. 1.4 - Write an integral that quantifies the change in...Ch. 1.4 - A regular N—gon (an N—sided polygon with sides...Ch. 1.4 - The area of a regular pentagon with Side length a...Ch. 1.4 - A dodecahedron is a Platonic solid with a surface...Ch. 1.4 - An icosahedron is a Platonic solid with a surface...Ch. 1.4 - Write an integral that quantifies the change in...Ch. 1.4 - Write an integral that quantifies the increase in...Ch. 1.4 - Write an integral that quantifies the increase in...Ch. 1.4 - Write an integral that quantifies the increase in...Ch. 1.4 - Suppose that a particle moves along a straight...Ch. 1.4 - Suppose that a particle moves along a straight...Ch. 1.4 - Suppose that a particle moves along a straight...Ch. 1.4 - Suppose that a particle moves along a straight...Ch. 1.4 - A ball is thrown upward from a height of 1.5 m at...Ch. 1.4 - A ball is thrown upward from a height of 3 m at an...Ch. 1.4 - The area A(t) DE a circular shape is growing at a...Ch. 1.4 - A spherical balloon is being in?ated at a constant...Ch. 1.4 - Water flows into a conical tank with...Ch. 1.4 - A horizontal cylindrical tank has cross-sectional...Ch. 1.4 - The following table lists the electrical power in...Ch. 1.4 - The average residential electrical power use (in...Ch. 1.4 - The data in the following table are used to...Ch. 1.4 - Minutes Watts Minutes Watts 15 200 165 170 30 180...Ch. 1.4 - The distribution of incomes as of 2012 in the...Ch. 1.4 - Newton’s law of gravity states that the...Ch. 1.4 - For a given motor vehicle, the maximum achievable...Ch. 1.4 - John is a 25—year 01d man who weighs 160 1b. He...Ch. 1.4 - Sandra is a 25—year old woman who weighs 120 lb....Ch. 1.4 - A motor vehicle has a maximum efficiency of 33 mpg...Ch. 1.4 - Although some engines are more efficient at given...Ch. 1.4 - [T] The following table lists the 2013 schedule of...Ch. 1.4 - [T] The following table provides hypothetical data...Ch. 1.4 - For the next two exercises use the data in the...Ch. 1.4 - For the next two exercises use the data in the...Ch. 1.4 - [T] Suppose you go on a road trip and record your...Ch. 1.4 - As a car accelerates, it does not accelerate at a...Ch. 1.4 - As a car accelerates, it does not accelerate at a...Ch. 1.4 - As a car accelerates, it does not accelerate at a...Ch. 1.4 - [T] The number 0f hamburgers 50111 at a restaurant...Ch. 1.4 - [T] An athlete runs by a motion detector, which...Ch. 1.5 - Why is u-substitution referred to as change of...Ch. 1.5 - . If f=gh , when reversing the chain rule,...Ch. 1.5 - In the following exercises, verify each identity...Ch. 1.5 - In the following exercises, verify each identity...Ch. 1.5 - In the following exercises, verify each identity...Ch. 1.5 - In the following exercises, verify each identity...Ch. 1.5 - In the following exercises, verify each identity...Ch. 1.5 - In the following exercises, find the...Ch. 1.5 - In the following exercises, find the...Ch. 1.5 - In the following exercises, find the...Ch. 1.5 - In the following exercises, find the...Ch. 1.5 - In the following exercises, find the...Ch. 1.5 - In the following exercises, find the...Ch. 1.5 - In the following exercises, find the...Ch. 1.5 - In the following exercises, find the...Ch. 1.5 - In the following exercises, find the...Ch. 1.5 - In the following exercises, find the...Ch. 1.5 - In the following Exercises, use a suitable change...Ch. 1.5 - In the following Exercises, use a suitable change...Ch. 1.5 - In the following Exercises, use a suitable change...Ch. 1.5 - In the following Exercises, use a suitable change...Ch. 1.5 - In the following Exercises, use a suitable change...Ch. 1.5 - In the following Exercises, use a suitable change...Ch. 1.5 - In the following Exercises, use a suitable change...Ch. 1.5 - In the following Exercises, use a suitable change...Ch. 1.5 - In the following Exercises, use a suitable change...Ch. 1.5 - In the following Exercises, use a suitable change...Ch. 1.5 - In the following Exercises, use a suitable change...Ch. 1.5 - In the following Exercises, use a suitable change...Ch. 1.5 - In the following Exercises, use a suitable change...Ch. 1.5 - In the following Exercises, use a suitable change...Ch. 1.5 - In the following Exercises, use a suitable change...Ch. 1.5 - In the following Exercises, use a suitable change...Ch. 1.5 - In the following Exercises, use a suitable change...Ch. 1.5 - In the following Exercises, use a calculator to...Ch. 1.5 - In the following Exercises, use a calculator to...Ch. 1.5 - In the following Exercises, use a calculator to...Ch. 1.5 - In the following Exercises, use a calculator to...Ch. 1.5 - In the following exercises, use a change of...Ch. 1.5 - In the following exercises, use a change of...Ch. 1.5 - In the following exercises, use a change of...Ch. 1.5 - In the following exercises, use a change of...Ch. 1.5 - In the following exercises, use a change of...Ch. 1.5 - In the following exercises, use a change of...Ch. 1.5 - In the following exercises, evaluate the...Ch. 1.5 - In the following exercises, evaluate the...Ch. 1.5 - In the following exercises, evaluate the...Ch. 1.5 - In the following exercises, evaluate the...Ch. 1.5 - In the following exercises, evaluate the...Ch. 1.5 - In the following exercises, evaluate the...Ch. 1.5 - If h(a)=h(b) in abg(h(x))h(x)dx , what can you say...Ch. 1.5 - Is the substitution u=1x2 02x1x2dx okay? If not,...Ch. 1.5 - In the following exercises, use a change of...Ch. 1.5 - In the following exercises, use a change of...Ch. 1.5 - In the following exercises, use a change of...Ch. 1.5 - In the following exercises, use a change of...Ch. 1.5 - In the following exercises, use a change of...Ch. 1.5 - In the following exercises, use a change of...Ch. 1.5 - In the following exercises, use a change of...Ch. 1.5 - Show that the avenge value of f(x) over an...Ch. 1.5 - €314. Find the area under the graph of f(t)=t(1 t...Ch. 1.5 - Find the area under the graph of g(t)=t(1 t 2)a...Ch. 1.5 - The area of a semicircle of radius 1 can be...Ch. 1.5 - The area of the top half of an ellipse with a...Ch. 1.5 - [T] The following graph is of a function of the...Ch. 1.5 - The following graph is of a function of the form...Ch. 1.6 - In the following exercises, compute each...Ch. 1.6 - In the following exercises, compute each...Ch. 1.6 - In the following exercises, compute each...Ch. 1.6 - In the following exercises, compute each...Ch. 1.6 - In the following exercises, compute each...Ch. 1.6 - In the following exercises, compute each...Ch. 1.6 - In the following exercises, compute each...Ch. 1.6 - In the following exercises, compute each...Ch. 1.6 - In the following exercises, find each indefinite...Ch. 1.6 - In the following exercises, find each indefinite...Ch. 1.6 - In the following exercises, find each indefinite...Ch. 1.6 - In the following exercises, find each indefinite...Ch. 1.6 - In the following exercises, find each indefinite...Ch. 1.6 - In the following exercises, find each indefinite...Ch. 1.6 - In the following exercises, find each indefinite...Ch. 1.6 - In the following exercises, find each indefinite...Ch. 1.6 - In the following exercises, find each indefinite...Ch. 1.6 - In the following exercises, find each indefinite...Ch. 1.6 - In the following exercises, find each indefinite...Ch. 1.6 - In the following exercises, find each indefinite...Ch. 1.6 - In the following exercises, find each indefinite...Ch. 1.6 - In the following exercises, find each indefinite...Ch. 1.6 - In the following exercises, verify by...Ch. 1.6 - In the following exercises, verify by...Ch. 1.6 - In the following exercises, verify by...Ch. 1.6 - In the following exercises, verify by...Ch. 1.6 - Write an integral to express the area under the...Ch. 1.6 - Write an integral to express the area under the...Ch. 1.6 - In the following exercises, use appropriate...Ch. 1.6 - In the following exercises, use appropriate...Ch. 1.6 - In the following exercises, use appropriate...Ch. 1.6 - In the following exercises, use appropriate...Ch. 1.6 - In the following exercises, use appropriate...Ch. 1.6 - In the following exercises, use appropriate...Ch. 1.6 - In the following exercises, use appropriate...Ch. 1.6 - In the following exercises, evaluate the definite...Ch. 1.6 - In the following exercises, evaluate the definite...Ch. 1.6 - In the following exercises, evaluate the definite...Ch. 1.6 - In the following exercises, evaluate the definite...Ch. 1.6 - In the following exercises, evaluate the definite...Ch. 1.6 - In the following exercises, integrate using the...Ch. 1.6 - In the following exercises, integrate using the...Ch. 1.6 - In the following exercises, integrate using the...Ch. 1.6 - In the following exercises, integrate using the...Ch. 1.6 - In the following exercises, integrate using the...Ch. 1.6 - In the following exercises, integrate using the...Ch. 1.6 - In the following exercises, does the...Ch. 1.6 - In the following exercises, does the...Ch. 1.6 - In the following exercises, does the...Ch. 1.6 - In the following exercises, does the...Ch. 1.6 - In the following exercises, does the...Ch. 1.6 - In the following exercises, does the...Ch. 1.6 - In the following exercises, f(x)0 for axb . Find...Ch. 1.6 - In the following exercises, f(x)0 for axb . Find...Ch. 1.6 - In the following exercises, f(x)0 for axb . Find...Ch. 1.6 - In the following exercises, f(x)0 for axb . Find...Ch. 1.6 - Find the area under the graph of the function...Ch. 1.6 - Compute the integral of f(x)=xex2 and find the...Ch. 1.6 - Find the limit, as N tends to in?nity, of the area...Ch. 1.6 - Show that abdtt=1/b1/adtt when 0ab .Ch. 1.6 - Suppose that f(x) > 0 for all x and that f and g...Ch. 1.6 - Use the previous exercise to find the...Ch. 1.6 - Show that if c > 0, then the integral of l/x from...Ch. 1.6 - The following exercises are intended to derive the...Ch. 1.6 - The following exercises are intended to derive the...Ch. 1.6 - The following exercises are intended to derive the...Ch. 1.6 - Pretend, fat the moment, that we do not know that...Ch. 1.6 - Pretend, fur the moment, that we do not know that...Ch. 1.6 - The sine integral, defined as S(x)=0xsinttdt is an...Ch. 1.6 - [T] The normal distribution in probability is...Ch. 1.6 - [T] Compute the right endpoint estimates R50 and...Ch. 1.7 - In the following exercises, evaluate each integral...Ch. 1.7 - In the following exercises, evaluate each integral...Ch. 1.7 - In the following exercises, evaluate each integral...Ch. 1.7 - In the following exercises, evaluate each integral...Ch. 1.7 - In the following exercises, evaluate each integral...Ch. 1.7 - In the following exercises, evaluate each integral...Ch. 1.7 - In the following Exercises, find each indefinite...Ch. 1.7 - In the following Exercises, find each indefinite...Ch. 1.7 - In the following Exercises, find each indefinite...Ch. 1.7 - In the following Exercises, find each indefinite...Ch. 1.7 - In the following Exercises, find each indefinite...Ch. 1.7 - In the following Exercises, find each indefinite...Ch. 1.7 - Explain the relationship cos1t+C=dt 1 t 2 =sin1t+C...Ch. 1.7 - Explain the relationship sec1+C=dt|t| t 2...Ch. 1.7 - Explain what is wrong with the following integral:...Ch. 1.7 - Explain what is wrong with the following integral:...Ch. 1.7 - In the following exercises, solve for the...Ch. 1.7 - In the following exercises, solve for the...Ch. 1.7 - In the following exercises, solve for the...Ch. 1.7 - In the following exercises, solve for the...Ch. 1.7 - In the following Exercises, compute the...Ch. 1.7 - In the following Exercises, compute the...Ch. 1.7 - In the following Exercises, compute the...Ch. 1.7 - In the following Exercises, compute the...Ch. 1.7 - In the following Exercises, compute the...Ch. 1.7 - In the following Exercises, compute the...Ch. 1.7 - In the following exercises, solve for the...Ch. 1.7 - In the following exercises, solve for the...Ch. 1.7 - In the following exercises, solve for the...Ch. 1.7 - In the following exercises, solve for the...Ch. 1.7 - In the following exercises, solve for the...Ch. 1.7 - In the following exercises, solve for the...Ch. 1.7 - In the following exercises, compute each integral...Ch. 1.7 - In the following exercises, compute each integral...Ch. 1.7 - In the following exercises, compute each integral...Ch. 1.7 - In the following exercises, compute each integral...Ch. 1.7 - In the following exercises, compute each integral...Ch. 1.7 - In the following exercises, compute each integral...Ch. 1.7 - In the following Exercises, compute each definite...Ch. 1.7 - In the following Exercises, compute each definite...Ch. 1.7 - In the following Exercises, compute each definite...Ch. 1.7 - In the following Exercises, compute each definite...Ch. 1.7 - For A > 0, compute I(A)=AAdt1+t2 and evaluate...Ch. 1.7 - For 1B , compute I(B)=1Bdtt t 2 1 and evaluate...Ch. 1.7 - Use the substitution u=2cotx and the identity...Ch. 1.7 - Approximate the points at which the graphs of...Ch. 1.7 - . [T] Approximate the points at which the graphs...Ch. 1.7 - Use the following graph to prove that...Ch. 1 - True or False. Justify your answer with a proof or...Ch. 1 - True or False. Justify your answer with a proof or...Ch. 1 - True or False. Justify your answer with a proof or...Ch. 1 - True or False. Justify your answer with a proof or...Ch. 1 - Evaluate the Riemann sums L4 and R4 for the...Ch. 1 - Evaluate the Riemann sums L4 and R4 for the...Ch. 1 - Evaluate the Riemann sums L4 and R4 for the...Ch. 1 - Evaluate the Riemann sums L4 and R4 for the...Ch. 1 - Evaluate the following integrals. 447....Ch. 1 - Evaluate the following integrals. 448. 043t 1+6 t...Ch. 1 - Evaluate the following integrals. 449....Ch. 1 - Evaluate the following integrals. 450. 0/4e...Ch. 1 - Find the antiderivative. 451. dx ( x+4 )3Ch. 1 - Find the antiderivative. 452. xIn(x2)dxCh. 1 - Find the antiderivative. 453. 4x2 1 x 6 dxCh. 1 - Find the antiderivative. 454. e 2x1+e 4xdxCh. 1 - Find the derivative. 455. ddt0tsinx 1+ x 2 dxCh. 1 - Find the derivative. 456. ddx1x34t2dtCh. 1 - Find the derivative. 457. ddx1In(x)(4t+et)dtCh. 1 - Find the derivative. 458. ddx0cosxet2dtCh. 1 - The following problems consider the historic...Ch. 1 - The following problems consider the historic...Ch. 1 - The following problems consider the historic...Ch. 1 - The following problems consider the historic...Ch. 1 - The following problems consider the historic...
Additional Math Textbook Solutions
Find more solutions based on key concepts
TRY IT YOURSELF 1
Find the mean of the points scored by the 51 winning teams listed on page 39.
Elementary Statistics: Picturing the World (7th Edition)
Solve the inequality 3x27 .
Precalculus
In Exercises 1-14, evaluate the iterated integral.
7.
University Calculus: Early Transcendentals (4th Edition)
Disk method Let R be the region bounded by the following curves. Use the disk method to find the volume of the ...
Calculus: Early Transcendentals (2nd Edition)
Identifying Probability Distributions. In Exercises 7–14, determine whether a probability distribution is given...
Elementary Statistics (13th Edition)
Mathematical Connections Explain why 25 cents is one-fourth of a dollar, yet 15 minutes is one-fourth of an hou...
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, subject and related others by exploring similar questions and additional content below.Similar questions
- Refer to page 313 for a nonlinear differential equation and its linear approximation. Instructions: • Linearize the given nonlinear system around the equilibrium points. • Analyze the stability of each equilibrium using the Jacobian matrix and its eigenvalues. • Discuss the limitations of linearization for determining global behavior. Link: [https://drive.google.com/file/d/1wKSrun-GlxirS3IZ9qoHazb9tC440 AZF/view?usp=sharing]arrow_forwardRefer to page 314 for a matrix and its decomposed form. Instructions: • Verify the given singular value decomposition of the matrix. • • Discuss the geometric interpretation of the left and right singular vectors. Use the SVD to analyze the matrix's rank and nullity. Link: [https://drive.google.com/file/d/1wKSrun-GlxirS3IZ9qoHazb9tC440 AZ F/view?usp=sharing]arrow_forwardRefer to page 312 for a set of mappings between two groups G and H. Instructions: • • Verify which of the provided mappings are homomorphisms. Determine the kernel and image of valid homomorphisms and discuss their properties. • State whether the groups are isomorphic, justifying your conclusion. Link: [https://drive.google.com/file/d/1wKSrun-GlxirS3IZ9qo Hazb9tC440 AZF/view?usp=sharing]arrow_forward
- 12:25 AM Sun Dec 22 uestion 6- Week 8: QuX Assume that a company X + → C ezto.mheducation.com Week 8: Quiz i Saved 6 4 points Help Save & Exit Submit Assume that a company is considering purchasing a machine for $50,000 that will have a five-year useful life and a $5,000 salvage value. The machine will lower operating costs by $17,000 per year. The company's required rate of return is 15%. The net present value of this investment is closest to: Click here to view Exhibit 12B-1 and Exhibit 12B-2, to determine the appropriate discount factor(s) using the tables provided. 00:33:45 Multiple Choice О $6,984. $11,859. $22,919. ○ $9,469, Mc Graw Hill 2 100-arrow_forwardNo chatgpt pls will upvotearrow_forward7. [10 marks] Let G = (V,E) be a 3-connected graph. We prove that for every x, y, z Є V, there is a cycle in G on which x, y, and z all lie. (a) First prove that there are two internally disjoint xy-paths Po and P₁. (b) If z is on either Po or P₁, then combining Po and P₁ produces a cycle on which x, y, and z all lie. So assume that z is not on Po and not on P₁. Now prove that there are three paths Qo, Q1, and Q2 such that: ⚫each Qi starts at z; • each Qi ends at a vertex w; that is on Po or on P₁, where wo, w₁, and w₂ are distinct; the paths Qo, Q1, Q2 are disjoint from each other (except at the start vertex 2) and are disjoint from the paths Po and P₁ (except at the end vertices wo, W1, and w₂). (c) Use paths Po, P₁, Qo, Q1, and Q2 to prove that there is a cycle on which x, y, and z all lie. (To do this, notice that two of the w; must be on the same Pj.)arrow_forward
- 6. [10 marks] Let T be a tree with n ≥ 2 vertices and leaves. Let BL(T) denote the block graph of T. (a) How many vertices does BL(T) have? (b) How many edges does BL(T) have? Prove that your answers are correct.arrow_forward4. [10 marks] Find both a matching of maximum size and a vertex cover of minimum size in the following bipartite graph. Prove that your answer is correct. ย ພarrow_forward5. [10 marks] Let G = (V,E) be a graph, and let X C V be a set of vertices. Prove that if |S||N(S)\X for every SCX, then G contains a matching M that matches every vertex of X (i.e., such that every x X is an end of an edge in M).arrow_forward
- Q/show that 2" +4 has a removable discontinuity at Z=2i Z(≥2-21)arrow_forwardRefer to page 100 for problems on graph theory and linear algebra. Instructions: • Analyze the adjacency matrix of a given graph to find its eigenvalues and eigenvectors. • Interpret the eigenvalues in the context of graph properties like connectivity or clustering. Discuss applications of spectral graph theory in network analysis. Link: [https://drive.google.com/file/d/1wKSrun-GlxirS3IZ9qoHazb9tC440 AZF/view?usp=sharing]arrow_forwardRefer to page 110 for problems on optimization. Instructions: Given a loss function, analyze its critical points to identify minima and maxima. • Discuss the role of gradient descent in finding the optimal solution. . Compare convex and non-convex functions and their implications for optimization. Link: [https://drive.google.com/file/d/1wKSrun-GlxirS31Z9qo Hazb9tC440 AZF/view?usp=sharing]arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra and Trigonometry (MindTap Course List)AlgebraISBN:9781305071742Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
College AlgebraAlgebraISBN:9781305115545Author:James Stewart, Lothar Redlin, Saleem WatsonPublisher:Cengage Learning
Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Big Ideas Math A Bridge To Success Algebra 1: Stu...AlgebraISBN:9781680331141Author:HOUGHTON MIFFLIN HARCOURTPublisher:Houghton Mifflin Harcourt
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Algebra and Trigonometry (MindTap Course List)
Algebra
ISBN:9781305071742
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
College Algebra
Algebra
ISBN:9781305115545
Author:James Stewart, Lothar Redlin, Saleem Watson
Publisher:Cengage Learning
Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage
Big Ideas Math A Bridge To Success Algebra 1: Stu...
Algebra
ISBN:9781680331141
Author:HOUGHTON MIFFLIN HARCOURT
Publisher:Houghton Mifflin Harcourt
Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill
Fundamental Theorem of Calculus 1 | Geometric Idea + Chain Rule Example; Author: Dr. Trefor Bazett;https://www.youtube.com/watch?v=hAfpl8jLFOs;License: Standard YouTube License, CC-BY