Chapter 11.4, Problem 10E

a.

To determine

Find $b_0\text{ and }{b}_1$.

Answer to Problem 10E

The intercept $b_0$ is 31.53.

The slope $b_1$ is 0.691.

Explanation of Solution

Calculation:

The given information is that the sample data consists of 8 values for x and y.

Slope or $b_1$:

$b_1=r\frac{s_y}{s_x}$

where,

r represents the correlation coefficient between x and y.

$s_y$ represents the standard deviation of y.

$s_x$ represents the standard deviation of x.

Software procedure:

Step-by-step procedure to find the mean, standard deviation for x and y values using MINITAB is given below:

• Choose Stat > Basic Statistics > Display Descriptive Statistics.
• In Variables enter the columns of y and x.
• Choose Options Statistics, select Mean and Standard deviation.
• Click OK.

Output obtained from MINITAB is given below:

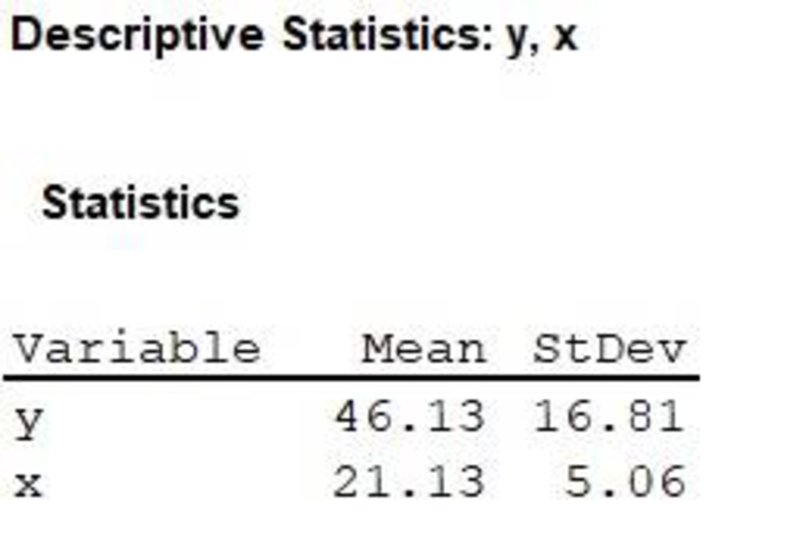
Correlation:

$r=\frac{1}{n-1}{\displaystyle \sum \left(\frac{x-\overline{x}}{s_x}\right)\left(\frac{y-\overline{y}}{s_y}\right)}$

Where,

$\overline{y}$ represents the mean of y values.

$\overline{x}$ represents the mean of x values.

$s_x$ represents the standard deviation of x.

$s_y$ represents the standard deviation of y.

n represents the sample size.

The table shows the calculation of correlation:

x	y	$x-\overline{x}$	$y-\overline{y}$	$\frac{x-\overline{x}}{s_x}$	$\frac{y-\overline{y}}{s_y}$	$\left(\frac{x-\overline{x}}{s_x}\right)\left(\frac{y-\overline{y}}{s_y}\right)$
23	51	1.87	4.87	0.369565	0.289709	0.107066
16	22	–5.13	–24.13	–1.01383	–1.43546	1.455313
17	56	–4.13	9.87	–0.81621	0.587151	–0.47924
19	34	–2.13	–12.13	–0.42095	–0.72159	0.303754
30	67	8.87	20.87	1.752964	1.241523	2.176345
19	59	–2.13	12.87	–0.42095	0.765616	–0.32228
18	55	–3.13	8.87	–0.61858	0.527662	–0.3264
27	25	5.87	–21.13	1.160079	–1.25699	–1.45821
Total						1.456

Thus, the correlation is

$\begin{array}{c}r=\frac{1.456}{8-1}\\ =\frac{1.456}{7}\\ =0.208\end{array}$

$b_1=r\frac{s_y}{s_x}$

Substitute r as 0.208, $s_y$ as 16.81 and $s_x$5.06

$\begin{array}{c}{b}_1=0.208\left(\frac{16.81}{5.06}\right)\\ =0.208\left(3.32\right)\\ =0.691\end{array}$

Thus, the slope $b_1$ is 0.691.

Intercept or $b_0$:

$b_0=\overline{y}-b_1\overline{x}$

$\overline{y}$ represents the mean of y values.

$\overline{x}$ represents the mean of x values.

$b_1$ represents the slope coefficient.

Substitute $\overline{y}$ as 46.13, $\overline{x}$ as 21.13 and $b_1$ as 0.691.

$\begin{array}{c}{b}_0=46.13-0.691\left(21.13\right)\\ =46.13-14.60\\ =31.53\end{array}$

Thus, the intercept $b_0$ is 31.53.

b.

To determine

Find the predicted value $\widehat{y}$ using the given value of x.

Answer to Problem 10E

The predicted value $\widehat{y}$ is 48.805.

Explanation of Solution

Calculation:

The given value of x is 25.

The estimated regression equation is $\widehat{y}=31.53+0.691x$

Substitute x as 25,

$\begin{array}{c}\widehat{y}=31.53+0.691\left(25\right)\\ =31.53+17.275\\ =48.805\end{array}$

Thus, the predicted value $\widehat{y}$ is 48.805.

c.

To determine

Find the residual standard deviation $s_e$.

Answer to Problem 10E

The residual standard deviation $s_e$ is 17.753.

Explanation of Solution

Calculation:

The residual standard deviation $s_e$ is calculated using the formula,

$s_e=\sqrt{\frac{{{\displaystyle \sum \left(y-\widehat{y}\right)}}^2}{n-2}}$

Where,

${{\displaystyle \sum \left(y-\widehat{y}\right)}}^2$ represents the sum of squares due to error

n represents the sample size.

Use the estimated regression equation to find the predicted value of y for each value of x.

y	$\widehat{y}$	$y-\widehat{y}$	${\left(y-\widehat{y}\right)}^2$
51	47.423	3.577	12.79493
22	42.586	–20.586	423.7834
56	43.277	12.723	161.8747
34	44.659	–10.659	113.6143
67	52.26	14.74	217.2676
59	44.659	14.341	205.6643
55	43.968	11.032	121.705
25	50.187	–25.187	634.385
Total			1,891.09

Substitute ${{\displaystyle \sum \left(y-\widehat{y}\right)}}^2$ as 1,891.09 and n as 8.

$\begin{array}{c}{s}_e=\sqrt{\frac{1,891.09}{8-2}}\\ =\sqrt{\frac{1,891.09}{6}}\\ =\sqrt{315.18}\\ =17.753\end{array}$

Thus, the residual standard deviation $s_e$ is 17.753.

d.

To determine

Find the sum of squares for x.

Answer to Problem 10E

The sum of squares for x is 178.8752.

Explanation of Solution

Calculation:

The table shows the calculation of sum of squares for x:

x	$x-\overline{x}$	${\left(x-\overline{x}\right)}^2$
23	1.87	3.4969
16	-5.13	26.3169
17	-4.13	17.0569
19	-2.13	4.5369
30	8.87	78.6769
19	-2.13	4.5369
18	-3.13	9.7969
27	5.87	34.4569
Total		178.8752

Thus, the sum of squares for x is 178.8752.

e.

To determine

Find the critical value for a 95% confidence or prediction interval.

Answer to Problem 10E

The critical value for a 95% confidence or prediction interval is 2.447.

Explanation of Solution

Calculation:

Critical value:

Software procedure:

Step-by-step procedure to find the critical value using MINITAB is given below:

• Choose Graph > Probability Distribution Plot choose View Probability > OK.
• From Distribution, choose ‘t’ distribution.
• In Degrees of freedom, enter 4.
• Click the Shaded Area tab.
• Choose Probability and Two tail for the region of the curve to shade.
• Enter the Probability value as 0.05.
• Click OK.

Output obtained from MINITAB is given below:

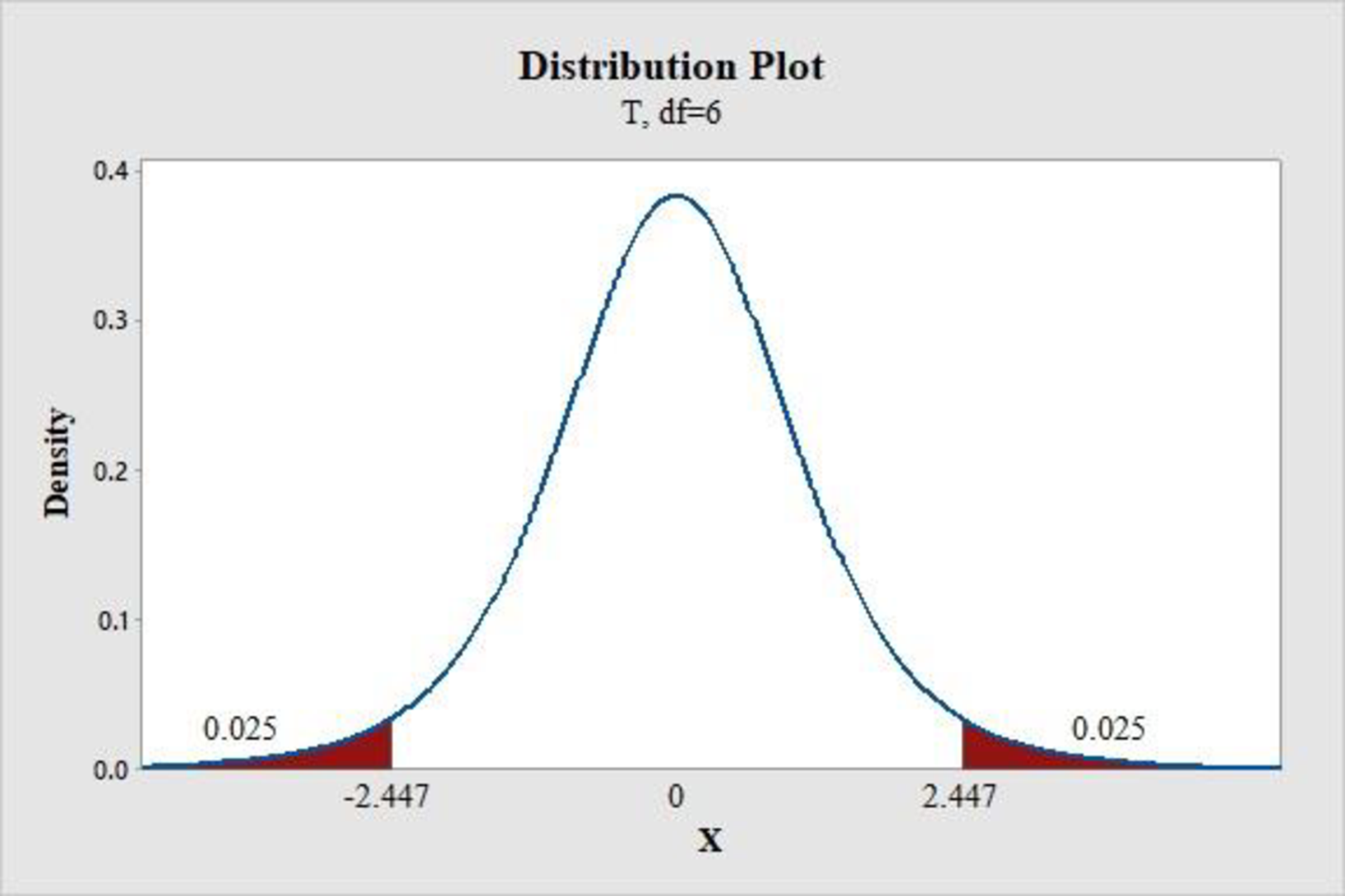
Thus, the critical value for a 95% confidence or prediction interval is 2.447.

f.

To determine

Construct the 95% confidence interval for the mean response for the given value of x.

Answer to Problem 10E

The 95% confidence interval for the mean response for the given value of x is $\left(28.9515,68.6656\right)$.

Explanation of Solution

Calculation:

The given value of x is 25.

Software procedure:

Step-by-step procedure to construct the 95% confidence interval for the mean response for the given value of x is given below:

• Choose Stat > Regression > Regression.
• In Response, enter the column containing the y.
• In Predictors, enter the columns containing the x.
• Click OK.
• Choose Stat > Regression > Regression>Predict.
• Choose Enter the individual values.
• Enter the x as 25.
• Click OK.

Output obtained from MINITAB is given below:

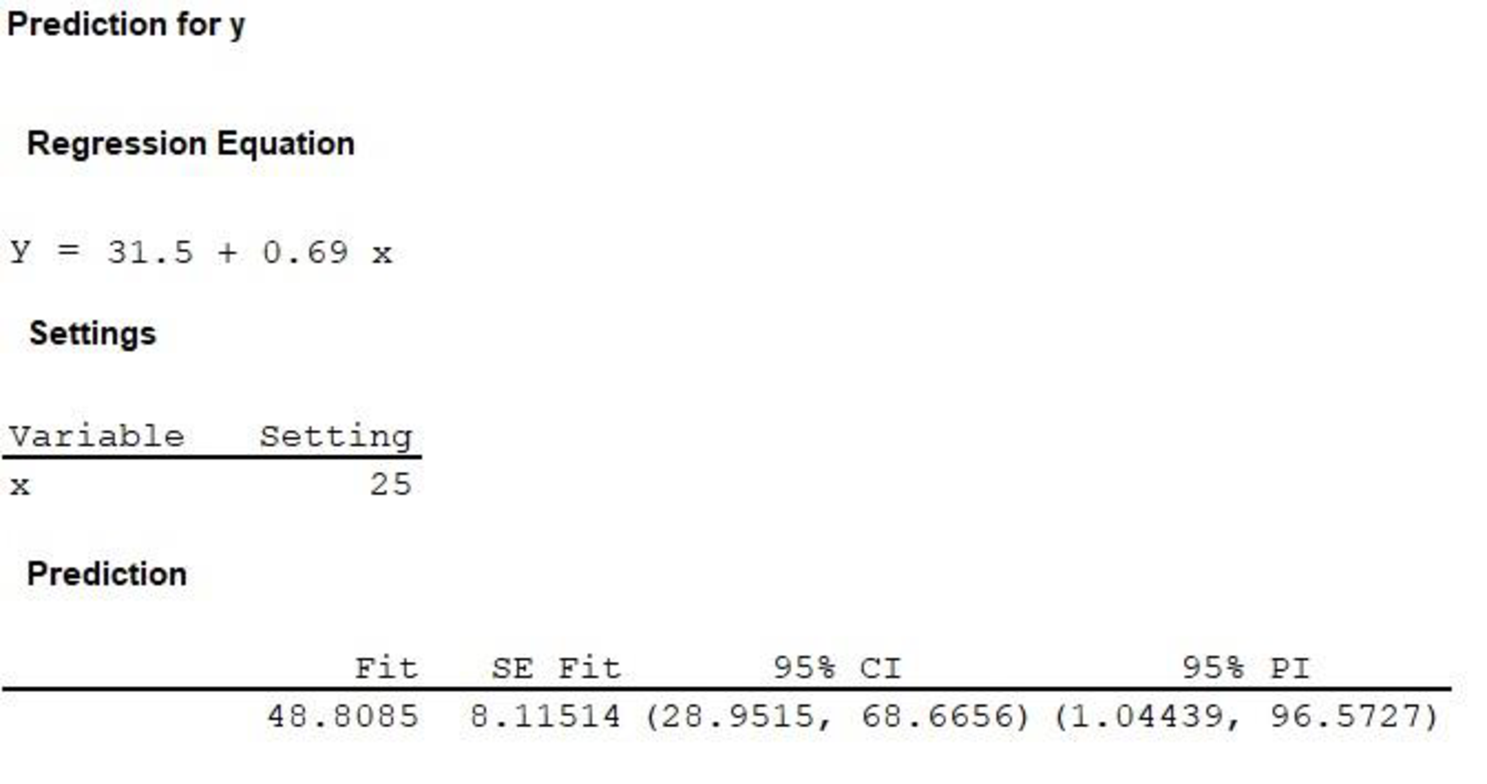
Interpretation:

Thus, the 95% confidence interval for the mean response for the given value of x is $\left(28.9515,68.6656\right)$

g.

To determine

Construct the 95% prediction interval for the individual response for the given value of x.

Answer to Problem 10E

The 95% prediction interval for the individual response for the given value of x is $\left(1.04439,96.5727\right)$.

Explanation of Solution

From the MINITAB output obtained in the previous part (f) it can be observed that the 95% prediction interval for the individual response for the given value of x is $\left(1.04439,96.5727\right)$