Mechanics of Materials (10th Edition)
10th Edition
ISBN: 9780134319650
Author: Russell C. Hibbeler
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Videos
Textbook Question
Chapter 11, Problem 11.6RP
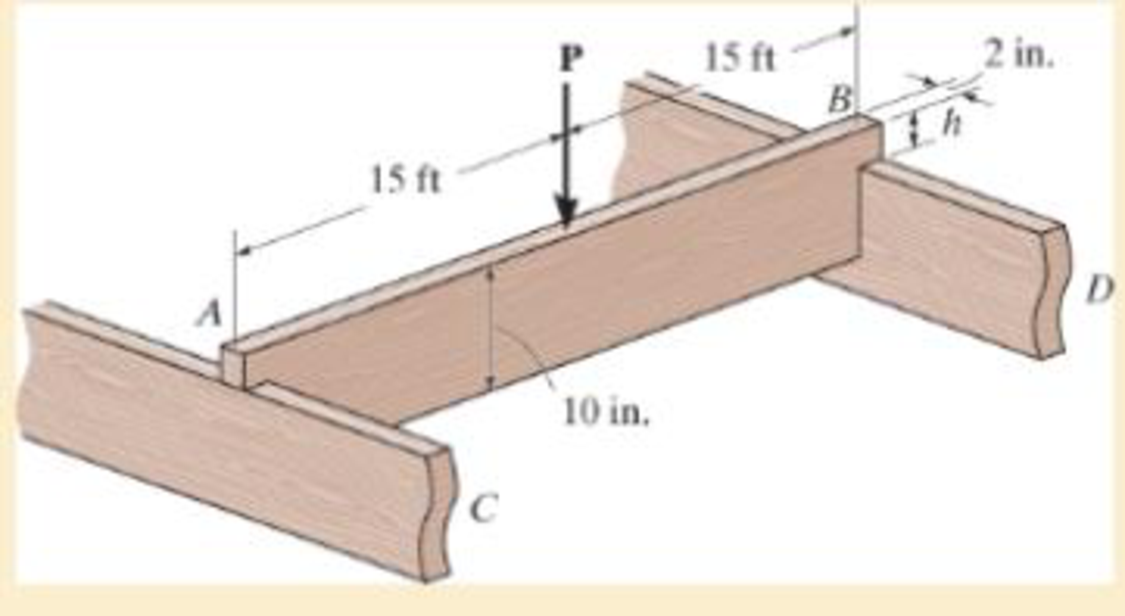
The simply supported joist is used in the construction of a floor for a building. In order to keep the floor low with respect to the sill beams C and D, the ends of the joists are notched as shown. If the allowable shear stress is τallow = 350 psi and the allowable bending stress is σallow = 1500 psi, determine the height h that will cause the beam to reach both allowable stresses at the same time. Also, what load P causes this to happen? Neglect the stress concentration at the notch.
Expert Solution & Answer
Want to see the full answer?
Check out a sample textbook solution
Students have asked these similar questions
Show all work. Indicate the origin that is used for each plane.
Identify the Miller indices for the following planes.
N
23
1
A)
X
B)
y
the following table gives weight gain time data for the oxidation of some metal at an elevated temperature
W(mg/cm2). Time (min)
4.66 20
11.7 50
41.1 175
a) determin whether the oxidation kinetics obey a linear, parabolic, or logarithmic rate expression.
b) Now compute W after a time of 1000 min
A cylindrical specimen of aluminum is pulled in tension. Use the stress v. strain plot below
for this specimen of Al to answer parts (a) - (f). Hint: Each strain increment is 0.004.
Be sure to include your engineering problem solving method per the class rubric.
400
350
300
250
Stress
(MPa) 200
150
100
50
Aluminum (Stress v. Strain)
0
0
0.02
0.04
0.06
0.08
Strain
0.1
0.12
0.14
0.16
a. Compute the modulus of elasticity.
b. Determine the yield strength at a strain offset of 0.002.
c. Determine the tensile strength of this metal.
d. Compute the ductility in percent elongation.
e. Compute the modulus of resilience.
f. Determine the elastic strain recovery for an unloaded stress of 340 MPa.
Chapter 11 Solutions
Mechanics of Materials (10th Edition)
Ch. 11.2 - Determine the minimum dimension a to the nearest...Ch. 11.2 - of the rod to safely support the load. The rod is...Ch. 11.2 - The wood has an allowable normal stress of allow =...Ch. 11.2 - of the beam's cross section to safely support the...Ch. 11.2 - Determine the minimum dimension b to the nearest...Ch. 11.2 - The beam is made of steel having an allowable...Ch. 11.2 - Determine its dimensions if it is to be...Ch. 11.2 - Determine the minimum width of the beam to the...Ch. 11.2 - if P=10 kip.Ch. 11.2 - If the allowable bending stress is allow = 22 ksi...
Ch. 11.2 - The allowable bending stress is allow = 24 ksi and...Ch. 11.2 - The allowable bending stress is allow = 22 ksi and...Ch. 11.2 - The allowable bending stress is allow = 24 ksi and...Ch. 11.2 - Select the lightest-weight wide-flange beam from...Ch. 11.2 - The beam has an allowable normal stress of allow =...Ch. 11.2 - The beam has an allowable normal stress of allow...Ch. 11.2 - If each nail can support a shear force of 200 lb....Ch. 11.2 - If each beam is to be designed to carry 90 lb/ft...Ch. 11.2 - Determine its height h so that it simultaneously...Ch. 11.2 - The beam is constructed from four boards. If each...Ch. 11.2 - Prob. 11.15PCh. 11.2 - The beam has an allowable normal stress of allow....Ch. 11.2 - Determine the maximum cable force P that can...Ch. 11.2 - to safely support the load. The wood has an...Ch. 11.2 - and the wood has an allowable normal stress of...Ch. 11.2 - If the glue can support a shear stress of allow, =...Ch. 11.2 - If the allowable bending stress is allow = 6 MPa,...Ch. 11.2 - Determine the width b if the height h=2b.Ch. 11.2 - The allowable bending stress is allow = 24 ksi and...Ch. 11.2 - if allow = 30 ksi and allow = 15 ksi. The journal...Ch. 11.2 - if allow = 30 ksi and allow = 15 ksi. The journal...Ch. 11.2 - Select the lightest-weight wide-flange beam from...Ch. 11.2 - The allowable bending stress is allow = 30 ksi and...Ch. 11.2 - The allowable bending stress is allow = 30 ksi and...Ch. 11.2 - If the maximum bending stress is not to exceed...Ch. 11.2 - Determine the maximum load that can safely be...Ch. 11.4 - Determine the variation in the width was a...Ch. 11.4 - The tapered beam supports a uniform distributed...Ch. 11.4 - The tapered beam supports the concentrated force P...Ch. 11.4 - The beam is made from a plate that has a constant...Ch. 11.4 - Determine the variation in the depth d of a...Ch. 11.4 - Determine the variation of the radius r of the...Ch. 11.4 - Prob. 11.37PCh. 11.4 - Determine the variation in the width b as a...Ch. 11.4 - The tubular shaft has an inner diameter of 15 mm....Ch. 11.4 - Prob. 11.40PCh. 11.4 - Prob. 11.41PCh. 11.4 - The pulleys fixed to the shaft are loaded as...Ch. 11.4 - Prob. 11.43PCh. 11.4 - Prob. 11.44PCh. 11.4 - Prob. 11.45PCh. 11 - The cantilevered beam has a circular cross...Ch. 11 - Select the lightest-weight wide-flange overhanging...Ch. 11 - Prob. 11.3RPCh. 11 - Determine the shaft's diameter to the nearest...Ch. 11 - Select the lightest-weight wide-flange beam from...Ch. 11 - The simply supported joist is used in the...Ch. 11 - The simply supported joist is used in the...Ch. 11 - by 4-in. pieces of wood braced as shown. If the...
Additional Engineering Textbook Solutions
Find more solutions based on key concepts
Comprehension Check 7-14
The power absorbed by a resistor can be given by P = I2R, where P is power in units of...
Thinking Like an Engineer: An Active Learning Approach (4th Edition)
What types of coolant are used in vehicles?
Automotive Technology: Principles, Diagnosis, And Service (6th Edition) (halderman Automotive Series)
Assume a telephone signal travels through a cable at two-thirds the speed of light. How long does it take the s...
Electric Circuits. (11th Edition)
The ____________ is always transparent.
Web Development and Design Foundations with HTML5 (8th Edition)
CONCEPT QUESTIONS
15.CQ3 The ball rolls without slipping on the fixed surface as shown. What is the direction ...
Vector Mechanics for Engineers: Statics and Dynamics
17–1C A high-speed aircraft is cruising in still air. How does the temperature of air at the nose of the aircra...
Thermodynamics: An Engineering Approach
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, mechanical-engineering and related others by exploring similar questions and additional content below.Similar questions
- Consider a single crystal of silver oriented such that a tensile stress is applied along a [112] direction. If slip occurs on a (011) plane and in a [111] direction and is initiated at an applied tensile stress of 15.9 MPa, compute the critical resolved shear stress.arrow_forwardA hypothetical component must not fail when a tensile stress of 15.25 MPa is applied. Determine the maximum allowable internal crack length if the surface energy of the component is 1.50 J/m2. Assume a modulus of elasticity of 350 GPa.arrow_forwardFresh air at 21.1 C in which partial pressure of water vapor is 0.018 atmosphere is blown at the rate of 214 m3/h first through a preheater and then adiabatically saturated in spray chambers to 100% saturation and again reheated this reheated air has humidity of 0.024 kg water vapor per kg dry air. It is assumed that the fresh air and the air leaving the re-heater have the same percentage humidity. Determine:- a- The temperature of preheater, spray-chamber and re-heater b- Heat requirement for preheating and re-heating 11:39 مarrow_forward
- Example(3): 0.15 kg/s steam at atmospheric pressure and superheated to 400 K is bled into an air stream at 320 K and 20 per cent relative humidity. What is the temperature, enthalpy, and relative humidity of the mixed stream if the air is flowing at 5 kg/ s? How much steam would be required to provide an exit temperature of 330 K and what would be the humidity of this mixture? 11:39 مarrow_forwardThe answer to the problem is 31.3rad/s. Please show me how to get the final answerarrow_forwardThe answer to the problem is 1.00 m/s^2. Please show me how to get the final answerarrow_forward
- The answer to the problem is 0.30. Please show me how to get the final answerarrow_forwardThe answer to the problem is 1.96 m/s. Please show me how to get the final answerarrow_forwardA cylindrical tank of diameter D is currently filled with water to a height h, as shown in the figure to the right. Water enters the tank through the pipe at (1) with a cross-sectional area A₁ and a uniform velocity V₁. The height of water in the tank is increasing at a constant rate of 5 mm/s. Given the parameters below, find the volumetric flow rate in the pipe at (2), V2, in cm³/s, and classify it as an inflow or outflow. D = 20 cm h = 0.5 m A₁ = 1 cm² V₁ = 0.1 m/s h 1 V₁ D Pwater = 1,000 kg/m³ V2 2arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Mechanics of Materials (MindTap Course List)Mechanical EngineeringISBN:9781337093347Author:Barry J. Goodno, James M. GerePublisher:Cengage Learning
Mechanics of Materials (MindTap Course List)
Mechanical Engineering
ISBN:9781337093347
Author:Barry J. Goodno, James M. Gere
Publisher:Cengage Learning
Mechanics of Materials Lecture: Beam Design; Author: UWMC Engineering;https://www.youtube.com/watch?v=-wVs5pvQPm4;License: Standard Youtube License