Chapter 10.2, Problem 22P

To determine

The verification of ${\overline{x}}_1\approx 4.00,s_1\approx 2.38,{\overline{x}}_2\approx 5.5,s_2\approx 2.78$ using calculator.

Explanation of Solution

To find the required statistics using the Minitab, follow the below instructions:

Step 1: Go to the Minitab software.

Step 2: Go to Stat > Basic statistics > Display Descriptive statistics.

Step 3: Select dataset ‘Field A’ and dataset ‘Field B’ in variables.

Step 4: Click on OK.

The obtained output is:

Statistics

Variable	N	N*	Mean	SE Mean	StDev	Minimum	Q1	Median	Q3	Maximum
Sample 1	7	0	4.000	0.900	2.380	2.000	2.000	3.000	6.000	8.000
Sample 2	8	0	5.500	0.982	2.777	2.000	3.000	5.500	7.750	10.000

From the above output we have find that the values of ${\overline{x}}_1,s_1,{\overline{x}}_2,s_2$ are approximately ${\overline{x}}_1\approx 4.00,s_1\approx 2.38,{\overline{x}}_2\approx 5.5,s_2\approx 2.78$.

(a)

(i)

To determine

The level of significance, null hypothesis and alternate hypothesis.

Answer to Problem 22P

Solution: The hypotheses are $H_0:{\mu }_1={\mu }_2\text{and}{H}_1:{\mu }_1<{\mu }_2$. The level of significance is 0.05.

Explanation of Solution

The level of significance is 0.05.Since, we want to conduct a test of the claim that population mean time lost due to stressors is greater than the population mean time lost due to intimidators. Therefore the null hypothesis is $H_0:{\mu }_1={\mu }_2$ v/s alternative hypothesisis $H_1:{\mu }_1<{\mu }_2$.

(ii)

To determine

To find: The sampling distribution that should be used along with assumptions and compute the value of the sample test statistic.

Answer to Problem 22P

Solution: We can use student’s t distribution. The sample test statisticis $t=-1.13$.

Explanation of Solution

Calculation:

Let’s assume that the population distributions of time lost due to intimidators and time lost due to stressors are each mound shape and approximately symmetrical. The population standard deviation (${\sigma }_1\text{and}{\sigma }_2$) are also unknown, so we can assume that sample test statistics follows a Student’s t-distribution.

Using ${\overline{x}}_1=4.00,{\overline{x}}_2=5.5,{\mu }_1-{\mu }_2=0,s_1^2={(2.38)}^2,s_2^2={(2.78)}^2,n_1=7,n_2=8$ where ${\overline{x}}_1\text{and}{\overline{x}}_2$ are the sample means for first sample and second sample respectively.

$s_1^2\text{and}{s}_2^2$ are the sample variance for first sample and second sample respectively.

$n_1\text{and}{n}_2$ are the sample size for first sample and second sample respectively.

The sample test statistic t is calculated as follows:

$\begin{array}{l}t=\frac{({\overline{x}}_1-{\overline{x}}_2)-({\mu }_1-{\mu }_2)}{\sqrt{\frac{s_1^2}{n_1}+\frac{s_2^2}{n_2}}}\\ t=\frac{(4.00-5.50)-0}{\sqrt{\frac{{(2.38)}^2}{7}+\frac{{(2.78)}^2}{8}}}\\ t=-1.1258\\ t\approx -1.13\end{array}$

Thus the test statistic is $t=-1.13$.

(iii)

To determine

To find: The P-value of the test statistic and sketch the sampling distribution showing the area corresponding to the P-value.

Answer to Problem 22P

Solution: The P-value of the sample test statistic is 0.1508.

Explanation of Solution

Calculation:

The given hypothesis test is two tailed.

$P-\text{value =}P\left(t<-1.13\right)$

D.F = Smaller of $n_1-1$ and $n_2-1$.

$\begin{array}{l}D.F=n_1-1\\ D.F=7-1\\ D.F=6\end{array}$

By using table 4 from Appendix

$0.125<p-\text{value}<0.250$

Graph:

To draw the required graphs using the Minitab, follow the below instructions:

Step 1: Go to the Minitab software.

Step 2: Go to Graph > Probability distribution plot > View probability.

Step 3: Select ‘t’ and enter D.f = 6.

Step 4: Click on the Shaded area > X value.

Step 5: Enter X-value as -1.13 and select ‘Left tail’.

Step 6: Click on OK.

The obtained distribution graph is:

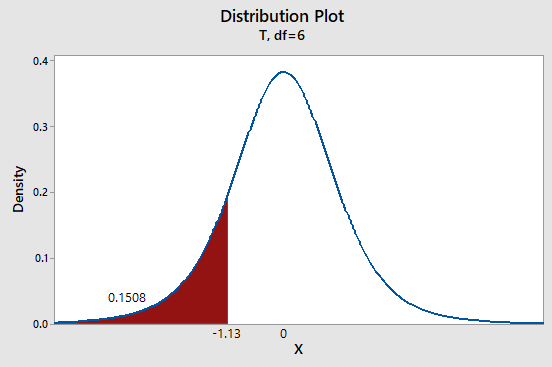
P-value = 0.1508

(iv)

To determine

Whether we reject or fail to reject the null hypothesis and whether the data is statistically significant for a level of significance of 0.05.

Answer to Problem 22P

Solution: The P-value $>\alpha$, hence we fail to reject $H_0$. The data is not statistically significant for a level of significance of 0.05.

Explanation of Solution

The P-value (0.1508) is greater than the level of significance ($\alpha$) of 0.05. Therefore we don’t have enough evidence to reject the null hypothesis $H_0$ i.e. the P-value is not statistically significant.

(v)

To determine

The interpretation for the conclusion.

Answer to Problem 22P

Solution: There is not enough evidence to conclude that population mean time lost due to stressors is greater than the population mean time lost due to intimidators.

Explanation of Solution

The P-value (0.1508) is greater than the level of significance ($\alpha$) of 0.05. Therefore we don’t have enough evidence to reject the null hypothesis $H_0$ i.e. the P-value is not statistically significant. There is not enough evidence to conclude that population mean time lost due to stressors is greater than the population mean time lost due to intimidators.

(b)

To determine

To find: The 90%confidence interval for ${\mu }_1-{\mu }_2$ and explain the meaning of the confidence interval in the context of the problem.

Answer to Problem 22P

Solution:

The 90% confidence interval for the difference of two means is $-4.09<({\mu }_1-{\mu }_2)<1.09$.

Explanation of Solution

Calculation:

The critical t-value for a two-tailed area of 0.10 is 1.943.

The difference of two means is

$\begin{array}{l}({\overline{x}}_1-{\overline{x}}_2)=4.00-5.50\\ ({\overline{x}}_1-{\overline{x}}_2)=-1.50\end{array}$

Now, the margin of error is computed as follows:

$\begin{array}{l}E=t_c\sqrt{\frac{s_1^2}{n_1}+\frac{s_2^2}{n_2}}\\ E=1.943\sqrt{\frac{{(2.38)}^2}{7}+\frac{{(2.78)}^2}{8}}\\ E=2.5888\\ E\approx 2.59\end{array}$

Now the confidence interval for the difference of two means;

$\begin{array}{l}({\overline{x}}_1-{\overline{x}}_2)-E<({\mu }_1-{\mu }_2)<({\overline{x}}_1-{\overline{x}}_2)+E\\ -1.50-2.59<({\mu }_1-{\mu }_2)<-1.50+2.59\\ -4.09<({\mu }_1-{\mu }_2)<1.09\end{array}$

The confidence interval for the difference of two means is $-4.09<({\mu }_1-{\mu }_2)<1.09$.

Interpretation:

At the 90% confidence level, we see that the difference of means ranges from negative to positives values. We cannot tell from this interval if population mean time lost due to stressors is greater than the population mean time lost due to intimidators.