Algebra 1
1st Edition
ISBN: 9780078884801
Author: McGraw-Hill/Glencoe
Publisher: Glencoe/McGraw-Hill School Pub Co
expand_more
expand_more
format_list_bulleted
Videos
Question
Chapter 10, Problem 67SGR
To determine
To calculate the length of the ramp.
Expert Solution & Answer
Answer to Problem 67SGR
6 feet
Explanation of Solution
Given:
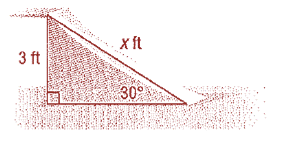
Concept used:
From the given figure,
Height of the ramp is 3 feet.
The angle that it makes with the ground is 30°.
Calculation:
Given that the height of the ramp is 3 feet and the angle that it makes with the ground is 30°.
To calculate the length of the ramp,
Calculating the value of
Conclusion:
Therefore, the length of the ramp is 6 feet.
Chapter 10 Solutions
Algebra 1
Ch. 10.1 - Prob. 1ACYPCh. 10.1 - Prob. 1BCYPCh. 10.1 - Prob. 2ACYPCh. 10.1 - Prob. 2BCYPCh. 10.1 - Prob. 3ACYPCh. 10.1 - Prob. 3BCYPCh. 10.1 - Prob. 4CYPCh. 10.1 - Prob. 5ACYPCh. 10.1 - Prob. 5BCYPCh. 10.1 - Prob. 1CYU
Ch. 10.1 - Prob. 2CYUCh. 10.1 - Prob. 3CYUCh. 10.1 - Prob. 4CYUCh. 10.1 - Prob. 5CYUCh. 10.1 - Prob. 6CYUCh. 10.1 - Prob. 7CYUCh. 10.1 - Prob. 8CYUCh. 10.1 - Prob. 9CYUCh. 10.1 - Prob. 10CYUCh. 10.1 - Prob. 11CYUCh. 10.1 - Prob. 12CYUCh. 10.1 - Prob. 13CYUCh. 10.1 - Prob. 14PPSCh. 10.1 - Prob. 15PPSCh. 10.1 - Prob. 16PPSCh. 10.1 - Prob. 17PPSCh. 10.1 - Prob. 18PPSCh. 10.1 - Prob. 19PPSCh. 10.1 - Prob. 20PPSCh. 10.1 - Prob. 21PPSCh. 10.1 - Prob. 22PPSCh. 10.1 - Prob. 23PPSCh. 10.1 - Prob. 24PPSCh. 10.1 - Prob. 25PPSCh. 10.1 - Prob. 26PPSCh. 10.1 - Prob. 27PPSCh. 10.1 - Prob. 28PPSCh. 10.1 - Prob. 29PPSCh. 10.1 - Prob. 30PPSCh. 10.1 - Prob. 31PPSCh. 10.1 - Prob. 32PPSCh. 10.1 - Prob. 33PPSCh. 10.1 - Prob. 34PPSCh. 10.1 - Prob. 35PPSCh. 10.1 - Prob. 36PPSCh. 10.1 - Prob. 37PPSCh. 10.1 - Prob. 38PPSCh. 10.1 - Prob. 39PPSCh. 10.1 - Prob. 40PPSCh. 10.1 - Prob. 41PPSCh. 10.1 - Prob. 42PPSCh. 10.1 - Prob. 43PPSCh. 10.1 - Prob. 44PPSCh. 10.1 - Prob. 45HPCh. 10.1 - Prob. 46HPCh. 10.1 - Prob. 47HPCh. 10.1 - Prob. 48HPCh. 10.1 - Prob. 49HPCh. 10.1 - Prob. 50HPCh. 10.1 - Prob. 51HPCh. 10.1 - Prob. 52HPCh. 10.1 - Prob. 53STPCh. 10.1 - Prob. 54STPCh. 10.1 - Prob. 55STPCh. 10.1 - Prob. 56STPCh. 10.1 - Prob. 57SRCh. 10.1 - Prob. 58SRCh. 10.1 - Prob. 59SRCh. 10.1 - Prob. 60SRCh. 10.1 - Prob. 61SRCh. 10.1 - Prob. 62SRCh. 10.1 - Prob. 63SRCh. 10.1 - Prob. 64SRCh. 10.1 - Prob. 65SRCh. 10.1 - Prob. 66SCh. 10.1 - Prob. 67SCh. 10.1 - Prob. 68SCh. 10.2 - Prob. 2ACYPCh. 10.2 - Prob. 2BCYPCh. 10.2 - Prob. 3ACYPCh. 10.2 - Prob. 3BCYPCh. 10.2 - Prob. 4CYPCh. 10.2 - Prob. 5ACYPCh. 10.2 - Prob. 5BCYPCh. 10.2 - Prob. 45PPSCh. 10.2 - Prob. 46PPSCh. 10.2 - Prob. 47PPSCh. 10.2 - Prob. 48PPSCh. 10.2 - Prob. 49PPSCh. 10.2 - Prob. 50PPSCh. 10.2 - Prob. 51PPSCh. 10.2 - Prob. 52HPCh. 10.2 - Prob. 53HPCh. 10.2 - Prob. 54HPCh. 10.2 - Prob. 55HPCh. 10.2 - Prob. 56HPCh. 10.2 - Prob. 57HPCh. 10.2 - Prob. 58STPCh. 10.2 - Prob. 59STPCh. 10.2 - Prob. 60STPCh. 10.2 - Prob. 61STPCh. 10.2 - Prob. 62SRCh. 10.2 - Prob. 63SRCh. 10.2 - Prob. 64SRCh. 10.2 - Prob. 65SRCh. 10.2 - Prob. 66SRCh. 10.2 - Prob. 67SRCh. 10.2 - Prob. 68SRCh. 10.2 - Prob. 69SRCh. 10.2 - Prob. 70SRCh. 10.2 - Prob. 71SRCh. 10.2 - Prob. 72SRCh. 10.2 - Prob. 73SRCh. 10.2 - Prob. 74SRCh. 10.2 - Prob. 75SRCh. 10.2 - Prob. 76SRCh. 10.2 - Prob. 77SRCh. 10.2 - Prob. 78SRCh. 10.2 - Prob. 79SRCh. 10.2 - Prob. 80SRCh. 10.2 - Prob. 81SRCh. 10.2 - Prob. 82SRCh. 10.2 - Prob. 83SRCh. 10.2 - Prob. 85SCh. 10.2 - Prob. 86SCh. 10.2 - Prob. 87SCh. 10.2 - Prob. 88SCh. 10.2 - Prob. 89SCh. 10.2 - Prob. 90SCh. 10.2 - Prob. 84SRCh. 10.2 - Prob. 1ACYPCh. 10.2 - Prob. 1BCYPCh. 10.2 - Prob. 1CYUCh. 10.2 - Prob. 2CYUCh. 10.2 - Prob. 3CYUCh. 10.2 - Prob. 4CYUCh. 10.2 - Prob. 5CYUCh. 10.2 - Prob. 6CYUCh. 10.2 - Prob. 7CYUCh. 10.2 - Prob. 8CYUCh. 10.2 - Prob. 9CYUCh. 10.2 - Prob. 10CYUCh. 10.2 - Prob. 11CYUCh. 10.2 - Prob. 12CYUCh. 10.2 - Prob. 13CYUCh. 10.2 - Prob. 14CYUCh. 10.2 - Prob. 15CYUCh. 10.2 - Prob. 16CYUCh. 10.2 - Prob. 17PPSCh. 10.2 - Prob. 18PPSCh. 10.2 - Prob. 19PPSCh. 10.2 - Prob. 20PPSCh. 10.2 - Prob. 21PPSCh. 10.2 - Prob. 22PPSCh. 10.2 - Prob. 23PPSCh. 10.2 - Prob. 24PPSCh. 10.2 - Prob. 25PPSCh. 10.2 - Prob. 26PPSCh. 10.2 - Prob. 27PPSCh. 10.2 - Prob. 28PPSCh. 10.2 - Prob. 29PPSCh. 10.2 - Prob. 30PPSCh. 10.2 - Prob. 31PPSCh. 10.2 - Prob. 32PPSCh. 10.2 - Prob. 33PPSCh. 10.2 - Prob. 34PPSCh. 10.2 - Prob. 35PPSCh. 10.2 - Prob. 36PPSCh. 10.2 - Prob. 37PPSCh. 10.2 - Prob. 38PPSCh. 10.2 - Prob. 39PPSCh. 10.2 - Prob. 40PPSCh. 10.2 - Prob. 41PPSCh. 10.2 - Prob. 42PPSCh. 10.2 - Prob. 43PPSCh. 10.2 - Prob. 44PPSCh. 10.3 - Prob. 1ACYPCh. 10.3 - Prob. 1BCYPCh. 10.3 - Prob. 1CCYPCh. 10.3 - Prob. 1DCYPCh. 10.3 - Prob. 2ACYPCh. 10.3 - Prob. 2BCYPCh. 10.3 - Prob. 2CCYPCh. 10.3 - Prob. 2DCYPCh. 10.3 - Prob. 3ACYPCh. 10.3 - Prob. 3BCYPCh. 10.3 - Prob. 3CCYPCh. 10.3 - Prob. 3DCYPCh. 10.3 - Prob. 4CYPCh. 10.3 - Prob. 1CYUCh. 10.3 - Prob. 2CYUCh. 10.3 - Prob. 3CYUCh. 10.3 - Prob. 4CYUCh. 10.3 - Prob. 5CYUCh. 10.3 - Prob. 6CYUCh. 10.3 - Prob. 7CYUCh. 10.3 - Prob. 8CYUCh. 10.3 - Prob. 9CYUCh. 10.3 - Prob. 10CYUCh. 10.3 - Prob. 11CYUCh. 10.3 - Prob. 12CYUCh. 10.3 - Prob. 13CYUCh. 10.3 - Prob. 14PPSCh. 10.3 - Prob. 15PPSCh. 10.3 - Prob. 16PPSCh. 10.3 - Prob. 17PPSCh. 10.3 - Prob. 18PPSCh. 10.3 - Prob. 19PPSCh. 10.3 - Prob. 20PPSCh. 10.3 - Prob. 21PPSCh. 10.3 - Prob. 22PPSCh. 10.3 - Prob. 23PPSCh. 10.3 - Prob. 24PPSCh. 10.3 - Prob. 25PPSCh. 10.3 - Prob. 26PPSCh. 10.3 - Prob. 27PPSCh. 10.3 - Prob. 28PPSCh. 10.3 - Prob. 29PPSCh. 10.3 - Prob. 30PPSCh. 10.3 - Prob. 31PPSCh. 10.3 - Prob. 32PPSCh. 10.3 - Prob. 33PPSCh. 10.3 - Prob. 34PPSCh. 10.3 - Prob. 35PPSCh. 10.3 - Prob. 36HPCh. 10.3 - Prob. 37HPCh. 10.3 - Prob. 38HPCh. 10.3 - Prob. 39HPCh. 10.3 - Prob. 40STPCh. 10.3 - Prob. 41STPCh. 10.3 - Prob. 42STPCh. 10.3 - Prob. 43STPCh. 10.3 - Prob. 44SRCh. 10.3 - Prob. 45SRCh. 10.3 - Prob. 46SRCh. 10.3 - Prob. 47SRCh. 10.3 - Prob. 48SRCh. 10.3 - Prob. 49SRCh. 10.3 - Prob. 50SRCh. 10.3 - Prob. 51SRCh. 10.3 - Prob. 52SRCh. 10.3 - Prob. 53SRCh. 10.3 - Prob. 54SRCh. 10.3 - Prob. 55SRCh. 10.3 - Prob. 56SRCh. 10.3 - Prob. 57SRCh. 10.3 - Prob. 58SRCh. 10.3 - Prob. 59SRCh. 10.3 - Prob. 60SRCh. 10.3 - Prob. 61SRCh. 10.3 - Prob. 62SRCh. 10.3 - Prob. 63SCh. 10.3 - Prob. 64SCh. 10.3 - Prob. 65SCh. 10.3 - Prob. 66SCh. 10.3 - Prob. 67SCh. 10.3 - Prob. 68SCh. 10.4 - Prob. 1CYUCh. 10.4 - Prob. 2CYUCh. 10.4 - Prob. 3CYUCh. 10.4 - Prob. 4CYUCh. 10.4 - Prob. 5CYUCh. 10.4 - Prob. 6CYUCh. 10.4 - Prob. 7CYUCh. 10.4 - Prob. 8PPSCh. 10.4 - Prob. 9PPSCh. 10.4 - Prob. 10PPSCh. 10.4 - Prob. 11PPSCh. 10.4 - Prob. 12PPSCh. 10.4 - Prob. 13PPSCh. 10.4 - Prob. 14PPSCh. 10.4 - Prob. 15PPSCh. 10.4 - Prob. 16PPSCh. 10.4 - Prob. 17PPSCh. 10.4 - Prob. 18PPSCh. 10.4 - Prob. 19PPSCh. 10.4 - Prob. 20PPSCh. 10.4 - Prob. 21PPSCh. 10.4 - Prob. 22PPSCh. 10.4 - Prob. 23PPSCh. 10.4 - Prob. 24PPSCh. 10.4 - Prob. 25PPSCh. 10.4 - Prob. 26PPSCh. 10.4 - Prob. 27PPSCh. 10.4 - Prob. 28PPSCh. 10.4 - Prob. 30PPSCh. 10.4 - Prob. 31HPCh. 10.4 - Prob. 32HPCh. 10.4 - Prob. 33HPCh. 10.4 - Prob. 34HPCh. 10.4 - Prob. 35HPCh. 10.4 - Prob. 36HPCh. 10.4 - Prob. 38STPCh. 10.4 - Prob. 39STPCh. 10.4 - Prob. 40STPCh. 10.4 - Prob. 41STPCh. 10.4 - Prob. 42SRCh. 10.4 - Prob. 43SRCh. 10.4 - Prob. 44SRCh. 10.4 - Prob. 45SRCh. 10.4 - Prob. 46SRCh. 10.4 - Prob. 47SRCh. 10.4 - Prob. 48SRCh. 10.4 - Prob. 49SRCh. 10.4 - Prob. 50SRCh. 10.4 - Prob. 51SRCh. 10.4 - Prob. 52SRCh. 10.4 - Prob. 53SRCh. 10.4 - Prob. 54SRCh. 10.4 - Prob. 55SRCh. 10.4 - Prob. 56SRCh. 10.4 - Prob. 57SRCh. 10.4 - Prob. 58SRCh. 10.4 - Prob. 59SRCh. 10.4 - Prob. 60SRCh. 10.4 - Prob. 61SRCh. 10.4 - Prob. 62SCh. 10.4 - Prob. 63SCh. 10.4 - Prob. 64SCh. 10.4 - Prob. 65SCh. 10.4 - Prob. 66SCh. 10.4 - Prob. 67SCh. 10.4 - Prob. 1CYPCh. 10.4 - Prob. 2ACYPCh. 10.4 - Prob. 2BCYPCh. 10.4 - Prob. 3ACYPCh. 10.4 - Prob. 3BCYPCh. 10.4 - Prob. 29PPSCh. 10.4 - Prob. 37HPCh. 10.5 - Prob. 1CYUCh. 10.5 - Prob. 2CYUCh. 10.5 - Prob. 3CYUCh. 10.5 - Prob. 4CYUCh. 10.5 - Prob. 6CYUCh. 10.5 - Prob. 7CYUCh. 10.5 - Prob. 8CYUCh. 10.5 - Prob. 9CYUCh. 10.5 - Prob. 10PPSCh. 10.5 - Prob. 11PPSCh. 10.5 - Prob. 12PPSCh. 10.5 - Prob. 13PPSCh. 10.5 - Prob. 14PPSCh. 10.5 - Prob. 15PPSCh. 10.5 - Prob. 16PPSCh. 10.5 - Prob. 17PPSCh. 10.5 - Prob. 18PPSCh. 10.5 - Prob. 19PPSCh. 10.5 - Prob. 20PPSCh. 10.5 - Prob. 21PPSCh. 10.5 - Prob. 22PPSCh. 10.5 - Prob. 23PPSCh. 10.5 - Prob. 24PPSCh. 10.5 - Prob. 25PPSCh. 10.5 - Prob. 26PPSCh. 10.5 - Prob. 27PPSCh. 10.5 - Prob. 28PPSCh. 10.5 - Prob. 30PPSCh. 10.5 - Prob. 31PPSCh. 10.5 - Prob. 32PPSCh. 10.5 - Prob. 33PPSCh. 10.5 - Prob. 34PPSCh. 10.5 - Prob. 35PPSCh. 10.5 - Prob. 36PPSCh. 10.5 - Prob. 37PPSCh. 10.5 - Prob. 39PPSCh. 10.5 - Prob. 40PPSCh. 10.5 - Prob. 41PPSCh. 10.5 - Prob. 42PPSCh. 10.5 - Prob. 43PPSCh. 10.5 - Prob. 44PPSCh. 10.5 - Prob. 45PPSCh. 10.5 - Prob. 46PPSCh. 10.5 - Prob. 47PPSCh. 10.5 - Prob. 48PPSCh. 10.5 - Prob. 49PPSCh. 10.5 - Prob. 50HPCh. 10.5 - Prob. 51HPCh. 10.5 - Prob. 52HPCh. 10.5 - Prob. 53HPCh. 10.5 - Prob. 54HPCh. 10.5 - Prob. 55STPCh. 10.5 - Prob. 56STPCh. 10.5 - Prob. 57STPCh. 10.5 - Prob. 58STPCh. 10.5 - Prob. 59SRCh. 10.5 - Prob. 60SRCh. 10.5 - Prob. 61SRCh. 10.5 - Prob. 62SRCh. 10.5 - Prob. 63SRCh. 10.5 - Prob. 64SRCh. 10.5 - Prob. 65SRCh. 10.5 - Prob. 66SRCh. 10.5 - Prob. 67SRCh. 10.5 - Prob. 68SRCh. 10.5 - Prob. 69SRCh. 10.5 - Prob. 70SRCh. 10.5 - Prob. 71SRCh. 10.5 - Prob. 72SRCh. 10.5 - Prob. 73SRCh. 10.5 - Prob. 74SRCh. 10.5 - Prob. 75SRCh. 10.5 - Prob. 76SRCh. 10.5 - Prob. 77SRCh. 10.5 - Prob. 78SRCh. 10.5 - Prob. 1ACYPCh. 10.5 - Prob. 1BCYPCh. 10.5 - Prob. 2CYPCh. 10.5 - Prob. 3ACYPCh. 10.5 - Prob. 3BCYPCh. 10.5 - Prob. 5CYUCh. 10.5 - Prob. 29PPSCh. 10.5 - Prob. 38PPSCh. 10.5 - Prob. 79SRCh. 10.5 - Prob. 80SRCh. 10.5 - Prob. 81SRCh. 10.5 - Prob. 82SRCh. 10.5 - Prob. 83SRCh. 10.5 - Prob. 84SRCh. 10.5 - Prob. 85SCh. 10.5 - Prob. 86SCh. 10.5 - Prob. 87SCh. 10.5 - Prob. 88SCh. 10.6 - Prob. 1ACYPCh. 10.6 - Prob. 1BCYPCh. 10.6 - Prob. 3CYPCh. 10.6 - Prob. 4ACYPCh. 10.6 - Prob. 4BCYPCh. 10.6 - Prob. 4CCYPCh. 10.6 - Prob. 1CYUCh. 10.6 - Prob. 2CYUCh. 10.6 - Prob. 3CYUCh. 10.6 - Prob. 4CYUCh. 10.6 - Prob. 5CYUCh. 10.6 - Prob. 6CYUCh. 10.6 - Prob. 7CYUCh. 10.6 - Prob. 8CYUCh. 10.6 - Prob. 9CYUCh. 10.6 - Prob. 10CYUCh. 10.6 - Prob. 11CYUCh. 10.6 - Prob. 12CYUCh. 10.6 - Prob. 13CYUCh. 10.6 - Prob. 14CYUCh. 10.6 - Prob. 15CYUCh. 10.6 - Prob. 16CYUCh. 10.6 - Prob. 17CYUCh. 10.6 - Prob. 18PPSCh. 10.6 - Prob. 19PPSCh. 10.6 - Prob. 20PPSCh. 10.6 - Prob. 21PPSCh. 10.6 - Prob. 22PPSCh. 10.6 - Prob. 23PPSCh. 10.6 - Prob. 24PPSCh. 10.6 - Prob. 25PPSCh. 10.6 - Prob. 26PPSCh. 10.6 - Prob. 27PPSCh. 10.6 - Prob. 28PPSCh. 10.6 - Prob. 29PPSCh. 10.6 - Prob. 30PPSCh. 10.6 - Prob. 31PPSCh. 10.6 - Prob. 32PPSCh. 10.6 - Prob. 33PPSCh. 10.6 - Prob. 34PPSCh. 10.6 - Prob. 35PPSCh. 10.6 - Prob. 36PPSCh. 10.6 - Prob. 37PPSCh. 10.6 - Prob. 38PPSCh. 10.6 - Prob. 39PPSCh. 10.6 - Prob. 40PPSCh. 10.6 - Prob. 41PPSCh. 10.6 - Prob. 42PPSCh. 10.6 - Prob. 43PPSCh. 10.6 - Prob. 44PPSCh. 10.6 - Prob. 45PPSCh. 10.6 - Prob. 46PPSCh. 10.6 - Prob. 47PPSCh. 10.6 - Prob. 49PPSCh. 10.6 - Prob. 50PPSCh. 10.6 - Prob. 51PPSCh. 10.6 - Prob. 52PPSCh. 10.6 - Prob. 53PPSCh. 10.6 - Prob. 54HPCh. 10.6 - Prob. 55HPCh. 10.6 - Prob. 57HPCh. 10.6 - Prob. 58HPCh. 10.6 - Prob. 59STPCh. 10.6 - Prob. 60STPCh. 10.6 - Prob. 61STPCh. 10.6 - Prob. 62STPCh. 10.6 - Prob. 63SRCh. 10.6 - Prob. 64SRCh. 10.6 - Prob. 65SRCh. 10.6 - Prob. 66SRCh. 10.6 - Prob. 67SRCh. 10.6 - Prob. 68SRCh. 10.6 - Prob. 69SRCh. 10.6 - Prob. 70SCh. 10.6 - Prob. 71SCh. 10.6 - Prob. 72SCh. 10.6 - Prob. 73SCh. 10.6 - Prob. 74SCh. 10.6 - Prob. 75SCh. 10.6 - Prob. 2CYPCh. 10.6 - Prob. 48PPSCh. 10.6 - Prob. 56HPCh. 10.7 - Prob. 1CYPCh. 10.7 - Prob. 2CYPCh. 10.7 - Prob. 3ACYPCh. 10.7 - Prob. 3BCYPCh. 10.7 - Prob. 4CYPCh. 10.7 - Prob. 1CYUCh. 10.7 - Prob. 2CYUCh. 10.7 - Prob. 3CYUCh. 10.7 - Prob. 4CYUCh. 10.7 - Prob. 5CYUCh. 10.7 - Prob. 6CYUCh. 10.7 - Prob. 7CYUCh. 10.7 - Prob. 8PPSCh. 10.7 - Prob. 9PPSCh. 10.7 - Prob. 10PPSCh. 10.7 - Prob. 11PPSCh. 10.7 - Prob. 12PPSCh. 10.7 - Prob. 13PPSCh. 10.7 - Prob. 14PPSCh. 10.7 - Prob. 15PPSCh. 10.7 - Prob. 16PPSCh. 10.7 - Prob. 17PPSCh. 10.7 - Prob. 18PPSCh. 10.7 - Prob. 19PPSCh. 10.7 - Prob. 22PPSCh. 10.7 - Prob. 26HPCh. 10.7 - Prob. 27HPCh. 10.7 - Prob. 28HPCh. 10.7 - Prob. 30HPCh. 10.7 - Prob. 31STPCh. 10.7 - Prob. 32STPCh. 10.7 - Prob. 33STPCh. 10.7 - Prob. 34STPCh. 10.7 - Prob. 35SRCh. 10.7 - Prob. 36SRCh. 10.7 - Prob. 37SRCh. 10.7 - Prob. 38SRCh. 10.7 - Prob. 39SRCh. 10.7 - Prob. 40SRCh. 10.7 - Prob. 41SRCh. 10.7 - Prob. 42SRCh. 10.7 - Prob. 43SRCh. 10.7 - Prob. 44SRCh. 10.7 - Prob. 45SRCh. 10.7 - Prob. 46SRCh. 10.7 - Prob. 47SRCh. 10.7 - Prob. 48SRCh. 10.7 - Prob. 49SRCh. 10.7 - Prob. 50SRCh. 10.7 - Prob. 51SRCh. 10.7 - Prob. 52SRCh. 10.7 - Prob. 53SRCh. 10.7 - Prob. 54SRCh. 10.7 - Prob. 55SCh. 10.7 - Prob. 56SCh. 10.7 - Prob. 57SCh. 10.7 - Prob. 58SCh. 10.7 - Prob. 59SCh. 10.7 - Prob. 20PPSCh. 10.7 - Prob. 21PPSCh. 10.7 - Prob. 23PPSCh. 10.7 - Prob. 24PPSCh. 10.7 - Prob. 25PPSCh. 10.7 - Prob. 29HPCh. 10.8 - Prob. 2ACYPCh. 10.8 - Prob. 2BCYPCh. 10.8 - Prob. 2CCYPCh. 10.8 - Prob. 3ACYPCh. 10.8 - Prob. 3BCYPCh. 10.8 - Prob. 4CYPCh. 10.8 - Prob. 5CYPCh. 10.8 - Prob. 1CYUCh. 10.8 - Prob. 2CYUCh. 10.8 - Prob. 3CYUCh. 10.8 - Prob. 4CYUCh. 10.8 - Prob. 5CYUCh. 10.8 - Prob. 6CYUCh. 10.8 - Prob. 7CYUCh. 10.8 - Prob. 8CYUCh. 10.8 - Prob. 9CYUCh. 10.8 - Prob. 10CYUCh. 10.8 - Prob. 11CYUCh. 10.8 - Prob. 12CYUCh. 10.8 - Prob. 13CYUCh. 10.8 - Prob. 14CYUCh. 10.8 - Prob. 15CYUCh. 10.8 - Prob. 16CYUCh. 10.8 - Prob. 17CYUCh. 10.8 - Prob. 18PPSCh. 10.8 - Prob. 19PPSCh. 10.8 - Prob. 20PPSCh. 10.8 - Prob. 21PPSCh. 10.8 - Prob. 22PPSCh. 10.8 - Prob. 23PPSCh. 10.8 - Prob. 24PPSCh. 10.8 - Prob. 25PPSCh. 10.8 - Prob. 26PPSCh. 10.8 - Prob. 27PPSCh. 10.8 - Prob. 28PPSCh. 10.8 - Prob. 29PPSCh. 10.8 - Prob. 30PPSCh. 10.8 - Prob. 31PPSCh. 10.8 - Prob. 32PPSCh. 10.8 - Prob. 33PPSCh. 10.8 - Prob. 34PPSCh. 10.8 - Prob. 35PPSCh. 10.8 - Prob. 36PPSCh. 10.8 - Prob. 37PPSCh. 10.8 - Prob. 38PPSCh. 10.8 - Prob. 39PPSCh. 10.8 - Prob. 40PPSCh. 10.8 - Prob. 41PPSCh. 10.8 - Prob. 42PPSCh. 10.8 - Prob. 43PPSCh. 10.8 - Prob. 44PPSCh. 10.8 - Prob. 45PPSCh. 10.8 - Prob. 46PPSCh. 10.8 - Prob. 47PPSCh. 10.8 - Prob. 48PPSCh. 10.8 - Prob. 49PPSCh. 10.8 - Prob. 50PPSCh. 10.8 - Prob. 51HPCh. 10.8 - Prob. 52HPCh. 10.8 - Prob. 53HPCh. 10.8 - Prob. 54HPCh. 10.8 - Prob. 56STPCh. 10.8 - Prob. 58STPCh. 10.8 - Prob. 60SRCh. 10.8 - Prob. 61SRCh. 10.8 - Prob. 62SRCh. 10.8 - Prob. 63SRCh. 10.8 - Prob. 64SRCh. 10.8 - Prob. 65SRCh. 10.8 - Prob. 66SRCh. 10.8 - Prob. 68SCh. 10.8 - Prob. 69SCh. 10.8 - Prob. 70SCh. 10.8 - Prob. 71SCh. 10.8 - Prob. 1CYPCh. 10.8 - Prob. 55HPCh. 10.8 - Prob. 57STPCh. 10.8 - Prob. 59STPCh. 10.8 - Prob. 67SRCh. 10 - Prob. 11QCCh. 10 - Prob. 12QCCh. 10 - Prob. 13QCCh. 10 - Prob. 14QCCh. 10 - Prob. 15QCCh. 10 - Prob. 16QCCh. 10 - Prob. 17QCCh. 10 - Prob. 18QCCh. 10 - Prob. 1QCCh. 10 - Prob. 2QCCh. 10 - Prob. 3QCCh. 10 - Prob. 4QCCh. 10 - Prob. 5QCCh. 10 - Prob. 6QCCh. 10 - Prob. 7QCCh. 10 - Prob. 8QCCh. 10 - Prob. 9QCCh. 10 - Prob. 10QCCh. 10 - Prob. 1MCQCh. 10 - Prob. 2MCQCh. 10 - Prob. 3MCQCh. 10 - Prob. 4MCQCh. 10 - Prob. 5MCQCh. 10 - Prob. 6MCQCh. 10 - Prob. 7MCQCh. 10 - Prob. 8MCQCh. 10 - Prob. 9MCQCh. 10 - Prob. 10MCQCh. 10 - Prob. 11MCQCh. 10 - Prob. 12MCQCh. 10 - Prob. 14MCQCh. 10 - Prob. 15MCQCh. 10 - Prob. 16MCQCh. 10 - Prob. 17MCQCh. 10 - Prob. 18MCQCh. 10 - Prob. 19MCQCh. 10 - Prob. 20MCQCh. 10 - Prob. 21MCQCh. 10 - Prob. 22MCQCh. 10 - Prob. 23MCQCh. 10 - Prob. 24MCQCh. 10 - Prob. 25MCQCh. 10 - Prob. 26MCQCh. 10 - Prob. 27MCQCh. 10 - Prob. 28MCQCh. 10 - Prob. 29MCQCh. 10 - Prob. 1SGRCh. 10 - Prob. 2SGRCh. 10 - Prob. 3SGRCh. 10 - Prob. 4SGRCh. 10 - Prob. 5SGRCh. 10 - Prob. 6SGRCh. 10 - Prob. 7SGRCh. 10 - Prob. 8SGRCh. 10 - Prob. 9SGRCh. 10 - Prob. 10SGRCh. 10 - Prob. 11SGRCh. 10 - Prob. 12SGRCh. 10 - Prob. 13SGRCh. 10 - Prob. 14SGRCh. 10 - Prob. 15SGRCh. 10 - Prob. 16SGRCh. 10 - Prob. 17SGRCh. 10 - Prob. 18SGRCh. 10 - Prob. 19SGRCh. 10 - Prob. 20SGRCh. 10 - Prob. 21SGRCh. 10 - Prob. 22SGRCh. 10 - Prob. 23SGRCh. 10 - Prob. 24SGRCh. 10 - Prob. 25SGRCh. 10 - Prob. 26SGRCh. 10 - Prob. 27SGRCh. 10 - Prob. 28SGRCh. 10 - Prob. 29SGRCh. 10 - Prob. 30SGRCh. 10 - Prob. 31SGRCh. 10 - Prob. 32SGRCh. 10 - Prob. 33SGRCh. 10 - Prob. 34SGRCh. 10 - Prob. 35SGRCh. 10 - Prob. 36SGRCh. 10 - Prob. 37SGRCh. 10 - Prob. 38SGRCh. 10 - Prob. 39SGRCh. 10 - Prob. 40SGRCh. 10 - Prob. 41SGRCh. 10 - Prob. 42SGRCh. 10 - Prob. 43SGRCh. 10 - Prob. 44SGRCh. 10 - Prob. 45SGRCh. 10 - Prob. 46SGRCh. 10 - Prob. 47SGRCh. 10 - Prob. 48SGRCh. 10 - Prob. 49SGRCh. 10 - Prob. 50SGRCh. 10 - Prob. 51SGRCh. 10 - Prob. 52SGRCh. 10 - Prob. 53SGRCh. 10 - Prob. 54SGRCh. 10 - Prob. 55SGRCh. 10 - Prob. 56SGRCh. 10 - Prob. 57SGRCh. 10 - Prob. 58SGRCh. 10 - Prob. 59SGRCh. 10 - Prob. 60SGRCh. 10 - Prob. 61SGRCh. 10 - Prob. 62SGRCh. 10 - Prob. 63SGRCh. 10 - Prob. 64SGRCh. 10 - Prob. 65SGRCh. 10 - Prob. 66SGRCh. 10 - Prob. 67SGRCh. 10 - Prob. 1PTCh. 10 - Prob. 2PTCh. 10 - Prob. 3PTCh. 10 - Prob. 4PTCh. 10 - Prob. 5PTCh. 10 - Prob. 6PTCh. 10 - Prob. 7PTCh. 10 - Prob. 8PTCh. 10 - Prob. 9PTCh. 10 - Prob. 10PTCh. 10 - Prob. 11PTCh. 10 - Prob. 12PTCh. 10 - Prob. 13PTCh. 10 - Prob. 14PTCh. 10 - Prob. 15PTCh. 10 - Prob. 16PTCh. 10 - Prob. 17PTCh. 10 - Prob. 18PTCh. 10 - Prob. 19PTCh. 10 - Prob. 20PTCh. 10 - Prob. 21PTCh. 10 - Prob. 22PTCh. 10 - Prob. 23PTCh. 10 - Prob. 25PTCh. 10 - Prob. 27PTCh. 10 - Prob. 1STPCh. 10 - Prob. 2STPCh. 10 - Prob. 3STPCh. 10 - Prob. 4STPCh. 10 - Prob. 5STPCh. 10 - Prob. 6STPCh. 10 - Prob. 7STPCh. 10 - Prob. 8STPCh. 10 - Prob. 9STPCh. 10 - Prob. 11STPCh. 10 - Prob. 12STPCh. 10 - Prob. 13STPCh. 10 - Prob. 14STPCh. 10 - Prob. 15STPCh. 10 - Prob. 13MCQCh. 10 - Prob. 24PTCh. 10 - Prob. 26PTCh. 10 - Prob. 10STPCh. 10 - Prob. 16STP
Additional Math Textbook Solutions
Find more solutions based on key concepts
CHECK POINT 1 Find a counterexample to show that the statement The product of two two-digit numbers is a three-...
Thinking Mathematically (6th Edition)
Rolles Theorem Determine whether Rolles Theorem applies to the following functions on the given interval. If so...
Calculus: Early Transcendentals (2nd Edition)
The graph of the inequality on the number line
Pre-Algebra Student Edition
Mathematical Connections Explain why a number and a numeral are considered different.
A Problem Solving Approach To Mathematics For Elementary School Teachers (13th Edition)
The following set of data is from sample of n=5: a. Compute the mean, median, and mode. b. Compute the range, v...
Basic Business Statistics, Student Value Edition
A box contains 3 marbles: 1 red, 1 green, and 1 blue. Consider an experiment that consists of taking 1 marble f...
A First Course in Probability (10th Edition)
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, algebra and related others by exploring similar questions and additional content below.Similar questions
- 2. Part II Real World Examples. Pesticides Pesticides that kill all insect species are not only bad for the environment, they can also be inefficient at controlling the pest species and can have unintended consequences. Suppose a pest insect species in a particular field has a population x(t) at a particular time t, and suppose its primary predator is another insect species with population y(t) at time t. Suppose the population of these species are accurately modeled by the system dx = 2.4x 1.2xy dt dy = −y+0.9xy dt Also suppose that at time t = 0 a pesticide is applied to the field that reduces both the pest and predator populations to a very small but non-zero numbers. 1. Use the Slopes app (or other similar app) to investigate what happens to this system. Choose 2 sets of initial conditions, both near zero. Let x(0) = 5y(0). Whatever initial values you choose for your first set, the second set of values should be 1/100 of the first. Plot the solutions. Be sure to expand the scale if…arrow_forward1. PART I: Investigating Key Concepts Using Graphs. Although the Lotka-Volterra system can be solved analytically, the solutions are beyond the scope of our class, so we will will explore key concepts such as Cyclic Behavior, Parameter Sensitivity and Equilibrium Points and other features of the Lotka-Volterra predator-prey model graphically by using the Slopes App by Tim Lucas. You can use any other app or program to graph the solutions of the predator-prey system, but the Slopes App is really convenient. Cyclic Behavior: The model predicts that predator and prey populations can exhibit cyclic or oscillatory behavior over time. When the prey population is high, it provides abundant food for predators, causing the predator population to increase. As the predator population increases, it exerts greater predation pressure on the prey, causing the prey population to decline. This, in turn, leads to a decrease in the predator population due to reduced food availability. The cycle then…arrow_forwardEquilibrium Points: The model has equilibrium points where both populations remain constant over time. These equilibrium points can be stable or unstable, depending on the values of the model parameters. A stable equilibrium represents a situation where predator and prey popula- tions coexist in a relatively stable manner. In the Lotka-Volterra Model it is rare to find perfect equilibrium solutions, more often the solutions exhibit oscillatory behaviour around the equilibrium points rather than staying at them. To find the equilibrium points for this system we set both dx/dt and dy/dt to zero and solve dx = ax - bxy dt dy dt == -cy + dxy We find we have 2 sets of equilibrium values, (0,0) when both predator and prey populations vanish, and (c/d, a/b) when there are just enough prey to support a constant predator population but there are not too many predators. Answer the following question for the system shown below. dx dt = 2.4x-1.2xy 33 dy dt = −y + xy 11. Find the equilibrium values…arrow_forward
- 2x^3-18x^2+35x+17=0, solve for xarrow_forwardL Question 7, 4.5.85 Part 1 of 4 > 527¢er 12/06/24 11:2 HW Score: 91.84%, 6.43 of 7 points Points: 0.43 of 1 The population, P, in thousands, of a resort community is given 100- 500t by P(t) = 31 ² +9 , t≥0, where t is time, in months. a) Find the population at t = 0, 1, 3, and 8 months. 80- 60- Ay b) Find the horizontal asymptote of the graph and determine the 40- A value that P(t) approaches as t goes to co? 20- c) Explain the meaning of the answer to part (b) in terms of the application. 0- 10+ 0 10 20 30 40 50 a) At t = 0, the population is At t = 1, the population is At t = 3, the population is At t=8, the population is (Simplify your answers. Round to the nearest integer as needed.) H Vo S (1,1) More View an example Get more help - Clear all Skill builder Check answ 4 Music DEC άtv Ը Warrow_forwardrth and K L Question 5, 5.6.11 Part 2 of 7 Complete the table given below. 627¢erwin=yes 12/06/24 10:57 PM > HW Score: 83.33%, 5 of 6 points C Sav × Points: 0 of 1 (Simplify your answers. Do not round until the final answers. Then round to four decimal places as needed.) Radioactive Substance a) Polonium (Po-200) Decay Rate, k Half-Life T 6.0274 % per min 11.5 mins b) Lead (Pb-196) % per min 37.0 mins View an example Get more help - A DEC 6 M tv P Clear all Skill builder Check answe A NA Warrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON
Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON
Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education
Algebra and Trigonometry (6th Edition)
Algebra
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:PEARSON
Contemporary Abstract Algebra
Algebra
ISBN:9781305657960
Author:Joseph Gallian
Publisher:Cengage Learning
Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning
Algebra And Trigonometry (11th Edition)
Algebra
ISBN:9780135163078
Author:Michael Sullivan
Publisher:PEARSON
Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:9780980232776
Author:Gilbert Strang
Publisher:Wellesley-Cambridge Press
College Algebra (Collegiate Math)
Algebra
ISBN:9780077836344
Author:Julie Miller, Donna Gerken
Publisher:McGraw-Hill Education
Quadrilaterals: Missing Angles and Sides; Author: rhornfeck;https://www.youtube.com/watch?v=knVj1O0L2TM;License: Standard YouTube License, CC-BY
STD IX | State Board | Types of Quadrilateral; Author: Robomate;https://www.youtube.com/watch?v=wh0KQ4UB0EU;License: Standard YouTube License, CC-BY