Chapter 10, Problem 21P

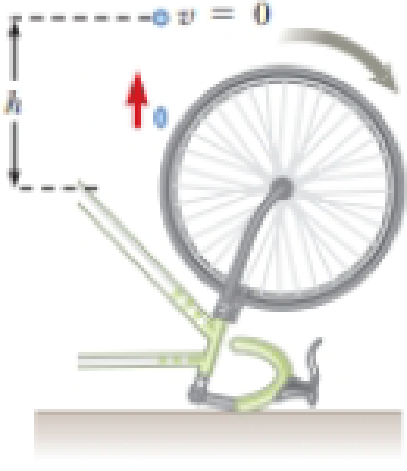
You have just bought a new bicycle. On your first riding trip, it seems that the hike comes to rest relatively quickly after you stop pedaling and let the bicycle coast on flat ground. You call the bicycle shop from which you purchased the vehicle and describe the problem. The technician says that they will replace the bearings in the wheels or do whatever else is necessary if you can prove that the frictional torque in the axle of the wheels is worse than −0.02 N · m. At first, you are discouraged by the technical sound of what you have been told and by the absence of any tool to measure torque in your garage. But then you remember that you are taking a physics class! You take your bike into the garage, turn it upside down and start spinning the wheel while you think about how to determine the frictional torque. The driveway outside the garage had a small puddle, so you notice that droplets of water are flying off the edge of one point on the tire tangentially, including drops that are projected straight upward, as shown in Figure P10.21. Ah-ha! Here is your torque-measuring method! The upward-projected drops leave the rim of the wheel at the same level as the axle. You measure the height to which a drop rises from the level of the axle: h₁ = 54.0 cm. The wet spot on the tire makes one revolution and another drop is projected upward. You measure its highest point: h₂ = 51.0 cm. You measure the radius of the wheel: r = 0.381 m. Finally, you take the wheel off the bike and find its mass: m = 0.850 kg. Because most of the mass of the wheel is at the tire, you model the wheel as a hoop. What do you tell the technician when you call back?

Figure P10.21