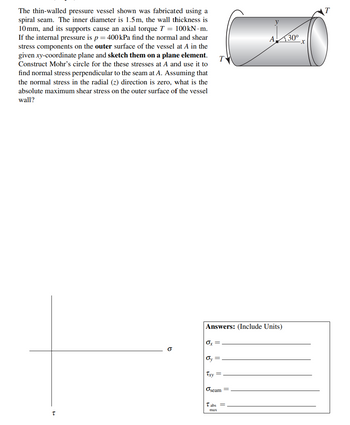
The thin-walled pressure vessel shown was fabricated using a spiral seam. The inner diameter is 1.5m, the wall thickness is 10mm, and its supports cause an axial torque T = 100kN.m. If the internal pressure is p = 400 kPa find the normal and shear stress components on the outer surface of the vessel at A in the given xy-coordinate plane and sketch them on a plane element. Construct Mohr's circle for the these stresses at A and use it to find normal stress perpendicular to the seam at A. Assuming that the normal stress in the radial (z) direction is zero, what is the absolute maximum shear stress on the outer surface of the vessel wall?

Describe the steelmaking process to obtain a cold thin coil, in SAE 1008 steel, MSG 20 (thickness 0.91 mm) plate with a width of 600 mm starting from a hot-rolled plate. MSG 8 (thickness 4.25 mm) with a width of 1200 mm Requirements: LE min = 300 MPa, LR between 400 and 500 MPa and A min = 20%. The sheet must have a good surface finish and dimensional control. Ensure high Tenacity. Cite and justify the necessary process. Cite the hardening mechanisms used

How would you solve this

Complete the following comparison table with the possible answers indicated in each comparison factor: