
Electrical Transformers and Rotating Machines
4th Edition
ISBN: 9781305494817
Author: Stephen L. Herman
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Question
thumb_up100%
Need assistance with solving equation (3.69) to (3.70). I'm not understanding why output voltage is outside of parenthesis in equation 3.70 and the # 1 is on inside of parenthesis?
This is not part of a graded assignment, I'm reading through the chapters and solving examples.
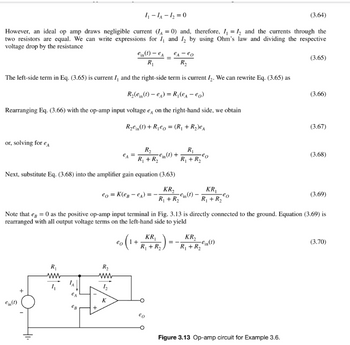
Transcribed Image Text:1₁-1A-1₂2=0
(3.64)
However, an ideal op amp draws negligible current (A=0) and, therefore, I₁=I₂ and the currents through the
two resistors are equal. We can write expressions for I and I₂ by using Ohm's law and dividing the respective
voltage drop by the resistance
ein (1) - eA
R₁
e₁ - eo
R₂
The left-side term in Eq. (3.65) is current I, and the right-side term is current I₂. We can rewrite Eq. (3.65) as
R₂(ein (1) - eA) = R₁ (e₁-eo)
Rearranging Eq. (3.66) with the op-amp input voltage e, on the right-hand side, we obtain
R₂ein (1) + R₁eo= (R₁ + R₂)еA
or, solving for e
R₂
R₁ + R₂
Next, substitute Eq. (3.68) into the amplifier gain equation (3.63)
KR₂
R₁ + R₂
ein (1)
R₁
www
eo = K(eBeA) =
+
R₂
www
12
K
ein (1) +
eo
R₁
R₁ + R₂
KR₁
R₁ + R₂,
eo
KR₁
R₁ + R₂
Note that eg = 0 as the positive op-amp input terminal in Fig. 3.13 is directly connected to the ground. Equation (3.69) is
rearranged with all output voltage terms on the left-hand side to yield
∙eo
KR₂
R₁ + R₂ in (1)
(3.65)
Figure 3.13 Op-amp circuit for Example 3.6.
(3.66)
(3.67)
(3.68)
(3.69)
(3.70)

Transcribed Image Text:The output voltage eo of the op amp shown in Fig. 3.12 is
eo = K(eB - eA)
(3.63)
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 1 steps

Knowledge Booster
Similar questions
- 2. Figure 1 shows a sensor consists of a core, an armature and a variable air gap. The core with 3.0 cm depth and 10000 relative permeability has 200 coil turns. On the other hand, the armature is a steel plate of thickness 0.2 cm and has similar relative permeability with the core. Assuming the relative permeability of air is unity and the permeability of free space is equal to 4x x 10-7 H.m, calculate the inductance of the sensor for air gaps of 0 mm and 2 mm. Please write the answers in 3 decimal places. 2.0 cm 1.0 cm 6.0 cm 3.0 cm 1.5 cm Figure Iarrow_forwardThe potentiometer Rx (adjustable resistor) in the given figure is to be designed to adjust current ix from 10 mA to 1 A. Calculate the values of R and Rx by setting the potentiometer to its minimum and maximum values. Assume V = 160 V. (NOTE: Choose a combination, which makes the potentiometer most sensitive.) V R ww Rx ww The value of R and Rx are Ω and kQ, respectively.arrow_forward5. The purpose of vibration absorber is to reduce vibration at resonance * Choose True Falsearrow_forward
- The equation underneath is do to with resonance, however resonance occurs when the input frequency is equal to the natural frequency what does this equation mean?arrow_forwardFigure 4 shows a simple apparatus for demonstrating resonance in a tube. A tube which is open at the top is filled with water and a vibrating tuning fork of unknown frequency is placed near the top of the tube. The water is allowed to flow through a valve, at the bottom of the tube. Tuning fork p water valve Figure 4 If, the first intensity in the sound is heard when the air column length is p. The next increase is heard when the air column length is y, and the last one is when the air column length is r. Draw the wave pattern happened at p, q and r. Label node and antinode. Calculate the respective frequencies.arrow_forwardvibrationsarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
 Electrical Transformers and Rotating MachinesMechanical EngineeringISBN:9781305494817Author:Stephen L. HermanPublisher:Cengage Learning
Electrical Transformers and Rotating MachinesMechanical EngineeringISBN:9781305494817Author:Stephen L. HermanPublisher:Cengage Learning Understanding Motor ControlsMechanical EngineeringISBN:9781337798686Author:Stephen L. HermanPublisher:Delmar Cengage Learning
Understanding Motor ControlsMechanical EngineeringISBN:9781337798686Author:Stephen L. HermanPublisher:Delmar Cengage Learning

Electrical Transformers and Rotating Machines
Mechanical Engineering
ISBN:9781305494817
Author:Stephen L. Herman
Publisher:Cengage Learning

Understanding Motor Controls
Mechanical Engineering
ISBN:9781337798686
Author:Stephen L. Herman
Publisher:Delmar Cengage Learning