
Algebra & Trigonometry with Analytic Geometry
13th Edition
ISBN: 9781133382119
Author: Swokowski
Publisher: Cengage
expand_more
expand_more
format_list_bulleted
Question
The data in Table 7E.3 give the number of nonconforming bearing and seal assemblies in samples of size 100. Construct a fraction nonconforming control chart for these data. If any points plot out of control, assume that assignable causes can be found and determine the revised control limits.
n=100;" " m=20;" " ∑_(i=1)^m▒D_i =117;" " p ̄=(∑_(i=1)^m▒D_i )/mn
"UC" "L" _p=p ̄+3√((p ̄(1-p ̄))/n)
"LC" "L" _p=p ̄-3√((p ̄(1-p ̄))/n)
Draw x-bar control chart and R chart
Remove the data that are not in control and recalculate UCL, CL, LCL and redraw control chart.
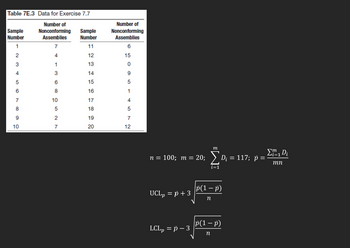
Transcribed Image Text:Table 7E.3 Data for Exercise 7.7
Number of
Sample
Nonconforming
Sample
Number of
Nonconforming
Number
Assemblies
Number
Assemblies
1
7
11
6
2
4
12
15
3
1
13
0
4
3
14
9
5
6
15
6
8
16
7
10
17
8
9
10
10
527
18
6145
5
19
7
20
12
m
D; = 117; p =
n = 100; m = 20; Σ D₁ =
i=1
ΣΤΟ
mn
p(1 − p)
UCL₂ = p +3
LCL₂ = p-3
n
|p(1 − p)
n
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 2 steps with 4 images

Knowledge Booster
Similar questions
- Use theorem 5.4.2 to form a proportion in which SV is a geometric mean. Hint: SVTRVS Exercises 1-6arrow_forwardFind the average rates of change of f(x)=x2+2x (a) from x1=3 to x2=2 and (b) from x1=2 to x2=0.arrow_forwardFind the values of x in each proportion: a x6=3x c 6x+4=2x+2 b x53=2x37 d x+35=x+57 e x2x5=2x+1x1 g x1x+2=103x2 f x(x+5)4x+4=95 h x+72=x+2x2arrow_forward
- The population P (in millions) of Texas from 2001 through 2014 can be approximated by the model P=20.913e0.0184t, where t represents the year, with t=1 corresponding to 2001. According to this model, when will the population reach 32 million?arrow_forwardSuppose that 1000 customers are surveyed and 850 are satisfied or very satisfied with a corporation's products and services. Test the hypothesis Ho:p 0.9 against H₁ :p 0.9ata = 0.095. Find the P-value. Reject ✓ Ho. The P-value is less than V (choose the least possible).arrow_forwardPlz all will ratearrow_forward
- P(1.15 < Z < 2.77) =arrow_forwardflx) = kx² (1-x) {kx 04 x 41 Mervise ther If the) is a p.d.f, Fund k b) Find the mean, mediany mede.arrow_forwardSuppose that Yt is the monthly value of the number of new home construction projects started in the United States. Because of the weather, Yt has a pronounced seasonal pattern; for example, housing starts are low in January and high in June. Let μJan denote the average value of housing starts in January and let μFeb, μMar,. . . . , μDec denote the average values in the other months. Show that the values of μJan, μFeb, . . . , μDec can be estimated from the OLS regression Yt = β0 + β1Febt + β2Mart + . . . .+ β11Dect + ut, where Febt is a binary variable equal to 1 if t February, Mart is a binary variable equal to 1 if t is March, and so forth.arrow_forward
- Suppose that 1000 customers are surveyed and 850 are satisfied or very satisfied with a corporation's products and services. Test the hypothesis Ho:p = 0.9 against H₁ :p # 0.9at a = 0.093. Find the P-value. Ho. The P-value is less than (choose the least possible).arrow_forwardCBS news reported that 4% of adult Americans have a food allergy consider selecting 10 adult Americans at random define the variable x as: x= number of people in the sample of 10 that have a food allergy a. p(x<3) b. p(x lesss than or equal to 3) c. p (x greater than or equal to 4) d. p (1 less than or equal to x less than or equal to 3)arrow_forwardA population of Emotional Quotient scores with μ = 38 and σ = 6 is standardized to create a new population with μ = 50 and σ = 10. Find the new score for a given original score or the old score for a given new score. original score new score X = 26 ? ? X = 65arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
- Algebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
 Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
Glencoe Algebra 1, Student Edition, 9780079039897...AlgebraISBN:9780079039897Author:CarterPublisher:McGraw Hill
 Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage Learning Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage

Glencoe Algebra 1, Student Edition, 9780079039897...
Algebra
ISBN:9780079039897
Author:Carter
Publisher:McGraw Hill


Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning

Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,