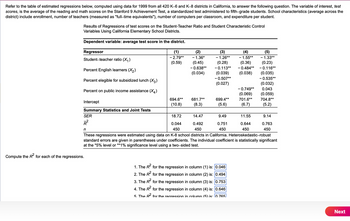
Refer to the table of estimated regressions below, computed using data for 1999 from all 420 K-6 and K-8 districts in California, to answer the following question. The variable of interest, test scores, is the average of the reading and math scores on the Stanford 9 Achievement Test, a standardized test administered to fifth-grade students. School characteristics (average across the district) include enrollment, number of teachers (measured as "full-time equivalents"), number of computers per classroom, and expenditure per student. Results of Regressions of test scores on the Student-Teacher Ratio and Student Characteristic Control Variables Using California Elementary School Districts. Dependent variable: average test score in the district. Regressor Student-teacher ratio (✗₁) Percent English learners (X2) Percent elegible for subsidized lunch (X3) Percent on public income assistance (X4) (1) - 2.79** (0.59) (2) - 1.36* (0.45) - 0.638** (0.034) (3) - 1.26** (0.28) - 0.113** (0.039) - 0.507** (0.027) (4) - 1.55** (0.36) - 0.484** (0.038) - 0.749** (0.069) (5) - 1.33** (0.23) - 0.116** (0.035) - 0.535** (0.032) Compute the R² for each of the regressions. 0.043 (0.059) 694.6** 681.7** 699.4** 701.6** 704.8** Intercept (10.8) (8.3) (5.6) (6.7) (5.2) Summary Statistics and Joint Tests SER 18.72 14.47 9.49 11.55 9.14 Ŕ² 0.044 0.492 0.751 0.644 0.763 n 450 450 450 450 450 These regressions were estimated using data on K-8 school districts in California. Heteroskedastic-robust standard errors are given in parentheses under coefficients. The individual coefficient is statistically significant at the *5% level or **1% significance level using a two-sided test. 1. The R² for the regression in column (1) is: 0.046 2. The R² for the regression in column (2) is: 0.494 3. The R² for the regression in column (3) is: 0.753 4. The R² for the regression in column (4) is: 0.646 5 The R²² for the regression in column (5) is: 0 765 Next 4. The R* for the regression in column (4) is: 0.646| 5. The R² for the regression in column (5) is: 0.765 (Round your response to three decimal places) Construct the homoskedasticity-only F-statistic for testing ẞ3 = ẞ4 = 0 in the regression shown in column (5). The homoskedasticity-only F-statistic for the test is: 255.66 (Round your response to two decimal places) Is the homoskedasticity-only F-statistic significant at the 5% level? A. Yes. B. No. Test B3 B40 in the regression shown in column (5) using the Bonferroni test. Note that the 1% Bonferroni critical value is 2.807. The t-statistic for ẞ3 in the regression in column (5) is: Is the Bonferroni test significant at the 1% level? A. Yes. B. No. (Round your response to three decimal places) The t-statistic for ẞ4 in the regression in column (5) is: (Round your response to three decimal places) Construct a 99% confidence interval for B₁ for the regression in column (5). The 99% confidence interval is: - 1.923 -0.737] (Round your response to three decimal places)

Help to solve this?

ect p.mheducation.com/ext/map/index.html?_con3con&external_browser%3D0&launchUrl=https%253A%252F%252Fnewconnect.mheducation.com%252F#/activity/question-group/... * D B homework i Saved Help Save & Exit Submit Check my work Required information [The following information applies to the questions displayed below.] Altira Corporation provides the following information related to its merchandise inventory during the month of August 2021: Aug.1 Inventory on hand–2,000 units; cost $5.30 each. 8 Purchased 8,000 units for $5.50 each. 14 Sold 6,000 units for $12.00 each. 18 Purchased 6,000 units for $5.60 each. 25 Sold 7,000 units for $11.00 each. 28 Purchased 4,000 units for $5.80 each. 31 Inventory on hand-7,000 units. Required: 1. Using calculations based on a perpetual inventory system, determine the inventory balance Altira would report in its August 31, 2021, balance sheet and the cost of goods sold it would report in its August 2021 income statement using the FIFO method. Cost of Goods…

Please avoid answers in image format thank you