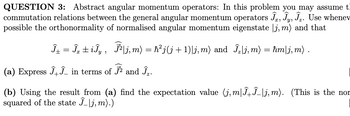
QUESTION 3: Abstract angular momentum operators: In this problem you may assume t commutation relations between the general angular momentum operators Ĵ, ĴÎy, Ĵz. Use whenev possible the orthonormality of normalised angular momentum eigenstate |j, m) and that α = αiĴy, J²|j,m) = ħ²j(j +1)|j,m) and Îz|j,m) = ħm|j,m) . (a) Express Ĵ+Ĵ_ in terms of №² and Ĵ2. (b) Using the result from (a) find the expectation value (j,m|Î+Î_|j,m). (This is the no squared of the state Î_|j,m).)

The coherent states for the one-dimensional harmonic oscillator are defined as eigenstates of the operatorof annihilation a (which is non-Hermitian):a |λ⟩ = λ |λ⟩ (1)where λ is a complex number in general.   a)prove that is a normalized consistent state. b)Show that the above state satisfies the minimum uncertainty relation, i.e., show that

I need the answer as soon as possible

The commutation relations among the angular momentum operators, Lx, Ly, Lz, and β, are distinctive and characteristic of all angular momentum systems. Define two new angular momentum operators as : Îx + ily Î_ = Îx – iÎy - Î+ 2 (a) Write the operator product, ÎÎ_, in terms of β and Î₂. Note: β = Îx² + Îy² + Îz². (b) Evaluate the commutator, [1²,1+]. (L+ is shorthand for Îx ± ily.) (c) Evaluate the commutator, [L₂,L+].

ext cenb. Consider a system whose states are given in term of complete and orthonormal set of kets |1>, |2 >, [3 >,14 > as follows: 1 1p >= |1 > + 기2 > +2|3 > + 기4 > i 214> Where the kets |n > are eigenstates of an observable A defined on the system as follows: 2 A]n > = na|n > with n = 1,2,3,4 and with a a constant number. have 4) eiyen vealue 1. If A is measured, which values will be found and with which probabilities? 2. Find the expectation value of A for the state |Ø >. 3. Assume that the state 14> is found after the measurement of A. If A is measured again immediately, which states will be found and with which probabilities? 4. Find the expectation value of A if the system is in the state |4 >. 5. Assume B another observable defined on the system, which is compatible with A. Write the uncertainty inequality between A and B. 6. If B is measured, which states will be found and with which probabilities?

please answer c) only 2. a) A spinless particle, mass m, is confined to a two-dimensional box of length L. The stationary Schrödinger equation is - +a) v(x, y) = Ev(x, y), for 0 < r, y < L. The bound- ary conditions on ý are that it vanishes at the edges of the box. Verify that solutions are given by 2 v(1, y) sin L where n., ny = 1,2..., and find the corresponding energy. Let L and m be such that h'n?/(2mL²) = 1 eV. How many states of the system have energies between 9 eV and 24 eV? b) We now consider a macroscopic box (L of order cm) so that h'n?/(2mL?) ~ 10-20 eV. If we define the wave vector k as ("", ""), show that the density of states g(k), defined such that the number of states with |k| between k and k +dk is given by g(k)dk, is Ak 9(k) = 27 c) Use the expression for g(k) to show that at room temperature the partition function for the translational energy of a particle in a macroscopic 2-dimensional box is Z1 = Aoq, where 2/3 oq = ng = mk„T/2nh?. Hence show that the average…

The Hamiltonian for the one dimensional quantum oscillator is 1 p² 1 Ĥ = 1² + ½ k²² = 12 + √ mw² ಠ2m 2m 2 where k = mw². 1) Define the operators ₁₁ and ₁₁ such that Ĥ = ½ħw (p² + ²). Define Ĥ2 as a function of 1 and p₁ such that Ĥ = hwĤ₂. - 2) Let us define the new operators â (1 + i₁) and ↠= ½(î₁ — ip₁). Express ₁ and p₁ as a function of â and â³. Knowing that [^^1,î₁] = i and [1, 1] = -i, calculate âât and â†â. Express Ĥ2 as a function of a and at. 3) Let us define Ñ such that Ĥ₂ = Ñ + ½. Knowing that Ĥ, Ĥ₂ and Ñ have the same eigenstates, what are their corresponding eigenvalues?

Consider the half oscillator" in which a particle of mass m is restricted to the region x > 0 by the potential energy U(x) = 00 for a O where k is the spring constant. What are the energies of the ground state and fırst excited state? Explain your reasoning. Give the energies in terms of the oscillator frequency wo = Vk/m. Formulas.pdf (Click here-->)

A qubit is in state |) = o|0) +₁|1) at time t = 0. It then evolves according to the Schrödinger equation with the Hamiltonian Ĥ defined by its action on the basis vectors: Ĥ0) = 0|0) and Ĥ|1) = E|1), where E is a constant with units of energy. a) Solve for the state of the qubit at time t. b) Find the probability to observe the qubit in state 0 at time t. Explain the result by referring to the way that the time-evolution transforms the Bloch sphere.